AP CALCULUS AB 2022 Exam Full Solution FRQ#6d
TLDRThe video script discusses the behavior of particle positions in a two-dimensional space as time \( T \) approaches infinity. The horizontal position \( X \) is given by the function \( 6 - 4e^{-T} \), and as \( T \) goes to infinity, \( X \) approaches 6 units away from the origin. The vertical position \( Y \) is determined by integrating a velocity function over time, resulting in \( Y \) approaching 3 units from the origin as \( T \) becomes infinite. The conclusion is that the particle in the \( X \) direction will eventually be farther from the origin compared to the \( Y \) direction, being six units away as opposed to three units for the \( Y \) particle.
Takeaways
- 📐 As time (T) approaches infinity, the position of the particle along the X-axis approaches 6 units away from the origin.
- 🚀 The X position is determined by the function (6 - 4e^{-T}), which tends to 6 as (T) tends to infinity.
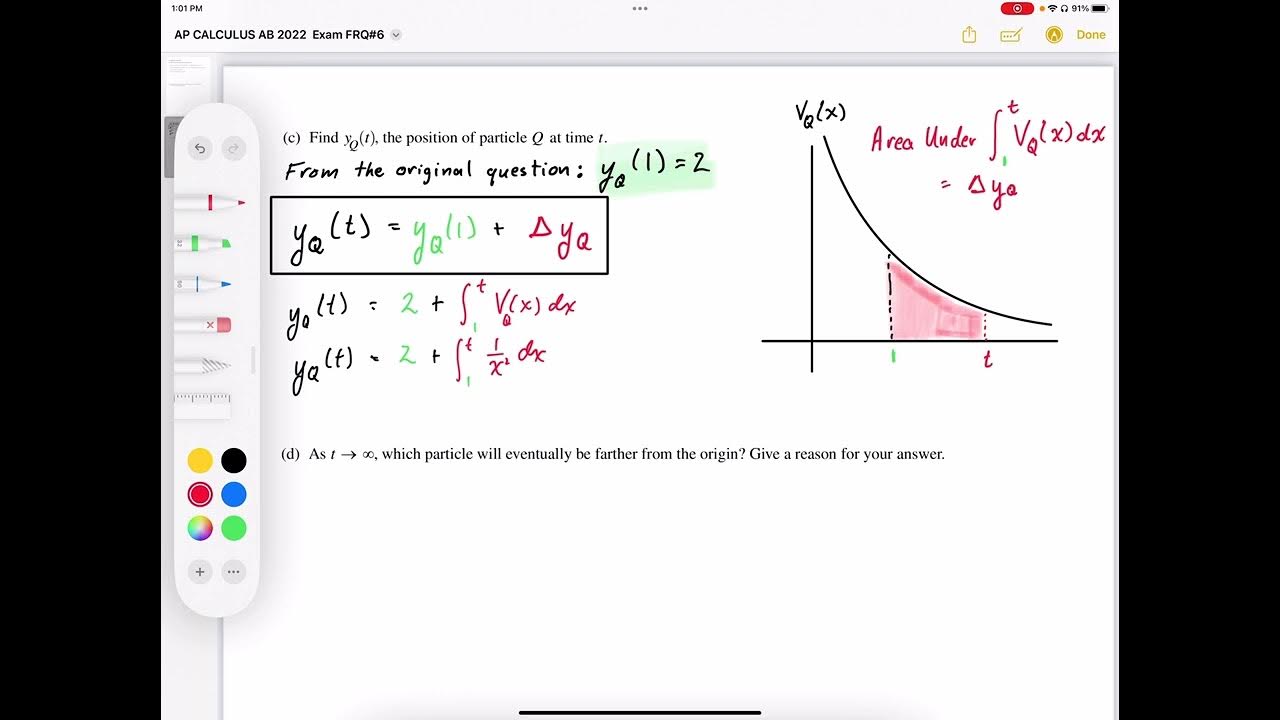
- 📉 The Y position is calculated using an antiderivative of a given function and approaches 3 units away from the origin as (T) tends to infinity.
- 🧮 The vertical position calculation involves integrating the velocity function and applying the limit as (T) goes to infinity.
- 🔢 The limit of the antiderivative (-1/X) from 1 to (T), as (T) approaches infinity, simplifies to (2 + (-1/infinity - (-1/1))), resulting in 3 for the Y position.
- 🏁 The particle that will be farther from the origin is the one moving along the X-axis, as it will be 6 units away compared to the Y particle at 3 units.
- ➗ The concept of limits is crucial in determining the behavior of functions as they extend to positive or negative infinity.
- ∫ The integration process is used to find the area under a curve, which in this case helps in determining the Y position as (T) goes to infinity.
- 🔁 The script involves a dynamic system where the position of a particle changes over time, represented by the variable (T).
- 📈 The X and Y positions are functions of time, and their behavior over time is analyzed to predict their long-term positions.
- 🤔 Understanding the implications of limits in calculus is essential for comprehending the ultimate positions of particles in motion.
- 📚 The transcript is a mathematical discussion that requires a good grasp of calculus concepts such as limits and integration.
Q & A
What happens to the X position as T approaches infinity?
-As T approaches infinity, the X position approaches 6 units away from the origin because the function given is 6 - 4e^(-t), and the limit of e^(-t) as T goes to infinity is zero.
What is the final Y position as T goes to infinity?
-The final Y position is 3 units away from the origin. This is determined by evaluating the limit of the antiderivative of the given function as T approaches infinity, which simplifies to 2 + (-1/∞ + 1/1), resulting in 3.
Which particle will be further from the origin as T goes to infinity?
-The particle associated with the X position will be further from the origin, as it approaches 6 units away compared to the Y particle, which approaches 3 units away.
How does the X position change with respect to time T?
-The X position changes according to the function 6 - 4e^(-t). As time T increases, the term -4e^(-t) approaches zero, causing the X position to get closer to 6.
What is the significance of evaluating the limit as T approaches infinity for the Y position?
-Evaluating the limit as T approaches infinity for the Y position is crucial to determine the final or limiting vertical position of the particle, which in this case is found to be 3 units from the origin.
What mathematical operation is used to find the area under the velocity curve?
-The area under the velocity curve is found using integration. Specifically, the antiderivative of the velocity function is evaluated from the bounds of 1 to T, where T approaches infinity.
How does the term 'e to the negative T' relate to the X position?
-The term 'e to the negative T' (e^(-t)) represents the exponential decay component of the X position function. As T increases, e^(-t) approaches zero, indicating that the X position stabilizes and moves closer to 6.
What does the term 'anti-differentiate' mean in the context of finding the Y position?
-To 'anti-differentiate' means to perform the reverse operation of differentiation, which is integration. It is used to find the Y position by integrating the given function over the specified interval.
Why is the limit of the antiderivative evaluated from 1 to infinity for the Y position?
-The limit from 1 to infinity is evaluated to find the total change in the Y position over an infinite time span, which gives the final displacement or the final Y position of the particle.
What is the role of the exponential function e^(-t) in the context of the problem?
-The exponential function e^(-t) models a decaying process over time. In the context of this problem, it is part of the functions describing the X and Y positions of a particle, influencing how these positions change as time T increases.
How does the concept of limits apply to the positions of the particle as T goes to infinity?
-The concept of limits is used to determine the behavior of the particle's positions as time extends indefinitely. It allows us to find the values that the X and Y positions approach as T becomes extremely large.
Outlines
🚀 Particle Position Analysis as T Approaches Infinity
The paragraph discusses the behavior of a particle's position relative to the origin as time T tends to infinity. It focuses on the X and Y coordinates of the particle's position. For the X position, the function given is six minus 4e to the power of negative T. By taking the limit as T approaches infinity, it is determined that X approaches 6, meaning the particle moves closer to six units away from the origin. For the Y position, the function is evaluated similarly, and it is found that Y approaches 3, indicating the particle moves closer to three units away from the origin. The particle that will be farther from the origin is the one with the X coordinate, as it ends up six units away compared to the Y coordinate, which is three units away.
Mindmap
Keywords
💡Infinity
💡Particle
💡Origin
💡X position and Y position
💡Limit
💡Anti-differentiate
💡Velocity curve
💡Displacement
💡Function
💡E to the negative T
💡Bounds
Highlights
As T goes to infinity, the position X approaches 6 units away from the origin.
The X position is calculated using the function 6 - 4e^(-t) and taking the limit as T approaches infinity.
The Y position approaches 3 units away from the origin as T goes to infinity.
The Y position is found by anti-differentiating the 1/X function and evaluating the limit as T approaches infinity.
The particle in the X direction will eventually be further from the origin compared to the Y direction.
The X particle will be 6 units from the origin, while the Y particle will be 3 units from the origin as T goes to infinity.
The transcript discusses the behavior of particle positions as time T approaches infinity in a mathematical context.
The position of the X particle is determined by evaluating the limit of a given exponential function.
The position of the Y particle is determined by anti-differentiating a function and evaluating its limit as T approaches infinity.
The transcript provides a step-by-step explanation of how to calculate the positions of particles in the X and Y directions.
The mathematical process involves taking limits, anti-differentiating functions, and evaluating expressions at infinity.
The transcript concludes that the X particle will be further from the origin than the Y particle at infinite time.
The X position calculation involves the exponential function e^(-∞) which approaches zero.
The Y position calculation involves the antiderivative of 1/X and evaluating it at bounds from 1 to infinity.
The transcript uses mathematical notation and concepts to explain the behavior of particle positions over time.
The final positions of the particles are determined by evaluating limits and anti-derivatives of given functions.
The X and Y positions of the particles are calculated independently and their behavior as T approaches infinity is analyzed.
The transcript provides a clear, concise explanation of the mathematical process used to determine the particle positions.
The conclusion that the X particle will be further from the origin is based on the calculated positions as T approaches infinity.
Transcripts
Browse More Related Video

2022 AP Calculus AB Exam FRQ #6

2022 AP Calculus AB Free Response #6

AP CALCULUS AB 2022 Exam Full Solution FRQ#6(a,b)
AP CALCULUS AB 2022 Exam Full Solution FRQ#6c

Rectilinear Motion or Particle Motion or Straight Line Motion!

Worked example: Motion problems with derivatives | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: