Math 1325 Lecture 11 5 - Applications
TLDRThe lecture covers the relationship between price and demand in the context of business and economics. It explains how as price increases, demand typically decreases, and vice versa, a concept known as elastic demand. The lecture introduces a formula for calculating elasticity, represented by the Greek letter 'eta', which is crucial for understanding consumer responsiveness to price changes. Using examples, the lecture demonstrates how to calculate elasticity and discusses its implications for business strategy, such as setting prices to optimize revenue. The material also touches on inelastic demand, where demand does not significantly change with price variations, using the example of insulin for diabetics. The lecture concludes with a more complex problem involving implicit differentiation and emphasizes the relationship between elasticity, price changes, and revenue, highlighting the importance of understanding these concepts for business decision-making.
Takeaways
- 📈 The relationship between price and demand is generally inverse; as price increases, demand decreases, and vice versa.
- 📉 Elastic demand refers to a situation where demand is highly responsive to price changes, while inelastic demand shows minimal change in demand despite price fluctuations.
- 💊 Examples of inelastic demand include essential goods like medicine, where the need for the product is more critical than its price.
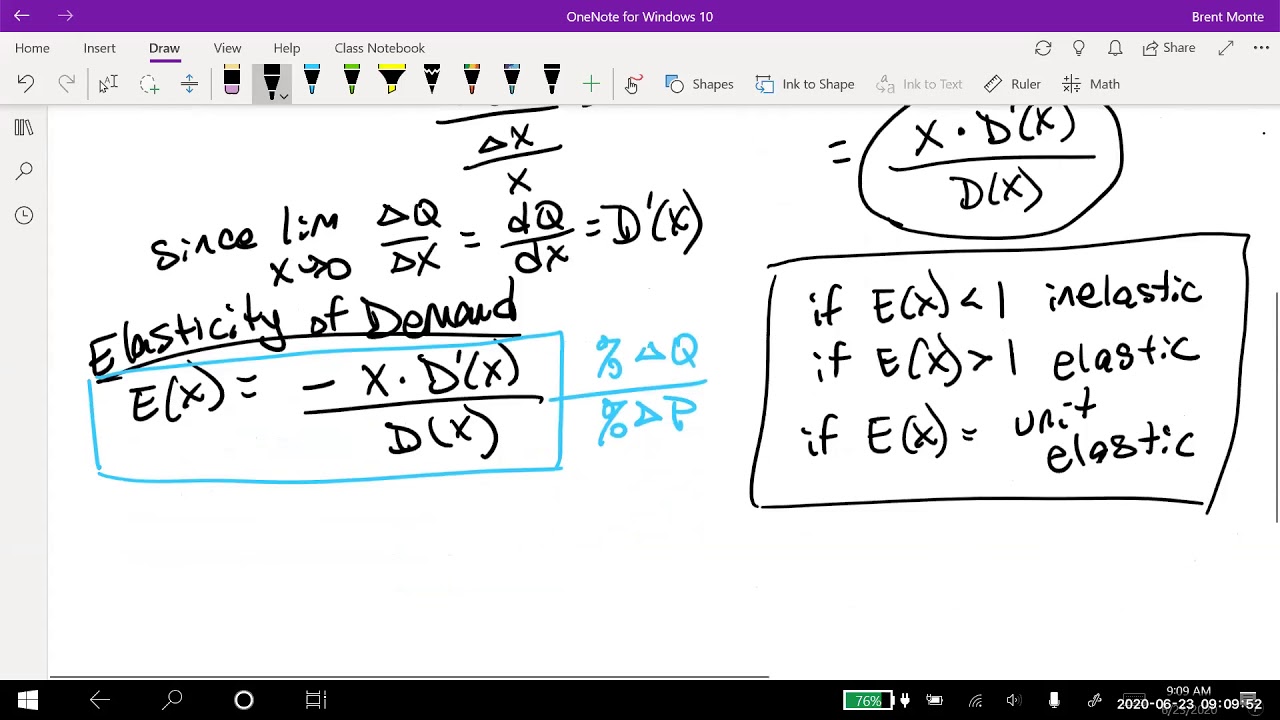
- 🧮 The elasticity of demand is quantified using a formula involving the derivative of quantity with respect to price, represented as \( \text{elasticity} = -\frac{P}{Q} \times \frac{dQ}{dP} \).
- 📌 To find the elasticity at specific points, you first determine the derivative of the quantity with respect to price, then use the price and quantity at those points in the elasticity formula.
- 🔍 When the elasticity is greater than one, demand is considered elastic, indicating that the percentage decrease in quantity demanded is greater than the percentage increase in price.
- 🔢 If elasticity equals one, it is known as unitary elasticity, which is the optimal point for setting a price to balance price increases and demand decreases.
- 📉 When elasticity is less than one, demand is inelastic, meaning that changes in price have a smaller relative impact on the quantity demanded.
- 💰 Revenue is related to price and elasticity, and it can be maximized at the unitary elasticity point, where an increase or decrease in price does not change revenue.
- ∫ Revenue can be calculated as price times quantity, and its change with respect to price is given by \( \text{revenue} = Q \times (1 - \text{elasticity}) \).
- 📚 For those interested in accounting, understanding how to maximize tax revenue using elasticity concepts is beneficial, although it is not tested in the course.
Q & A
What is the relationship between price and demand for a product?
-The relationship between price and demand is typically inverse; as the price of a product increases, demand generally decreases, and vice versa. This is because higher prices can make a product less affordable to consumers, while lower prices can increase the affordability and thus the number of consumers willing to buy the product.
What is meant by 'elastic demand' in the context of economics?
-Elastic demand refers to a situation where the demand for a product is highly responsive to changes in its price. If demand is elastic, a small change in price will result in a proportionally larger change in the quantity demanded.
Can you explain the concept of 'inelastic demand'?
-Inelastic demand occurs when the quantity demanded of a product does not change significantly in response to changes in price. This is often the case with essential goods or services, such as medicine, where consumers continue to purchase the product regardless of price because they need it.
How is the elasticity of demand calculated?
-The elasticity of demand is calculated using the formula: ε = (-dQ/dP) * (P/Q), where ε is the elasticity, dQ/dP is the derivative of quantity with respect to price, P is the price, and Q is the quantity.
What does it mean if the elasticity of demand is greater than one?
-If the elasticity of demand is greater than one, it indicates that the demand is elastic. This means that the percentage decrease in quantity demanded is greater than the percentage increase in price, making consumers highly responsive to price changes.
What is the implication if the elasticity of demand is less than one?
-If the elasticity of demand is less than one, it suggests that the demand is inelastic. In this case, the percentage change in quantity demanded is less than the percentage change in price, implying that the quantity demanded does not vary much with price changes.
At what point is the revenue maximized in relation to price and demand?
-Revenue is maximized at the point where the demand is unitary elastic, which is when the elasticity of demand equals one. At this point, an increase or decrease in price does not change the total revenue.
How does the revenue respond when the demand is elastic?
-When demand is elastic, an increase in price leads to a proportionally larger decrease in quantity demanded, which results in a decrease in revenue. Conversely, a decrease in price leads to a proportionally larger increase in quantity demanded, increasing revenue.
How does the revenue respond when the demand is inelastic?
-When demand is inelastic, an increase in price leads to a smaller decrease in quantity demanded, which results in an increase in revenue. Similarly, a decrease in price leads to a smaller increase in quantity demanded, which may not be enough to offset the reduced price, potentially leading to a decrease in revenue.
What is the role of implicit differentiation in finding the elasticity of demand?
-Implicit differentiation is used when the demand function is not explicitly solved for quantity (Q) in terms of price (P). It allows us to find the derivative dQ/dP, which is necessary for calculating the elasticity of demand when the demand function is given implicitly.
Why is it important to understand the concept of elasticity in the context of tax revenue?
-Understanding elasticity is crucial for tax revenue because it helps policymakers determine the optimal tax rates to maximize revenue without discouraging economic activity. It's a tool to predict how changes in tax rates will affect the taxable base and overall revenue collected.
Outlines
📚 Introduction to Price, Demand, and Elasticity Concepts
This paragraph introduces the lecture on the application of mathematical concepts to business and economics, focusing on the relationship between price and demand. It explains the intuitive nature of these concepts, where an increase in price typically leads to a decrease in demand and vice versa. The paragraph also distinguishes between elastic and inelastic demand, using examples like the iPhone and insulin to illustrate these points. The elasticity of demand is quantified using a formula involving the derivative of quantity with respect to price, and a simple demand function is used to demonstrate how to calculate elasticity at different price points.
🔍 Calculating Elasticity and Understanding its Implications
The second paragraph delves deeper into calculating the elasticity of demand using the formula provided and discusses the implications of different elasticity values on pricing strategies. It explains that when elasticity is greater than one, demand is considered elastic, meaning that a percentage change in price results in a larger percentage change in demand. Conversely, when elasticity is less than one, demand is inelastic, indicating that demand is less responsive to price changes. The paragraph also clarifies that elasticity is not the same as the derivative or the slope of the demand function, but rather a measure of consumer responsiveness to price changes. It concludes with a more complex problem that requires the use of implicit differentiation to find the elasticity of demand.
📈 Revenue, Elasticity, and Pricing Strategies
This paragraph explores the relationship between revenue and elasticity, explaining how changes in price affect revenue differently depending on whether demand is elastic or inelastic. It presents a formula that relates the change in revenue to price, quantity, and elasticity. The paragraph also discusses how to identify points of unitary elasticity, where revenue is maximized, and how to determine intervals of elastic and inelastic demand using the demand function. It concludes with a brief mention of a more complex topic of maximizing tax revenue, which is not covered in detail due to its complexity and specificity to accounting.
📊 Analyzing Demand Elasticity and Revenue Maximization
The final paragraph focuses on identifying different types of elasticity (unitary, inelastic, and elastic) and determining where revenue increases or decreases. It guides through the process of finding the point at which demand is unitary elastic by setting the elasticity to one and solving for quantity. The paragraph also explains how to find intervals of inelastic and elastic demand and how these relate to revenue changes. It emphasizes that revenue is maximized at unitary elasticity and provides a graphical representation to illustrate these concepts. Lastly, it touches on the application of these ideas in the context of maximizing tax revenue, a topic that is recommended for those interested in accounting.
Mindmap
Keywords
💡Price
💡Demand
💡Elasticity
💡Derivative
💡Revenue
💡Implicit Differentiation
💡Unitary Elastic Demand
💡Inelastic Demand
💡Economic Formula
💡Optimizing Revenue
💡Tax Revenue Maximization
Highlights
The lecture discusses the relationship between price and demand in business and economics
As price increases, demand decreases, and vice versa
Elastic demand means demand is 'stretchy' and changes significantly with price
Inelastic demand means demand does not change much with price changes, like for life-saving medicines
Economists use a formula with elasticity (Greek letter epsilon) to quantify demand elasticity
The elasticity formula is elasticity = - (Price/Quantity) * (dQuantity/dPrice)
Example: Elasticity calculated for different prices using a demand function P + 5Q = 100
Elasticity > 1 means demand is elastic, % decrease in demand > % increase in price
Elasticity < 1 means demand is inelastic, demand does not change much with price changes
Elasticity = 1 is called unitary elasticity, optimal point to set price to balance price and demand
Revenue and elasticity are related - if elasticity > 1, increasing price decreases revenue, and vice versa
Revenue is maximized at unitary elasticity where elasticity equals 1
Example using implicit differentiation to find elasticity for demand function P = 1000Q^-2
Identifying intervals of elastic, inelastic and unitary elastic demand using elasticity
Revenue increases when demand is elastic, decreases when demand is inelastic
Graphing the revenue function from the demand function can visualize these relationships
Maximizing tax revenue involves a similar process of analyzing demand and supply
The lecture provides a comprehensive overview of price, demand, and elasticity in business economics
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: