Elasticity of Demand - Example
TLDRIn this video, Professor Imani delves into the concept of elasticity in economics, specifically price elasticity of demand. She explains elasticity as the percentage change in quantity demanded over the percentage change in price. Using a simple demand function, 200 - 5p, she demonstrates how to calculate the elasticity (E of P) at a given price. The formula involves multiplying the negative price by the derivative of the demand function and dividing by the original demand function. After simplifying, she finds that the elasticity function is P/(40 - P). By plugging in a price of $25, she calculates an elasticity of approximately 1.67, which is considered elastic. This means that a 1% increase in price would lead to a 1.67% decrease in quantity demanded. The video concludes with a discussion on the implications of elasticity for revenue maximization: if E of P > 1 (elastic), it's better to decrease price; if E of P < 1 (inelastic), increasing price is advisable; and if E of P = 1 (unit elastic), the current price maximizes revenue. Professor Imani encourages viewers to explore more on the topic through her linked resources, including a playlist of calculus videos and a subscription to her channel for further learning.
Takeaways
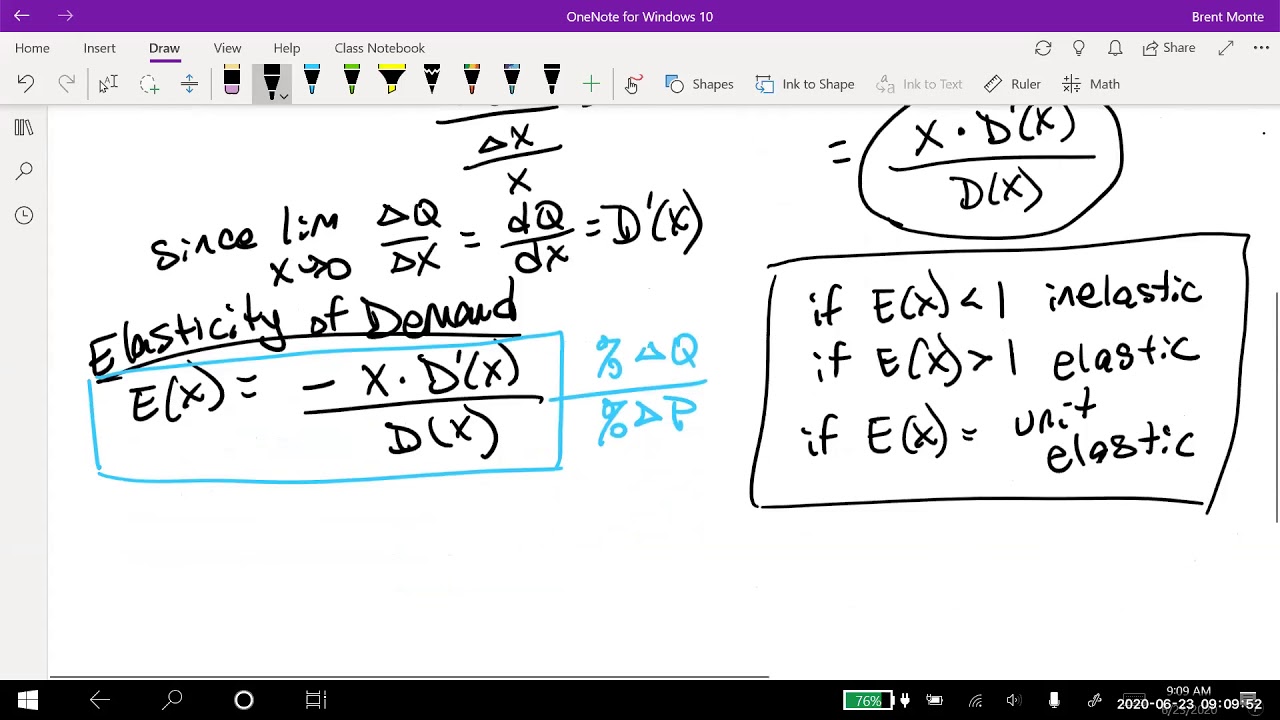
- 📚 Elasticity (E of P) is represented by the formula E(P) = -P * (dQ/dP) / (Q/P), where P is the price and Q is the quantity demanded.
- 🔢 The elasticity formula measures the percentage change in quantity demanded over the percentage change in price.
- 📉 When calculating elasticity, the negative sign indicates that price and quantity demanded move in opposite directions.
- 🌟 For the demand function Q = 200 - 5P, the derivative dQ/dP is -5, which is used in the elasticity formula.
- ➗ Simplifying the elasticity formula for the given demand function results in E(P) = P / (40 - P).
- 🔍 At a price P = 25, the elasticity E(25) is calculated to be 5/3, or approximately 1.67.
- 📈 An elasticity value greater than 1 indicates that the demand is elastic, suggesting that a price increase will lead to a proportionally larger decrease in quantity demanded.
- 📉 Conversely, if elasticity is less than 1, demand is inelastic, meaning a price increase will lead to a smaller proportional decrease in quantity demanded, potentially increasing revenue.
- 🔳 When elasticity equals 1, the demand is unit elastic, which is the point of maximum revenue.
- 💡 To maximize revenue, one should consider the elasticity of demand when deciding on pricing strategies.
- 📚 For more theoretical understanding, the professor recommends watching a linked video and checking out a playlist of calculus videos.
- 🔗 The professor also encourages subscribing to the channel for more educational content on calculus, statistics, and algebra.
Q & A
What is the formula for price elasticity of demand?
-The formula for price elasticity of demand is given as e of p, which is equal to negative p times the derivative of the demand function with respect to p, divided by the original demand function itself.
What does the term 'elasticity' represent in economics?
-Elasticity in economics represents the percentage change in quantity demanded over the percentage change in price. It measures how responsive the quantity demanded is to a change in price.
What is the demand function used in the example?
-The demand function used in the example is 200 - 5p, where p represents the price.
How is the derivative of the demand function calculated in the example?
-The derivative of the demand function (200 - 5p) is calculated as the derivative of 200 minus 5 times the derivative of p, which results in -5.
What is the simplified form of the elasticity function e of p?
-The simplified form of the elasticity function e of p is p divided by (40 - p), after dividing both the numerator and the denominator by 5.
At what price does the elasticity equal approximately 1.67?
-The elasticity equals approximately 1.67 when the price (p) is set to $25.
What does an elasticity value greater than one indicate?
-An elasticity value greater than one indicates that the demand is elastic. This means that the percentage change in quantity demanded is greater than the percentage change in price, so increasing the price would decrease revenue.
What is the implication of an elasticity value less than one?
-An elasticity value less than one indicates that the demand is inelastic. This means that the percentage change in quantity demanded is less than the percentage change in price, so increasing the price would increase revenue.
What is the term used for an elasticity value equal to one?
-An elasticity value equal to one is termed as 'unit elastic'. At this point, the percentage change in quantity demanded is equal to the percentage change in price, and it represents the condition for maximum revenue.
How does the concept of elasticity help in pricing strategy?
-The concept of elasticity helps in pricing strategy by determining the optimal price point to maximize revenue. If demand is elastic, a lower price is preferred, while for inelastic demand, a higher price can be set to increase revenue.
What does the professor suggest doing for more theoretical understanding of elasticity?
-The professor suggests watching another video that she will link at the end of the current video for a more theoretical understanding of where elasticity comes from and its significance.
What additional resources does the professor offer for those interested in further learning?
-The professor offers a playlist of all her calculus videos, and encourages subscribing to her channel for more content on calculus, statistics, and algebra.
Outlines
📚 Introduction to Elasticity in Economics
Professor Imani begins by introducing the concept of elasticity (e of p) in economics, which is a measure of how sensitive the quantity demanded is to a change in price. The formula for elasticity is given as negative p times the derivative of the demand function (d prime of p) over the original demand function (d of p). The professor emphasizes that elasticity is the percentage change in quantity over the percentage change in price. An example is provided using a simple demand function, 200 - 5p, and the elasticity is calculated as p/(40 - p). The professor then interprets the elasticity value at a price of $25, which is approximately 1.67, indicating that a 1% increase in price would lead to a 1.67% decrease in quantity demanded. This is further explained in terms of revenue and the concept of elasticity being greater than one, which is referred to as elastic. The professor suggests that if elasticity is greater than one, it's better to decrease the price to maximize revenue.
🔍 Elasticity and Its Impact on Pricing Strategy
The second paragraph delves deeper into the implications of different elasticity values on pricing strategy. If the elasticity of demand (e of p) is less than one, the demand is considered inelastic, meaning that a 1% increase in price leads to less than a 1% decrease in quantity demanded. In this case, increasing the price is beneficial as it leads to higher revenue. When elasticity equals one, the demand is unit elastic, and any price change does not affect total revenue. The professor provides a hypothetical example where increasing the price by 1% results in a 0.67% decrease in quantity demanded, which is advantageous for revenue. The video concludes with an encouragement to continue learning and a prompt to subscribe for more educational content on calculus, statistics, and algebra.
Mindmap
Keywords
💡Elasticity
💡Demand Function
💡Derivative
💡Price
💡Quantity Demanded
💡Revenue
💡Elastic vs. Inelastic
💡Unit Elastic
💡Percentage Change
💡Theoretical Understanding
💡Calculus
Highlights
Elasticity is defined as e of p, representing the percentage change in quantity over the percentage change in price.
The formula for price elasticity of demand is negative p times the derivative of the demand function over the original demand function.
For a demand function d(p) = 200 - 5p, the derivative is -5, which is used to calculate elasticity.
Elasticity calculation results in the formula 5p / (200 - 5p) after substituting the demand function into the elasticity formula.
Simplifying the elasticity formula by dividing by 5 gives p / (40 - p).
At a price of $25, the elasticity is calculated to be 5/3, or approximately 1.67.
An elasticity value greater than one indicates the demand is elastic, suggesting that a price increase will lead to a proportionally larger decrease in quantity demanded.
If elasticity is less than one, the demand is inelastic, meaning a price increase will result in a smaller percentage decrease in quantity demanded, potentially increasing revenue.
An elasticity of exactly one is termed unit elastic, which is the point of maximum revenue.
The concept of elasticity is crucial for understanding how changes in price affect revenue and quantity demanded.
The video provides a practical example to illustrate the calculation and interpretation of price elasticity.
The professor links to another video for a more theoretical discussion on elasticity.
A playlist of calculus videos is available for further study.
The channel also offers videos on statistics and algebra for a broader educational experience.
The video encourages viewers to subscribe for more content on calculus and related subjects.
The importance of continuous learning and practice in grasping the concept of elasticity is emphasized.
The video concludes with a motivational message to keep working towards understanding elasticity.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: