Elasticity of Demand

PClark Calc

14 Apr 202010:10

EducationalLearning

32 Likes 10 Comments

TLDRIn this video, Pete Clark discusses the concept of elasticity of demand, a business calculus application that measures how demand reacts to price changes. Using the demand function D = 100 - P^2, he demonstrates how to calculate elasticity at a given price point, such as $5, resulting in an inelastic demand (2/3). He explains the implications for revenue and how to interpret elasticity values, including inelastic (<1), elastic (>1), and unitary (=1). The video also covers how to find the optimal market price for maximum revenue by setting elasticity to one, exemplifying the practical use of calculus in business decision-making.

Takeaways

📚 The video is a practical calculus lesson focusing on the business application of elasticity of demand.
📈 Elasticity of demand measures the sensitivity of the quantity demanded to a change in price.
🔍 The demand function in the example is given as 100 - P^2, illustrating an inverse relationship between price and demand.
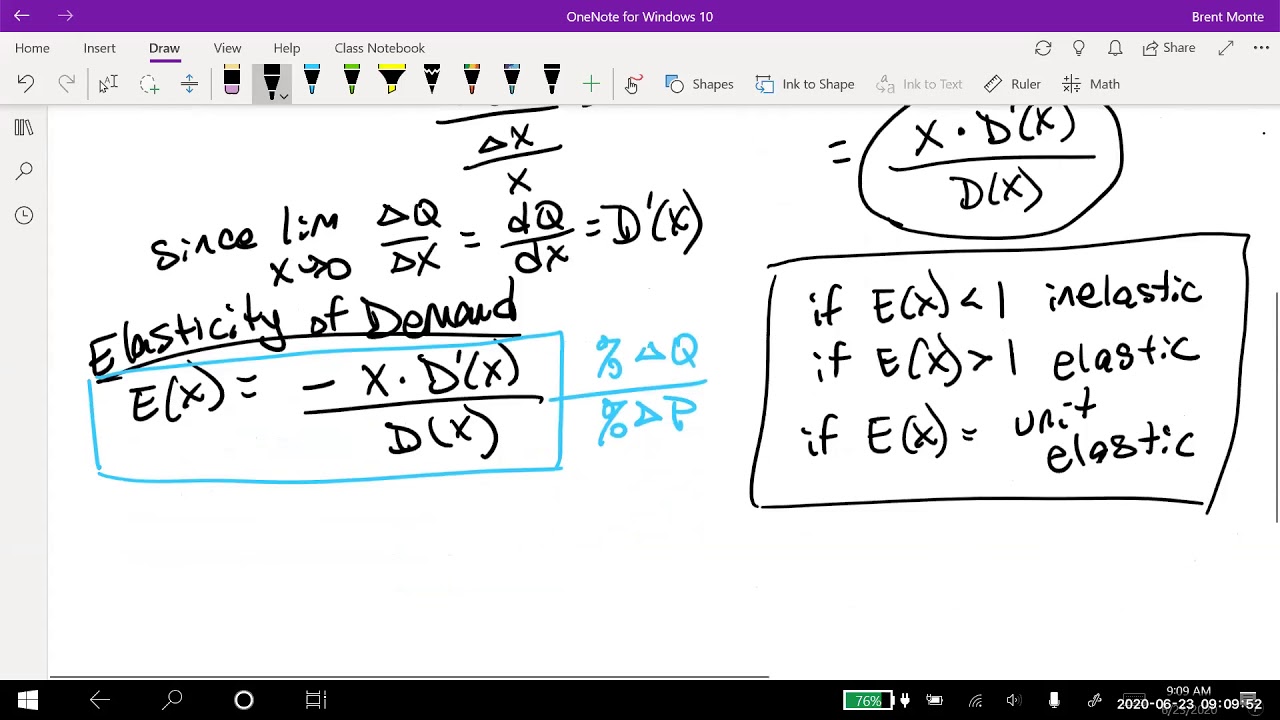
🧩 The formula for elasticity of demand as a function of price is negative P times the derivative of demand (D') divided by the demand (D).
📉 The negative sign in the formula is a convention to reflect the inverse relationship between price and demand.
🔑 The elasticity formula is derived from the ratio of the relative rate of change of demand to the relative rate of change of price.
📝 To calculate elasticity at a specific point, use the derivative of the demand function and evaluate it at that point.
💰 If elasticity is less than one (inelastic demand), raising the price can increase revenue because demand doesn't decrease proportionally.
🛒 For elastic demand, where elasticity is greater than one, a price cut can lead to a proportionally larger increase in demand, potentially increasing revenue.
🔢 At the point where elasticity equals one, the demand is perfectly responsive to price changes, indicating a point of maximum revenue.
✂️ To find the optimal price for maximum revenue, set elasticity to one and solve for the price variable.
📉 The video provides an example calculation of elasticity at two different prices, demonstrating how to interpret the results for business decisions.

Q & A

What is the primary topic discussed in the video?
-The primary topic discussed in the video is the concept of elasticity of demand in the context of business calculus, specifically how it is calculated and interpreted.
What is elasticity of demand?
-Elasticity of demand measures the responsiveness of the quantity demanded of a good to a change in its price. It is a way to quantify how sensitive demand is to price changes.
What is the demand function given in the example?
-The demand function given in the example is D = 100 - P^2, where D represents the quantity demanded and P represents the price.
How is the formula for elasticity of demand derived?
-The formula for elasticity of demand is derived from the ratio of the relative rate of change of demand to the relative rate of change of price, adjusted by a negative sign to give it a positive value as per economic convention.

Outlines

00:00

📚 Introduction to Elasticity of Demand in Business Calculus

This paragraph introduces the concept of elasticity of demand in the context of business calculus. It explains that elasticity measures how sensitive the demand for a product is to changes in its price. The demand function given is D = 100 - P^2, illustrating an inverse relationship between price and demand. The formula for price elasticity of demand is introduced as -P * (D'/D), where D' is the derivative of demand with respect to price, and the negative sign is a convention to give elasticity a positive value. The paragraph also explains the economic implications of different elasticity values, such as inelastic demand (elasticity < 1) and elastic demand (elasticity > 1).

05:01

💰 Understanding Elasticity's Impact on Pricing Strategy

This paragraph delves into the implications of elasticity values for pricing strategies. It uses the example of a product with an elasticity of 2/3 at a market price of $5, indicating inelastic demand. The paragraph explains that increasing the price would not significantly reduce demand, thus potentially increasing revenue. It contrasts this with elastic demand, where a price cut could significantly boost demand. The concept of using elasticity to find the optimal price for maximum revenue is also discussed, with the example of setting elasticity to 1 to solve for the price that maximizes revenue.

10:02

🔍 Applying Elasticity to Real-World Market Scenarios

The final paragraph discusses the application of elasticity in real-world market scenarios. It provides examples of inelastic and elastic demand, such as commodities like gasoline and oil versus luxury items like furniture and jewelry. The paragraph also mentions the use of elasticity to determine the optimal market price for maximizing revenue, using the derived formula from setting elasticity to 1. The speaker, Pete Clark, invites viewers to explore his textbooks for more information on calculus applications in business.

Mindmap

Keywords

💡Elasticity of Demand

Elasticity of demand is a measure of how sensitive the quantity demanded of a good is to a change in its price. It is a fundamental concept in economics that helps to understand consumer behavior. In the video, it is used to analyze how changes in the unit price of a product affect the quantity of that product that consumers are willing to purchase. The script provides the formula for calculating elasticity of demand and discusses its implications for business decisions, such as setting prices to maximize revenue.

💡Derivative

In calculus, a derivative represents the rate at which a function changes with respect to its variable. In the context of the video, the derivative is used to find the rate at which demand changes with respect to price, which is essential for calculating the elasticity of demand. The script explains that the derivative of the demand function is crucial for understanding how the demand for a product reacts to price changes.

💡Business Calculus

Business calculus is the application of calculus to solve problems in business and economics. It involves using concepts like derivatives to understand and predict changes in variables such as demand, cost, and revenue. The video specifically discusses a business calculus application, the elasticity of demand, which is used to make informed decisions about pricing strategies in a business setting.

💡Demand Function

A demand function is a mathematical relationship that expresses the quantity demanded of a good or service as a function of its price and possibly other variables. In the video, the demand function is given as 100 - P^2, where P is the price. This function is used to illustrate the inverse relationship between price and demand, which is a key concept in understanding market behavior.

💡Unit Price

Unit price refers to the cost per unit of a product or service. In the video, the unit price is the independent variable in the demand function, and changes in the unit price are analyzed to determine their impact on demand. The script uses the unit price to demonstrate how elasticity of demand is calculated and interpreted.

💡Inelastic Demand

Inelastic demand occurs when the quantity demanded of a good is relatively unresponsive to a change in its price. The video explains that if the elasticity of demand is less than one, the demand is considered inelastic. This means that a change in price will result in a smaller proportional change in demand, which has implications for pricing strategies aimed at maximizing revenue.

💡Elastic Demand

Elastic demand is the opposite of inelastic demand, where the quantity demanded of a good is highly responsive to a change in its price. If the elasticity of demand is greater than one, the demand is elastic. The video uses this concept to discuss how a price cut could lead to a proportionally larger increase in demand, which can be used to formulate pricing strategies.

💡Revenue

Revenue is the total income that a company generates from the sale of its goods or services. In the context of the video, revenue is discussed in relation to the elasticity of demand. The script explains that understanding the elasticity of demand can help businesses determine whether raising or lowering prices will increase or decrease their revenue.

💡Optimal Price

The optimal price is the price point at which a business can maximize its revenue. The video discusses how elasticity of demand can be used to find the optimal price. By setting the elasticity of demand equal to one, the video demonstrates how to calculate the price that would result in the maximum revenue for a business.

💡Polynomial Function

A polynomial function is a mathematical expression involving a sum of powers in a single variable. In the video, the demand function given is a polynomial function, 100 - P^2. This function is used to illustrate the concept of elasticity of demand and to perform the necessary calculus to calculate the derivative and subsequently the elasticity at different prices.

💡Commodities

Commodities are basic goods used in commerce that are interchangeable with other goods of the same type. The video mentions commodities as examples of products that often have inelastic demand. This means that consumers will continue to purchase these essential goods even if the price increases, making them less sensitive to price changes.

💡Marginal Revenue

Marginal revenue is the additional revenue that a company generates from selling one more unit of a good or service. While not explicitly defined in the script, the concept is alluded to when discussing the relationship between price, demand, and revenue. The video suggests that elasticity can indirectly be used to maximize revenue, which is related to understanding marginal revenue.

Highlights

Introduction to the concept of elasticity of demand in business calculus.

Elasticity of demand measures the reaction of demand to a change in price.

Demand function is presented as 100 minus P squared, illustrating the inverse relationship between price and demand.

Definition of elasticity formula using the derivative of the demand function.

Elasticity is the ratio of the relative rate of change of demand to the relative rate of change of price.

The negative sign in the elasticity formula is a convention to avoid negative values in economic discussions.

Calculating elasticity for a given point in the market using the derivative of the demand function.

Example calculation of elasticity at a market price of $5, resulting in an elasticity of 2/3.

Interpretation of elasticity values to determine the type of demand: inelastic, elastic, or unitary.

Implications of inelastic demand on pricing strategy to increase revenue.

Explanation of elastic demand and its impact on pricing and revenue.

Use of elasticity to find the optimal market price for maximizing revenue.

Calculation of elasticity at a market price of $8, showing an elastic market.

Determination of the optimal market price using the elasticity function set to one.

Practical applications of elasticity in various products and commodities.

Availability of Pete Clark's textbooks on Amazon for further study on calculus applications.

Transcripts

Browse More Related Video

Elasticity of Demand - Example

Math 1325 Lecture 11 5 - Applications

Math 11 - Section 3.7

3.7 - Elasticity of Demand

What is Elasticity?

Y1 10) Price Elasticity of Demand - PED