Math 11 - Section 3.7

The video begins with an introduction to the concept of elasticity of demand in economics. It explains that elasticity measures how the quantity demanded changes in response to price changes. The formula for elasticity is derived, showing it as the percentage change in quantity demanded over the percentage change in price. The video uses algebra to simplify the formula, resulting in the expression involving the derivative of quantity with respect to price (dQ/dx), divided by the quantity demanded (Q). The presenter emphasizes that the elasticity value can be positive or negative, but in economics, it's often presented as a positive number to avoid dealing with absolute values.

This paragraph delves into the implications of different elasticity values. Demand is classified as inelastic if the elasticity (denoted as E of X or Y of X) is less than 1, meaning that changes in price have a smaller proportional impact on quantity demanded. An example given is insulin, where demand remains relatively stable even with price increases due to its necessity. If elasticity is greater than 1, demand is elastic, and small price changes lead to significant changes in quantity demanded, as illustrated by the example of substitutable goods like canned beans. Unit elasticity, where the elasticity equals 1, is also discussed, indicating that price and quantity changes are proportional. The paragraph concludes with strategies for maximizing revenue based on the elasticity of demand.

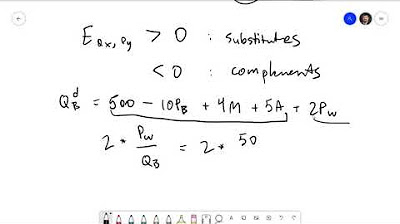
The presenter provides a step-by-step calculation of elasticity using a specific demand function (D of X = 500 - 2X). After finding the derivative of the demand function (dQ/dx), the elasticity formula is applied to find the general expression for elasticity. The elasticity at a given price ($57) is then calculated, and it is determined to be inelastic. The implications for revenue maximization are discussed, concluding that the price should be increased for inelastic demand to maximize revenue. The process of finding the price that maximizes revenue when elasticity equals one is also demonstrated.

The video continues with an example involving a demand function that includes a square root (Q = √(300 - X)). The elasticity is calculated, and it is found to be elastic at a price of $250. The presenter explains that for elastic demand, decreasing the price can lead to increased revenue. The process to find the price that results in unit elasticity and thus maximizes revenue is shown, with the conclusion that the price should be set to $125 for this purpose. The elasticity value is interpreted to mean that a 1% increase in price results in a 2.5% decrease in demand, reinforcing the principle that for elastic goods, a price decrease can increase total revenue.

The presenter tackles a word problem involving an exponential demand function for computer apps (Q = 50 * e^(-0.12X)). The elasticity is calculated to be 0.12X, and it is determined to be inelastic when the price is $3. The implications for total revenue with a small price increase are discussed, noting that increasing the price would increase total revenue due to the inelastic demand at that price level. The video concludes with a confirmation that for revenue maximization, the price should be increased for inelastic demand.

The video concludes with a summary of the elasticity of demand, emphasizing its importance in understanding how price changes affect quantity demanded and, subsequently, total revenue. The presenter reiterates the key points: inelastic demand (elasticity < 1) requires a price increase to maximize revenue, elastic demand (elasticity > 1) benefits from a price decrease to maximize revenue, and unit elastic demand (elasticity = 1) is already at the optimal price for revenue maximization. The video encourages viewers to apply these concepts to various types of demand functions and to seek further clarification during live sessions if needed.