Calculating the Elasticity of Demand

Marginal Revolution University

27 Jan 201515:52

EducationalLearning

32 Likes 10 Comments

TLDRThis lecture on the elasticity of demand explains how to measure responsiveness of quantity demanded to price changes. It covers the formula for calculating elasticity, provides examples, and highlights the importance of the midpoint formula to avoid calculation inconsistencies. The relationship between elasticity and total revenue is explored, with graphical illustrations demonstrating inelastic and elastic demand curves. Applications like the war on drugs and effects of drought on crop revenues are discussed, emphasizing the practical implications of demand elasticity in various scenarios.

Takeaways

📊 Elasticity of demand is a measure of how responsive the quantity demanded is to a change in price, with more responsive demand being more elastic.
🔢 The numeric measure of elasticity is calculated by dividing the percentage change in quantity demanded by the percentage change in price.
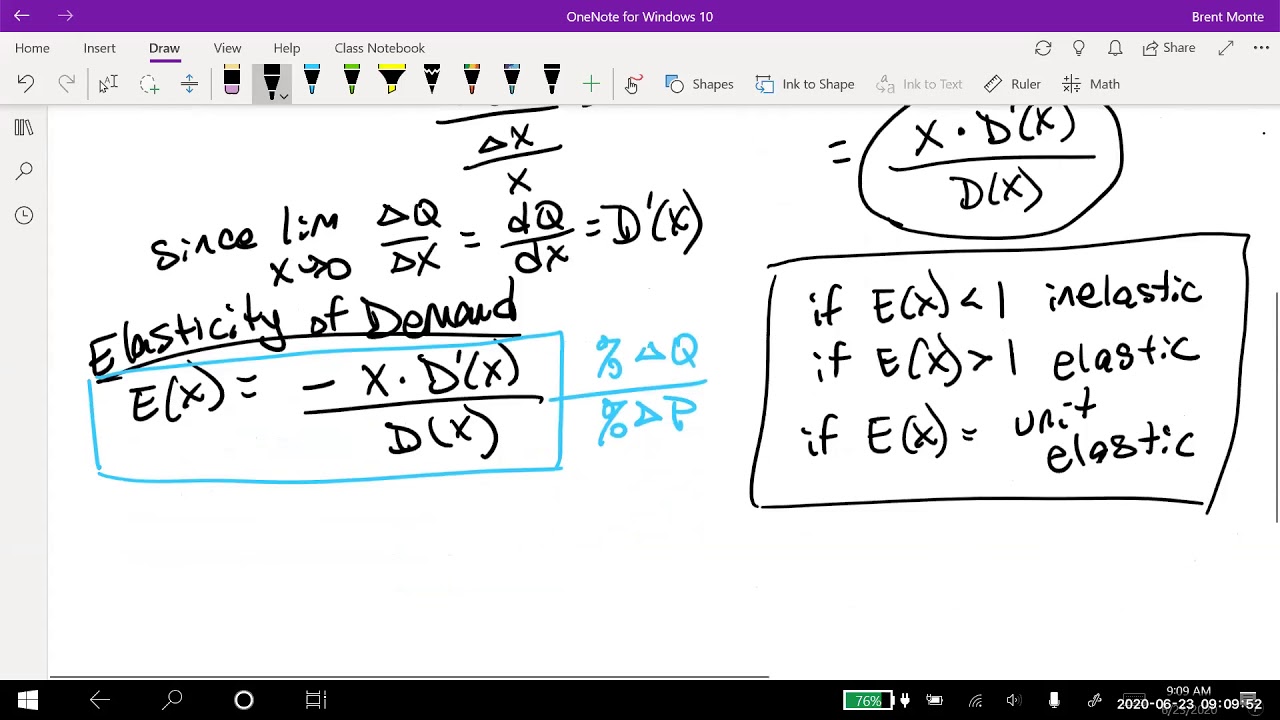
⚖️ Elasticity values are always negative, but the negative sign is often dropped for simplicity, with values less than one indicating inelastic demand, greater than one indicating elastic demand, and equal to one indicating unit elastic demand.
📉 An example given is that if the price of oil increases by 10% and the quantity demanded falls by 5%, the elasticity of demand for oil is -0.5, which is inelastic.
🔄 The Midpoint Formula is used to calculate elasticity to avoid inconsistencies that arise from changing the base of percentage changes.
📐 The formula for elasticity using the Midpoint Formula is (change in quantity / average quantity) / (change in price / average price).
💡 Understanding the calculation of percentage change is crucial, as it can be tricky due to changes in the base value, hence the use of the Midpoint Formula.
📚 A specific example is provided where an initial price of $10 with a quantity demanded of 100, and a subsequent price rise to $20 with a quantity demanded falling to 90, results in an elasticity of 0.158, indicating inelastic demand.
💰 The relationship between elasticity of demand and total revenue is significant; inelastic demand leads to revenue increasing when prices rise, while elastic demand leads to revenue decreasing when prices rise.
📉 Graphical representations of demand curves help illustrate the relationship between price changes, quantity demanded, and total revenue, with steeper curves indicating inelastic demand and flatter curves indicating elastic demand.
🧑‍🏫 The script uses real-world examples, such as the war on drugs and the impact of drought on corn growers, to demonstrate the practical applications of understanding elasticity of demand.

Q & A

What is the basic concept of elasticity of demand?
-Elasticity of demand measures the responsiveness of the quantity demanded to a change in price. It indicates how much the quantity demanded changes for a given percentage change in price.
How is the elasticity of demand calculated?
-The elasticity of demand is calculated as the percentage change in quantity demanded divided by the percentage change in price, often using the Midpoint Formula to ensure consistency in calculations.
What does a negative elasticity of demand signify?
-A negative elasticity of demand signifies the inverse relationship between price and quantity demanded; as price increases, quantity demanded decreases, and vice versa.
What are the three categories of demand elasticity, and how are they determined?
-The three categories are inelastic, elastic, and unit elastic. Demand is inelastic if the absolute value of elasticity is less than one, elastic if it's greater than one, and unit elastic if it's exactly one.
Why is the Midpoint Formula used to calculate elasticity?
-The Midpoint Formula is used to avoid inconsistencies that arise from calculating percentage changes from different bases. It ensures the same elasticity value whether calculated from a lower or higher base.
Can you provide an example of calculating elasticity of demand from the script?
-Yes, an example given in the script is when the price of oil increases by 10% and the quantity demanded falls by 5%. The elasticity of demand is calculated as -5% / 10%, which equals -0.5, and is often reported as 0.5 after dropping the negative sign.
How does the elasticity of demand relate to total revenue?
-The relationship between elasticity of demand and total revenue depends on whether the demand is inelastic, elastic, or unit elastic. With inelastic demand, an increase in price leads to an increase in revenue, while with elastic demand, an increase in price leads to a decrease in revenue. Unit elastic demand means a change in price does not affect total revenue.

Outlines

00:00

📊 Elasticity of Demand Basics

This paragraph introduces the concept of elasticity of demand, which is a measure of how responsive the quantity demanded is to changes in price. It explains that a higher elasticity indicates a more responsive demand. The paragraph also outlines how to calculate elasticity using the formula: elasticity of demand = (% change in quantity demanded) / (% change in price). An example is given where a 10% increase in the price of oil leads to a 5% decrease in quantity demanded, resulting in an elasticity of -0.5, which is typically expressed as 0.5 due to the inverse relationship between price and quantity. The paragraph concludes by defining different types of demand curves based on elasticity: inelastic (elasticity < 1), elastic (elasticity > 1), and unit elastic (elasticity = 1).

05:01

📈 Calculating Elasticity and Its Implications

This section delves deeper into calculating elasticity, emphasizing the importance of using the Midpoint Formula to avoid inconsistencies when calculating percentage changes. An example calculation is provided, where an initial price of $10 and a quantity demanded of 100 change to a price of $20 and a quantity of 90, resulting in an elasticity of 0.158, indicating inelastic demand. The paragraph further explains the relationship between elasticity and total revenue, showing that with inelastic demand, an increase in price leads to an increase in revenue, whereas with elastic demand, an increase in price leads to a decrease in revenue. This relationship is illustrated with graphs and a thought experiment.

10:05

🌾 Applications of Elasticity in Real Life

The paragraph discusses the real-world applications of elasticity of demand, using the war on drugs and its impact on drug dealers' revenues as an example. It explains that because drugs typically have inelastic demand, enforcement actions that raise prices also increase total revenues for sellers, potentially exacerbating issues related to drug prohibition. Another application is presented through a quote from NPR's food blog about the Midwest drought and its counterintuitive effect on corn growers' revenues due to likely inelastic demand for corn, which could explain why revenues increased despite reduced yields.

15:08

📚 Conclusion and Preview of Elasticity of Supply

In conclusion, the paragraph summarizes the key points about elasticity of demand and encourages students to practice these concepts, perhaps through sketching graphs to solidify their understanding. It also previews the next topic, which will be the elasticity of supply, suggesting that the concepts covered in this lecture will provide a solid foundation for understanding supply elasticity. The paragraph ends with a prompt for students to engage with practice questions or proceed to the next video.

Mindmap

Keywords

💡Elasticity of Demand

Elasticity of demand measures the responsiveness of the quantity demanded to a change in price. It is a key concept in understanding consumer behavior and market dynamics. In the video, elasticity is defined as the percentage change in quantity demanded divided by the percentage change in price. The script uses the example of oil to illustrate how elasticity is calculated and its implications for market behavior.

💡Percentage Change

Percentage change is a way to express how much a quantity has increased or decreased, relative to its original amount. In the context of the video, it is used to calculate the elasticity of demand by comparing the percentage change in quantity demanded to the percentage change in price. The script explains the calculation using the formula involving the change in quantity and price divided by their respective averages.

💡Inelastic Demand

Inelastic demand refers to a situation where the quantity demanded does not change significantly in response to a change in price. In the video, it is defined as when the absolute value of the elasticity of demand is less than one. The script uses the example of oil to show that a 10% increase in price results in only a 5% decrease in quantity demanded, indicating inelastic demand.

💡Elastic Demand

Elastic demand is the opposite of inelastic demand, where the quantity demanded is highly responsive to price changes. The video explains that if the elasticity of demand is greater than one, the demand curve is considered elastic. This concept is crucial for understanding how price changes can significantly affect the quantity demanded and, consequently, the market.

💡Unit Elastic

Unit elastic describes a specific case where the elasticity of demand is exactly one. In the video, it is referred to as the 'knife point case' where the demand curve is neither elastic nor inelastic. The script suggests that in such a scenario, a change in price does not affect the total revenue, as the percentage change in quantity demanded offsets the percentage change in price.

💡Midpoint Formula

The Midpoint Formula is used to calculate the percentage change in elasticity to avoid inconsistencies that arise from changing the base of calculation. The video emphasizes its importance by explaining that it involves dividing the change in quantity or price by their respective averages. This ensures that the elasticity calculation remains consistent regardless of the direction of price change.

💡Total Revenue

Total revenue is the income generated from the sale of goods or services, calculated as the product of price and quantity sold. In the video, the relationship between elasticity of demand and total revenue is explored, showing that inelastic demand leads to an increase in revenue when prices rise, while elastic demand results in a decrease in revenue under the same circumstances.

💡Revenue Rectangles

Revenue rectangles are a visual representation used in the video to illustrate the relationship between price, quantity, and total revenue. They are depicted on a graph as rectangles with the length representing quantity and the height representing price. The size of these rectangles helps to understand how changes in price and quantity affect total revenue.

💡Practice Question

A practice question in the video asks what would happen to total revenues if the elasticity of demand for eggs, estimated to be 0.1, faces a 10% price increase. This is an application of the concept of inelastic demand and its impact on revenue. The video script suggests using the previously discussed concepts to answer such questions.

💡Application of Elasticity

The video provides real-world applications of elasticity of demand, such as the war on drugs and the impact of droughts on agricultural revenues. It suggests that understanding elasticity can help explain why certain market interventions, like prohibition, may lead to unintended consequences like increased seller revenues, and how natural disasters can paradoxically benefit certain industries due to inelastic demand.

Highlights

Elasticity of demand measures the responsiveness of quantity demanded to a change in price.

Elastic demand means a higher responsiveness to price changes.

The numeric measure of elasticity is calculated using the formula: elasticity = (% change in quantity demanded) / (% change in price).

Elasticity of demand is always negative due to the inverse relationship between price and quantity demanded.

Demand is considered inelastic if the absolute value of elasticity is less than one.

Elastic demand is indicated when the elasticity is greater than one.

Unit elastic demand occurs when elasticity equals one.

Percentage change in quantity is calculated using the Midpoint Formula to avoid inconsistencies.

An example calculation shows how to determine elasticity using price and quantity changes.

Inelastic demand results in increased revenue when prices rise due to the small change in quantity demanded.

Elastic demand leads to decreased revenue when prices rise due to a significant drop in quantity demanded.

The relationship between elasticity and total revenue can be visualized through graphical analysis.

A practice question illustrates the impact of a 10% price increase on total revenues for a product with an elasticity of 0.1.

The war on drugs is difficult due to the inelastic demand for drugs, leading to increased seller revenues despite enforcement actions.

The paradoxical effect of drought on corn growers' revenues can be explained by understanding elasticity of demand.

Elasticity of demand has practical applications in understanding market behaviors and policy impacts.

Upcoming lectures will cover the elasticity of supply, which shares similar concepts with demand elasticity.

Transcripts

Browse More Related Video

Math 11 - Section 3.7

Math 1325 Lecture 11 5 - Applications

Y1 10) Price Elasticity of Demand - PED

What is Elasticity?

Elasticity of Demand - Example

Managerial Economics 2.5: More Elasticity