3.7 - Elasticity of Demand
TLDRThe video script delves into the concept of demand elasticity and its crucial role in economic decision-making for pricing strategies. It explains the inverse relationship between product pricing and the quantity consumers are willing to purchase. The script outlines how producers and manufacturers use the concept of demand elasticity to understand the sensitivity of quantity demanded to price changes. By analyzing the demand function and its derivative, the video demonstrates how to calculate elasticity and identify the optimal pricing point that maximizes revenue. The process involves setting up an elasticity function, evaluating it at different price points, and finding the price where elasticity equals one—indicating unit elasticity and the sweet spot for pricing. The video uses mathematical examples to illustrate these concepts, emphasizing the practical application of derivatives in real-world business scenarios.
Takeaways
- 📈 The concept of demand relates price to the quantity people are willing to buy, typically showing an inverse relationship.
- 💰 Producers aim to price high to increase revenue, while consumers prefer lower prices to afford more products.
- 🔍 Demand elasticity is a measure of how sensitive the quantity demanded is to changes in price, crucial for pricing strategy.
- ⚖️ Elastic demand means a significant change in quantity with a small price change, while inelastic demand shows little change in quantity despite price changes.
- 📊 To find the optimal pricing for maximizing revenue, one must analyze the demand function and calculate its elasticity.
- 🧮 The revenue function is calculated by multiplying the price by the quantity demanded, which can be derived from the demand function.
- 🔑 The price that maximizes revenue is found where the elasticity of demand is equal to one, indicating a balance between price increases and quantity demanded.
- 🤔 At a price where elasticity is less than one, demand is inelastic, and small price increases can still increase revenue.
- 📉 Conversely, when elasticity is greater than one, demand is elastic, and price increases can lead to revenue decreases due to significant quantity decreases.
- 💡 The derivative of the demand function plays a key role in finding the elasticity function and, subsequently, the optimal pricing for revenue maximization.
- 📦 The process of finding the optimal price involves differentiating the demand function, setting up the elasticity function, and solving for critical points that represent potential revenue maxima.
Q & A
What is the general idea behind the economic concept of demand?
-The general idea behind demand is comparing the pricing of a product to the quantity that people are willing to buy. Typically, there is an inverse relationship between these two values, meaning that as price decreases, the quantity demanded increases, and as price increases, the quantity demanded decreases.
Why would producers be interested in the elasticity of demand?
-Producers are interested in the elasticity of demand because it helps them understand how small changes in price, typically an increase, will impact the demand for their product. This knowledge is crucial for making pricing decisions that maximize revenue without causing a significant drop in the quantity demanded.
How is revenue generally calculated in the context of pricing and quantity?
-Revenue is generally calculated as the price charged per item multiplied by the quantity of items purchased. It represents the total income generated from sales.
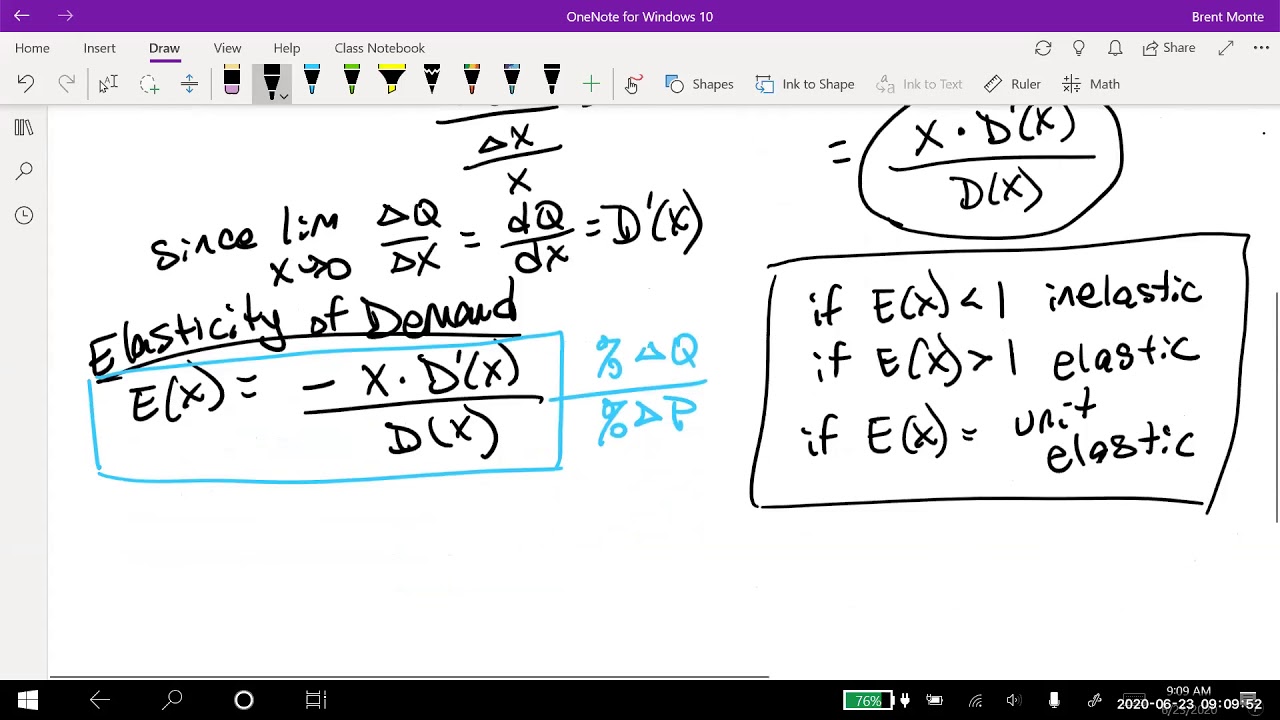
What is the definition of elasticity of demand?
-Elasticity of demand is defined as the sensitivity or responsiveness of the quantity demanded of a good to a change in the price of that good. It measures how much the quantity demanded responds to a change in the price.
What does it mean if demand is considered 'elastic'?
-If demand is considered 'elastic', it means that the percentage change in the quantity demanded is greater than the percentage change in price. In other words, a small change in price results in a proportionally larger change in quantity demanded, indicating the demand is highly sensitive to price changes.
What does it mean if demand is considered 'inelastic'?
-If demand is considered 'inelastic', it means that the percentage change in the quantity demanded is less than the percentage change in price. This indicates that the demand is not very sensitive to price changes and that consumers will continue to buy the product even if the price increases.
How can a company determine the optimal pricing for a product to maximize revenue?
-A company can determine the optimal pricing for a product to maximize revenue by analyzing the elasticity of demand. The optimal price is typically found where the elasticity of demand is equal to one, indicating that the product has unit elasticity and that changes in price and quantity are balanced, thus maximizing revenue.
What is the mathematical representation of the elasticity of demand?
-The elasticity of demand (E) is mathematically represented as E = (% change in quantity demanded) / (% change in price), or more specifically, E = (ΔQ/Q) / (ΔP/P), where ΔQ is the change in quantity demanded, Q is the original quantity demanded, ΔP is the change in price, and P is the original price.
What is the significance of finding the price at which elasticity equals one?
-Finding the price at which elasticity equals one is significant because it represents the point where the demand has unit elasticity. This is the price level at which the percentage change in quantity demanded equals the percentage change in price, indicating the optimal pricing point to maximize revenue without losing too many customers due to price sensitivity.
How does the concept of elasticity help in understanding the relationship between price and quantity demanded?
-The concept of elasticity helps in understanding the relationship between price and quantity demanded by quantifying the responsiveness of the quantity demanded to changes in price. It provides a measure of how sensitive the demand is to price changes, which is crucial for pricing strategies and revenue optimization.
What is the revenue function, and how is it derived from the demand function?
-The revenue function represents the total revenue a company can expect from sales at a given price. It is derived from the demand function by multiplying the demand (quantity) by the price. In mathematical terms, if the demand function is represented as q = d(x), then the revenue function R(x) is given by R(x) = x * d(x), where x is the price.
Outlines
📈 Understanding Demand and Pricing Strategy
This paragraph introduces the concept of demand in relation to pricing. It explains the inverse relationship between product pricing and the quantity consumers are willing to purchase. It highlights the interest of producers in analyzing how price changes, particularly increases, affect the quantity demanded. The paragraph also touches on the idea of elasticity of demand, which measures the sensitivity of demand to price changes, and the importance of finding a pricing 'sweet spot' to maximize revenue without deterring consumers.
📊 Analyzing Price Changes and Revenue Impact
The second paragraph delves into the specifics of how a company, using a hypothetical Blu-ray rental service as an example, can assess the impact of price changes on revenue. It outlines the calculation of demand and revenue at a price point of two dollars, and then examines the effects of a 10 percent price increase on both the quantity demanded and the resulting revenue. The summary emphasizes the importance of balancing price increases with the potential decrease in rentals to maintain or increase overall revenue.
📉 The Effect of Higher Pricing on Demand and Revenue
This paragraph explores a scenario where the rental price is set at four dollars and the impact of a subsequent 10 percent increase is analyzed. It demonstrates that while doubling the price from two to four dollars halves the expected rentals, the revenue remains the same due to the price increase. However, the paragraph reveals that further increasing the price from four dollars results in a more significant drop in rentals, leading to decreased revenue. This illustrates the concept of demand elasticity and how it can influence business decisions.
🔍 Deriving the Elasticity Function
The fourth paragraph focuses on the creation of an elasticity function to generalize the sensitivity of demand to price changes across various prices. It defines elasticity as a ratio involving the derivative of the demand function and uses the given demand function to derive the elasticity function. The paragraph explains the significance of this function in predicting how changes in price will affect the quantity demanded, which is crucial for a producer's pricing strategy.
🤝 Balancing Price and Quantity Demanded
In this paragraph, the concept of unit elasticity is introduced as the point where the change in price is matched by an equal change in quantity demanded. It describes how to find this balance point by setting the elasticity function equal to one and solving for the price. The paragraph also discusses the revenue function, which is derived by multiplying the price by the demand function, and emphasizes the importance of this function in determining optimal pricing for maximizing revenue.
🔑 The Role of Elasticity in Maximizing Revenue
The sixth paragraph establishes the relationship between elasticity and revenue. It explains that revenue increases when demand is inelastic (elasticity < 1) and decreases when demand is elastic (elasticity > 1). The paragraph identifies the point of unit elasticity as the optimal pricing strategy, where changes in price and quantity demanded are balanced, resulting in maximized revenue. It also discusses the process of confirming that the critical value found from the revenue function indeed represents a maximum.
📝 Applying Elasticity to Optimal Pricing Strategy
The seventh paragraph applies the concept of elasticity to a new example with a given demand function involving a square root expression. It outlines the steps to find the elasticity function, determine if demand is elastic or inelastic at a specific price point, and calculate the optimal pricing for maximizing revenue. The summary highlights the practical implications of pricing strategies on customer retention and overall revenue.
🧮 Calculating Elasticity and Optimal Pricing
The final paragraph provides a detailed calculation of the elasticity function for a given demand function and evaluates the elasticity at a price of $250. It determines that demand is elastic at this price, suggesting that the product is likely overpriced. The paragraph then demonstrates how to find the optimal pricing that results in unit elasticity and maximized revenue, concluding that $200 is the optimal price for the product based on the given demand function.
Mindmap
Keywords
💡Derivative
💡Elasticity of Demand
💡Revenue
💡Price Sensitivity
💡Optimal Pricing
💡Quantity Demanded
💡Inverse Relationship
💡Maximizing Revenue
💡Critical Values
💡Unit Elasticity
💡Revenue Function
Highlights
Derivative and its relation to the economic concept of demand are discussed in the video.
Demand is inversely related to pricing, with lower prices typically resulting in higher quantities demanded.
Producers aim to price high to increase revenue, while consumers prefer lower prices.
Retailers and manufacturers are interested in the impact of small price changes on demand.
Elasticity of demand is defined as the sensitivity of demand to price changes.
Demand elasticity is crucial for analyzing how price changes affect the quantity demanded and revenue.
The concept of 'sweet spot' pricing is introduced, where revenue is maximized without deterring customers.
An example of a Blu-ray rental company's demand function is used to illustrate price and revenue relationships.
The video demonstrates how to calculate demand and revenue at different pricing points.
The importance of maximizing revenue while considering the quantity demanded at various price levels is emphasized.
The elasticity function is derived from the demand function using the derivative.
The video shows how to find the price that maximizes revenue by setting the elasticity function equal to one.
Revenue is found to increase when elasticity is less than one, indicating inelastic demand.
Revenue decreases when elasticity is greater than one, showing elastic demand and sensitivity to price changes.
The optimal pricing strategy is identified where elasticity equals one, resulting in unit elasticity.
The video concludes with an example of applying elasticity to a demand function with a radical expression.
The process of finding the optimal price point using the elasticity function is demonstrated step by step.
The video concludes by emphasizing the practical applications of derivatives in analyzing real-world business scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: