What is Elasticity?
TLDRIn this video, Professor Monte explains the concept of elasticity in economics, which is the ratio of the percent change in quantity to the percent change in price. He clarifies that when price increases, quantity demanded decreases, and vice versa, which is why elasticity is often a negative number, but it is typically presented as a positive for ease of understanding. The formula for price elasticity of demand is detailed, involving the derivative of the demand function. Monte discusses the implications of different elasticity values: if the elasticity is greater than one, the demand is elastic; if it's less than one, it's inelastic; and at unit elasticity, revenue is maximized. He also emphasizes the importance of correctly calculating elasticity to avoid confusion with negative values and provides a link to another video for further understanding of the topic.
Takeaways
- 📈 **Elasticity Definition**: Elasticity is the ratio of the percent change in quantity demanded to the percent change in price.
- 🔢 **Elasticity Example**: An elasticity of 0.3 means a 1% increase in price leads to a 0.3% decrease in quantity demanded.
- ↔️ **Opposite Directions**: Price and quantity demanded always move in opposite directions; when one goes up, the other goes down.
- 💡 **Formula Origin**: The elasticity formula is derived from the demand function and involves calculus concepts like derivatives.
- 📉 **Derivative Significance**: The derivative of the demand function (dQ/dp) indicates how quantity demanded changes with price, and it's always negative because of the inverse relationship.
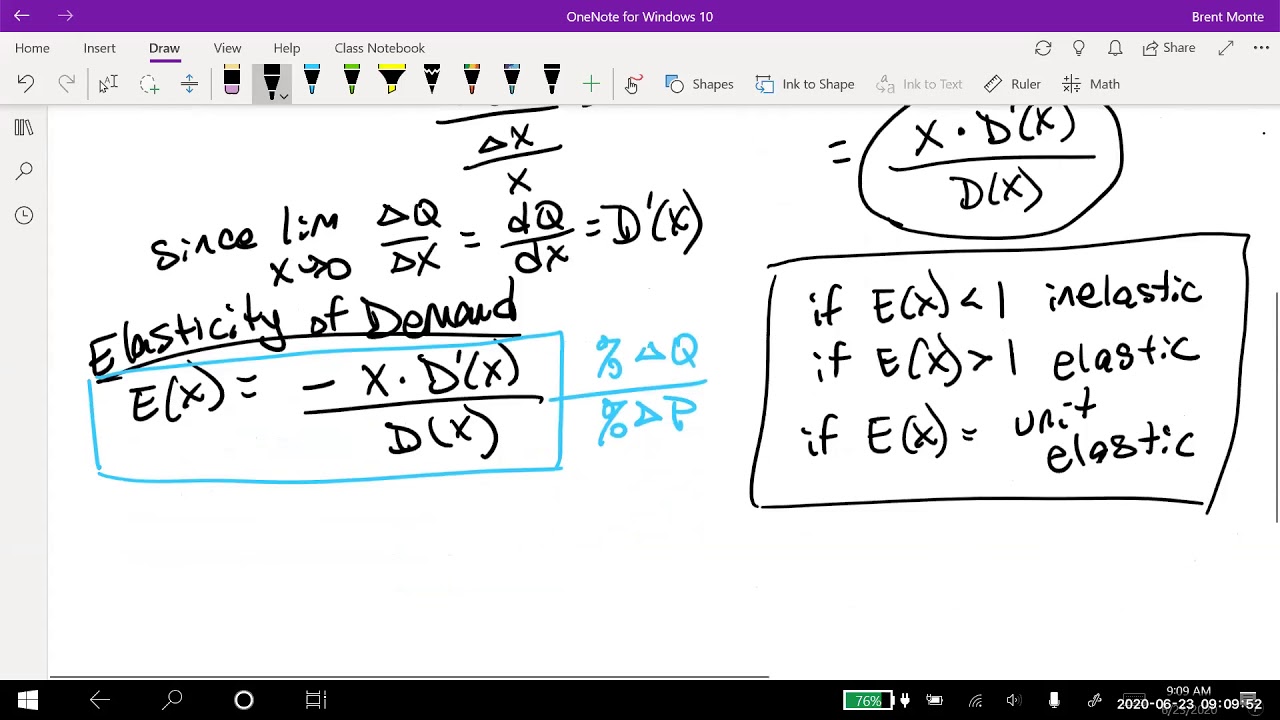
- ➗ **Elasticity Formula**: The elasticity formula is Price × (Derivative of demand function) / (Original demand function), with a negative sign to account for the inverse relationship.
- ⚠️ **Sign Importance**: Elasticity is always a positive number because the negative sign in the formula cancels out the negative derivative.
- 🔍 **Elasticity Interpretation**: If elasticity (e) is greater than 1, demand is elastic; if less than 1, it's inelastic; and at 1, it's unitary elasticity where revenue is maximized.
- 📚 **Further Study**: For a deeper understanding, look into other videos that work out elasticity problems and discuss elastic and inelastic demand.
- 👍 **Engagement Encouraged**: The speaker encourages viewers to subscribe to the channel and like the video to help others find it.
- 📅 **Continuing Education**: More discussions on the topic will be provided in future videos.
Q & A
What is the basic definition of elasticity in economics?
-Elasticity is the ratio of the percent change in quantity to the percent change in price. It measures how the quantity demanded or supplied of a good responds to a change in the price of that good.
How does the direction of change in price and quantity relate in the context of elasticity?
-Price and quantity typically move in opposite directions. When the price of a good increases, the quantity demanded usually decreases, and vice versa.
What does an elasticity value of 0.3 signify?
-An elasticity value of 0.3 indicates that for every 1 percent increase in price, the quantity demanded decreases by 0.3 percent.
Why is the sign of the elasticity formula always negative, and how is it typically handled in calculations?
-The sign is always negative because the derivative of the demand function with respect to price (dQ/dp) is negative, reflecting the inverse relationship between price and quantity. This is often handled by including a negative sign in the formula to ensure the elasticity value is positive.
What does it mean if the elasticity (e) is greater than 1?
-If the elasticity is greater than 1, it is considered elastic, which means the percentage change in quantity is greater than the percentage change in price.
What is the significance of unit elasticity?
-Unit elasticity occurs when the elasticity equals 1. At this point, the percentage change in quantity is equal to the percentage change in price, and it is where revenue is maximized for a firm.
How is the elasticity formula derived from the basic concept?
-The elasticity formula is derived by considering the change in quantity relative to the original quantity and the change in price relative to the original price. As the change in price approaches zero, the formula approaches the derivative of quantity with respect to price, which is the demand function.
What is the role of calculus in determining the elasticity formula?
-Calculus is used to understand the derivative of the demand function (dQ/dp) as the change in price approaches zero, which is a key component in the elasticity formula.
Why is it important to ensure the elasticity value is positive in economic analysis?
-A positive elasticity value is important because it reflects the inverse relationship between price and quantity demanded in a more intuitive manner and avoids confusion with absolute values.
What does it imply if the elasticity value is less than 1?
-If the elasticity value is less than 1, it indicates inelastic demand, meaning the percentage change in quantity is less than the percentage change in price.
How can one determine whether a good is elastic or inelastic based on elasticity values?
-A good is considered elastic if the elasticity value is greater than 1, inelastic if it is less than 1, and unitary elastic if it equals 1.
What is the practical application of understanding elasticity in business decision-making?
-Understanding elasticity helps businesses determine how sensitive the demand for their product is to price changes, which can inform pricing strategies, maximize revenue, and predict consumer behavior.
Outlines
📚 Introduction to Elasticity
Professor Monte begins by defining elasticity as the ratio of the percent change in quantity to the percent change in price. He explains that if the elasticity is 0.3, it means a 1% increase in price will result in a 0.3% decrease in quantity demanded. The professor also clarifies that price and quantity always move in opposite directions. He further delves into the mathematical derivation of the elasticity formula, emphasizing the role of calculus and the concept of the derivative of the demand function. The video aims to provide a clear understanding of how quantity changes in response to price changes.
🔢 Elasticity Formula and Significance
The second paragraph focuses on the elasticity formula, which is the price times the derivative of the demand function over the original demand function. The professor discusses the importance of considering the sign of the derivative, which is always negative as price and quantity demanded move in opposite directions. To avoid dealing with negative numbers, a negative sign is often included in the formula, ensuring that the elasticity value is always positive. The significance of different elasticity values is also explained: if the elasticity is greater than 1, the demand is elastic; if less than 1, it's inelastic; and at exactly 1, it's unitary elasticity, which is when revenue is maximized. The paragraph concludes with a reminder to watch another video for a practical problem-solving approach to understanding elasticity.
Mindmap
Keywords
💡Elasticity
💡Percent Change
💡Price and Quantity
💡Demand Function
💡Derivative
💡Unit Elasticity
💡Inelastic
💡Elastic
💡Revenue Maximization
💡Positive Number
💡Absolute Value
Highlights
Elasticity is defined as the ratio of the percent change in quantity to the percent change in price.
An elasticity value of 0.3 means a 1% price increase results in a 0.3% decrease in quantity demanded.
Price and quantity demanded always move in opposite directions due to the law of demand.
The formula for elasticity is derived from the demand function and involves calculus concepts.
As the change in price approaches zero, the formula approaches the derivative of the demand function.
The derivative of the demand function (dq/dp) represents the rate at which quantity demanded changes with price.
The elasticity formula is often adjusted with a negative sign to ensure the result is positive.
Elasticity is always a positive number because of the adjustment for the negative derivative.
An elasticity value greater than one indicates elastic demand, less than one indicates inelastic demand, and equal to one is unit elasticity.
Unit elasticity occurs where revenue is maximized.
The sign of the derivative is always negative because price and quantity demanded move in opposite directions.
Care must be taken to ensure the negative sign in the elasticity formula cancels out with the negative derivative.
If the elasticity calculation results in a negative number, the process was not done correctly.
The elasticity of demand is crucial for understanding how price changes affect quantity demanded and overall revenue.
The video provides a detailed explanation of the elasticity formula and its practical implications in economics.
The presenter encourages viewers to watch additional videos for more examples and applications of elasticity.
The video concludes with a call to action for viewers to subscribe and like the content for further insights.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: