Managerial Economics 1.2: Univariate Optimization

SebastianWaiEcon
29 Jul 202107:31
EducationalLearning
32 Likes 10 Comments

TLDRIn this video on Managerial Economics by Sebastian Y, the focus is on optimizing a univariate function, a fundamental concept in decision-making for firms, governments, and individuals. The video explains that optimization involves finding the best values for choice variables to achieve an optimal outcome, as defined by an objective function. For a univariate function with a single choice variable, derivatives are used to identify the optimal value. The process involves calculating the first derivative to find where the function increases or decreases and setting it to zero to find the first order condition (FOC). The second derivative is then used to determine if the point is a maximum or minimum by checking its sign. An example is provided with the function f(x) = -x^2 + 10x + 5, where the optimal value of x is found to be 5, leading to a maximum value of the function at 30. The video concludes by highlighting the importance of this basic optimization technique, which will be further explored in more complex multivariate functions in subsequent videos.

Takeaways
  • 📈 **Optimization in Managerial Economics**: The video focuses on how to optimize a univariate function within the context of managerial economics, which involves decision-making by firms, governments, or individuals.
  • 🎯 **Objective of Optimization**: The goal is to find the optimal values for choice variables that lead to the best outcome, such as maximizing utility for individuals or profit for firms.
  • 🔑 **Choice Variables**: Economic agents control certain variables that are crucial to the outcome, known as choice variables.
  • 📙 **Objective Function**: This mathematical relationship between choice variables and the outcome of interest is represented as the objective function.
  • 📉 **Continuous vs. Discrete Choices**: The video discusses optimizing in continuous choice spaces, which involves an infinite number of possibilities, as opposed to discrete choices with a set number of options.
  • 🧮 **Use of Derivatives**: Derivatives are essential for optimizing functions in continuous choice spaces, with the first derivative indicating the rate of change of the function.
  • 🔍 **First Order Condition (FOC)**: The optimal value of a function is found where its first derivative equals zero, known as the first order condition.
  • 🔄 **Second Derivative Test**: The sign of the second derivative helps determine if a point is a maximum or minimum; a negative second derivative indicates a maximum.
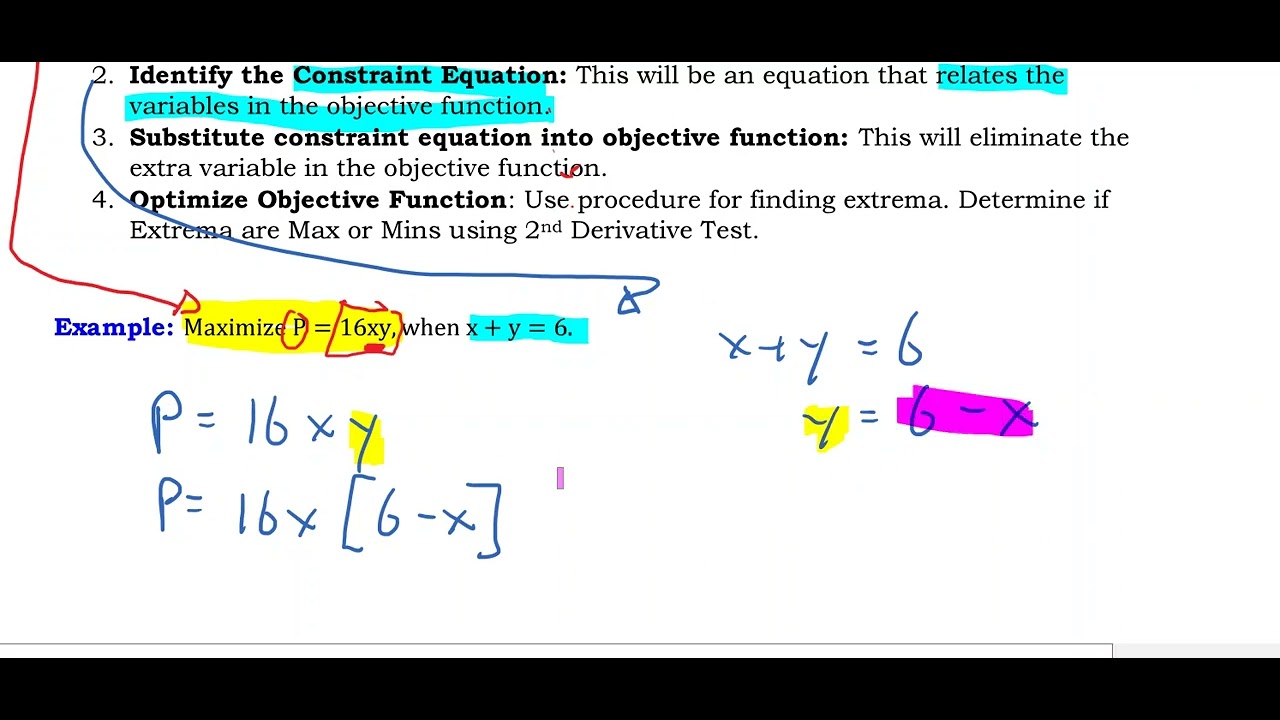
  • 📌 **Example Problem**: The video provides an example of maximizing the function f(x) = -x^2 + 10x + 5, which involves finding the first and second derivatives.
  • 📐 **Graphical Interpretation**: The second derivative's sign is related to the function's concavity; a negative second derivative means the function is concave down, indicating a maximum.
  • 🧮 **Maximizing the Example Function**: By setting the first derivative to zero and solving, the video finds that x = 5 maximizes the given function.
  • 📝 **Final Value Calculation**: The maximum value of the example function is calculated by substituting the maximizing value of x back into the function, resulting in a maximum value of 30.
Q & A

  • What is the primary focus of managerial economics?

    -Managerial economics focuses on decision making, which can be by firms, governments, or individual people, and is approached as optimization problems.

  • What are the different priorities decision makers might have when optimizing?

    -Individuals might aim to maximize their utility, while firms generally aim to maximize profit. A factory manager might want to minimize costs to produce a certain amount of output.

  • What are choice variables in the context of optimization problems?

    -Choice variables are the factors over which the economic agent has control and are used to find optimal outcomes in optimization problems.

  • What is the objective function in optimization?

    -The objective function is a mathematical relationship between choice variables and the outcome of interest, such as a firm's profit based on the price set and advertising done.

  • Why do we use derivatives to optimize functions in a continuous choice space?

    -Derivatives are used in a continuous choice space because there are an infinite number of possibilities, and they help identify the optimal value of the function.

  • What is the first order condition in optimization?

    -The first order condition (FOC) is the equation where the derivative of the objective function with respect to the choice variable equals zero, indicating a potential optimal value.

  • How can we determine if a critical point found by the first order condition is a maximum or minimum?

    -We use the second derivative test. If the second derivative is negative, it indicates a maximum, and if it's positive, it indicates a minimum.

  • What does the sign of the second derivative indicate about the function's shape?

    -If the second derivative is negative, the function is concave (shaped like a downward-facing parabola), and if it's positive, the function is convex (shaped like an upward-facing parabola).

  • In the given example, what is the objective function?

    -The objective function in the example is f(x) = -x^2 + 10x + 5.

  • What is the maximum value of the example objective function?

    -The maximum value of the example objective function is 30, which occurs when x = 5.

  • What will be the topic of the next video in the series?

    -The next video will introduce methods for optimizing multivariate objective functions.

Outlines
00:00
📈 Optimization of Univariate Functions

In this paragraph, Sebastian Y introduces the concept of managerial economics and its focus on decision-making through optimization problems. He explains that economic agents, whether firms, governments, or individuals, have control over certain variables known as choice variables. The goal is to find the optimal values for these variables to achieve the best outcome. The relationship between choice variables and the outcome is represented by the objective function. The video focuses on univariate functions, which have a single choice variable, and emphasizes the use of derivatives to optimize these functions in a continuous choice space. The first order condition (FOC) is introduced as a critical step in optimization, which is found by setting the derivative of the objective function to zero. An example is provided with the function f(x) = -x^2 + 10x + 5, and the process of finding the maximum value by solving the FOC and using the second derivative to confirm the nature of the critical point (maximum or minimum) is demonstrated.

05:02
📊 Maximizing a Univariate Objective Function

This paragraph continues the discussion on optimizing a univariate function by explaining how to determine whether a critical point found by the first order condition is a maximum or minimum using the second derivative. The sign of the second derivative indicates the concavity of the function, with a negative second derivative indicating a maximum and a positive one indicating a minimum. The concept is illustrated graphically, showing how the derivative changes from positive to negative at the maximum point. The example function f(x) = -x^2 + 10x + 5 is revisited, and the maximum value of the function is calculated by substituting the maximizing value of x (which is 5) back into the function, resulting in f(5) = 30. The paragraph concludes by emphasizing the foundational nature of this type of optimization problem and hints at the upcoming discussion on multivariate functions in the next video.

Mindmap
Keywords
💡Managerial Economics
Managerial Economics is the application of economic concepts to managerial decision-making. It involves using economic theories and quantitative methods to optimize business operations and performance. In the video, it serves as the overarching theme, with the focus on how to approach decision-making as optimization problems within a firm or organization.
💡Decision Making
Decision making refers to the process of selecting a course of action from among multiple alternatives. In the context of the video, it is a central concept where economic agents, such as firms or individuals, make choices to maximize utility or profits. The video emphasizes that decision making in managerial economics is often framed as an optimization problem.
💡Optimization
Optimization is the process of selecting the best solution from all possible alternatives. It is a key concept in the video, where the goal is to find values for choice variables that lead to the most desirable outcome, such as maximizing profit or minimizing cost. The video discusses how optimization is approached mathematically using objective functions and derivatives.
💡Choice Variables
Choice variables are the factors or inputs that an economic agent can control in making a decision. They are central to the optimization process. In the video, the choice variables might represent the price a firm sets or the amount of advertising it does, which are then used to calculate the objective function, such as profit.
💡Objective Function
An objective function is a mathematical function that defines the goal of an optimization problem. It expresses the relationship between choice variables and the outcome of interest. In the video, the objective function is used to represent a firm's profit in relation to its choice variables, which could include price and advertising.
💡Univariate Function
A univariate function is a function of a single variable. In the context of the video, the focus is on optimizing a univariate objective function, which simplifies the optimization process by considering only one choice variable at a time. This approach is useful for understanding the basic principles of optimization before moving on to more complex multivariate functions.
💡Continuous Choice Space
Continuous choice space refers to a scenario where there are an infinite number of possible choices. The video mentions that optimizing in a continuous space requires the use of calculus, specifically derivatives, to find the optimal value. This is in contrast to a discrete choice space where there is a finite set of options that can be easily evaluated.
💡Derivatives
Derivatives are a fundamental concept in calculus and are used to find the rate at which a function is changing. In the video, derivatives are essential for optimizing functions in a continuous choice space. The first derivative indicates whether the function is increasing or decreasing, and the second derivative helps determine if a point is a maximum or minimum.
💡First Order Condition (FOC)
The first order condition is a mathematical condition that arises in optimization problems. It is found by setting the first derivative of the objective function equal to zero. In the video, the FOC is identified as the starting point for finding the optimal value of the choice variable that maximizes or minimizes the objective function.
💡Second Derivative
The second derivative is the derivative of the first derivative of a function. It provides information about the concavity of the function. In the video, the sign of the second derivative is used to determine if a point is a maximum or minimum. A negative second derivative indicates a maximum, while a positive second derivative indicates a minimum.
💡Concavity
Concavity refers to the curvature of a function. A function is said to be concave if its graph curves inwards like a bowl, indicating that the second derivative is negative. In the video, concavity is discussed in relation to the second derivative, helping to determine the nature of the optimal point found by the first order condition.
Highlights

Managerial economics focuses on decision making, which can be by firms, governments, or individuals.

Decision making is approached as optimization problems with different priorities such as maximizing utility or profit.

Economic agents control choice variables to optimize outcomes, which are related through the objective function.

Objective functions mathematically relate choice variables to outcomes of interest, like a firm's profit.

Univariate objective functions have a single choice variable, which simplifies the optimization process.

Continuous choice bases involve an infinite number of possibilities, necessitating the use of derivatives for optimization.

Derivatives are fundamental in finding the optimal value of a function; a positive derivative indicates an increasing function.

The first order condition (FOC) is found by setting the derivative of the objective function to zero.

The sign of the first derivative determines if the function is increasing or decreasing, guiding towards the optimal value.

The second derivative test is used to determine if a critical point is a maximum or minimum by its sign.

A negative second derivative indicates a maximum, while a positive one indicates a minimum.

The second derivative's sign also corresponds to the concavity of the function, with a negative sign indicating a downward-facing parabola.

An example is provided with the objective function f(x) = -x^2 + 10x + 5, which is optimized by finding the value of x that maximizes it.

The maximizing value of x in the example is found to be 5, indicating the maximum value of the function at that point.

The maximum value of the example objective function is calculated to be 30 when x is 5.

This method of optimizing a univariate objective function is foundational and will be applied throughout the course.

Upcoming topics will cover optimization methods for multivariate objective functions, expanding on the concepts introduced.

Transcripts

Browse More Related Video

Introduction to Optimization

Analyzing a function with its derivative | AP Calculus AB | Khan Academy

Use the Second Derivative Test to Find Any Extrema and Saddle Points: f(x,y) = -4x^2 + 8y^2 - 3

Finding Absolute Extrema (Max/Min) on an Open Interval (a, b)

Maxima , minima and point of inflection

Business Calculus - Math 1329 - Section 4.4 - Applications of Exponential and Logarithmic Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Optimization TechniquesManagerial EconomicsDecision MakingMathematical ModelingDerivativesProfit MaximizationUtility MaximizationContinuous ChoiceFirst Order ConditionSecond DerivativeParabolic Functions