Business Calculus - Math 1329 - Section 4.4 - Applications of Exponential and Logarithmic Functions
TLDRThis video script delves into the application of exponential and logarithmic functions, focusing on the analysis of the function f(x) = x * e^(3x). The presenter explores the function's zeros, end behavior, and uses calculus to find extrema, which are applicable in real-world scenarios. The function's graph is characterized by its behavior at negative infinity and a horizontal asymptote at positive infinity. The derivative and second derivative are calculated to determine the function's increasing/decreasing nature and concavity, leading to the identification of a maximum and an inflection point. The script also addresses an optimization problem involving the present value of land, using continuous compound interest to determine the optimal time to sell for maximizing profit. The problem is solved using derivatives to find the critical point that represents the maximum present value, which is found to be after 100 years.
Takeaways
- 📈 The function f(x) = x * e^(3x) has a zero at x = 0 and exhibits different end behaviors: it goes to negative infinity on the left and approaches 0 (horizontal asymptote) on the right.
- 🧮 To analyze the end behavior of functions, take limits as x approaches positive and negative infinity, which can be evaluated using L'Hôpital's rule for indeterminate forms.
- 📌 The first derivative of f(x) = x * e^(-3x) is found by applying the product rule and can be factored to find critical points.
- 🔍 Setting the first derivative equal to zero gives critical points which help determine intervals of increase or decrease.
- ✍️ A sign chart for the first derivative indicates that there is a maximum at x = 1/3 due to the change from positive to negative.
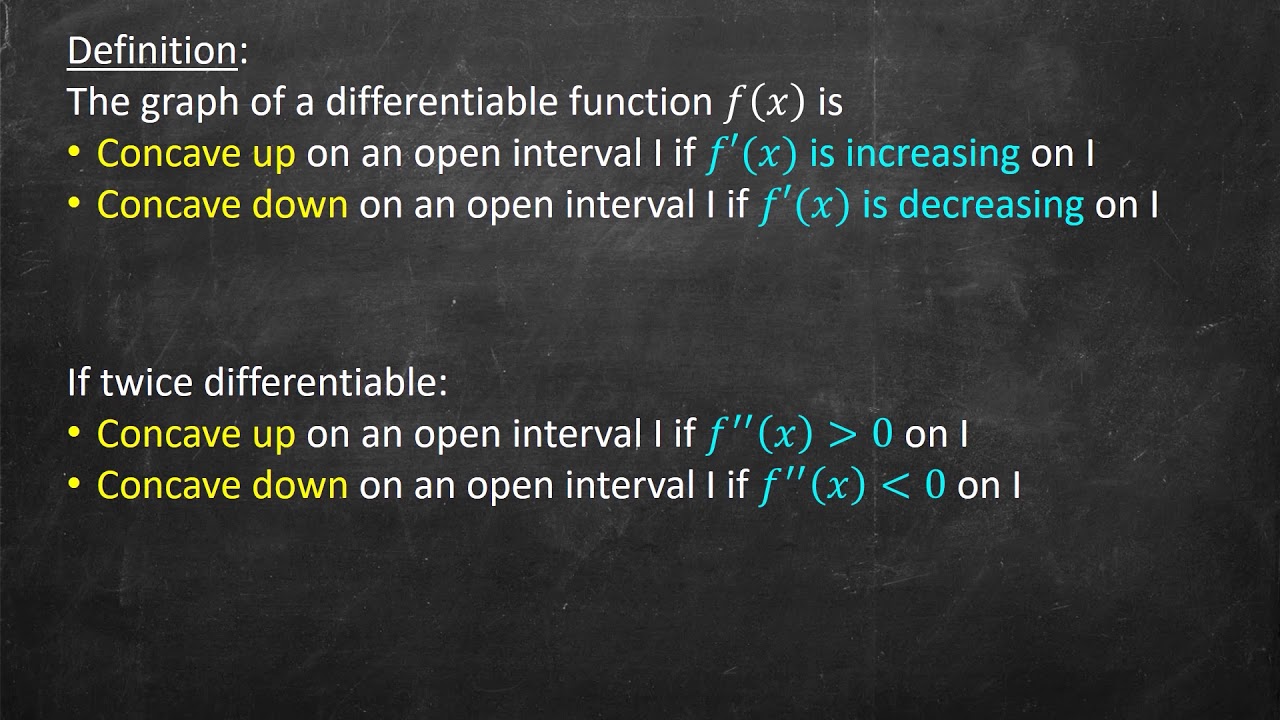
- 📉 The second derivative test is used to find points of inflection; setting the second derivative to zero and solving for x gives the inflection point at x = 2/3.
- 📚 The concept of continuous compound interest is used to derive the present value function P(T) = V(T) * e^(-RT), where V(T) is the future value function and R is the interest rate.
- 💡 To maximize the present value of an asset, take the derivative of the present value function and find its critical points.
- 🔢 For the land valuation problem, the critical point occurs at T = 100 years, indicating that Bob should sell the land after 100 years to maximize its present value.
- 📘 A sign chart for the derivative of the present value function confirms that the critical point found is indeed a maximum.
- 🏞 The application of exponential and logarithmic functions along with calculus can be used to solve real-world problems, such as optimizing the sale time of an asset to maximize its present value.
- 📈 Understanding the behavior of complex functions through calculus helps in predicting and optimizing outcomes in various scenarios.
Q & A
What is the function f(x) discussed in the transcript?
-The function f(x) discussed is f(x) = x * e^(3x).
What is the first step in analyzing the function f(x)?
-The first step is to find the zeros of the function, which occurs when f(x) equals 0.
What does setting x * e^(-3x) equal to 0 yield in terms of solutions?
-It yields that x equals 0, since e^(-3x) cannot equal 0 for any real number x.
How does the function behave as x approaches negative infinity?
-As x approaches negative infinity, the function approaches negative infinity as well.
What is the horizontal asymptote of the function as x approaches positive infinity?
-The horizontal asymptote is y = 0 as x approaches positive infinity.
How is the derivative of f(x) = x * e^(-3x) found?
-The derivative is found using the product rule, resulting in f'(x) = (1 - 3x) * e^(-3x).
What does the second derivative of f(x) tell us about the function's concavity?
-The second derivative tells us that the function is concave down before x = 1/3 and concave up after x = 1/3, indicating an inflection point at x = 1/3.
What is the significance of the inflection point in the context of the function's graph?
-The inflection point at x = 2/3 indicates a change in the concavity of the function, from concave down to concave up.
In the context of Bob's land, what is the formula for the present value (P) of a future value (V) with continuous compounding?
-The formula for the present value is P = V * e^(-RT), where R is the interest rate and T is the time in years.
What is the critical value for T that maximizes the present value of Bob's land?
-The critical value for T that maximizes the present value is T = 100 years.
What is the conclusion for Bob regarding the best time to sell his land to maximize its present value?
-The conclusion is that Bob should sell the land in 100 years to maximize its present value.
Outlines
📈 Analyzing Complex Functions with Calculus
The video begins by introducing section 4.4, focusing on the application of exponential and logarithmic functions. The host aims to explore the graphs of more complex functions, not just basic exponentials and logarithms, and plans to use calculus to find extrema of these functions for real-world applications. The first problem involves the function f(x) = x * e^(3x). The zeros of the function are determined, revealing that x = 0 is the only zero, indicating the graph passes through the origin. The end behavior of the function is analyzed by examining the limits as x approaches negative and positive infinity, leading to conclusions about the graph's behavior at the ends. The use of L'Hôpital's rule is mentioned for evaluating limits of the form ∞/∞.
🧮 Derivatives and End Behavior of Functions
The host calculates the first derivative of the given function and uses it to find critical points where the derivative is zero. By analyzing the sign of the derivative, a sign chart is created to determine the intervals where the function is increasing or decreasing, and to identify a maximum point at x = 1/3. The second derivative is then taken to determine concavity and find an inflection point at x = 2/3. Using this information, a rough sketch of the function's graph is outlined, showing the function's behavior from negative infinity to its horizontal asymptote at zero on the right side.
🏞️ Optimizing Land Value with Continuous Compounding
The video presents a problem involving Bob, who owns land with a future value given by V(T) = 12,000 * e^(√T) dollars, with the interest rate at 5% compounded continuously. The goal is to determine the optimal time for Bob to sell the land to maximize its present value. The formula for present value with continuous compounding is derived and applied to create a function P(T) for the present value of the land. By taking the derivative of this function and finding critical points, the host determines that the present value is maximized after 100 years, suggesting that Bob should consider selling the land at that time for the highest present value.
🎓 Conclusion and Final Thoughts
The video concludes with a summary of the key points discussed, emphasizing the importance of understanding the behavior of complex functions and how to maximize present value in financial contexts. The host thanks the viewers for watching and hints at future topics to be covered in the next session.
Mindmap
Keywords
💡Exponential Functions
💡Logarithmic Functions
💡Derivatives
💡Extrema
💡Horizontal Asymptote
💡Continuous Compound Interest
💡Present Value
💡Optimization
💡Limit
💡L'Hôpital's Rule
💡Inflection Point
Highlights
The function f(x) = x * e^(3x) is explored, with its graph and behavior analyzed using calculus.
The zeros of the function are found, revealing the function passes through the origin.
The end behavior of the function is determined, showing it approaches negative infinity on the left and levels off to 0 on the right, indicating a horizontal asymptote.
L'Hôpital's rule is applied to evaluate the limit of the function as x approaches infinity.
The derivative of the function is taken to find intervals of increase and decrease, and to identify extrema.
A sign chart is constructed to analyze the behavior of the function, indicating a maximum at x = 1/3.
The second derivative is calculated to determine concavity and locate an inflection point at x = 2/3.
A sketch of the function's graph is described, illustrating its shape and key features.
An optimization problem is presented involving the present value of land owned by Bob, with future value given by V(T) = 12,000 * e^(sqrt(T)) dollars.
The concept of continuous compound interest is used to formulate an equation for the present value of the land.
The present value function P(T) is derived and simplified to maximize Bob's return on selling the land.
The derivative of the present value function is taken to find critical points for optimization.
A critical value at T = 100 years is identified as the point to maximize the present value of the land.
A sign chart confirms the critical point as an absolute maximum, advising Bob to wait 100 years for the maximum present value.
Practical implications of the optimization problem are discussed, considering the time frame and potential beneficiaries.
The video concludes with a summary of the findings and a prompt to join the next session.
Transcripts
Browse More Related Video

Finding Concavity and Inflection Points
Concavity and the 2nd Derivative Test

Fiding Relative Max, Min and Inflection Point with Derivatives F4

Calculus I: Finding Intervals of Concavity and Inflection point

Calculus: Maximum and minimum values on an interval

Increasing/decreasing functions [IB Maths AI SL/HL]
5.0 / 5 (0 votes)
Thanks for rating: