Calculus Review for Economics: Basic Derivatives
TLDRThe video script provides an in-depth explanation of derivatives in calculus and their practical applications, particularly in economics. It begins by defining derivatives as the slope of a function at a specific point, using the concept of 'rise over run' or 'change in Y over change in X'. The script illustrates the process of finding derivatives through examples, including a quadratic function and a more complex function involving exponents and constants. It emphasizes the importance of understanding the mathematical rules behind derivatives. The video also explores the concept of marginal cost in economics, explaining how the derivative of a total cost function can be used to determine the marginal cost at any given quantity. The script concludes with a discussion on fixed costs and how they differ from marginal costs. The presenter offers to answer questions in the comments section and promises a follow-up video on partial derivatives.
Takeaways
- 📈 Derivatives represent the slope of a function at a specific point, which can be thought of as the rate of change of 'rise over run'.
- 🎓 The notation for derivatives can be written using the Greek letter 'Δ' (delta) or the regular letter 'd' to denote 'change in'.
- 📚 The derivative of a function like y = 2x^2 - 5x + 4 is found by applying the power rule: multiply the exponent by the coefficient and then subtract 1 from the exponent.
- ⛰ The derivative of a constant, such as 4 in the function, is always zero because the slope of a horizontal line (constant value) is zero.
- 🔍 Setting the derivative equal to zero can help find critical points on a curve, such as minimum or maximum values.
- 📌 In economics, derivatives are used to find marginal values like marginal cost, which is the additional cost of producing one more unit.
- 💰 The marginal cost function can be derived from the total cost function by taking its derivative with respect to quantity.
- 📊 A fixed cost, which does not change with the number of units produced, is not included in the marginal cost function.
- 🧮 To find the marginal cost for a specific unit, plug the quantity into the marginal cost function.
- 🔑 Understanding why mathematical rules work is as important as knowing the rules themselves for a deeper comprehension of the subject.
- 📘 The process of finding derivatives involves focusing on the exponents and using the established procedures, which can be applied to more complex functions as well.
- 🔄 For more advanced applications, partial derivatives are used, which will be covered in a follow-up video.
Q & A
What does a derivative represent in the context of calculus?
-A derivative represents the slope of a function at a particular point, or the slope of the tangent line to a curve at that point. It is calculated as the change in Y (rise) over the change in X (run).
What is the symbol used to denote the derivative of a function with respect to x?
-The symbol used to denote the derivative is 'dy/dx' or 'd/dx', where 'dy' represents the change in the dependent variable Y, and 'dx' represents the change in the independent variable X.
How do you find the derivative of a function like y = 2x^2 - 5x + 4?
-To find the derivative, you apply the power rule, which states that you multiply the exponent by the coefficient and then subtract one from the exponent. For y = 2x^2 - 5x + 4, the derivative would be 4x - 5.
Why is the derivative of a constant, such as 4 in the function y = 2x^2 - 5x + 4, always zero?
-The derivative of a constant is zero because a constant does not change with respect to the independent variable X. Therefore, the slope of a constant function is always flat, which means it has a slope of zero.
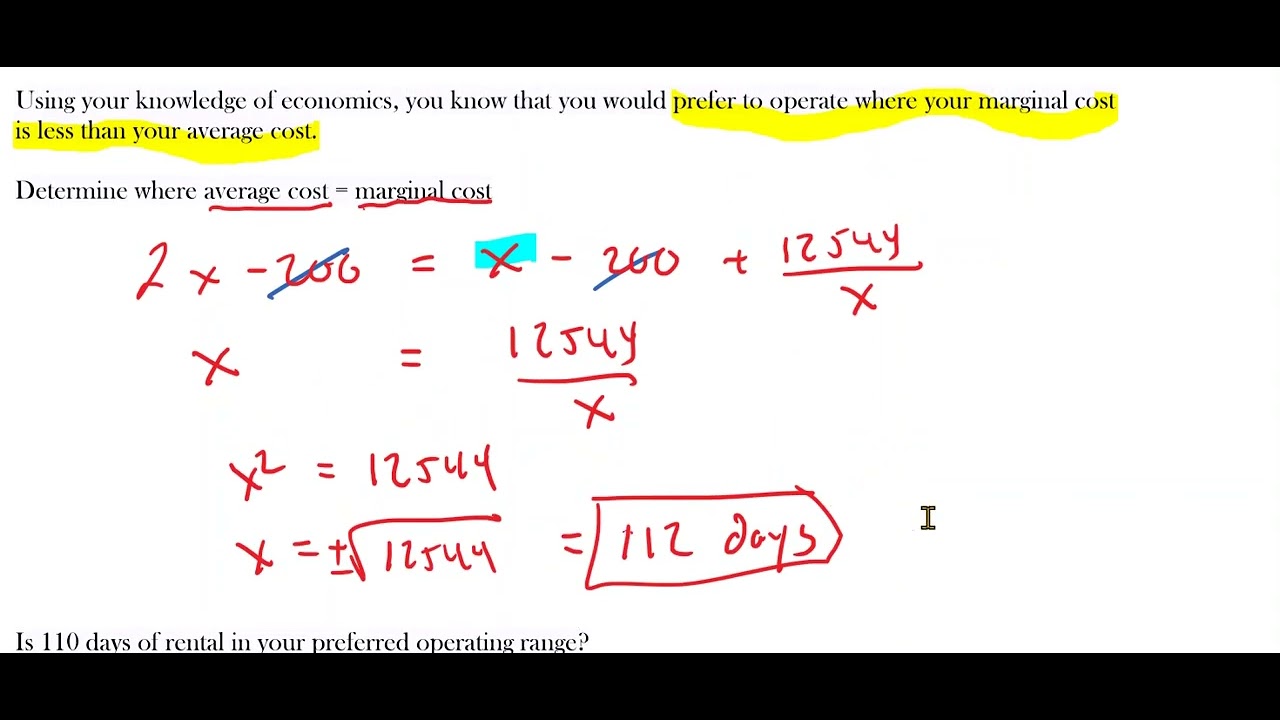
How can you use the derivative to find the minimum cost if a parabola represents the cost of doing something?
-To find the minimum cost, you set the derivative (which represents the slope) equal to zero and solve for the independent variable X. The point where the slope is zero corresponds to the minimum or maximum of the parabola.
What is the marginal cost function in relation to a total cost function?
-The marginal cost function is the derivative of the total cost function with respect to the quantity. It represents the additional cost incurred when producing one more unit of a good.
How does the concept of marginal cost relate to the fixed cost in a cost function?
-The fixed cost is the amount that does not change with the production of more units and is also the cost when no units are produced. It is a part of the total cost function but does not affect the marginal cost, which is concerned with the change in total cost as the quantity changes.
What is the significance of finding the marginal cost for producing the fourth unit?
-The marginal cost for the fourth unit represents the additional cost of producing that specific unit. It is useful for understanding the cost structure and making decisions about production levels.
How can you find the total cost of producing all four units using the total cost function?
-To find the total cost of producing all four units, you plug the quantity (4 in this case) into the total cost function and then add the fixed cost to the result.
What is the role of derivatives in economics, particularly in relation to the concept of marginals?
-Derivatives, which represent slopes, are crucial in economics for understanding marginal concepts such as marginal cost, marginal benefit, marginal tax rate, and marginal utility. They help in determining how an economic variable changes with respect to another variable.
Why is it important to understand not just the mathematical rule but also the reason behind it when dealing with derivatives?
-Understanding the reason behind the mathematical rule helps in applying the rule correctly and interpreting the results accurately. It provides a deeper insight into the behavior of the function and its implications in real-world scenarios.
Outlines
📚 Introduction to Derivatives and Their Applications
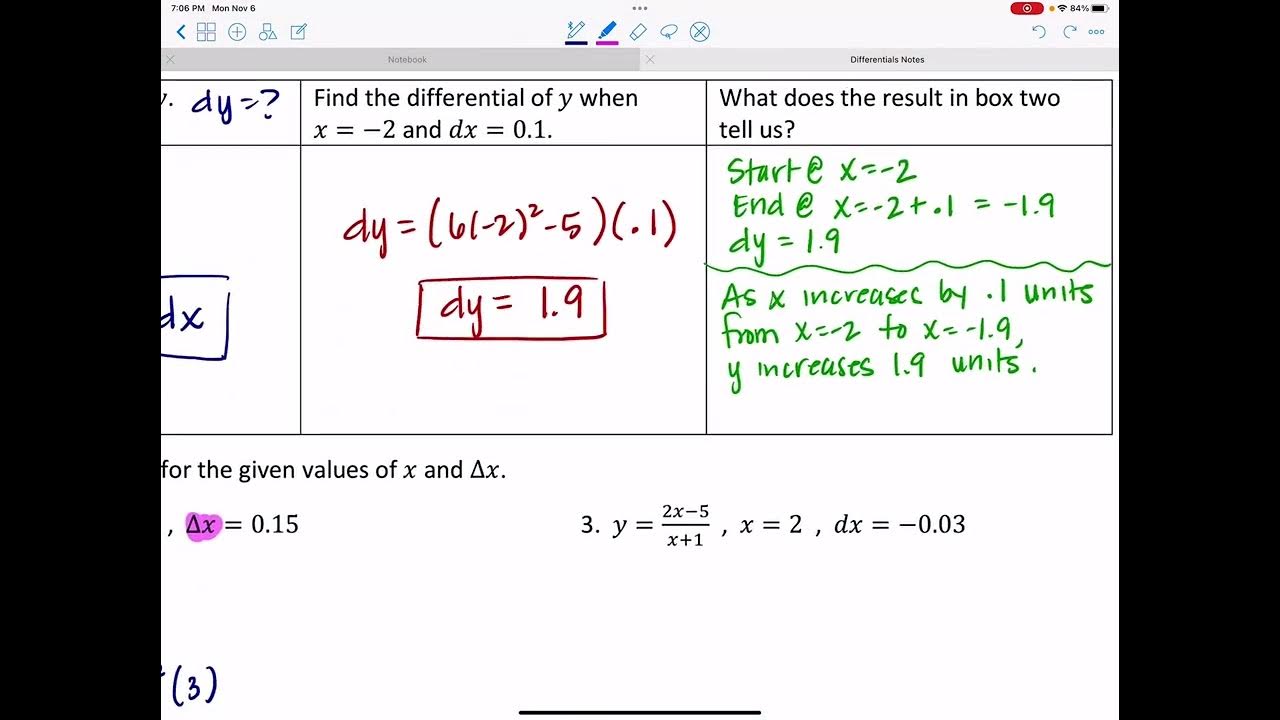
The first paragraph introduces the concept of derivatives in calculus, explaining that they represent the slope of a function at a specific point, or the slope of the tangent line to a curve at that point. The explanation uses the term 'rise over run' to describe the slope, and introduces the notation dy/dx to represent the derivative. An example is given using the function y = 2x^2 - 5x + 4, where the derivative is calculated by applying the power rule. The constant term's derivative is zero, as it represents a horizontal line with no slope. The paragraph concludes with a discussion on how derivatives can be used to find the slope of a function at any point, and how this can be applied to find the minimum cost in a given scenario, such as production costs.
🔍 Finding Minimum Values and Marginal Concepts
The second paragraph delves into the application of derivatives to find minimum values of a function, which corresponds to points where the slope is zero. It guides through the process of setting the derivative equal to zero and solving for the variable to find the point of minimum value. The example continues with the function from the previous paragraph, showing how to find the minimum cost when x equals 1.25. The paragraph also touches on the concept of marginal cost in economics, explaining how the derivative of a total cost function gives the marginal cost, and how this can be used to determine the cost of producing additional units. The process of finding the derivative of a more complex function is also demonstrated, emphasizing the importance of focusing on exponents and applying the power rule.
📈 Derivatives in Economics: Marginal Cost and Total Cost
The third paragraph discusses the prevalence of derivatives in economic studies, particularly in relation to marginal concepts such as marginal cost, benefit, tax rate, and utility. It explains that derivatives, representing slope, are used to determine how costs change with an increase in production. The paragraph illustrates how to derive a marginal cost function from a total cost function and how to calculate the marginal cost for a specific unit of production. It also differentiates between fixed costs, which do not change with production volume, and marginal costs, which are the additional costs associated with producing one more unit. The example provided calculates the marginal cost of producing the fourth unit and contrasts it with the total cost of producing four units, highlighting the utility of derivatives in economic analysis.
Mindmap
Keywords
💡Derivative
💡Slope
💡Tangent Line
💡Change in Y over Change in X (Δy/Δx)
💡Exponent
💡Quadratic Equation
💡Minimum Cost
💡Marginal Cost
💡Fixed Cost
💡Partial Derivatives
💡Economic Studies
Highlights
Derivatives provide the slope of a function at a point, which is equivalent to the slope of the tangent line at that point.
Slope is defined as rise over run, which can be mathematically expressed as the change in Y over the change in X.
The symbol Δ (delta) represents change, and dy/dx denotes the change in Y over the change in X, indicating the slope.
The power rule for derivatives states that for a function y = ax^n, the derivative is found by multiplying the exponent by the coefficient and then subtracting 1 from the exponent.
The derivative of a constant term, such as 4 in the example, is always zero because the slope of a constant function is flat.
Derivatives can be used to find the minimum or maximum values of a function by setting the derivative equal to zero and solving for the variable.
In economics, derivatives are used to find marginal values, such as marginal cost, which is the additional cost of producing one more unit.
The marginal cost function can be derived from the total cost function by taking the derivative with respect to the quantity.
Fixed costs are included in the total cost function but do not contribute to the marginal cost, as they do not change with the production of additional units.
To find the marginal cost for a specific unit, such as the fourth unit, plug the unit number into the marginal cost function.
The total cost of producing multiple units can be found by plugging the quantity into the total cost function and adding the fixed cost.
Derivatives are essential in economics for understanding how costs change as production levels vary.
The process of finding derivatives involves focusing on the exponents and applying the power rule method.
For more complex functions, the same principles of derivatives apply, requiring attention to the exponents and the use of the power rule.
Derivatives are used to find the slope of a function at any given point, which can be useful for various applications in economics.
Understanding the 'why' behind mathematical rules, such as the derivative, is crucial for applying them effectively in practical scenarios.
The video provides a comprehensive review of derivatives and their application in economics, with examples and explanations.
Upcoming content will cover partial derivatives and their use in economics, offering further insight into advanced economic concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: