AP Calculus AB: Lesson 6.4 Rectilinear Motion
TLDRIn this educational video, Michelle Krummel explores rectilinear motion, focusing on the relationship between velocity and displacement. She explains how integrating velocity functions over a given interval yields displacement, and emphasizes the difference between net distance and total distance traveled. Through examples, she demonstrates calculating position and distance using definite integrals and the importance of understanding a particle's motion direction. The lesson concludes with estimating integral values using various Riemann sums and calculating average acceleration, providing a comprehensive understanding of motion analysis.
Takeaways
- 📚 The concept of rectilinear motion, or motion in a plane, is discussed, emphasizing the relationship between the integral of velocity and displacement.
- 📈 The definite integral of a velocity function from a to b can be used to find the net change in position, or displacement, of an object.
- 🔢 The net distance traveled by a particle can be calculated by integrating the absolute value of the velocity function over a given interval.
- 🚀 An example is provided where the velocity function is given by v(t) = 2t^2 - 18, and the position at different times is calculated using integration.
- 📉 The script explains how to find the total distance traveled by integrating the absolute value of the velocity function, even when the direction changes multiple times.
- 📝 The importance of considering the sign of the net change in position is highlighted, with the use of absolute values to represent distances as positive quantities.
- 📉 The process of finding the position of a particle at a given time involves integrating the velocity function from a known position to the desired time.
- 🔍 The script provides a step-by-step example of calculating the position of a particle at time t=4, given its position at t=2, using integration.
- 📊 The concept of using a graph to determine the areas under the velocity curve to find displacement is introduced, with an example of calculating the position at time t=3 from t=8.
- ⏱️ The acceleration of a particle at a specific time is found by taking the derivative of the velocity function, which is illustrated with a graph.
- 📏 The script concludes with various methods of estimating integral values, such as using Riemann sums and the trapezoidal rule, and their applications in motion problems.
Q & A
What is rectilinear motion?
-Rectilinear motion refers to motion in a straight line along a plane. It is the simplest form of motion where an object moves in a single direction without changing its course.
How is the net change of an antiderivative function on a closed interval from 'a' to 'b' calculated?
-The net change of an antiderivative function on a closed interval from 'a' to 'b' is calculated by evaluating the antiderivative function at 'b' and subtracting the evaluation of the antiderivative function at 'a'.
What is the relationship between integrating a velocity function and displacement?
-Integrating a velocity function over an interval gives the net change in position, which is also known as displacement. The velocity is the rate of change of position, so integrating it provides the total distance an object has moved from its initial position.
How can you find the total distance traveled by a particle when its velocity changes direction multiple times?
-To find the total distance traveled by a particle when its velocity changes direction multiple times, you can integrate the absolute value of the velocity function over the interval of interest. This will give the sum of the distances traveled in all directions.
What is the formula for calculating the position of a particle at time 't' given its velocity function?
-The position of a particle at time 't' can be calculated using the formula p(t) = p(a) + ∫(v(t) dt) from 'a' to 't', where p(a) is the initial position at time 'a' and v(t) is the velocity function.
What is the difference between net distance and total distance in the context of motion?
-Net distance refers to the straight-line distance from the starting point to the final position, considering direction, and can be negative. Total distance, on the other hand, is the sum of all distances traveled regardless of direction and is always a positive value.
How do you find the position of a particle at a given time when the velocity function and initial position at another time are known?
-To find the position of a particle at a given time, you set up a definite integral of the velocity function from the initial time to the desired time, add the result to the initial position at the starting time, and solve for the position at the desired time.
What is the significance of the x-intercepts in the context of integrating the absolute value of a velocity function?
-The x-intercepts are significant because they indicate the points where the velocity changes direction. When integrating the absolute value of a velocity function to find total distance, you need to separate the intervals at these points and consider the direction of motion on each interval.
How can you estimate the value of a definite integral using a Riemann sum with equal subintervals?
-To estimate the value of a definite integral using a Riemann sum with equal subintervals, you divide the interval into equal parts, choose a representative value (such as the left, right, or midpoint) from each subinterval, multiply each by the width of the subinterval, and sum these products.
What is the formula for calculating average acceleration over a time interval?
-The formula for calculating average acceleration over a time interval from 't1' to 't2' is the change in velocity (v(t2) - v(t1)) divided by the change in time (t2 - t1).
Outlines
📘 Introduction to Rectilinear Motion and Definite Integrals
Michelle Krummel introduces the concept of rectilinear motion and how definite integrals can be used to find the net change of a function over an interval. She explains that integrating a rate of change function, such as velocity, gives the net change in position, known as displacement. By integrating the absolute value of the velocity function, we can find the total distance traveled. The process is illustrated through an example problem involving the integration of a velocity function to determine the position of a particle at a specific time.
🧮 Evaluating Definite Integrals to Find Net Distance
Krummel continues by discussing the method of finding net distance traveled by integrating the velocity function. She explains how to split intervals into sub-intervals where the particle moves in the same direction, calculating the net distance for each and summing the absolute values. This method is demonstrated through another example, emphasizing the use of definite integrals and anti-derivatives to solve for position changes. The importance of correctly evaluating integrals, either by hand or using a calculator, is highlighted.
📊 Total Distance Traveled and Absolute Value Integrals
The lesson shifts focus to calculating the total distance traveled by integrating the absolute value of the velocity function. Krummel explains how to handle integrals when the velocity function changes sign, requiring separation into sub-intervals and applying absolute values. She demonstrates this with a problem involving a velocity function that crosses the x-axis, explaining the steps to integrate and adjust for negative intervals. The total distance is computed, showing the difference between net and total distance.
🔢 Using Definite Integrals for Position and Velocity Calculations
Krummel addresses how to find the position of a particle at different times using definite integrals of the velocity function. She presents a method to calculate changes in position by integrating velocity over specific intervals. An example problem is solved, showing the steps to integrate and adjust for starting positions. The concept of net distance versus total distance is reinforced, emphasizing the importance of considering direction changes and using absolute values in integrals.
📐 Riemann Sums and Trapezoidal Rule for Integral Approximations
The lesson concludes with an explanation of using Riemann sums and the trapezoidal rule to approximate definite integrals. Krummel demonstrates how to apply left, right, and midpoint Riemann sums to estimate the value of integrals over specified intervals. She also explains the trapezoidal rule, showing how to average the bases of sub-intervals to improve approximation accuracy. Example problems illustrate the application of these methods, providing a comprehensive understanding of integral approximations in various contexts.
Mindmap
Keywords
💡Rectilinear motion
💡Definite integral
💡Antiderivative
💡Net change
💡Displacement
💡Velocity function
💡Total distance
💡Sub-intervals
💡Absolute value
💡Acceleration
Highlights
Rectilinear motion, or motion in a plane, is explored using definite integrals to evaluate the net change of antiderivative functions.
Integrating a rate of change function results in the net amount of change, applicable to motion problems where velocity is the rate of change of position.
Displacement, the net change in position, is obtained by integrating the velocity function over a given interval.
Total distance traveled by a particle can be found by integrating the absolute value of the velocity function.
Example provided with velocity function v(t) = 2t^2 - 18, calculating position and displacement from t=2 to t=4.
The concept of net distance and total distance is differentiated, with examples of their calculation.
Integration of the absolute value of velocity function is demonstrated to find total distance traveled.
The importance of considering direction changes in motion when calculating total distance is discussed.
An example with velocity function v(t) = 3t^2 + 6 is used to find position at different times and total distance traveled.
The method of using a definite integral to find the change in position when given initial and final positions is illustrated.
The use of signed areas in calculating net distance traveled, especially when motion includes direction changes, is explained.
An example with velocity function v(t) = 0.5e^(t)(t - 2)^3 is given to find position at different times using definite integrals.
The calculation of total distance traveled using the integral of the absolute value of a complex velocity function is demonstrated.
The application of the definite integral in determining the position of a particle when the velocity function and initial position are known is shown.
The concept of acceleration as the derivative of velocity is introduced, with an example of calculating it from a given velocity function.
The use of different Riemann sums (left, right, midpoint) to estimate the value of a definite integral is explained with examples.
The calculation of average acceleration over an interval and its approximation to instantaneous acceleration is discussed.
The lesson concludes with a summary of the concepts covered and a preview of the next topic on accumulation of change functions.
Transcripts
Browse More Related Video
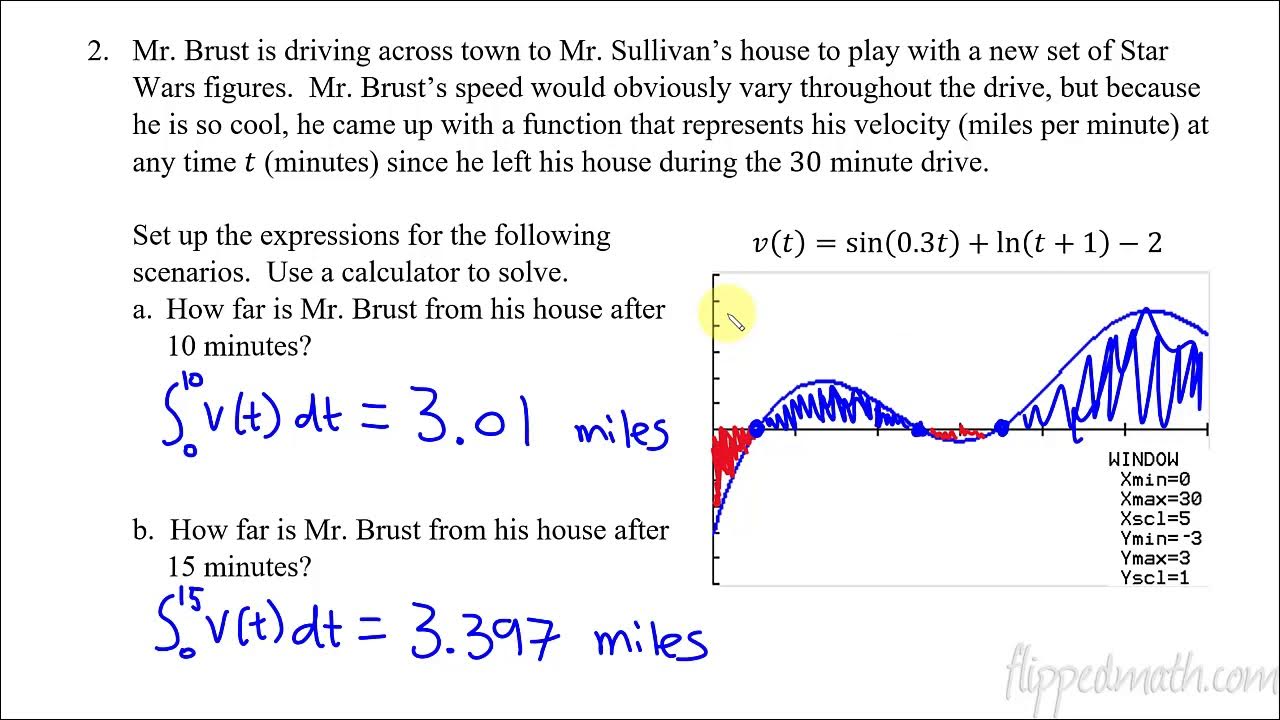
Calculus AB/BC – 8.2 Connecting Position, Velocity, and Acceleration of Functions Using Integrals
Distance and Displacement
AP Calculus AB: Lesson 6.5 Accumulation of Change
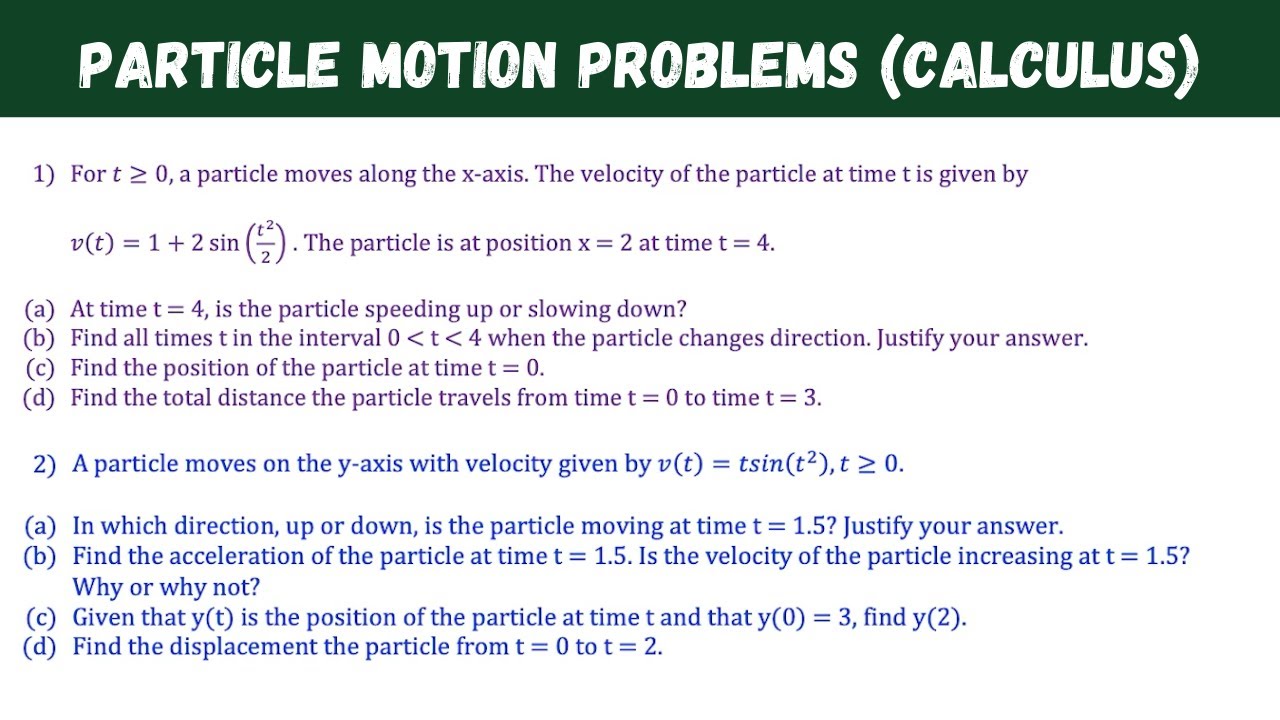
Particle motion problems - Calculus
Position, Distance & Displacement in Physics - [1-2-1]
2.2 Kinematics in One Dimension | General Physics
5.0 / 5 (0 votes)
Thanks for rating: