The Volume of a Cylindrical Wedge with Triangular Cross-Sections

Andrew Misseldine
13 May 202008:41
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging lecture, the presenter delves into the complexities of calculating volumes using cross-sectional slicing, a method that can be quite challenging. The lecture focuses on a specific example involving a wedge cut from a circular cylinder by two planes, one perpendicular to the cylinder's axis and the other at a 30-degree angle. The presenter guides viewers through the process of describing the solid and finding its volume by stacking right-angled cross-sections, each resembling a triangle with a 30-degree angle. Utilizing the tangent ratio to determine the height of these triangles, the presenter simplifies the problem using symmetry and integral calculus. The final step involves integrating the area function with respect to x, resulting in the exact volume of the wedge. The lecture concludes with an invitation for viewers to engage with the content by liking, subscribing, and asking questions, encouraging further exploration of volume calculation through integrals.

Takeaways
  • ๐Ÿ“š The lecture focuses on finding the volume of a solid using cross-sectional slicing, which can be challenging and requires multiple examples for clarity.
  • ๐Ÿ” The example involves a wedge cut out from a circular cylinder of radius four by two planes, one perpendicular to the axis of the cylinder and the other at a 30-degree angle to the x-axis.
  • ๐Ÿ“ The cross-sections are perpendicular to the x-axis and resemble right triangles, with the height determined by the tangent of a 30-degree angle.
  • ๐Ÿงฎ The volume of the wedge is calculated by integrating the area function across the x-axis, taking advantage of symmetry to simplify the integral.
  • ๐Ÿ“ˆ The base of the solid is described by a semicircle with a radius of 4, and the equation y = โˆš(6t - x^2) is used to represent this base.
  • ๐Ÿค” The height of the cross-sections is found using the tangent ratio, which relates the opposite side (height H) to the adjacent side (y-coordinate) in a right triangle.
  • ๐ŸŒ The area of each cross-section is calculated as 1/2 * base * height, where the base is the y-coordinate and the height is y times the tangent of 30 degrees.
  • โœ… The integral to find the volume is set up as the integral from 0 to 4 of (16 - x^2) with respect to x, divided by the square root of 3.
  • ๐Ÿงฎ The antiderivative of the integral is found, leading to the final volume calculation of the wedge as 128/โˆš3 times 3, which simplifies to 128โˆš3.
  • ๐Ÿ“‰ Rationalizing the denominator is mentioned as an option for those who prefer to avoid square roots, but it's not necessary for this calculation.
  • ๐Ÿ“ The final volume of the wedge cut from the cylinder is given as an exact value, which can be approximated if needed by using a calculator.
Q & A

  • What is the shape of the solid described in the lecture?

    -The solid is a wedge cut out of a circular cylinder of radius four by two planes.

  • What is the orientation of the first plane relative to the axis of the cylinder?

    -The first plane is perpendicular to the axis of the cylinder.

  • At what angle does the second plane intersect the x-axis of the XY plane?

    -The second plane intersects the x-axis of the XY plane at a 30-degree angle or ฯ€/6 radians.

  • What is the radius of the base circle of the solid?

    -The radius of the base circle is 4 units.

  • How is the equation of the semicircle described in terms of x and y?

    -The equation of the semicircle is y = โˆš(6)t - x^2.

  • What shape are the cross-sections of the solid perpendicular to the x-axis?

    -The cross-sections are right triangles with a 30-degree angle.

  • How is the height of the cross-sectional triangles related to the y-coordinate?

    -The height of the cross-sectional triangles is y times the tangent of 30 degrees, which simplifies to y/โˆš3.

  • What is the area of one of the cross-sectional triangles in terms of y?

    -The area of one of the cross-sectional triangles is (1/2) * y * (y/โˆš3), which simplifies to y^2 / (2โˆš3).

  • What are the bounds of integration for the volume of the wedge?

    -The bounds of integration for the volume of the wedge are from x = -4 to x = 4.

  • How does the volume of the wedge relate to the integral of the area function?

    -The volume of the wedge is the integral of the area function a(x) dx from 0 to 4, using symmetry to simplify the calculation.

  • What is the final expression for the volume of the wedge after integrating?

    -The final expression for the volume of the wedge is (64/3โˆš3) after integrating and simplifying the area function over the given bounds.

  • How can the final volume be calculated for an approximate value?

    -The final volume can be calculated for an approximate value by inputting the exact expression (64/3โˆš3) into a calculator.

Outlines
00:00
๐Ÿ“ Finding Volume of a Wedge Cut from a Cylinder

This paragraph discusses finding the volume of a wedge cut out of a circular cylinder using cross-sectional slicing. The scenario involves two planes cutting a wedge out of the cylinder, with one plane perpendicular to the cylinder's axis and the other at a 30-degree angle. The process involves describing the solid, stacking cross sections perpendicular to the x-axis, and calculating the area of each right triangle cross-section. The integration process is explained, considering symmetry and the area function derived from the base circle's equation. The paragraph concludes with the exact volume calculation and a mention of the upcoming content.

05:02
๐Ÿ“Š Integral Calculation and Symmetry Utilization

This paragraph delves into the integration process and symmetry utilization for calculating the volume of the wedge cut from a cylinder. It discusses setting up the integral for volume calculation, determining the bounds of integration, and simplifying the integral using symmetry. The area function derived from the base circle's equation is integrated, leading to the antiderivative calculation. Symmetry allows simplification of the integral, resulting in an exact volume calculation. The paragraph ends with an invitation to engage with the content further and a closing remark.

Mindmap
Keywords
๐Ÿ’กCross-sectional slicing
Cross-sectional slicing is a method used to find the volume of an object by dividing it into thin slices and calculating the volume of each slice. In the video, this technique is used to determine the volume of a wedge cut out from a cylindrical shape. The concept is central to the video's theme of calculating volumes using calculus.
๐Ÿ’กWedge
A wedge is a triangular slice that has been removed from a larger shape, in this case, a circular cylinder. The wedge is the solid in question, and the video discusses how to calculate its volume. It is a key concept as the entire lecture revolves around finding the volume of this specific geometric figure.
๐Ÿ’กCylindrical shape
A cylindrical shape is a three-dimensional figure with straight parallel sides and a circular or oval cross-section. In the video, the cylinder has a radius of four and serves as the base from which a wedge is removed. The cylindrical shape is essential as it provides the context for the wedge's formation and the problem at hand.
๐Ÿ’กPlanes
Planes in geometry are two-dimensional surfaces that extend infinitely in all directions. In the context of the video, two planes intersect with the cylinder to create the wedge. One plane is perpendicular to the axis of the cylinder, and the other is at a 30-degree angle to the x-axis of the XY plane. These planes are crucial for defining the boundaries of the wedge.
๐Ÿ’กVolume
Volume refers to the amount of space an object occupies, typically measured in cubic units. The primary goal of the video is to calculate the volume of the wedge using calculus. Volume is a fundamental concept in the study of geometry and is the central focus of the video's mathematical exploration.
๐Ÿ’กIntegration
Integration is a mathematical technique used to find the area under a curve, which can be extended to calculate the volume of a three-dimensional shape. In the video, integration is used to sum up the volumes of the cross-sectional slices to find the total volume of the wedge. It is a key method in the video's approach to solving the volume problem.
๐Ÿ’กSymmetry
Symmetry in mathematics refers to the property of an object to be invariant to some transformations, such as reflection or rotation. The video mentions the use of symmetry to simplify the integral used to calculate the volume. This concept is applied to reduce the complexity of the integral and make the calculation more manageable.
๐Ÿ’กRight triangle
A right triangle is a triangle with one angle measuring 90 degrees. In the video, the cross-sections of the wedge are described as right triangles, which are used to calculate the area of each slice. The right triangle is a basic geometric shape that plays a significant role in the calculation of the wedge's volume.
๐Ÿ’กTangent ratio
The tangent ratio, often abbreviated as 'tan', is a trigonometric function that relates the angle of a right triangle to the ratio of the opposite side to the adjacent side. In the video, the tangent ratio is used to determine the height of the right triangles in the cross-sections, which is essential for calculating their areas.
๐Ÿ’กTrigonometric functions
Trigonometric functions are mathematical functions that relate the angles of a triangle to the lengths of its sides. In the context of the video, sine and cosine of 30 degrees are used to express the tangent of 30 degrees, which is necessary for finding the height of the right triangles in the cross-sectional slices.
๐Ÿ’กAntiderivatives
Antiderivatives, also known as integrals or primitives, are functions whose derivative is a given function. In the video, the antiderivative is used to find the area under the curve described by the cross-sectional slices, which is then used to calculate the volume of the wedge. The concept of antiderivatives is central to the integration process demonstrated in the video.
Highlights

Introduction to a challenging concept of finding volumes using cross-sectional slicing.

Description of a solid formed by a wedge cut out of a circular cylinder by two planes.

Illustration from James Stewart's calculus textbook used for visual aid.

One plane is perpendicular to the axis of the cylinder, simplifying the cross-sectional analysis.

The other plane intersects the x-axis at a 30-degree angle, introducing aๆ–œไธ‰่ง’๏ผˆobtuse angle).

Use of symmetry in the integration process to simplify calculations.

Derivation of the equation for the base circle of the solid with a radius of 4.

Description of the cross-sections as right triangles with a 30-degree angle.

Application of the tangent ratio to find the height of the cross-sectional triangles.

Substitution of sine and cosine values for 30 degrees to rationalize the expression.

Integration of the area function over the x-axis to find the volume of the solid.

Identification of the bounds of integration from -4 to 4, utilizing the symmetry of the solid.

Transformation of the integral using the relationship between y and x derived from the semicircle's equation.

Integration of the modified area function to find the antiderivative and subsequently the volume.

Final calculation of the volume results in an expression involving square root of 3.

Option to approximate or use exact values for practical applications.

Encouragement for viewers to like, subscribe, and ask questions for further clarification.

Anticipation of the next video covering more about volume calculations using integrals.

Transcripts

Browse More Related Video

Calculus 1 Lecture 5.2: Volume of Solids By Disks and Washers Method

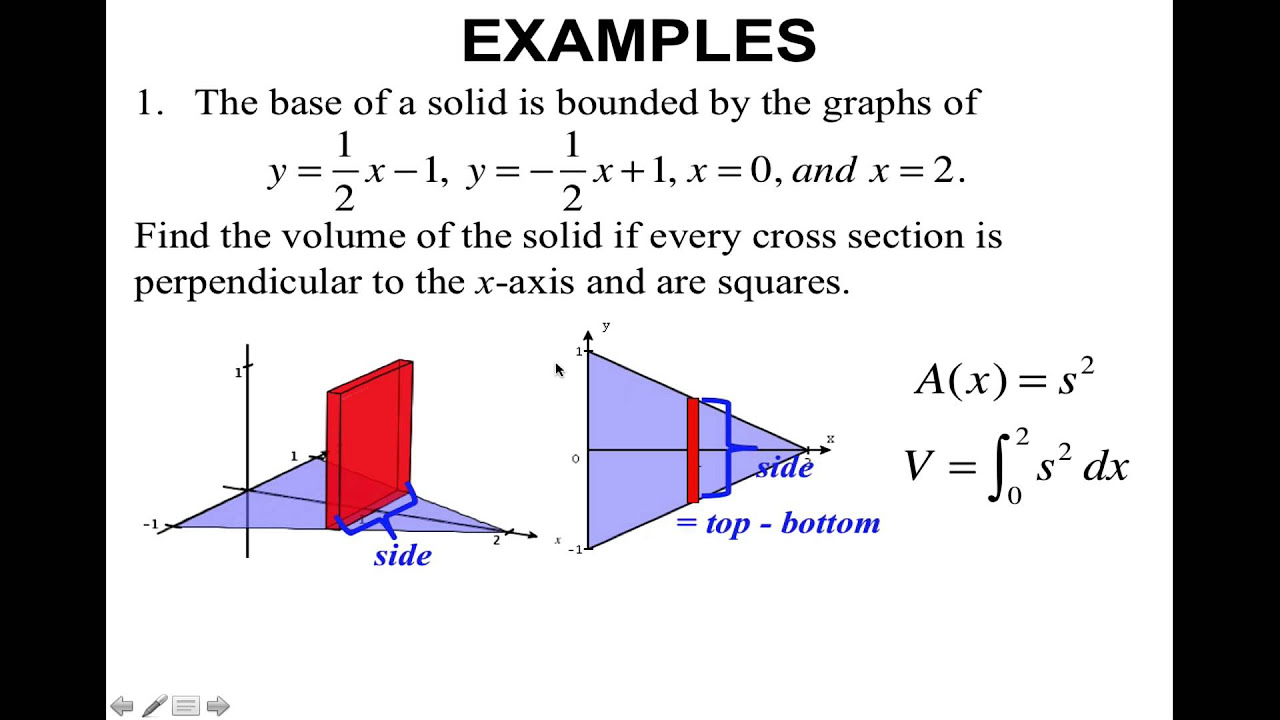
Topic 43-Volumes of Solids with Known Cross Sections

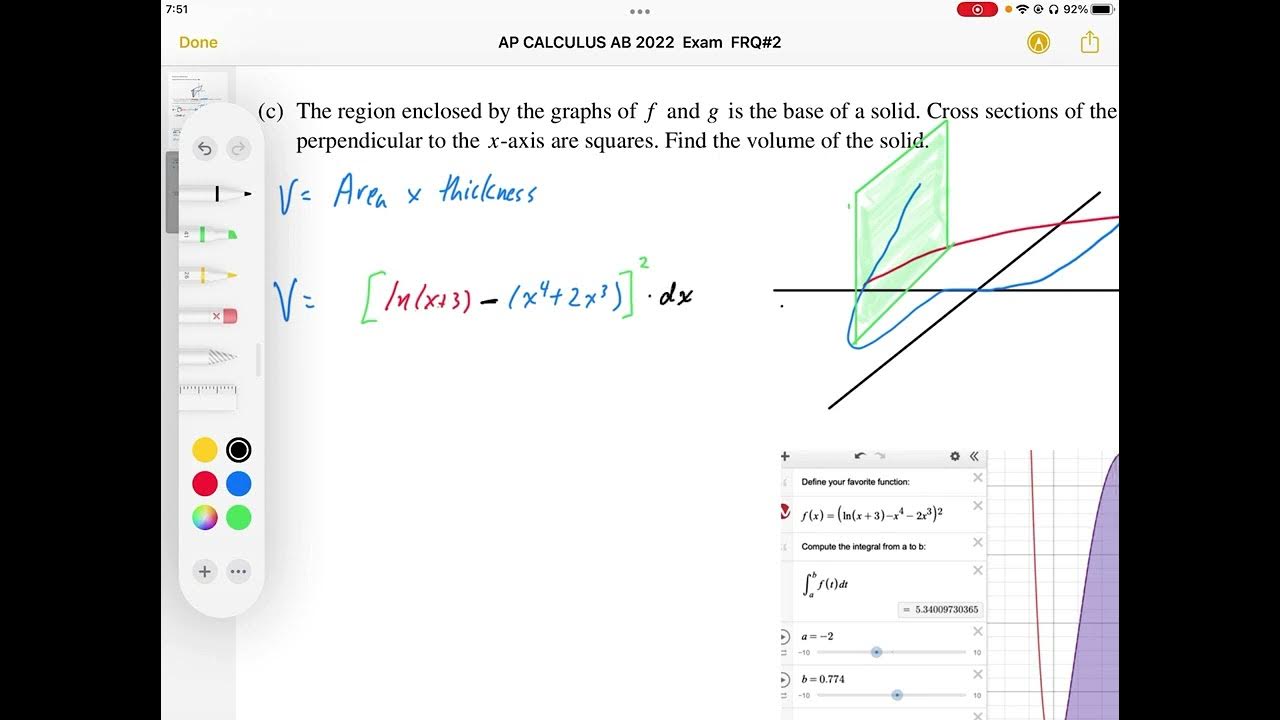
AP CALCULUS AB 2022 Exam Full Solution FRQ#2c

Finding the Volumes of Known Cross Sections โ– AP Calculus AB

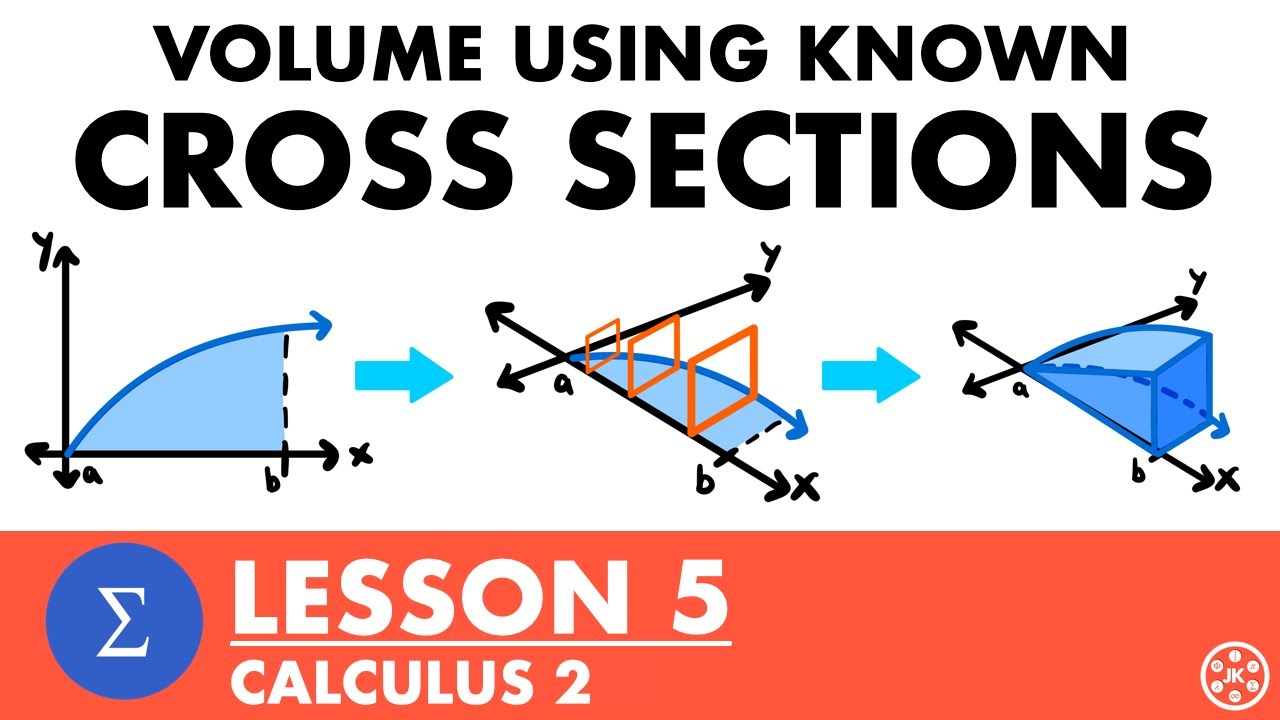
Volume Using Known Cross Sections (Slicing) | Calculus 2 Lesson 5 - JK Math

2022 AP Calculus BC Exam FRQ #5

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Volume CalculationCross-Sectional SlicingCalculus LectureIntegralsGeometryMathematical AnalysisEducational ContentProblem SolvingMath TutorialSTEMJames Stewart