Finding the Volumes of Known Cross Sections ❖ AP Calculus AB
TLDRThe video script discusses a common topic in AP Calculus AB tests: calculating the volume of a solid with known cross-sections perpendicular to the x-axis. The presenter uses the example of a solid bounded by the curves y=x^2 and y=x+2, where each cross-section is an isosceles right triangle. The process involves sketching the region, identifying the base and the cross-section shape, and integrating the area of the cross-section from the points of intersection. The presenter emphasizes the importance of visualizing the 3D shape and understanding that the distance between the curves represents the length of the sides of the cross-sections. The video concludes with an integral setup and a reminder that the integration should be manageable for viewers. The final answer to the example is 4.05, offering a clear demonstration of how to approach such problems.
Takeaways
- 📚 The video discusses calculating the volume of a solid with known cross-sections, a common topic in AP Calculus AB free response sections.
- 📏 To find the volume, integrate the area of the cross-section from point A to B, perpendicular to the x-axis.
- 📈 The base of the solid is the region between two curves, and the solid grows three-dimensionally from this base.
- 🔍 Cross-sections can be any geometric shape that can be calculated for area, such as squares, semicircles, or right triangles.
- 📐 For the example given, the base is bounded by y=x^2 and y=x+2, and each cross-section is an isosceles right triangle.
- 🖋️ Sketch the region and curves to visualize the solid and the cross-sections, which helps in understanding the problem.
- ✍️ The area of an isosceles right triangle is calculated as one-half times the square of the length of one leg (1/2 * H^2).
- 🔢 To find the distance between the curves, subtract the bottom curve from the top curve to get the length of the legs of the triangle.
- 🔍 Points of intersection between the curves are found by setting the two equations equal to each other and solving for x.
- 🧮 The integral to find the volume is set up from the points of intersection, integrating the squared difference of the top and bottom curves, multiplied by 1/2.
- ✅ The final step is to perform the integration, which can be done by hand or using a calculator, to find the volume of the solid.
- 📺 The video emphasizes the importance of understanding the shape of the cross-section, the base of the solid, and the distance between the curves for successful integration.
Q & A
What is the main topic of the video?
-The video is about calculating the volume of a solid with known cross-sections, a common topic tested on the AP Calculus AB exam.
What is the general approach to find the volume of a solid whose cross-sections are perpendicular to the x-axis?
-The general approach is to integrate from point A to B the area of the cross-section.
What is the shape of the base region R in the example provided in the video?
-The base region R is bounded by the curves y = x^2 and y = x + 2.
What type of triangles are the cross-sections of the solid in the example?
-The cross-sections of the solid are isosceles right triangles.
How is the area of an isosceles right triangle calculated?
-The area of an isosceles right triangle is calculated as one-half times the base times the height (1/2 * base * height).
What is the formula used to calculate the volume of the solid in the example?
-The formula used to calculate the volume is 1/2 * (top curve - bottom curve)^2 dx, integrated from the points of intersection.
How does one find the points of intersection for the curves?
-The points of intersection are found by setting the two curves equal to each other and solving for x.
What are the x-values of the points of intersection in the example?
-The x-values of the points of intersection are x = -1 and x = 2.
Why is it important to visualize the distance between the two curves?
-The distance between the two curves determines the length of the sides of the cross-sections, which is essential for calculating the area of the cross-sections and, subsequently, the volume of the solid.
What is the final answer obtained for the volume of the solid in the example?
-The final answer for the volume of the solid in the example is 4.05.
What is the significance of the phrase 'the base of a solid' in volume problems?
-The phrase 'the base of a solid' indicates the region that defines the shape of the cross-sections when calculating the volume of the solid.
How does the choice of cross-section shape affect the volume calculation?
-The choice of cross-section shape determines the formula used to calculate the area of the cross-section, which is then integrated to find the volume. Different shapes require different area formulas.
Outlines
📚 Introduction to Calculating Volumes of Solids with Known Cross-Sections
This paragraph introduces the topic of calculating the volume of solids with known cross-sections, a common subject in AP Calculus AB tests, typically found in the free response section. The method involves integrating the area of cross-sections perpendicular to the x-axis from point A to B. An example is given using a standard parabola y=x^2, where the cross-sections are squares that change in size based on the curve's distance from the x-axis. The paragraph emphasizes the importance of sketching the region and understanding that the cross-sections can be any geometric shape for which the area can be calculated. The first example involves finding the volume of a solid with a base bounded by y=x^2 and y=x+2, where each cross-section is an isosceles right triangle.
📐 Calculating the Area and Setting Up the Integral for Cross-Sections
The paragraph delves into the process of calculating the area of isosceles right triangle cross-sections and setting up the integral to find the volume. It explains that the area of an isosceles right triangle is one-half times the square of the length of its legs, denoted as H. The distance H between the curves y=x^2 and y=x+2 is determined by subtracting the y-values of the curves at a given x. The integral to be solved is then expressed as half the difference of the top curve (x+2) and the bottom curve (x^2), squared, integrated from x=-1 to x=2. The paragraph advises on potential simplification of the integral and encourages viewers to attempt the integration either manually or using a calculator, with the expected result being approximately 4.05 for the volume.
📝 Conclusion and Encouragement for Further Practice
The final paragraph wraps up the discussion by emphasizing the key elements to focus on when approaching volume problems: identifying the base of the solid, the shape of the cross-section, and the need to integrate the area of that shape. It highlights the importance of visualizing the distance between the two curves as it forms part of the area formula, regardless of the shape of the cross-section. The paragraph concludes with a thank you note for watching and an encouragement to subscribe to the channel for more content.
Mindmap
Keywords
💡Volumes of known cross-sections
💡AP Calculus AB test
💡Free response section
💡Solid of revolution
💡Cross-sections
💡Integration
💡Isosceles right triangle
💡Base of the solid
💡Points of intersection
💡Area formula
💡Sketching
Highlights
The video focuses on calculating the volume of solids with known cross-sections, a common topic in AP Calculus AB.
Volumes are typically found by integrating the area of cross-sections perpendicular to the x-axis from point A to B.
The base of the solid is determined by the region between two curves, such as y=x^2 and y=x+2.
Cross-sections can be any geometric shape, as long as the area can be calculated.
An example solid's cross-sections are isosceles right triangles, with the base determined by the distance between curves.
The area of an isosceles right triangle is given by (1/2) * base * height, where base and height are equal.
To find the distance between curves, subtract the bottom curve's equation from the top curve's equation.
Points of intersection between the curves are found by setting the two equations equal to each other and solving for x.
The integral to find the volume is set up as 1/2 * (top curve - bottom curve)^2, integrated from the points of intersection.
The final answer to the example problem is approximately 4.05 cubic units.
The process emphasizes the importance of identifying the base of the solid, the shape of the cross-section, and the distance between curves.
Visualization of the 3-dimensional shape is crucial, even though it cannot be drawn in full.
The distance between the curves is a key component in the area formula for any chosen cross-section shape.
Integration techniques may vary, and calculators can be used for complex integrals.
The video provides a step-by-step guide to setting up and solving the volume problem.
Subscribing to the channel is encouraged for more educational content.
Transcripts
Browse More Related Video

Calculus AB/BC – 8.7 Volumes with Cross Sections: Squares and Rectangles

Calculating volume by known cross sections

Volume of Solids with Known Cross Sections
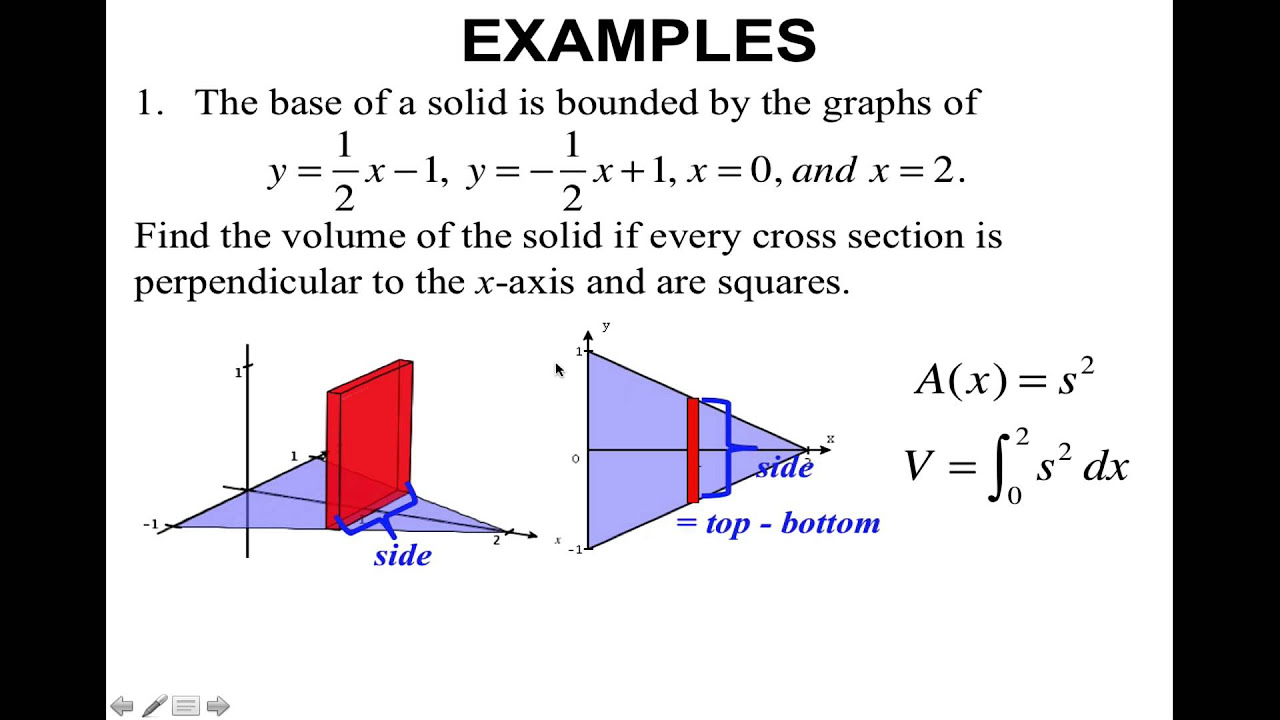
Topic 43-Volumes of Solids with Known Cross Sections

Volume with cross sections: squares and rectangles (no graph) | AP Calculus AB | Khan Academy

Volumes with Known Cross Sections with Calculus, pg 2
5.0 / 5 (0 votes)
Thanks for rating: