2022 Live Review 6 | AP Calculus AB | Working with Applications of Integrals: Part 1

Advanced Placement

26 Apr 202252:03

EducationalLearning

32 Likes 10 Comments

TLDRThe video script is a detailed educational discussion on AP Calculus, specifically focusing on applications of integrals, which is a significant part of the AP exam. The speakers, Mark Corelli and Verge Portelis, both high school teachers, delve into various calculus problems, including differential equations, slope fields, and particle motion. They use a step-by-step approach to guide students through solving problems, emphasizing the importance of understanding the process rather than just the answers. The session includes a mix of theoretical explanations and practical problem-solving, with a focus on helping students prepare for the AP Calculus exam. The transcript also highlights the use of technology, such as calculators, in solving calculus problems and offers a glimpse into the pedagogical methods used to teach complex mathematical concepts to students.

Takeaways

📚 The session focuses on AP Calculus, specifically the applications of integrals, differential equations, slope fields, and particle motion.
🔢 A warm-up problem is presented involving a definite integral to find the value of a function given certain conditions, highlighting the use of the Fundamental Theorem of Calculus.
✅ The importance of setting up integrals correctly is emphasized, ensuring that the known value matches the lower bound for accurate calculations.
🤔 The session discusses the concept of acceleration and velocity in the context of particle motion, differentiating between when a particle is speeding up or slowing down.
📈 The use of slope fields to visualize and solve differential equations is covered, including identifying potential solutions and understanding their graphical representations.
🧮 A detailed walkthrough of solving a differential equation with an initial condition is provided, pointing out common mistakes and emphasizing the steps to find the correct solution.
🚶 The application of calculus to real-world problems, such as modeling velocity and displacement of a person moving along a path, is discussed.
📉 The concept of instantaneous acceleration is introduced, and its relation to the average acceleration over an interval is explained.
🛣️ The session touches on the calculation of total distance traveled by integrating the absolute value of velocity, as opposed to displacement.
🔍 The importance of graph recognition in calculus is highlighted, noting that students should be familiar with parent functions and their graphs without a calculator.
⏱️ The instructors provide a preview of the next session, which will cover more applications of integrals, including area, volume, and rate problems.

Q & A

What is the main topic of discussion in the transcript?
-The main topic of discussion is AP Calculus, specifically focusing on applications of integrals, including differential equations, slope fields, particle motion, and accumulation functions.
What mathematical concept is used to relate the given h(3) to h(7) in the warm-up problem?
-The concept used is the Fundamental Theorem of Calculus (FTC), which allows the calculation of h(7) from h(3) by setting up a definite integral from 3 to 7 of the derivative function h'(x).
What is the purpose of using a calculator for the warm-up problem?
-The purpose of using a calculator is to efficiently solve the problem, as manual calculations involving integration and differentiation can be complex and time-consuming.
What is the context of the particle's motion along the x-axis as described in the transcript?
-The context is a physics problem where the particle's position is given by a function of time, x(t) = sin(2t), and the task is to describe the motion of the particle at a specific time, t = 2π/3.
How does the sign of the velocity and acceleration of the particle at t = 2π/3 help in determining the nature of its motion?
-The sign of the velocity indicates the direction of the particle's motion (left or right), while the sign of the acceleration indicates whether the particle is speeding up (increasing velocity) or slowing down (decreasing velocity). If the velocity and acceleration have the same sign, the particle is speeding up; if they have opposite signs, it is slowing down.
What is the significance of the slope field in the context of differential equations?
-A slope field represents the possible instantaneous rates of change (slopes) of a function at different points in its domain. It is used to visualize and approximate solutions to differential equations.
How does the process of solving a differential equation relate to the concept of anti-differentiation?
-Solving a differential equation often involves finding the anti-derivative (integral) of the given derivative function, which represents the original function whose derivative is the one provided in the equation.
What is the role of the initial condition in solving a differential equation?
-The initial condition provides a specific value or starting point that helps in determining the particular solution to the differential equation from the family of all possible solutions.
Why is it important to understand the parent functions and their graphs in calculus?
-Understanding parent functions and their graphs is crucial as it helps in recognizing patterns, predicting the behavior of more complex functions, and solving problems more efficiently without relying solely on a calculator.
What is the significance of the absolute value in the context of finding the total distance traveled by Craig in the given problem?
-The absolute value is used to ensure that the distance traveled is always a positive quantity, regardless of the direction of motion. It accounts for both eastward and westward movements when calculating the total distance.
How does the concept of integration by parts relate to the process of solving the given problems in the transcript?
-Integration by parts is a specialized technique for integrating products of functions, which is not directly mentioned in the transcript. However, the general concept of integration is central to solving the problems, as it is used to find areas, volumes, and other accumulations represented by the integrals in the problems.

Outlines

00:00

📚 Introduction to AP Calculus: Day 6

The video begins with a casual and humorous introduction, where the host hands over a pen and discusses the importance of having a pen for the session. The main topic of discussion is AP Calculus, with a focus on applications of integrals, differential equations, slope fields, and particle motion. The host, Mark Corelli, and his colleague Verge Portalis, plan to spend two days on these topics, emphasizing their significance in the AP exam. They also present a warm-up problem involving differential equations and the use of a calculator to solve it.

05:01

🔢 Solving Differential Equations and Understanding Motion

The second paragraph delves into solving differential equations using the fundamental theorem of calculus. The hosts illustrate how to approach a problem where the derivative of a function is given, and one must find the value of the function at a different point. They also discuss the motion of a particle along the x-axis, using derivatives to determine velocity and acceleration at a specific time. The emphasis is on understanding the direction and speed of the particle's movement.

10:02

📈 Analyzing Slope Fields and Identifying Particular Solutions

In the third paragraph, the discussion shifts to slope fields and identifying particular solutions to differential equations. The hosts guide viewers on how to recognize the shape of potential solutions by analyzing the slope field. They also touch on the importance of graph recognition and understanding parent functions without the aid of a calculator, which is crucial for success in AP Calculus.

15:04

🎓 Solving Differential Equations and Finding Errors

The fourth paragraph focuses on solving a separable differential equation step by step, identifying the first error in a given solution process. The hosts emphasize the importance of methodically working through each step and understanding the implications of each operation on the solution. They also discuss the significance of initial conditions in finding particular solutions.

20:04

🧮 Calculating Displacement and Analyzing Motion

The fifth paragraph involves calculating displacement and analyzing the motion of an object. The hosts work through a problem involving a particle moving along a path with a given velocity function. They discuss the use of integrals to find displacement and the importance of understanding the context of motion, such as whether an object is speeding up or slowing down.

25:05

🚶‍♂️ Craig's Motion Analysis and Jocelyn's Path

The sixth paragraph presents a scenario where Craig is traveling along a path with a velocity function that changes over time. The hosts calculate Craig's displacement and discuss his position relative to his starting point. They also introduce a table showing Jocelyn's velocity and position at selected times and ask viewers to find the integral of her velocity function over a specific interval.

30:07

🤔 Identifying the Correct Differential Equation

In the seventh paragraph, the hosts challenge viewers to identify the correct differential equation that generates a given slope field. They discuss various strategies for solving this type of problem, including looking for gaps in the slope field, analyzing the behavior of the slopes in different regions, and considering the implications of parallel slope segments.

35:12

📈 Final Thoughts and Preview of Upcoming Sessions

The final paragraph wraps up the session with a summary of the topics covered and a preview of what will be discussed in the next session. The hosts emphasize the importance of understanding the accumulation model and the fundamental theorem of calculus. They also mention that the next session will cover more applications of integrals, including area, volume, and rate problems.

Mindmap

Keywords

💡AP Calculus

AP Calculus is a high school mathematics course that covers the concepts of calculus, which is a branch of mathematics focused on limits, functions, derivatives, integrals, and infinite series. In the video, the speakers are discussing various topics related to AP Calculus, such as differential equations, integrals, and their applications. This is the main theme of the video as it revolves around teaching and understanding calculus concepts.

💡Differential Equations

Differential equations are mathematical equations that involve a function and its derivatives. They are used to describe many phenomena in the real world, from the motion of planets to the spread of diseases. In the script, the speakers discuss differential equations in the context of slope fields and particle motion, which are key concepts in understanding how changes occur over time in various systems.

💡Integrals

Integrals are a fundamental concept in calculus that represent the area under a curve or the accumulation of a quantity. The speakers in the video are preparing to spend two days on applications of integrals, which are crucial for understanding the accumulation of quantities over time and are tested on the AP Calculus exam.

💡Slope Fields

A slope field is a graphical representation that shows the possible slopes of a differential equation at various points in the plane. It helps visualize the solutions to differential equations. In the video, slope fields are mentioned as a topic to be covered, and they are used to discuss the behavior of solutions to differential equations.

💡Particle Motion

Particle motion refers to the movement of a particle along a path as a function of time. It is a common application of calculus, particularly in the context of physics. The script discusses particle motion in relation to the position of a particle along the x-axis, using calculus to find the velocity and acceleration at specific points in time.

💡Anti-differentiation

Anti-differentiation, also known as integration, is the process of finding a function given its derivative. It is the reverse operation to differentiation. The speakers mention anti-differentiation in the context of using derivatives to find the original function, which is essential for solving problems involving accumulation, such as finding the area under a curve.

💡Fundamental Theorem of Calculus (FTC)

The Fundamental Theorem of Calculus is a key theorem that links the concept of the definite integral to the antiderivative. It states that the definite integral of a function can be computed by finding the antiderivative and subtracting the value at the lower limit of integration from the value at the upper limit. In the video, FTC is alluded to when discussing the relationship between derivatives and integrals.

💡Calculator Active Problems

Calculator active problems are those that require the use of a calculator to solve, often due to the complexity or the numerical values involved. In the script, it is noted that a particular problem is calculator active, indicating that students are expected to use a calculator to perform the calculations, which is a common practice in solving AP Calculus problems.

💡Point-Slope Form

Point-slope form is a method used to find the equation of a line given a point on the line and the slope of the line. It is expressed as $ y - y_1 = m(x - x_1) $, where $ m $ is the slope and $ (x_1, y_1) $ is the point on the line. The speakers in the video relate the concept of point-slope form to the process of finding the equation of a tangent line to a curve at a given point.

💡Euler's Method

Euler's method is a numerical technique used to approximate the solution of a first-order differential equation. It is named after the Swiss mathematician Leonhard Euler and is mentioned in the video as a topic that connects to the discussion of derivatives and integrals. Euler's method is used to estimate the value of functions and is applicable in various scientific fields.

💡Displacement

Displacement is the change in position of an object. It is a vector quantity that considers both magnitude and direction. In the context of the video, displacement is discussed in relation to the motion of a particle along a path, where the integral of the velocity function over an interval gives the total displacement during that time period.

Highlights

Mark Corelli and Verge Portelis discuss AP Calculus, focusing on applications of integrals, differential equations, slope fields, and particle motion.

They introduce a warm-up problem involving a differential equation and the use of definite integrals to find the value of a function.

The concept of the Fundamental Theorem of Calculus (FTC) is mentioned, emphasizing its importance in accumulation and anti-differentiation.

Mark and Verge demonstrate how to set up and solve a problem using a calculator for active learning.

The importance of graph recognition and understanding parent functions without a calculator is emphasized.

An example of solving a differential equation with an initial condition is provided, highlighting common mistakes and how to avoid them.

The session includes a practical application of calculus to model Craig's velocity and displacement over a time interval.

The concept of average acceleration is derived and calculated using integrals and the concept of velocity.

The use of absolute value to calculate total distance traveled is explained, emphasizing the difference between displacement and distance.

Jocelyn's motion is analyzed using a table of velocity and position values, and the integral is used to find her displacement over a given time frame.

The problem-solving strategy of working through a problem before looking at the answer choices is recommended for active learning.

The session concludes with a review of the day's topics and a preview of the next day's focus on volume and rate problems.

The importance of understanding the context of motion problems, such as the direction of velocity and acceleration, is discussed.

Mark and Verge illustrate the process of finding a particular solution to a differential equation using given functions and their derivatives.

The significance of correctly interpreting the signs of velocity and acceleration in the context of the x-axis is explained.

An example of sketching a solution curve that passes through a given point using a slope field is provided.

The method for finding the equation of a tangent line to a solution curve at a specific point is demonstrated.

Mark and Verge discuss the interpretation of integrals in the context of accumulation and the calculation of total displacement.

Transcripts

Browse More Related Video

2021 Live Review 6 | AP Calculus AB | Application of Integrals (Part 1)

AP Calculus AB/BC Multiple Choice Practice Test (2012 AP CED Problems)

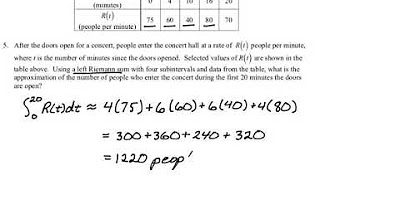
AP Calc AB & BC Practice MC Review Problems #1

2022 Live Review 2 | AP Calculus BC | Euler's Method, Logistics, and Differential Equations

2022 Live Review 8 | AP Calculus AB | Reviewing Multiple-Choice & Free-Response Questions

AP Calculus Practice Exam Part 2 (MC #11-20)

Related Tags

AP Calculus Integral Applications Differential Equations Mark Corelli Verge Portelis Educational Content Mathematical Analysis Exam Preparation Online Learning Calculus Tutorial Academic Webinar