Calculating a p-value for a Two-tailed Test

Matthew Simmons
12 Jul 201803:47
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a clear explanation on how to calculate the p-value for a two-tailed hypothesis test concerning the proportion of voters favoring a particular candidate. The scenario involves testing if the preference for Candidate 'A' significantly differs from a given proportion of 0.86. The script guides the viewer through the process of using a standard normal distribution with a z-test statistic of 1.675. It explains how to find the area under the curve corresponding to the right tail and then double it to account for the left tail, as this is a two-tailed test. The video demonstrates the use of Google Sheets to find the area of the right tail, which is approximately 0.04967, and then multiplies this by two to get the total p-value of 0.0939. The summary emphasizes the step-by-step methodology, making it accessible for viewers to understand hypothesis testing in the context of proportions.

Takeaways
  • 📊 Conducting a two-tailed hypothesis test to determine if the proportion of voters preferring Candidate 'A' significantly differs from 0.86.
  • 📈 The test statistic (z) is calculated to be 1.675, indicating the position relative to the mean in standard deviations.
  • 📉 The p-value is the sum of the areas in the two tails of the standard normal distribution that exceed the test statistic.
  • 🔍 The standard normal distribution is used because the test statistic is a z-score, with a mean of zero and a standard deviation of one.
  • 📍 The right tail area corresponding to the test statistic of 1.675 is found using a tool like Google Sheets.
  • 🔢 The area to the right of 1.675 (right tail area) is approximately 0.04967 when calculated.
  • 🔁 Since it's a two-tailed test, the left tail area is the same as the right tail area, also 0.04967.
  • 🤝 To find the total p-value, multiply the area of one tail by 2, accounting for both tails in the test.
  • 📝 The final p-value, after rounding to four decimal places, is 0.0939, representing the total area in both tails.
  • 🔧 Utilizing tools like Google Sheets can facilitate the calculation of p-values and areas under the standard normal curve.
  • 📚 Keeping more decimal places in intermediate steps can lead to a more accurate final p-value.
  • 👍 The video provides a step-by-step guide on how to calculate the p-value for a two-tailed hypothesis test using a z-score.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is about one sample hypothesis testing, specifically calculating the p-value.

  • What is the context of the study in the video?

    -The context is a study to determine if the proportion of voters who prefer Candidate 'A' is significantly different from 0.86.

  • What type of hypothesis test is being performed?

    -A two-tailed test is being performed.

  • What is the test statistic obtained from the sample data?

    -The test statistic obtained is a z-score of 1.675.

  • What is the p-value in the context of hypothesis testing?

    -The p-value is the area under the curve in the tails of the distribution that represents the probability of observing a test statistic as extreme as, or more extreme than, the one calculated from the sample data.

  • Why is the p-value calculated as the sum of the areas of two tails?

    -The p-value is the sum of the areas of two tails because it's a two-tailed test, which means we are interested in deviations from the null hypothesis in both directions.

  • What distribution is being used for the test statistic in this video?

    -The standard normal distribution is being used since the test statistic is a z-score.

  • What is the mean and standard deviation of the standard normal distribution?

    -The mean of the standard normal distribution is 0 and the standard deviation is 1.

  • How does one find the area of the right-hand tail for the given z-score?

    -One can find the area of the right-hand tail using a tool like Google Sheets, which has a function to compute the area under the normal distribution curve from a given lower bound to positive infinity.

  • What is the calculated area of the right-hand tail for the z-score of 1.675?

    -The calculated area of the right-hand tail is approximately 0.04967.

  • How is the final p-value calculated for a two-tailed test?

    -The final p-value for a two-tailed test is calculated by multiplying the area of one tail by 2, which accounts for the area in both tails.

  • What is the final p-value obtained from the video?

    -The final p-value obtained is 0.0939, after rounding to four decimal places.

Outlines
00:00
📊 Hypothesis Testing and Calculating the p-value

This paragraph introduces a video on hypothesis testing, specifically focusing on calculating the p-value. The context is a study to determine if the proportion of voters favoring Candidate 'A' is significantly different from 0.86, using a two-tailed test. The sample data yields a z-score of 1.675, which is used to find the p-value. The p-value is the combined area under the standard normal distribution curve in the tails beyond the test statistic. The mean for the standard normal distribution is zero, and the test statistic's location to the right signifies the area of the right tail. The left tail's area is identical. Using Google Sheets, the right tail's area is calculated with the lower bound as 1.675 and the upper bound as a very large positive number (represented as six nines). The computed area for the right tail is approximately 0.04967, and since it's a two-tailed test, this value is multiplied by 2 to get the total p-value, which is 0.0939 when rounded to four decimal places.

Mindmap
Keywords
💡hypothesis testing
Hypothesis testing is a statistical method used to determine whether there is enough evidence to support a claim or hypothesis. In the video, hypothesis testing is used to evaluate if the proportion of voters favoring Candidate 'A' significantly differs from 0.86. It is central to the video's theme as it guides the entire process of calculating the p-value.
💡p-value
The p-value is a statistic that measures the strength of the evidence against a null hypothesis. In the context of the video, the p-value is calculated to determine if the observed difference in voter preferences is statistically significant. It is a key concept as the entire video is about finding this value through a two-tailed test.
💡two-tailed test
A two-tailed test is a type of hypothesis test that considers two separate outcomes, one in each tail of the distribution. It is used when the research question is about the difference in proportions, regardless of the direction. In the video, a two-tailed test is performed to see if the proportion of voters for Candidate 'A' is significantly different from 0.86.
💡test statistic
A test statistic is a numerical value computed from sample data that is used to make a decision in hypothesis testing. In the video, the test statistic is represented by 'z', which is equal to 1.675. It is crucial as it determines the location on the standard normal distribution curve for calculating the p-value.
💡z-score
The z-score is a measure of how many standard deviations an element is from the mean. In the video, the z-score of 1.675 is used to identify the position on the standard normal distribution curve. It is significant as it directly influences the calculation of the p-value and the decision-making process in hypothesis testing.
💡standard normal distribution
The standard normal distribution, also known as the Gaussian distribution or bell curve, is a common probability distribution where data points are symmetrically distributed around a mean of zero with a standard deviation of one. The video uses this distribution to find the area under the curve corresponding to the z-score of 1.675.
💡tail area
In the context of hypothesis testing, the tail area refers to the region in the tail(s) of the distribution curve that is beyond the critical value. The video discusses calculating the area of the right and left tails, which together form the p-value for a two-tailed test. Understanding tail areas is essential for interpreting the significance of the test statistic.
💡Google Sheets
Google Sheets is a cloud-based spreadsheet program that allows users to create, edit, and analyze data in a collaborative manner. In the video, Google Sheets is used to calculate the area under the standard normal curve, which helps in finding the p-value. It is an example of how technology can facilitate statistical analysis.
💡mean
The mean, often referred to as the average, is a measure of central tendency in statistics. In the video, the mean is set to zero for the standard normal distribution, which is a key assumption for calculating the z-score and the subsequent p-value.
💡standard deviation
The standard deviation is a measure of the amount of variation or dispersion in a set of values. In the video, the standard deviation is set to one for the standard normal distribution, which is a key characteristic of this distribution and is used in conjunction with the mean to locate the z-score on the curve.
💡null hypothesis
The null hypothesis is a statement of no effect or no difference, which is tested in statistical hypothesis testing. In the video, the null hypothesis is that the proportion of voters favoring Candidate 'A' is equal to 0.86. The p-value calculated will determine if there is enough evidence to reject this null hypothesis.
💡significance level
The significance level, often denoted by alpha, is the threshold p-value below which the null hypothesis is rejected. Although not explicitly mentioned in the video, the concept is implicitly present as the p-value is compared to a predetermined significance level to make a decision about the null hypothesis.
Highlights

The video is about one sample hypothesis testing and calculating the p-value.

The study aims to determine if the proportion of voters who prefer Candidate A is significantly different from 0.86.

A two-tailed test is being performed.

The sample data produces a test statistic z = 1.675.

The p-value is the area of the tails under the curve.

For a two-tailed test, the p-value is the sum of the areas of the right and left tails.

The test statistic z = 1.675 is from the standard normal distribution with mean 0 and standard deviation 1.

The area of the right tail can be found using the test statistic and the standard normal distribution.

The area of the left tail will be the same as the right tail in a two-tailed test.

Google sheets can be used to calculate the area of the right tail with a lower bound of 1.675 and an upper bound of positive infinity.

The area of the right tail is approximately 0.04967.

The area of the left tail is also 0.04967.

The p-value is calculated by multiplying the area of one tail by 2 for a two-tailed test.

The final p-value is 0.0939 when rounded to four decimal places.

The p-value represents the total area of the tails under the curve.

The video provides a step-by-step guide to calculating the p-value in a two-tailed hypothesis test.

The standard normal distribution is used to find the areas of the tails for the p-value calculation.

Google sheets is a useful tool for calculating the area of the tails and finding the p-value.

The video demonstrates the practical application of hypothesis testing in determining if a proportion is significantly different from a given value.

Transcripts

Browse More Related Video

Finding P-Value for a Z-Test Using the TI-84

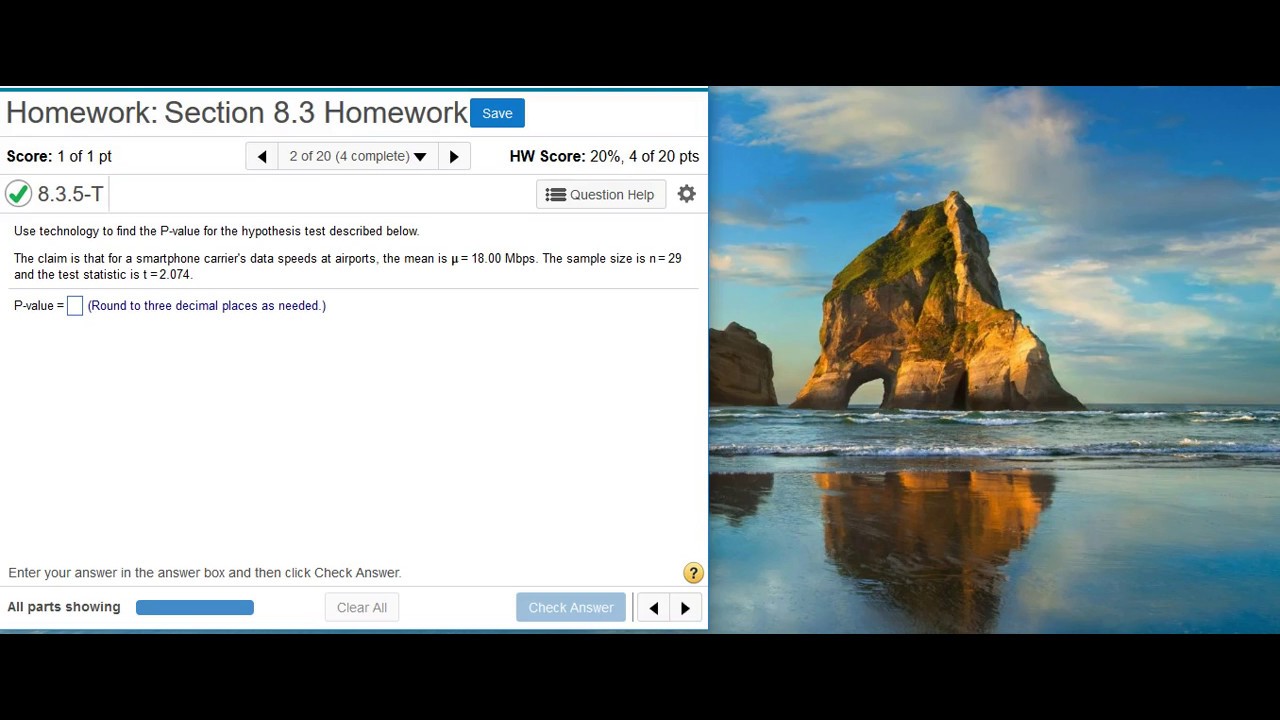
Finding a P-value given the test statistic

Elementary Stats Lesson #18

Elementary Statistics - Chapter 8 - Hypothesis Testing Part 3 Revised

Using a z-score to complete hypothesis testing for a claim of equality

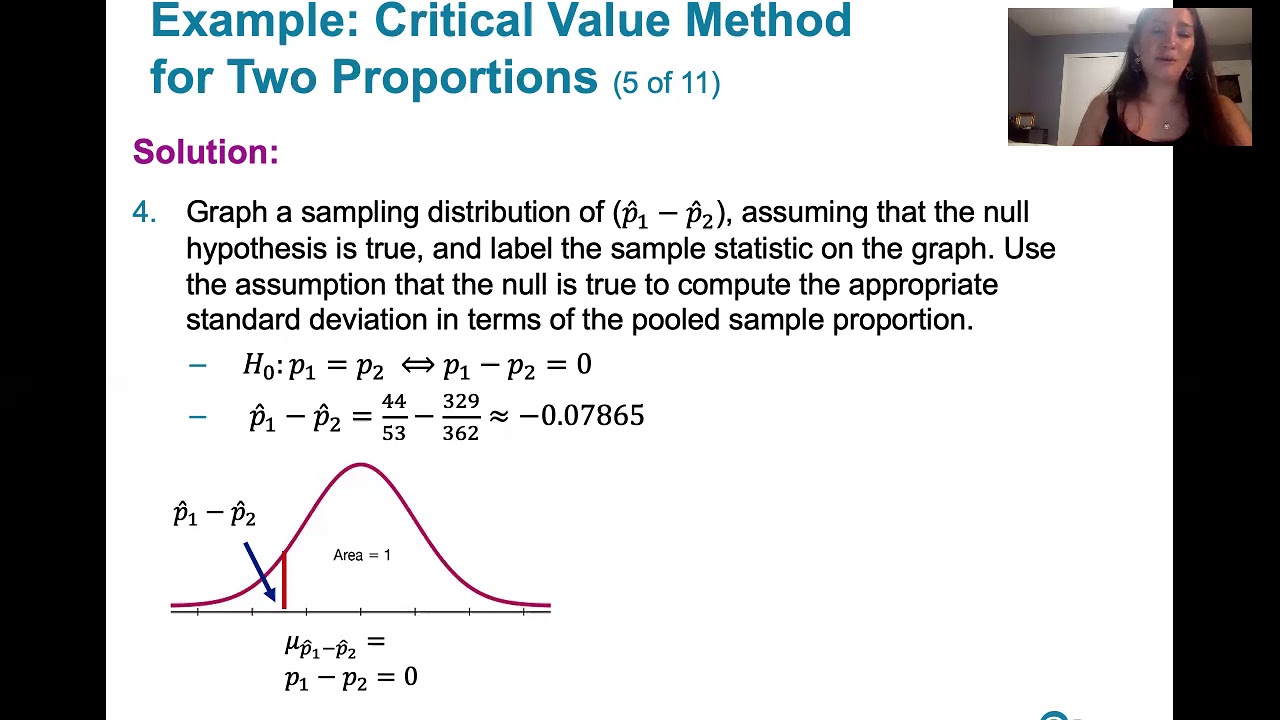
9.1.3 Two Proportions - Example, Critical Value Method, Normal Approximation

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Hypothesis TestingP-Value CalculationTwo-Tailed TestStandard NormalZ-ScoreStatistical AnalysisVoter PreferencesGoogle SheetsData AnalysisProbabilityEducational Content