Applying Generalized Integration Rules To Special Cases
TLDRThis video lesson focuses on applying generalized integration rules to special cases in calculus. The instructor begins with a review of the conditions required for applying a general integration rule, emphasizing that the derivative of the function (dU) must be a factor of the integrand. The power rule for integration is explained, along with how to adjust it for different functions by substituting x with another function U. The video demonstrates how to handle cases where dU is missing a constant factor by multiplying and dividing by that factor, thus making the integrand suitable for the rule. The lesson also covers the integration of exponential functions (e^U) and the natural logarithm (1/U), showing how to manipulate the integrand to fit the rules. Throughout the video, the instructor encourages students to verify their work by differentiating the antiderivative to ensure it matches the original integrand. The summary serves as a guide for students to practice these techniques and prepare for their assignments.
Takeaways
- 📚 To apply a general integration rule, the derivative of the function U (dU) must be a factor of the integrand.
- 🔍 When integrating a function like x^n, the power rule involves adding 1 to the exponent, dividing by the new exponent, and then adding the constant of integration.
- ✅ The integral of a function is its antiderivative, and you can check your work by differentiating the result to see if you get back the original integrand.
- 🚫 If dU is not a factor, you cannot apply the generalized power rule and need to integrate differently, such as by expanding the product.
- 😌 If dU is missing a constant factor, you can multiply and divide by that constant to make it a factor, allowing you to apply the integration rule.
- 📐 If dU is missing a variable factor, you cannot apply the general integration rule and must find another method to integrate.
- 🤔 Multiplying by a constant inside the integral and outside does not change the value of the integral, which can be helpful when adjusting dU to be a factor.
- 📉 When integrating a function multiplied by a constant, you can factor out the constant, as the integral of a function times a constant is the constant times the integral of the function.
- 🎓 The derivative of the integral of e^U is e^U, plus the constant of integration, which is a straightforward rule when dU is present in the integrand.
- 🧮 For the integral of 1/U, provided dU is a factor, it equals the natural log of U plus a constant, which is useful when the integrand is a reciprocal of a function.
- 📝 Always verify your integration work by differentiating the antiderivative; it should match the original integrand, confirming the integration was done correctly.
Q & A
What is the general rule for applying integration to a function other than x?
-The general integration rule can be applied to a function, often denoted as U, if the derivative of that function (dU) is a factor of the integrand.
What is the power rule for integration?
-The power rule for integration states that the integral of x to the nth power is (x to the power of n+1) divided by (n+1) plus the constant of integration.
When can you apply the power rule to an integral with a different function U?
-You can apply the power rule to an integral with a different function U if the derivative of U (dU) is a factor in the integrand.
What is the process if dU is not a factor in the integrand?
-If dU is not a factor in the integrand, you cannot directly apply the generalized power rule. Instead, you may need to expand the product, differentiate and integrate each term, or use other integration techniques.
How can you integrate an expression if dU is missing a constant factor?
-If dU is missing a constant factor, you can multiply and divide by that constant within the integral so that dU becomes a factor, allowing you to apply the generalized rule.
What is the integral of a function multiplied by a constant?
-The integral of a function multiplied by a constant is the same as the constant multiplied by the integral of the function itself. This is because you can factor out the constant from the integral.
How do you verify the correctness of an integral?
-You can verify the correctness of an integral by differentiating the antiderivative with respect to x and checking if you get back to the original integrand.
What is the integral of e to the power of U?
-The integral of e to the power of U is e to the power of U plus a constant (C), provided that the derivative of U (dU) is a factor in the integrand.
What rule is used when integrating 1 over U, provided dU is a factor?
-When integrating 1 over U with dU as a factor, the integral becomes the natural log of U plus a constant (C).
Why can't you apply the power rule if dU is missing a variable factor?
-You cannot apply the power rule if dU is missing a variable factor because doing so would change the type of function you're integrating, which is not allowed.
What is the process for integrating an expression like 3x squared plus 5 squared?
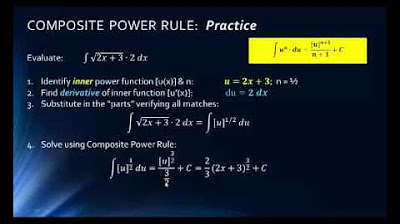
-First, you identify U and dU. If dU is not a factor, you might need to multiply by a constant to make it a factor. Then, apply the power rule to integrate U squared. The result will be a constant times U to the power of (n+1)/(n+1) plus the constant of integration.
Outlines
😀 Understanding Generalized Integration Rules
This paragraph reviews the application of generalized integration rules to special cases. It emphasizes that the power rule can be applied only when the derivative of a function U is a factor in the integrand. The paragraph provides an example of integrating 6X times 3x squared plus 5 squared by substituting it with U squared and applying the power rule after confirming that the derivative of U is a factor. The process of integrating by expanding the product and applying the power rule to each term is also discussed. The importance of checking work by differentiating the antiderivative to obtain the original integrand is highlighted.
😉 Factoring Out Constants in Integration
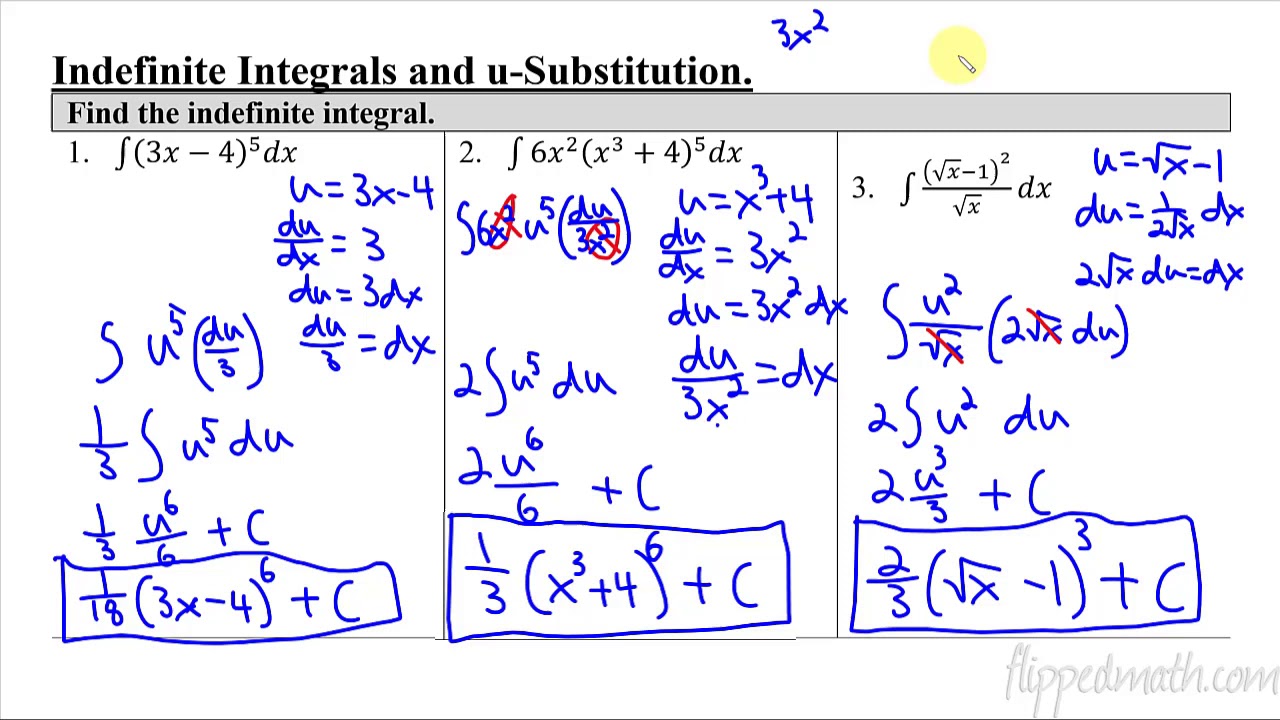
The second paragraph discusses a strategy for integrating when the derivative of U is not directly a factor but is missing a constant factor. It shows how to multiply and divide by a constant to make it a factor, allowing the application of the generalized power rule. An example is given where the integral of (3x squared plus 5 squared) is solved by multiplying by 6 and then dividing by 6 to make the derivative a factor. The paragraph also clarifies that this method is only applicable when the missing factor is constant, not variable.
🎓 Applying Power Rules to Special Cases
The third paragraph continues the discussion on applying power rules to integrals where the derivative of U is missing a constant factor. It demonstrates the process of multiplying by the missing constant and then dividing by the same amount to maintain the integral's value. The paragraph provides an example of integrating 1 minus 2x squared to the third power, showing each step of the integration process, including the correction of a mistake and the final simplification of the integral.
📚 Generalized Power Rule for Special Cases
The fourth paragraph focuses on the application of the generalized power rule to special cases. It explains that if the derivative of U is missing a variable factor, a different integration method must be used. However, if a constant factor is missing, the integral can be manipulated by multiplying and dividing by that constant. The paragraph provides an example of integrating x squared times x cubed minus 1 to the negative second power, showing the steps to correctly apply the power rule.
🧮 Simplifying Integration with Constant Factors
The fifth paragraph illustrates the process of integrating expressions where the derivative of U is missing a constant factor. It provides an example of integrating 4X plus 6 times x squared plus three X plus seven to the negative third power. The paragraph shows how to rewrite the integral to make the derivative of U a factor by multiplying and dividing by the necessary constants, and then applying the power rule.
📈 Applying e^U Integration Rules
The sixth paragraph shifts the focus to integrating expressions involving e to the power of U. It explains that the integral of e to the U is simply e to the U plus C, provided that the derivative of U is a factor in the integrand. The paragraph provides an example of integrating e to the negative x squared, showing how to adjust the integral to make the derivative a factor, and then applying the e to the U rule.
🧐 Factoring for e^U and 1/U Integration
The seventh paragraph deals with the integration of e to the power of U, specifically when U is x squared minus 8X. It shows how to factor and adjust the integral to match the derivative of U with the correct constant factor. The paragraph emphasizes the importance of verifying the integration by differentiating the result to ensure it matches the original integrand.
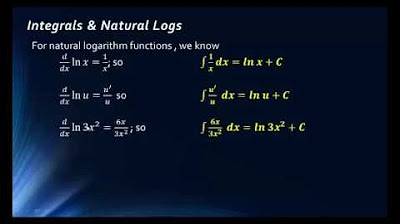
📖 Integration of 1/U and Natural Logarithm
The eighth and final paragraph discusses the integration of 1 over U, which is the natural logarithm of U plus C, provided that the derivative of U is a factor. It warns against mistakenly applying power rules when they are not appropriate, such as when the exponent would result in division by zero. The paragraph provides an example of integrating 1 over (2x - 1), showing the steps to multiply by the necessary constant to make the derivative a factor, and then applying the 1/U integration rule. The importance of checking work by differentiation is reiterated.
Mindmap
Keywords
💡Generalized Integration Rules
💡Power Rule
💡Constant of Integration
💡Derivative of a Function
💡Anti-derivative
💡Factoring out Constants
💡Integration by Substitution
💡Exponential Function
💡Natural Logarithm
💡Integration by Parts
💡Differentiation Under the Integral Sign
Highlights
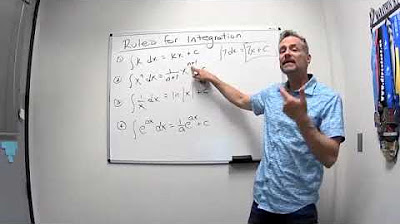
General integration rules can only be applied to a function U if its derivative is a factor of the integrand.
Power rule for integration involves adding one to the exponent, dividing by the new exponent, and adding the constant of integration.
When changing the variable from x to another function U, the same power rule applies provided the derivative of U is in the integrand.
The integral of a function multiplied by a constant is the constant multiplied by the integral of the function.
If the derivative of U is missing a constant factor, it can be introduced by multiplying and dividing by that constant, allowing the application of the generalized rule.
If the derivative of U is missing a variable factor, a general integration rule cannot be applied, and an alternative integration method is required.
The integral of a sum or difference can be split into the sum or difference of each integral.
The integral of e to the U is e to the U plus C, provided the derivative of U is a factor of the integrand.
The integral of 1 over U is the natural log of U plus C, as long as the derivative of U is a factor of the integrand.
Verifying the correctness of an integral by differentiating the antiderivative and checking if it matches the original integrand is a good practice.
To integrate more complex expressions, it may be necessary to expand the product, differentiate, and integrate each term.
Multiplying and dividing by one (using a constant factor) does not change the value of the integral, which can be leveraged to make the integrand fit the rule.
When the derivative of U is not directly a factor, creative manipulation such as multiplying by a missing constant factor and then dividing by the same can sidestep the need for expanding expressions.
The choice between leaving the integral in rational exponent form or converting it to a radical form is a matter of preference and both are acceptable.
The process of integrating by parts or using substitution can be avoided by carefully manipulating the integrand to fit the form required by the generalized rules.
When applying the power rule to an expression like x^n, it is crucial to ensure that the derivative of the function raised to the power is indeed a factor in the integrand.
The integral of a function is an antiderivative, and understanding this relationship is key to verifying the correctness of integration solutions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: