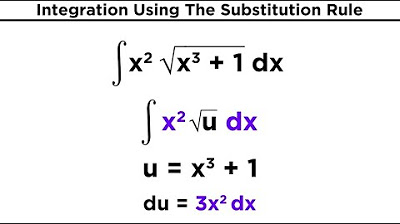
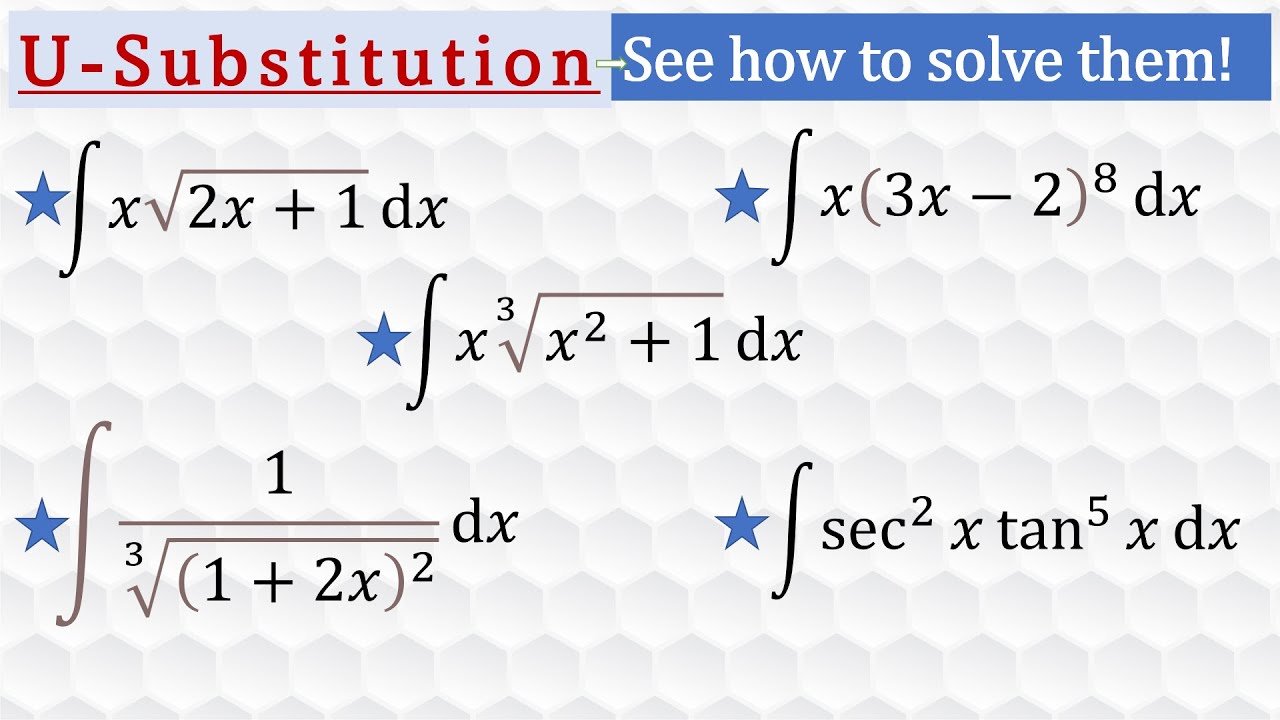
Generalized Integration Rules
TLDRThis lesson dives into the process of generalizing integration rules, focusing on the power rule, the rule for integrating e^x, and the rule for integrating 1/x. The instructor explains how to adapt these rules for different functions by ensuring the derivative of the inner function (du) is a factor of the integrand. The power rule is generalized to integrate functions like U^n, where U can be any expression, not just x. The same principle applies to integrating e^U and 1/U, with the crucial condition that du must be present in the integrand. The video provides several examples to illustrate the process, emphasizing the importance of verifying the antiderivative by differentiating it back to the original integrand. The instructor also highlights situations where the generalized power rule cannot be applied due to the absence of du in the integrand, and how to handle such cases by expanding or manipulating the integral. The lesson concludes with a reminder to always check work for accuracy, reinforcing the key concepts covered.
Takeaways
- 📚 Start by understanding the power rule for integration, which involves multiplying the exponent by one and then dividing by the new exponent, followed by adding the constant of integration.
- 🔄 Use the reverse power rule to check your work by differentiating the antiderivative to see if it matches the original integrand.
- 🎓 When integrating a composite function, ensure that the derivative of the inner function (dU) is a factor of the integrand to apply the generalized power rule correctly.
- 📈 For composite functions, if dU is not a factor, you cannot use the generalized power rule; instead, you may need to perform algebraic manipulations to make it applicable.
- 🧮 The integral of e^U (where U is a function of x) is e^U, provided that the derivative of U is a factor of the integrand.
- 🔬 Verify the integration of e^U by differentiating e^U plus a constant and checking if it yields the original integrand, e^U times the derivative of U.
- ✅ When integrating 1/U, it becomes the natural log of U, but only if the derivative of U is a factor of the integrand.
- 📉 If the derivative of U is not a factor, you cannot directly apply the generalized rule for 1/U, and you must find an alternative method to integrate.
- 🔠 For any function integrated in the form of U^n, where U is not necessarily x, the derivative of U must be a factor of the integrand to apply the generalized power rule.
- 🔄 Always check your work by differentiating the result of the integration to confirm that it matches the original integrand.
- 📝 Remember that the process of integrating composite functions requires the application of the chain rule in reverse, ensuring that the derivative of the inner function multiplies the integrand.
Q & A
What is the general power rule for integration?
-The general power rule for integration states that the integral of x to the nth power is (x to the (n+1) power) / (n+1) plus a constant of integration, provided that the derivative of the inner function is a factor of the integrand.
How do you integrate a function that is a composition of two functions?
-To integrate a composition of two functions, you apply the chain rule in reverse. Ensure that the derivative of the inner function is a factor in the integrand, then apply the power rule to the outer function.
What is the antiderivative of x to the fourth power?
-The antiderivative of x to the fourth power is one-fifth x to the fifth power plus a constant of integration.
How can you verify your work after integrating a function?
-To verify your work, take the derivative of the antiderivative and check if it matches the original integrand.
What is the integral of e to the x power?
-The integral of e to the x power is e to the x plus a constant of integration, provided that the derivative of the inner function (in this case, x) is a factor of the integrand.
What is the integral of 1 over x?
-The integral of 1 over x is the natural log of x plus a constant of integration.
What happens if the derivative of the inner function is not a factor of the integrand when trying to use the generalized power rule?
-If the derivative of the inner function is not a factor of the integrand, you cannot apply the generalized power rule. You will need to use other integration techniques or manipulate the integral to make the derivative a factor.
How do you integrate a function like 3x squared plus one to the fourth power?
-First, ensure that the derivative of the inner function (3x squared + 1) is a factor of the integrand. Then, treat the function as a composition and apply the power rule to the outer function, which in this case is (3x squared + 1) to the fourth power.
What is the integral of 2x times x squared minus 1 to the one-half power?
-First, rewrite the integral to make the power a whole number by multiplying by one in the form of the reciprocal of the given power. Then, apply the power rule to the expression x squared minus 1 to the three halves power and multiply by the constant factor, which is 2x in this case.
Why is it important to check your work when integrating?
-Checking your work by differentiating the antiderivative ensures that you have correctly applied the integration rules and that your final answer is indeed the antiderivative of the original integrand.
What is the integral of a constant times a function?
-The integral of a constant times a function is the constant multiplied by the integral of the function. This is because integration and differentiation are linear operations.
Outlines
📚 Introduction to Generalizing Integration Rules
The video begins with an introduction to generalizing integration rules, focusing on extending the power rule, the rule for integrating e^x, and the rule for integrating 1/x. The aim is to adapt these rules for different forms of x, such as when x is replaced with another variable or expression. The process starts with integrating x to the fourth power as a basic example and then moves on to more complex compositions of functions.
🔄 Applying the Power Rule to Compositions of Functions
The lesson then delves into how to integrate more complex expressions like (3x^2 + 1)^4 by applying the power rule to compositions of functions. It's emphasized that the derivative of the inner function (in this case, 3x^2 + 1) must be a factor of the integrand for the generalized power rule to be applicable. The video demonstrates the correct integration process and the importance of checking the antiderivative by differentiating it back to the original integrand.
📉 Generalized Integration Rules for e^U and 1/U
The video continues by generalizing the integration rules for e^U and 1/U, explaining that the derivative of U must be a factor of the integrand to apply these rules. Examples are provided to illustrate how to integrate expressions like 7x - 1 to the third power and x^2 - 1 to the one-half power using the generalized power rule, with verification through differentiation to ensure correctness.
⛓ Chain Rule and Integration by Parts
The script touches on the concept of the chain rule in integration, showing how to handle expressions that are not direct applications of the power rule. An example is given where the derivative of the inner function is not explicitly present, and the expression is manipulated to make it suitable for integration using the generalized power rule. The process involves expanding the product and ensuring the derivative of the inner function is a factor before applying the rule.
🔢 Integrating Sums and Differences Using Power Rule
The video moves on to integrating sums and differences of terms, such as -72 + 192x^2 - 128x^4. The presenter demonstrates how to integrate each term separately using the power rule and then combines the results to find the antiderivative of the entire expression. The importance of verifying the antiderivative by differentiation is reiterated.
✅ Verifying Integration with e^U and 1/U
The final part of the video deals with verifying the integration of e^U and 1/U using the generalized rules. It includes examples of integrating e^(5x) and e^(x^2), emphasizing that the derivative of U must be a factor of the integrand. The video also covers integrating 1/U, showing that it simplifies to the natural log of U, provided the derivative of U is a factor. The presenter advises viewers to verify their work by differentiating the antiderivative to match the original integrand.
Mindmap
Keywords
💡Integration
💡Power Rule
💡Chain Rule
💡Constant of Integration
💡Derivative
💡Inner Function
💡Outer Function
💡Generalized Power Rule
💡Antiderivative
💡Natural Logarithm
💡Exponential Function
Highlights
Generalizing the power rule for integration is covered, focusing on integrating x to the power and e to the x, as well as the rule for integrating 1 over x.
The power rule for integration is explained by reversing the differentiation power rule, multiplying by the exponent and subtracting one from the exponent.
The importance of including the constant of integration when finding antiderivatives is emphasized.
The process of differentiating the antiderivative to verify the work is demonstrated, ensuring the derivative matches the original integrand.
The concept of a composition of functions is introduced, where the power rule is applied to more complex expressions like 3x squared plus one to the fourth power.
The chain rule is discussed in the context of integration, highlighting the need for the derivative of the inner function to be a factor in the integrand.
The generalized power rule for integration is presented, applicable when the derivative of the inner function (dU) is a factor of the integrand.
Examples of incorrectly applying the power rule are shown, illustrating the necessity for dU to be present in the integrand.
The integral of e to the U is discussed, noting that it equals e to the U, provided that the derivative of U is a factor of the integrand.
The natural log function is explored in the context of integrating 1 over X, with the integral equating to the natural log of U when dU is a factor.
The process of checking one's work by differentiating the antiderivative is emphasized as a good practice for ensuring the correctness of the integration.
A detailed walkthrough of integrating more complex functions like 2x times x squared minus 1 to the power of one-half is provided.
The sum and difference rule for integration is demonstrated by integrating each part of a complex function separately and then combining the results.
The concept of expanding products and manipulating expressions to fit the generalized integration rules is discussed, allowing for the integration of more complex functions.
A reminder is given that the generalized power rule cannot be applied if the derivative of the inner function is not a factor of the integrand.
The importance of verifying the antiderivative by differentiation is reiterated to ensure the integration process was performed correctly.
The transcript concludes with a summary of the generalized integration rules for power functions, e to the U, and 1 over U, highlighting the conditions for their application.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: