Business Calculus - Math 1329 - Section 5.2 - Integration by Substitution

Doug Ray

13 Apr 202029:00

EducationalLearning

32 Likes 10 Comments

TLDRThe transcript outlines a comprehensive guide on integration by substitution, a technique used to simplify complex integrals. The process involves selecting an appropriate function to substitute, denoted as 'u', and finding its derivative to replace the original integrand and differential 'dx'. The guide walks through six examples, illustrating how to apply this method to various functions. It demonstrates how to back-substitute to return to the original variable and solve for constants of integration. The examples range from polynomial integration to exponential functions and logarithms, showcasing the versatility of the technique. The transcript also touches on practical applications, such as modeling sales predictions and price-demand functions, offering a blend of theoretical and real-world insights into the power of integration by substitution.

Takeaways

📚 **Integration by Substitution**: This technique is used to integrate complex functions that were derived using the chain rule, essentially the reverse process.
🔍 **Choosing 'u'**: The 'inside' function that can be isolated and whose derivative is present in the integrand is chosen as 'u' for substitution.
🧮 **Derivative and Differential**: After choosing 'u', find its derivative 'Du' with respect to 'x', and solve for 'dx' in terms of 'du'.
➡️ **Substitution in Integral**: Replace 'u' and 'dx' in the integral with the chosen expressions to simplify the integral for easier evaluation.
🔄 **Back Substitution**: After integrating with respect to 'u', convert back to the original variable 'x' to find the final integral.
📈 **Example 1**: Integrate a function involving 'x' cubed plus 'x' squared by choosing the inside function as 'u' and following the substitution steps.
📉 **Example 2**: For a function involving an exponential term, choose the exponent as 'u' and integrate to find the anti-derivative.
🔢 **Example 3**: When dealing with rational functions, sometimes long division before integration can simplify the process.
🛒 **Example 6**: To find total sales over time, integrate the rate of change of sales with respect to time, using substitution to solve.
🎸 **Example 7**: For price-demand functions, integrate the rate of change of price with respect to quantity demanded to find the price function.
💡 **Initial Conditions**: Use given points (like demand and price at a certain quantity) to find the constant of integration 'C'.
✅ **Solving for Demand**: Once the price function is established, solve for the quantity demanded at a given price by setting up an equation and solving for 'x'.

Q & A

What is the main topic of the video script?
-The main topic of the video script is integration by substitution, a method used in calculus to integrate complex functions.
What is the significance of choosing the right u-substitution in integration by substitution?
-Choosing the right u-substitution is crucial because it should be the inside function whose derivative appears in the integrand, allowing for the cancellation of terms and simplification of the integral.
How does the process of integration by substitution help in evaluating integrals?
-Integration by substitution helps in evaluating integrals by transforming complex integrands into simpler forms that are easier to integrate, often by canceling out complicated terms within the integrand.
What is the first example given in the script to demonstrate integration by substitution?
-The first example given is the integral of five times x cubed plus x squared quantity raised to the fourth times 3x squared plus 2x.
What is the purpose of solving for DX in the integration by substitution process?
-Solving for DX is necessary to replace the differential DX in the integral with an expression involving DU, which simplifies the integral and makes it easier to evaluate.
How does back-substitution play a role in the integration by substitution process?
-Back-substitution is used to convert the simplified integral, which is now in terms of U, back into the original variable (X) to obtain the final integral solution.
What is the integral of 5u to the fourth in the context of the first example?
-The integral of 5u to the fourth is 5u to the fifth divided by 5, which simplifies to u to the fifth plus C. After back-substitution, it becomes x cubed plus x squared raised to the fifth plus C.
What is the method suggested in the script for dealing with integrals where the degree of the numerator is greater or equal to the degree of the denominator?
-The script suggests using long division (or synthetic division for simpler cases) to break down the integral into smaller parts that can be more easily handled, particularly when the degree of the numerator is greater than or equal to the degree of the denominator.
How does the script handle the integral of a function that involves a changing rate, such as the sales prediction example?
-The script integrates the rate function (s prime of T) over the given interval to find the total sales function (s of T). It also uses the initial condition (no initial sales) to find the constant of integration and then evaluates the total sales function at specific time points.
What is the integral of the price demand function DP/DX in the context of the guitar pricing example?
-The integral of the price demand function DP/DX is the price function P of X. The script uses a u-substitution method to integrate the given derivative function and then applies an initial condition to find the specific form of the price function.

Outlines

00:00

📚 Introduction to Integration by Substitution

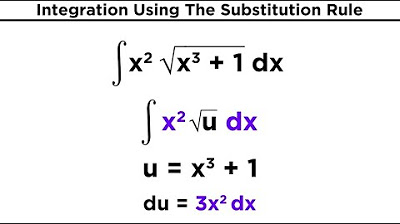
This paragraph introduces the concept of integration by substitution, which is a technique used to integrate complex functions that are derivatives found using the chain rule. It emphasizes the importance of choosing an appropriate inside function (u) and finding its derivative (du/dx) to simplify the integral. The process involves replacing dx with du/(3x^2 + 2x) and simplifying the integral to make it easier to evaluate. An example is provided to illustrate the steps of the process, including the selection of u, taking the derivative, solving for dx, and performing the substitution to obtain a simpler integral.

05:02

🧮 Examples of Integration by Substitution

This paragraph presents four examples of using integration by substitution to solve integrals. The first example involves integrating a function of x to the fifth power times e to the power of x squared minus nine. The second example is the integral of 1 over (2x - 3), which requires a substitution to simplify. The third example deals with the integral of x times the square root of (x squared + 5), where the substitution is made for x squared + 5. The fourth example involves a polynomial function, where long division is suggested before applying substitution. Each example demonstrates the steps of substitution, including choosing a substitution variable, finding its derivative, and simplifying the integral.

10:03

📈 Application in Sales Prediction and Guitar Pricing

This paragraph discusses two real-world applications of integration. The first application is predicting total sales of a company based on a given function of time, where the integral of the derivative function is calculated to find the total sales after a certain period. The second application is related to the pricing of guitars, where the rate of price change is given, and the goal is to find the price-demand function and determine the price at which a certain number of guitars will be demanded. The paragraph outlines the process of solving for the integral, applying initial conditions to find constants, and evaluating the function at specific points to answer the given questions.

15:06

🎓 Final Thoughts and Conclusion

The final paragraph wraps up the discussion on integration by substitution and its applications. It summarizes the process of solving integrals using substitution and highlights the importance of understanding the technique for solving complex integrals. The paragraph concludes with a thank you note to the viewers for watching the video and ends with a piece of background music, signaling the end of the content.

Mindmap

Keywords

💡Integration by Substitution

Integration by substitution is a technique used to evaluate integrals that involve derivatives found using the chain rule. It is considered the reverse process of the chain rule. In the video, this concept is central to solving complex integrals by transforming them into simpler forms that can be more easily integrated. For example, the script describes the process of choosing an inside function (u), finding its derivative (du), and then substituting these into the integral to simplify it.

💡Derivative

A derivative in calculus represents the rate of change of a function with respect to its variable. In the context of the video, derivatives are used to identify potential substitution variables (u) that can simplify the integral. For instance, when the script mentions finding 'du', it refers to the derivative of the chosen function u with respect to x.

💡Chain Rule

The chain rule is a fundamental theorem in calculus for deriving the derivative of a composite function. It is mentioned in the video as a concept that is used before applying integration by substitution. The script suggests that integration by substitution can be thought of as the opposite of the chain rule, which helps in integrating expressions derived using the chain rule.

💡Differential

In the context of the video, a differential represents an infinitesimally small change in a variable. 'du' and 'dx' are used to express the differentials of u and x, respectively. The video explains how to find 'du' with respect to 'dx' and then substitute these differentials in the integral to transform it into a more manageable form.

💡Back Substitution

Back substitution is the process of replacing the substitution variable (u) back with the original variable (x) after integrating. This step is essential to return to the original variable of integration and provide the integral's solution in the context of the original problem. The script illustrates back substitution in several examples, showing how the simplified integral solution is converted back to involve x.

💡Exponential Function

An exponential function is a mathematical function where the variable appears in the exponent. In the video, exponential functions are integrated using substitution. For example, the script discusses integrating 'e to the u' and then back substituting to find the integral involving the original variable.

💡Natural Logarithm

The natural logarithm is the logarithm of a number to the base e (approximately 2.71828). It is used in integration, particularly when the integral involves the inverse of an exponential function. The video demonstrates the integration of '1/u', which results in the natural logarithm of the absolute value of u, and then back substitution is applied.

💡Long Division

Long division is a method of dividing one polynomial by another. In the video, it is used as a technique to simplify the integral of a rational function before integrating. The script shows how long division can break down the integrand into simpler parts that are easier to integrate.

💡Synthetic Division

Synthetic division is a faster method of polynomial division, especially when dividing by a linear factor. The video briefly mentions it as a potential method for long division in the context of integrating rational functions. It is used to find the quotient, which represents the simplified form of the integrand.

💡Initial Conditions

Initial conditions are used to determine the constant of integration, which is an undetermined value added to an integral's result. In the video, an initial condition is given as 'no initial sales', which helps find the constant of integration for the sales function, setting s(0) to zero.

💡Demand Function

A demand function relates the quantity demanded of a product to its price. In the video, the demand function is used to find the price at which a certain quantity of guitars will be demanded. The script involves integrating the rate of change of price with respect to quantity to find the price-demand function.

Highlights

Integration by substitution is introduced as the reverse process of the chain rule.

The process involves selecting an inside function (u) and finding its derivative (du/dx).

Substitution simplifies the integral by replacing dx with du/(3x^2 + 2x) and the integrand with u.

The integral of 5u^4 is solved, resulting in 5u^5/5 + C, which simplifies to u^5 + C.

Back substitution is used to convert the solution back into the original function in terms of x.

In the second example, u-substitution is applied to integrate x^5 * e^(x^6 - 9).

The derivative of the exponent x^6 - 9 is used for u, with du/dx = 6x^5, leading to dx = du/6x^5.

The integral of e^u is found to be e^u + C, and back substitution yields the final solution.

For the third example, u-substitution is used on 1/(2x - 3), with u = 2x - 3, simplifying the integral.

The integral of 1/u is found to be ln|u| + C, and back substitution is performed to get the final answer.

In the fourth example, the integral of x * sqrt(x^2 + 5) is solved using substitution with u = x^2 + 5.

The integral is simplified to (1/2) * integral of u^(1/2) du, which is (2/3)u^(3/2) + C after integration.

Back substitution and simplification result in the final answer of (1/3)(x^2 + 5)^(3/2) + C.

In the fifth example, long division is used before integration to simplify the integrand x^2 + 5x - 7 / (x - 3).

The integral of 17/(x - 3) is approached with u-substitution, where u = x - 3, simplifying the process.

The final solution is a combination of the integral results, including ln|x - 3| + C.

A company's sales prediction function is integrated from 0 to 14 months to find total sales, assuming no initial sales.

The price-demand function for guitars is derived from the rate of price change, and used to find the price for 600 guitars demanded and the demand at $120 per guitar.

Transcripts

Browse More Related Video

Integration Using The Substitution Rule

4.5 - Integration Techniques - Substitution

❖ Integration using U-Substitution ❖

BusCalc 13.2 Integration by Substitution

Indefinite Integration (part IV)

Integration By Parts