AP Physics 1: Rotational Kinematics Review
TLDRIn this engaging lesson, the concepts of rotational kinematics are explored through a discussion on angular velocity, acceleration, and their relationships with linear motion. The video delves into the equations of uniformly angularly accelerated motion, highlighting the difference between angular and tangential velocities, and introduces the concept of centripetal acceleration. The lesson emphasizes the importance of understanding the relationship between period and frequency, and how these concepts apply to real-world scenarios like the conical pendulum. The video serves as a comprehensive review for students preparing for the AP Physics 1 exam.
Takeaways
- 📌 Angular velocity (ω) is represented by a curly W and is calculated as the change in angular position (Δθ) over the change in time (Δt).
- 📌 Angular displacement refers to the angular position final minus the angular position initial.
- 📌 Units for angular velocity can be expressed in radians per second or revolutions per minute, with one revolution equating to 360 degrees or 2π radians for conversion purposes.
- 📌 Angular acceleration (α), symbolized as a 'fishy thing', is the rate of change of angular velocity with respect to time.
- 📌 The dimensions for angular acceleration are radians per second squared.
- 📌 In rotational kinematics, the equations for velocity and acceleration are analogous to their linear counterparts, with angular displacement and velocity replacing linear displacement and velocity.
- 📌 Uniformly Angularly Accelerated Motion (U fishy M) equations apply when angular acceleration is constant, similar to Uniformly Accelerated Motion (UAM) equations.
- 📌 Objects undergoing uniform circular motion have a constant angular velocity, but their tangential velocity (linear velocity in the rotational context) can vary with radius size.
- 📌 Centripetal acceleration is directed towards the center of the circle and is caused by the centripetal force, which is the net force acting in the in-direction.
- 📌 The equation for centripetal acceleration is tangential velocity squared divided by the radius, or equivalently, radius times angular velocity squared.
- 📌 Period (T) and frequency are inversely related, with the period being the time for one full cycle or revolution, and frequency being the number of cycles per second (measured in Hertz).
Q & A
What is the symbol for angular velocity and what does it represent?
-The symbol for angular velocity is omega (ω), which represents the rate of change of angular position with respect to time.
How is angular displacement defined in the context of rotational motion?
-Angular displacement is defined as the change in angular position, calculated as the final angular position minus the initial angular position.
What are the dimensions of angular velocity and a common unit used to express it?
-The dimensions for angular velocity are radians per second, and it is often expressed in revolutions per minute.
What is the relationship between the number of degrees in one revolution and radians?
-In one revolution, there are 360 degrees, which is equivalent to 2π radians.
How is angular acceleration defined and what are its dimensions?
-Angular acceleration is defined as the rate of change of angular velocity with respect to time, and its dimensions are radians per second squared.
What is the significance of the 'U fishy M' equations in rotational kinematics?
-The 'U fishy M' equations are used when the angular acceleration is constant, and they are analogous to the Uniformly Accelerated Motion (UAM) equations, but with angular displacement and angular velocity substituted for linear displacement and linear velocity.
What is the difference between angular velocity and tangential velocity?
-Angular velocity is the rate at which an object rotates around an axis, while tangential velocity is the linear velocity of a rotating object along the tangent to the circle at the point of interest.
Why do objects with the same angular velocity but different radii have different tangential velocities?
-Objects with the same angular velocity but different radii have different tangential velocities because tangential velocity depends on both the angular velocity and the radius; a larger radius results in a longer arc length and thus a higher linear speed.
What is centripetal acceleration and how is it related to tangential velocity and radius?
-Centripetal acceleration is the acceleration directed towards the center of the circle in a rotating system. It is related to tangential velocity and radius by the equation: centripetal acceleration = (tangential velocity squared) / radius, and also by the equation: centripetal acceleration = radius × (angular velocity squared).
What does the term 'centripetal' mean and why is it significant in circular motion?
-The term 'centripetal' is Latin for 'center-seeking'. It is significant in circular motion because it describes the net force acting inwards, towards the center of the circle, which causes objects to follow a curved path.
How are period and frequency related and what are their respective dimensions?
-Period and frequency are inversely related. The period is the time taken for one complete cycle or revolution and is measured in seconds or seconds per cycle, while frequency is the number of cycles per second or revolutions per second, with the unit 'Hertz'.
How can the period of a rotating object be calculated using angular velocity?
-The period of a rotating object can be calculated using the equation: period = 2π / angular velocity. This equation is derived from the definition of angular velocity, which is the change in angular position (2π radians for one full circle) over the change in time (the period).
Outlines
📚 Introduction to Rotational Kinematics
This paragraph introduces the topic of Rotational Kinematics, which is part of the AP Physics 1 exam curriculum. The discussion begins with an explanation of angular velocity, denoted by the symbol 'omega', and its relation to the change in angular position over time. The concept of angular displacement is also introduced as the difference between the final and initial angular positions. The dimensions of angular velocity are explained as radians per second, with a common alternative being revolutions per minute. The conversion between degrees and radians is highlighted, emphasizing the importance of understanding 360 degrees is equivalent to 2π radians. The paragraph then moves on to angular acceleration, its symbol 'alpha', and its relationship with change in angular velocity over time, with dimensions in radians per second squared. The connection between linear and rotational equations for velocity and acceleration is discussed, leading to the Uniformly Angularly Accelerated Motion (U fishy M) equations. The relationship between linear and angular motion is further explored through a practical demonstration involving a stopper on a string, illustrating the concepts of uniform circular motion and the difference between angular and tangential velocities. The paragraph concludes with a discussion on the angular velocity of the stopper versus a piece of tape on the string, clarifying that both have the same angular velocity but different tangential velocities due to varying radii.
🔄 Understanding Tangential and Centripetal Concepts
This paragraph delves deeper into the concepts of tangential and centripetal motion. It begins by distinguishing between tangential velocity, which is the linear velocity of an object moving in a circle, and its direction, which is constantly changing, leading to the conclusion that tangential velocity is not constant. The discussion then introduces centripetal acceleration, a net force acting towards the center of the circle, and its relationship with tangential velocity and radius. The equation for centripetal acceleration is provided, along with an explanation of its components. The paragraph continues with an exploration of the centripetal force, clarifying that it is not a new force but rather the net force acting inwards. The importance of correctly identifying and summing forces in the in-direction is emphasized, with a practical example of a conical pendulum used to illustrate the concepts. The problem-solving process for such a scenario is outlined, including the construction of a Free Body Diagram and the application of force components. The paragraph concludes with a brief mention of the relationship between period and frequency, setting the stage for further discussion in the next paragraph.
🕒 Period and Frequency Relations in Rotational Motion
The final paragraph of the script focuses on the relationship between period and frequency in the context of rotational motion. It starts by defining the period as the time taken for one complete cycle or revolution, with its dimensions being seconds or seconds per cycle. Frequency is introduced as the number of cycles or revolutions per second, measured in Hertz. The inverse relationship between period and frequency is highlighted. The paragraph then revisits the equation for angular velocity, explaining how the period can be derived from it and how it relates to the angular displacement and time. The conversion between degrees and radians is again noted, with the explanation that radians are dimensionless and thus are not needed as a placeholder in the equation for period. The video script concludes with a wrap-up of the Rotational Kinematics review and a teaser for the next video on rotational dynamics. The audience is also directed to the instructor's website for additional resources and review materials.
Mindmap
Keywords
💡Angular Velocity
💡Angular Acceleration
💡Uniformly Angularly Accelerated Motion
💡Centripetal Acceleration
💡Centripetal Force
💡Tangential Velocity
💡Period and Frequency
💡Free Body Diagram
💡Conical Pendulum
💡SOH-CAH-TOA
Highlights
Introduction to Rotational Kinematics as a key topic in AP Physics 1 exam.
Definition of angular velocity with the symbol omega and its equation relating to change in angular position over time.
Explanation of angular displacement and its relation to final and initial angular positions.
Units of angular velocity in radians per second and its conversion to revolutions per minute.
Description of angular acceleration, its symbol, and its relationship with change in angular velocity over time.
Comparison between linear and rotational equations for velocity and acceleration.
Introduction to Uniformly Angularly Accelerated Motion (U fishy M) equations.
Demonstration of constant angular velocity using a stopper on a string and its limitations due to gravity.
Clarification on the difference between angular velocity and tangential (linear) velocity.
Explanation of the relationship between radius, angular velocity, and tangential velocity.
Definition and characteristics of Uniform Circular Motion with respect to angular acceleration.
Discussion on tangential velocity's dimensions and its distinction from centripetal acceleration.
Explanation of centripetal force as the net force in the in-direction and its non-existence in Free Body Diagrams.
Meaning of 'centripetal' as 'center-seeking' and its role in centripetal acceleration.
Equation for centripetal acceleration relating tangential velocity and radius.
Procedure for solving problems involving conical pendulum and Free Body Diagrams.
Introduction to period and frequency, their inverse relationship, and units.
Derivation of the period equation from angular velocity and its relation to the frequency.
Clarification on the use of radians in the period equation and their dimensionless nature.
Conclusion of the review on Rotational Kinematics and transition to the next topic, rotational dynamics.
Transcripts
Browse More Related Video

AP Physics C: Rotational Kinematics Review (Mechanics)
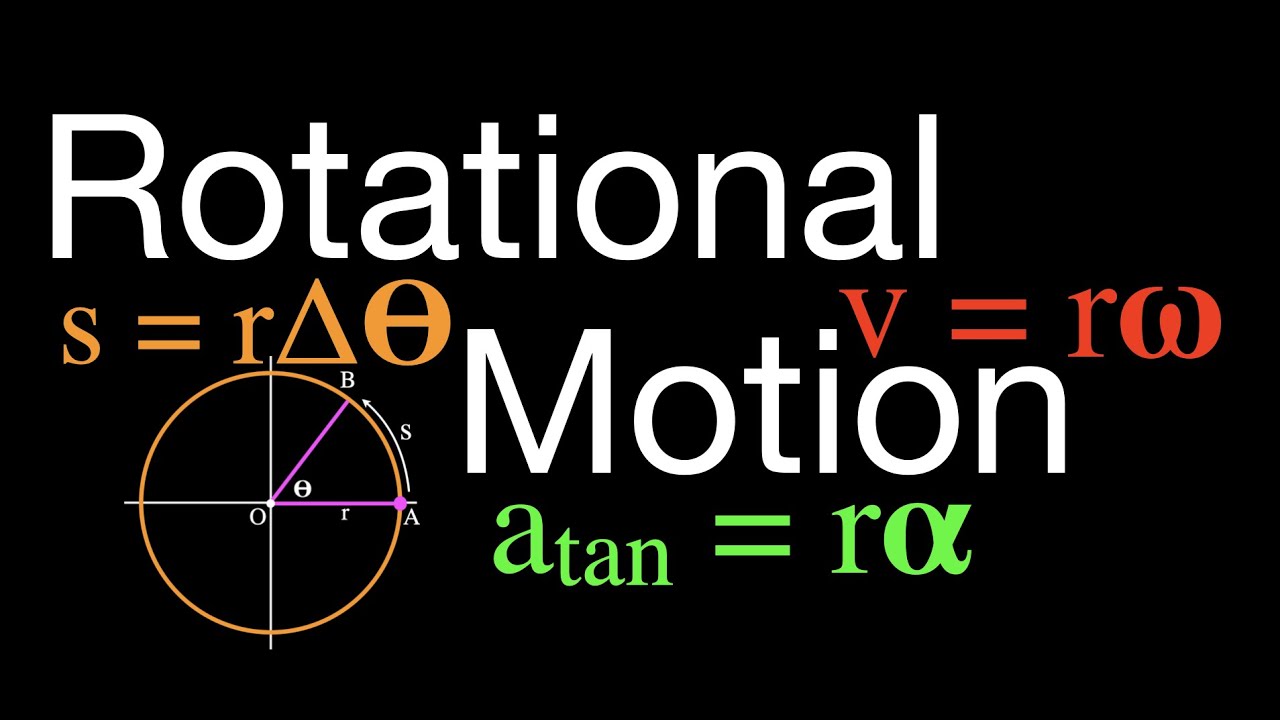
Rotational Motion: An Explanation, Angular Displacement, Velocity and Acceleration
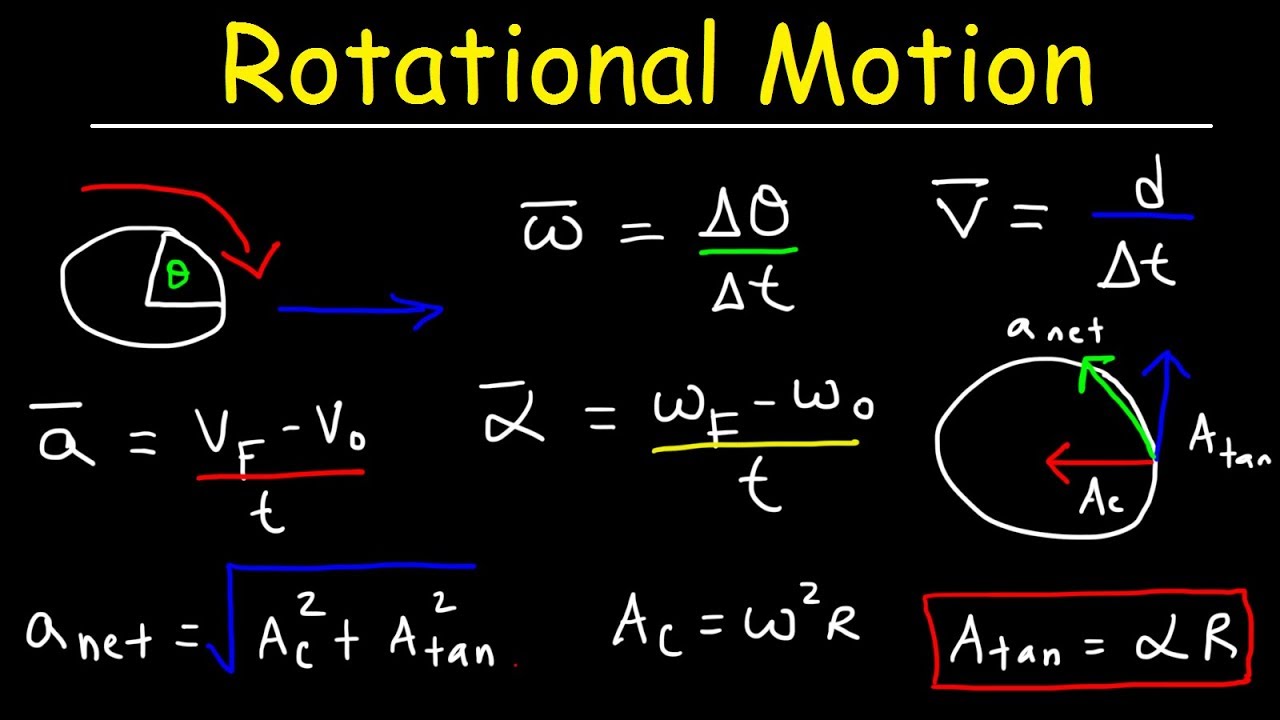
Rotational Motion Physics, Basic Introduction, Angular Velocity & Tangential Acceleration
Relating angular and regular motion variables | Physics | Khan Academy

AP Physics 1 - 10 Minute Recap

Rotational Kinematics Physics Problems, Basic Introduction, Equations & Formulas
5.0 / 5 (0 votes)
Thanks for rating: