Relating angular and regular motion variables | Physics | Khan Academy

Khan Academy Physics
29 Jul 201614:36
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the relationship between angular and linear motion variables, particularly useful for understanding rotational motion. It explains how angular displacement, measured in radians, can be converted to arc length, which is more practical for circular motion. The instructor also demonstrates the connection between angular velocity and linear speed, as well as the distinction between tangential acceleration, which changes speed, and centripetal acceleration, which changes direction. The script clarifies these concepts with clear mathematical relationships, making the complex topic of rotational dynamics more accessible.

Takeaways
  • πŸ“š Angular motion variables are often more useful for analyzing rotational motion, such as the motion of a tennis ball on a string.
  • πŸ”„ Angular displacement (Δθ) is measured in radians, which simplifies the calculation of arc length and other motion variables.
  • πŸ“ The arc length (s) is the distance an object travels along a circular path and is found by multiplying the radius by the angular displacement in radians.
  • πŸ”„ Angular velocity (Ο‰) is the rate of rotation and is related to linear speed by multiplying the radius by the angular velocity.
  • πŸš€ Angular acceleration (Ξ±) is the rate at which angular velocity changes and is related to the change in speed by considering the radius.
  • πŸ”— The relationship between angular variables and linear variables is crucial for understanding rotational motion dynamics.
  • πŸ“ The formula for arc length is s = r * Δθ, where Δθ is in radians, making it easy to calculate the distance traveled.
  • ⏱ Angular velocity is directly proportional to the speed of an object moving in a circle, with the formula v = r * Ο‰.
  • πŸ”„ Tangential acceleration is the component of acceleration that changes the speed of an object and is given by a = R * Ξ±.
  • πŸŒ€ Centripetal acceleration is the component that changes the direction of the velocity and is found using the formula a_c = v^2 / R.
  • 🧩 Total acceleration of an object in circular motion can be found using the Pythagorean theorem, combining tangential and centripetal components.
Q & A

  • Why are angular motion variables more useful for describing rotational motion compared to regular motion variables?

    -Angular motion variables are more useful for rotational motion because they provide a consistent description of motion for all points in a rotating system, such as every point on a string tied to a tennis ball moving in a circle having the same angular displacement, velocity, and acceleration.

  • What is the simplest angular motion variable and how is it measured?

    -The simplest angular motion variable is angular displacement, which represents the angle through which an object has rotated. It is typically measured in radians in physics.

  • Why is the arc length a more useful quantity than the regular displacement for rotational motion?

    -The arc length is more useful because it represents the actual path traced by the object in space during its rotation, which is easier to calculate and more relevant for many problems involving rotational motion.

  • How can the arc length of an object moving in a circular path be calculated if the angular displacement is given in radians?

    -The arc length can be calculated by multiplying the radius of the circular path by the angular displacement in radians. This is because one radian is defined as the angle for which the arc length is equal to the radius.

  • What is the relationship between angular velocity and regular velocity?

    -The relationship between angular velocity (omega) and regular velocity (speed) is given by the formula: speed = radius Γ— angular velocity. It shows how the rate of rotation (angular velocity) translates to the speed of the object along the circular path.

  • How does angular acceleration relate to regular acceleration?

    -Angular acceleration (alpha) is related to the change in speed of the object over time. It is given by the formula: tangential acceleration = radius Γ— angular acceleration, which represents the component of acceleration that changes the speed of the object.

  • What is the difference between centripetal acceleration and tangential acceleration?

    -Centripetal acceleration is the component of acceleration that changes the direction of the velocity and is always directed towards the center of the circular path. Tangential acceleration, on the other hand, changes the magnitude of the velocity (speed) and is directed parallel to the direction of motion.

  • How can the total acceleration of an object moving in a circle be found?

    -The total acceleration can be found by using the Pythagorean theorem on the two perpendicular components of acceleration: the tangential acceleration (R Γ— alpha) and the centripetal acceleration (vΒ²/R). The total acceleration squared is the sum of the squares of these two components.

  • Why is it not necessary to calculate the regular displacement of an object in circular motion for most problems?

    -Calculating the regular displacement of an object in circular motion is not necessary because it involves complex calculations like the law of cosines and does not provide useful information for most problems involving rotational motion.

  • What is the significance of using radians as the unit for angular displacement?

    -Radians are significant because they simplify the calculations involving angular displacement and arc length. The definition of a radian ensures that the arc length is directly proportional to the radius when the angular displacement is measured in radians.

  • How does the instructor illustrate the concept of angular motion variables being more convenient for rotational problems?

    -The instructor uses the example of a tennis ball tied to a string and whirled in a circle, demonstrating that angular motion variables like displacement, velocity, and acceleration remain consistent for every point on the string, making them more convenient for describing rotational motion.

Outlines
00:00
πŸ“š Introduction to Angular Motion Variables

The instructor begins by revisiting the concept of angular motion variables introduced in the previous video, emphasizing their usefulness in analyzing rotational motion, exemplified by a tennis ball on a string. Angular displacement, measured in radians, is identified as the simplest variable, representing the angle of rotation. The video aims to demonstrate the translation of angular variables into linear motion variables, starting with the conversion of angular displacement to arc length, which is more practical and straightforward than calculating linear displacement.

05:02
πŸ”— Relating Angular Displacement to Arc Length

The second paragraph delves into the relationship between angular displacement and arc length, highlighting the convenience of using radians for this conversion. The instructor explains that the arc length, denoted by 's', can be easily calculated as the product of the radius of the circular path and the angular displacement in radians. This method is advantageous because radians are defined such that the arc length equals the radius when the angle is one radian. The paragraph also introduces the concept of angular velocity, defined as the rate of change of angular displacement over time, and its conversion into linear speed by multiplying the radius with the angular velocity.

10:04
πŸš€ Connecting Angular Velocity and Speed to Acceleration

In the third paragraph, the instructor discusses the connection between angular acceleration and linear acceleration. Angular acceleration, represented by the Greek letter alpha, is the rate of change of angular velocity over time. The instructor illustrates how to derive the formula for tangential acceleration, which is the component of acceleration that changes the speed of an object moving in a circle, by multiplying the radius with angular acceleration. The paragraph also distinguishes between tangential acceleration, which affects speed, and centripetal acceleration, which is responsible for changing the direction of velocity. The total acceleration of an object in circular motion can be found using the Pythagorean theorem, considering both tangential and centripetal components.

Mindmap
Keywords
πŸ’‘Angular Motion Variables
Angular motion variables are a set of physical quantities used to describe the rotational motion of objects. In the context of the video, they are contrasted with regular motion variables for describing circular motion, such as in the case of a tennis ball on a string. The video argues that angular variables are often more useful for these types of problems because they provide a consistent measure of rotation across all points of the object, including the tennis ball.
πŸ’‘Angular Displacement
Angular displacement, denoted as delta theta in the script, is the measure of the angle through which an object has rotated. It is a fundamental angular motion variable and is typically measured in radians. The video explains that converting angular displacement to linear displacement can be complex, but it can be easily related to the arc length, which is a more useful quantity in many problems.
πŸ’‘Radians
Radians are the unit of measurement for angular displacement. The video emphasizes the convenience of using radians because it simplifies the calculation of arc length. One radian is defined as the angle for which the arc length of a circle is equal to the radius of that circle, making it easy to convert between angular and linear measurements.
πŸ’‘Arc Length
Arc length is the distance along the path that an object traces when it moves along a circular path. In the video, it is identified as a more useful quantity than linear displacement for circular motion. The script explains that arc length can be easily calculated from the radius and the angular displacement when measured in radians.
πŸ’‘Angular Velocity
Angular velocity is the rate at which an object rotates, measured in radians per second. It is represented by the Greek letter omega in the script. The video discusses how angular velocity can be related to the speed of an object moving in a circle, emphasizing that a higher angular velocity corresponds to a higher speed.
πŸ’‘Speed
Speed is the scalar quantity that represents how fast an object is moving, without regard to its direction. The video explains the relationship between angular velocity and speed, showing that the speed of an object in circular motion can be found by multiplying the radius of the circle by the angular velocity.
πŸ’‘Angular Acceleration
Angular acceleration is the rate of change of angular velocity over time, denoted by the Greek letter alpha in the script. The video discusses how angular acceleration can be related to the change in speed of an object in circular motion, distinguishing it from the centripetal acceleration which changes the direction of velocity.
πŸ’‘Tangential Acceleration
Tangential acceleration is the component of acceleration that changes the magnitude of the velocity, or speed, of an object moving in a circle. The video explains that it is calculated as the radius times the angular acceleration and is directed along the tangent to the circle at the object's position.
πŸ’‘Centripetal Acceleration
Centripetal acceleration is the acceleration directed towards the center of a circle that an object experiences when moving in circular motion. The video clarifies that this acceleration changes the direction of the object's velocity, not its speed, and is calculated using the formula speed squared divided by the radius.
πŸ’‘Total Acceleration
Total acceleration is the vector sum of all acceleration components acting on an object. In the context of the video, it is explained that the total acceleration can be found by using the Pythagorean theorem to combine the tangential and centripetal accelerations, which are perpendicular to each other.
πŸ’‘Law of Cosines
The law of cosines is a mathematical formula used to find a side of a triangle when the other two sides and the included angle are known. The video mentions it in the context of calculating linear displacement in circular motion, but notes that it is more complex and not the focus of the lesson.
Highlights

Angular motion variables are more useful for rotational motion problems than regular motion variables.

All points on a rotating string, including a tennis ball, share the same angular displacement, velocity, and acceleration.

Translating angular motion variables to regular motion variables is essential for solving rotational problems.

Angular displacement, represented by delta theta, is measured in radians for convenience in calculations.

Arc length is a more useful quantity than linear displacement for rotational motion, representing the path traced by an object.

The arc length can be easily calculated using the radius and angular displacement in radians.

Radians are defined such that the arc length equals the radius when the object has rotated through one radian.

Angular velocity is the rate of rotation and is related to the speed of an object in circular motion.

The speed of an object can be found by multiplying the radius by the angular velocity.

Angular acceleration is the rate of change of angular velocity and is related to changes in the object's speed.

Tangential acceleration is the component of acceleration that changes the speed of an object, calculated as radius times angular acceleration.

Centripetal acceleration is the component that changes the direction of the velocity, directed inward towards the center of the circle.

Total acceleration can be found using the Pythagorean theorem by combining tangential and centripetal acceleration components.

Understanding the relationship between angular and regular motion variables is crucial for analyzing rotational motion.

The video demonstrates practical applications of angular motion variables in real-world scenarios like a tennis ball on a string.

The importance of using radians in physics for simplifying the relationship between angular and linear quantities is emphasized.

The video provides a clear explanation of how to convert between angular and regular motion variables for better understanding of rotational dynamics.

Transcripts

Browse More Related Video

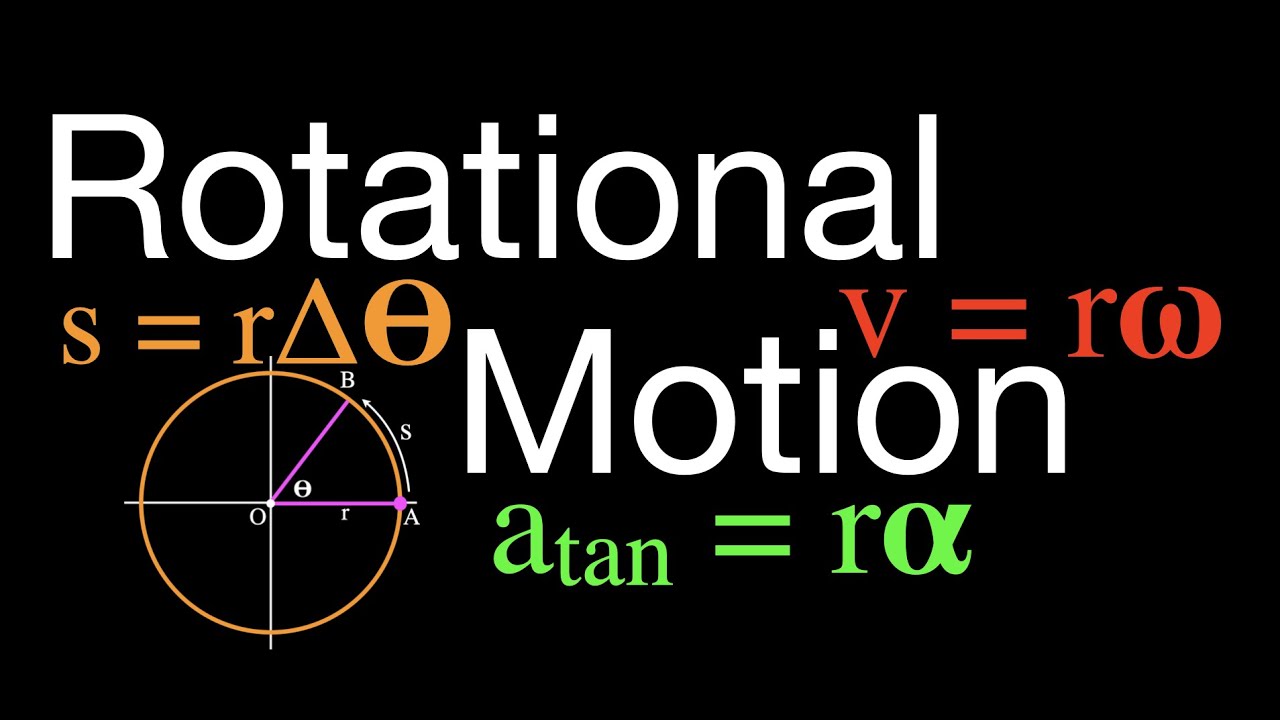
Rotational Motion: An Explanation, Angular Displacement, Velocity and Acceleration

Circular Motion: Acceleration (Angular, Tangential, Centripetal)

AP Physics C: Rotational Kinematics Review (Mechanics)

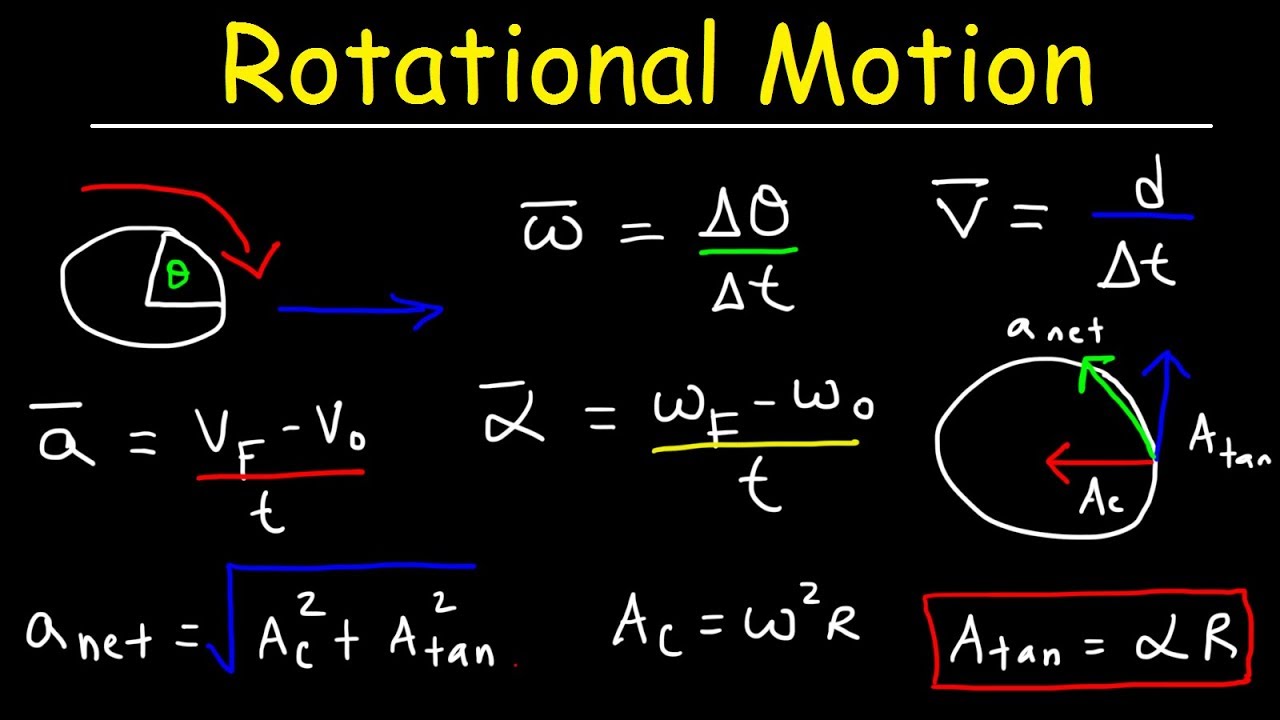
Rotational Motion Physics, Basic Introduction, Angular Velocity & Tangential Acceleration

angular velocity: what is it and how is it calculated

Uniform Circular Motion Class 11

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Angular MotionLinear MotionPhysics TutorialTennis Ball ExampleArc LengthAngular DisplacementAngular VelocitySpeed CalculationAcceleration TypesCentripetal ForceTangential Acceleration