AP Physics 1 - 10 Minute Recap
TLDRThis comprehensive review for AP Physics 1 covers essential topics for exam preparation. Kinematics is introduced with an emphasis on constant acceleration and the application of kinematic equations in projectile motion. The importance of free-body diagrams and net force in analyzing forces is highlighted. Circular motion is explained without introducing new forces, focusing on centripetal acceleration and force. Gravitation is discussed in the context of Newton's law and the concept of gravitational field or acceleration due to gravity. Work and energy are explored, with a clear distinction between scalar and vector quantities, and the conservation of energy is a key principle. Linear momentum is defined as a vector, and the role of impulse in changing momentum is covered. Rotational dynamics are discussed, including torque, rotational inertia, and the relationship between linear and angular motion variables. The concept of rolling without slipping is introduced, emphasizing the absence of kinetic friction. Finally, simple harmonic motion is characterized by its periodic and sinusoidal nature, with examples of pendulum and mass-spring systems. The review concludes with a resource link for more detailed notes, aiming to assist students in their last-minute exam preparation.
Takeaways
- 📐 **Kinematics**: Kinematic equations apply only when acceleration is constant. For projectile motion, consider zero horizontal acceleration and vertical acceleration due to gravity (g).
- 🎯 **Forces and Free Body Diagrams**: To solve force problems, draw a free body diagram, decompose vectors into components, and apply net force equals mass times acceleration (F=ma).
- 🔄 **Circular Motion**: No new forces are introduced in circular motion; centripetal acceleration is the acceleration towards the center of the circle due to the change in direction of the velocity vector.
- 🪐 **Gravitation**: Gravity is mg near Earth's surface, but for greater distances, use Newton's law of gravitation, and remember that gravitational acceleration is g times the mass of the body over r squared.
- 🔩 **Work and Energy**: Work is a scalar equal to the force parallel to the displacement times the displacement, and it causes a change in the energy of the system (Work = Change in Energy).
- ⚙️ **Energy Systems**: Consider kinetic, potential (gravitational and spring), and rotational energies when analyzing energy systems. Use conservation of energy if no work is done on the system.
- 🚀 **Linear Momentum**: Momentum is a vector (mv), and its direction is crucial. Impulse (FΔt) causes a change in momentum, and in collisions, conservation of momentum applies if there are no external forces.
- 🌀 **Rotational Dynamics**: Relate linear motion variables to angular motion by multiplying by the radius (r). Torque causes angular acceleration, and it requires a force to be perpendicular to the radius vector.
- 🤸 **Rotational Inertia**: It's a measure of how difficult it is to rotate an object, influenced by the mass distribution relative to the axis of rotation.
- 🛷 **Rolling Without Slipping**: Implies no kinetic friction between the object and the surface, allowing for smooth rolling without energy loss due to friction.
- 🔁 **Conservation of Angular Momentum**: Applies when there's no external torque, such as in the case of divers spinning and changing their rotational inertia to increase spin speed.
- 🏋️♂️ **Simple Harmonic Motion**: Characterized by a restoring force towards the equilibrium point, resulting in periodic and sinusoidal motion, with an exchange between potential and kinetic energy.
Q & A
What are the conditions required to apply the kinematic equations in physics?
-The kinematic equations can be applied when the acceleration is constant. If the acceleration is changing or varies as a function of time, the kinematic equations are not applicable.
How should one approach solving projectile motion problems?
-For projectile motion problems, one should recognize that horizontal acceleration is zero and vertical acceleration is downward with 'g' (acceleration due to gravity). Then, set up the kinematic variables, assign them, and use the kinematic equations to solve the problem.
What is the process for solving problems with forces?
-The process involves three steps: 1) Draw a free body diagram, 2) Decompose the vectors into horizontal and vertical components, and 3) Apply the net force equals mass times acceleration (F_net = m*a) in the vertical or horizontal direction.
When dealing with circular motion, what is a common misconception?
-A common misconception is that there are new forces acting in circular motion. However, there are no new forces; the free body diagram remains the same, with the only difference being the presence of centripetal acceleration directed towards the center of the circle.
What is centripetal force and how is it related to centripetal acceleration?
-Centripetal force is not a new type of force but rather the net force directed towards the center of a circle. It is responsible for the centripetal acceleration, which is given by the formula centripetal force equals mass times centripetal acceleration (F_c = m*a_c), where a_c is v^2/r.
How is gravitational force calculated near and far from the Earth's surface?
-Near the Earth's surface, gravitational force is calculated as mg, where m is the mass and g is the acceleration due to gravity. Far from the Earth's surface, where a more accurate calculation is needed (e.g., a few hundred miles away), Newton's law of gravitation is used, and the gravitational field or acceleration due to gravity is calculated as g times the mass of the planetary body over r squared (g = G*M/r^2).
What is the relationship between work and energy in a system?
-Work is a scalar quantity equal to the force parallel to the displacement times the displacement. Work causes a change in the energy of the system, and the amount of work done on a system is equal to the change in energy (Work = ΔE).
What types of energy are considered when analyzing a system that contains different components?
-When analyzing a system, one should consider kinetic energy (both translational and rotational), gravitational potential energy (if the system contains the Earth or a planetary body), and spring potential energy (if the system contains a spring).
How is momentum defined and what is the key factor to consider when dealing with it?
-Momentum is a vector quantity defined as the product of mass and velocity (p = m*v). The direction of the velocity is crucial because momentum, unlike work and energy, is a vector, and thus its direction is significant.
What is the fundamental principle behind the impulse-momentum theorem?
-The impulse-momentum theorem states that the impulse (the integral of force over time, F*Δt) is equal to the change in momentum (Δp). Impulse is the cause of a change in momentum.
What are the key differences between elastic and inelastic collisions?
-In elastic collisions, both momentum and kinetic energy are conserved. In inelastic collisions, momentum is conserved, but kinetic energy is not, due to energy losses often caused by deformation during the collision.
How does torque relate to angular acceleration?
-Torque causes angular acceleration, similar to how force causes linear acceleration in Newton's second law of motion. Torque is the product of a force applied perpendicular to the radius (r) from the axis of rotation.
What is the role of rotational inertia in the equation for net torque?
-Rotational inertia (I) is a measure of how difficult it is to rotate an object and it appears in the equation for net torque as net torque equals rotational inertia times angular acceleration (τ_net = I*α).
What is the concept of rolling without slipping?
-Rolling without slipping refers to a situation where there is no kinetic friction between the rolling object and the surface. This means the object rolls smoothly without any energy loss due to friction.
How is angular momentum conserved in a system?
-Angular momentum is conserved in a system when there is no external torque acting on it. This conservation principle is used in scenarios such as collisions or when the rotational inertia of a system changes without an external torque.
What characterizes simple harmonic motion and how is it described mathematically?
-Simple harmonic motion is characterized by a restoring force that pulls the object back towards an equilibrium point. Mathematically, it is described by a periodic and sinusoidal motion, often represented by sine or cosine functions.
What types of energy are involved in the motion of a pendulum and a mass on a spring?
-In the case of a pendulum, gravitational potential energy is converted to kinetic energy and back to gravitational potential energy. For a mass on a horizontal spring, it's a conversion between spring potential energy and kinetic energy. For a vertical mass on a spring, there's an interchange between gravitational potential energy, spring potential energy, and kinetic energy.
Outlines
📚 AP Physics 1 Kinematics and Forces Overview
This paragraph provides a comprehensive review of key concepts in AP Physics 1. It begins with kinematics, emphasizing the use of kinematic equations for constant acceleration and the importance of setting up variables correctly for projectile motion problems. The speaker then discusses forces, highlighting the process of drawing free body diagrams and applying Newton's second law (F = ma). Circular motion is covered next, clarifying that there are no new forces introduced but rather a new type of acceleration, centripetal acceleration, which is always directed towards the center of the circle. Gravitation is also explained, with the distinction between the formula for gravity near the Earth's surface (mg) and Newton's law of gravitation for larger distances. The paragraph concludes with a discussion on work and energy, including the calculation of work, the concept of energy systems, and the application of conservation of energy.
🚀 AP Physics 1 Momentum, Rotation, and Simple Harmonic Motion
The second paragraph delves into the topics of momentum, impulse, and collisions within AP Physics 1. It explains that impulse causes a change in momentum and is crucial in collision problems, where conservation of momentum can be applied if the system includes all objects and there are no external forces. The difference between elastic and inelastic collisions is also highlighted, with the conservation of kinetic energy being the key factor. The paragraph then transitions into rotation, discussing the rotational analogs of linear motion variables and the relationship between linear and angular motion. Torque is introduced as the cause of angular acceleration, and its requirement for a force to be perpendicular to the radius vector is emphasized. Rotational inertia is defined as the resistance to rotation, with factors affecting it being mass distribution and distance from the axis of rotation. The concept of rotational kinetic energy and its calculation using the formula (1/2)Iω² is also covered. Rolling without slipping is explained in terms of the absence of kinetic friction, leading to a smooth roll without energy loss. Finally, angular momentum and its conservation, especially in scenarios involving changing rotational inertia without external torque, are discussed. The paragraph concludes with an overview of simple harmonic motion, characterized by a restoring force towards an equilibrium point, resulting in periodic, sinusoidal motion, and the exchange of potential and kinetic energy in pendulum and mass-spring systems.
Mindmap
Keywords
💡Kinematics
💡Projectile Motion
💡Free Body Diagram
💡Centripetal Force
💡Gravitation
💡Work and Energy
💡Linear Momentum
💡Rotational Motion
💡Conservation Laws
💡Simple Harmonic Motion
💡Rolling Without Slipping
Highlights
Kinematic equations can only be applied when acceleration is constant.
In projectile motion, horizontal acceleration is zero, and vertical acceleration is downward with g.
Free body diagrams are crucial for solving force problems, followed by applying net force equals mass times acceleration (F=ma).
In circular motion, there are no new forces introduced; centripetal acceleration is the acceleration directed towards the center of the circle.
Centripetal force is not a new force but the net force towards the center of the circle, calculated as mass times centripetal acceleration.
Gravitation near the Earth's surface is calculated as mg, while further away, Newton's law of gravitation is used.
Work is a scalar quantity equal to the force parallel to the displacement, causing a change in energy of the system.
Energy systems include kinetic, gravitational potential, spring potential, and rotational kinetic energy.
Conservation of energy states that if no work is done on a system, there's no change in energy unless energy is conserved.
Momentum is a vector quantity, and its direction is crucial, equal to mass times velocity (p=mv).
Impulse causes a change in momentum, calculated as the force times the time (FΔt = Δp).
In collisions, conservation of momentum applies if the system includes both objects and there are no external forces.
Elastic and inelastic collisions are distinguished by whether kinetic energy is conserved or not.
Rotation involves converting linear motion variables to angular motion variables by multiplying by the radius (r).
Torque causes angular acceleration and requires a force to be perpendicular to the radius vector (τ = rF⊥).
Rotational inertia (I) is a measure of how difficult it is to rotate an object and depends on mass distribution relative to the axis of rotation.
Rolling without slipping implies no kinetic friction between the object and the surface, resulting in no energy loss from friction.
Conservation of angular momentum applies when there's no external torque, such as in the case of a diver tucking in to spin faster.
Simple harmonic motion is characterized by a restoring force towards the equilibrium point, resulting in periodic and sinusoidal motion.
In simple harmonic motion, there's an exchange of potential and kinetic energy, with different forms of energy involved depending on the system.
Transcripts
Browse More Related Video
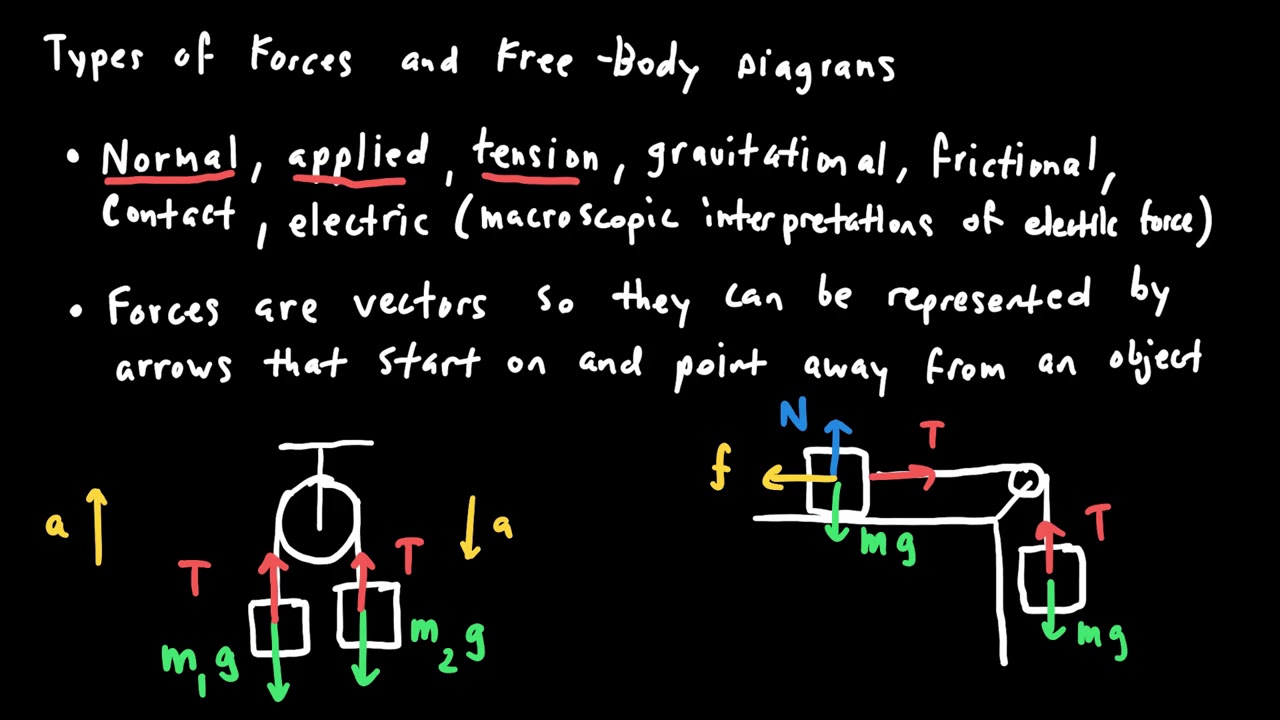
AP Physics 1 Dynamics Review
AP Physics 1: Rotational Dynamics Review

2022 Live Review 6 | AP Physics C: Mechanics | Rotational Dynamics and Angular Momentum
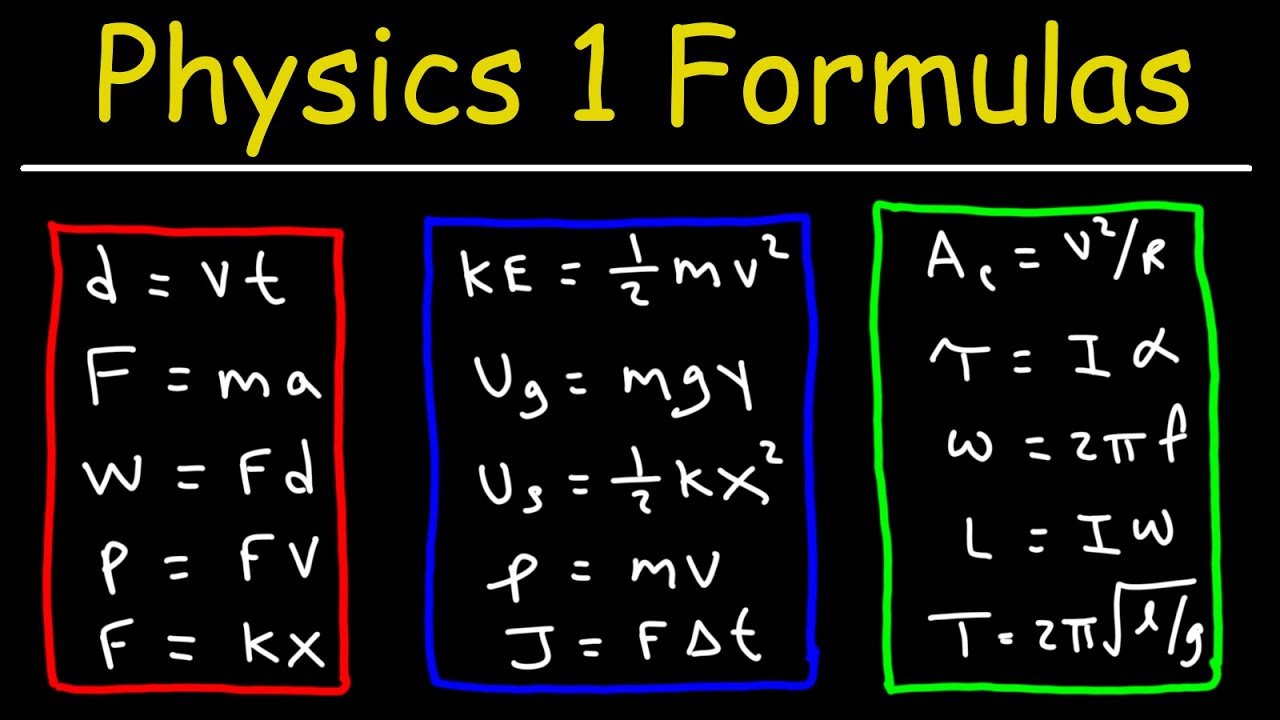
Physics 1 Formulas and Equations - Kinematics, Projectile Motion, Force, Work, Energy, Power, Moment
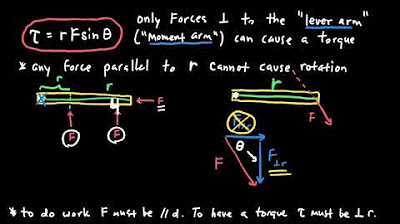
AP Physics 1 Torque and Rotational Motion Review

2022 Live Review 7 | AP Physics 1 | Understanding Torque and Rotational Motion
5.0 / 5 (0 votes)
Thanks for rating: