AP Physics C: Rotational Kinematics Review (Mechanics)

Flipping Physics
5 Apr 201711:33
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive review of rotational kinematics for the AP Physics C mechanics curriculum. It covers key concepts such as angular velocity (both average and instantaneous), angular acceleration, and their units. The script introduces uniformly angularly accelerated motion, often referred to as 'u fishy m', and its five variables and four equations. It also explains arc length, tangential velocity, and acceleration, emphasizing the use of radians in calculations. The video distinguishes between uniform circular motion, where angular acceleration is zero, and non-uniform circular motion, which involves both centripetal and tangential accelerations. The script concludes with a discussion on the period of an object and its relationship to angular velocity, providing a solid foundation for students preparing for the AP Physics C exam.

Takeaways
  • πŸ“š The script is a review of rotational kinematics for the AP Physics C mechanics curriculum.
  • πŸ”  The symbol for angular velocity is the lowercase Greek letter omega (Ο‰).
  • ⏱ Average angular velocity is the change in angular position over time, while instantaneous angular velocity is the derivative of angular position with respect to time.
  • πŸ“ Angular velocity units are typically radians per second in physics, but often revolutions per minute in practical applications.
  • πŸ“‰ The symbol for angular acceleration is alpha (Ξ±), sometimes humorously referred to as the 'fishy thing'.
  • ⏳ Average angular acceleration is the change in angular velocity over time, and instantaneous angular acceleration is its derivative with respect to time.
  • πŸ”„ Uniformly angularly accelerated motion equations are analogous to uniformly accelerated motion equations and apply when angular acceleration is constant.
  • πŸ“ The equation for arc length is s = rΔθ, where s is arc length, r is radius, and Δθ is angular displacement.
  • πŸŒ€ Radians are used for angular displacement in the arc length equation, with one revolution equaling 360 degrees or 2Ο€ radians.
  • πŸš€ The derivative of the arc length equation with respect to time gives the tangential velocity, which is the linear velocity when moving along a circle.
  • πŸ›‘ Centripetal acceleration is the acceleration directed towards the center of the circle in circular motion, calculated as tangential velocity squared over radius or radius times angular velocity squared.
  • πŸ”„ Non-uniform circular motion involves both centripetal and tangential accelerations, resulting in a total acceleration directed between the circle's center and its circumference.
Q & A

  • What is the symbol for angular velocity and its units in physics?

    -The symbol for angular velocity is the lower case Greek letter omega (Ο‰), and its units in physics are usually radians per second.

  • How is average angular velocity calculated?

    -Average angular velocity is calculated as the change in angular position divided by the change in time.

  • What is the symbol for angular acceleration and its units?

    -The symbol for angular acceleration is alpha (Ξ±), and its units are radians per second squared.

  • What are the uniformly angularly accelerated motion (u fishy m) equations used for?

    -The u fishy m equations are used for solving problems where the angular acceleration is constant.

  • What is the equation for arc length and what does each variable represent?

    -The equation for arc length is s = r * Δθ, where s is the arc length, r is the radius, and Δθ is the angular displacement.

  • What happens when you take the derivative of the arc length equation with respect to time?

    -Taking the derivative of the arc length equation (s = r * Δθ) with respect to time gives tangential velocity (v_t = r * Ο‰), where Ο‰ is angular velocity.

  • How do you derive tangential acceleration from tangential velocity?

    -By taking the derivative of tangential velocity (v_t = r * Ο‰) with respect to time, we get tangential acceleration (a_t = r * Ξ±), where Ξ± is angular acceleration.

  • What is centripetal acceleration and its equation?

    -Centripetal acceleration is the acceleration directed towards the center of a circular path. Its equation is a_c = v_t^2 / r, where v_t is tangential velocity and r is the radius.

  • What is the relationship between centripetal force and Newton's Second Law?

    -Centripetal force is the net force acting inward on an object moving in a circular path and is calculated as F_c = m * a_c, where m is mass and a_c is centripetal acceleration.

  • What conditions are necessary for non-uniform circular motion?

    -Non-uniform circular motion occurs when the angular acceleration is non-zero, resulting in both centripetal and tangential accelerations.

  • How do you calculate the angular velocity of an object in uniform circular motion?

    -Angular velocity (Ο‰) is calculated as Ο‰ = 2Ο€ / T, where T is the period of one full revolution.

Outlines
00:00
πŸ“š Introduction to Rotational Kinematics

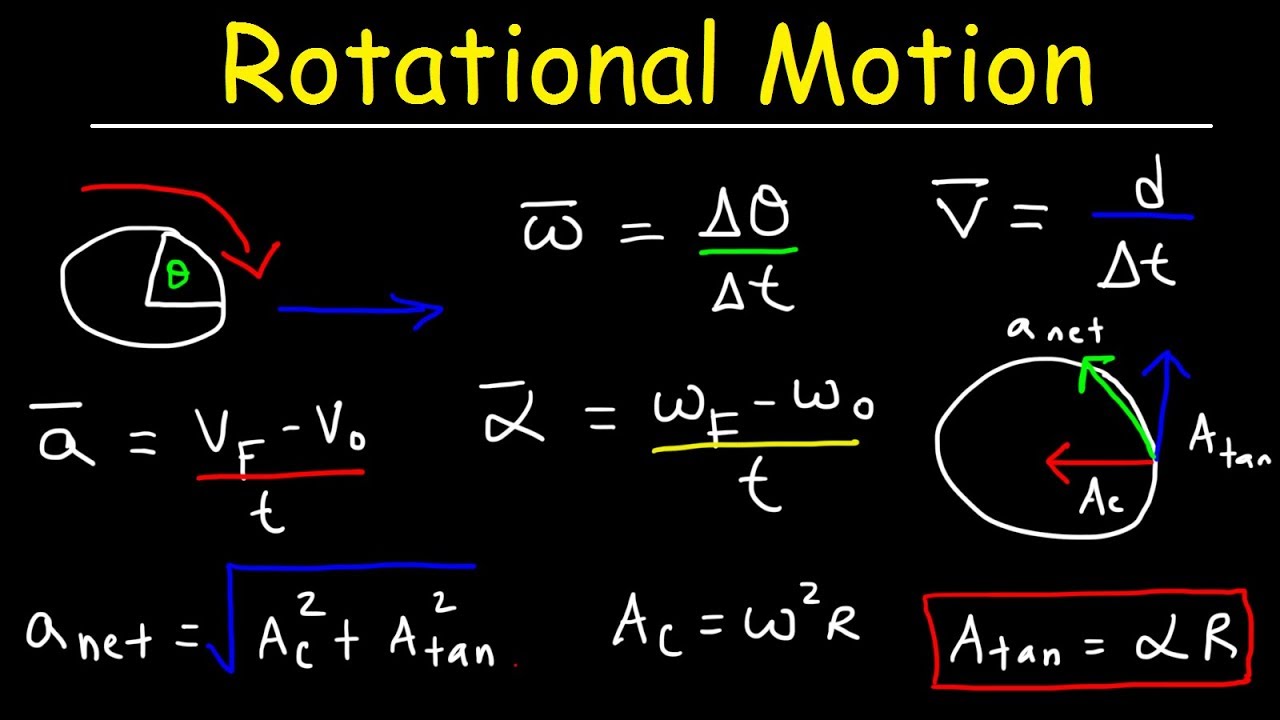
The video begins with an introduction to rotational kinematics as part of the AP Physics C mechanics curriculum. The instructor, Mr. P, engages with students to review key concepts such as angular velocity (represented by the Greek letter omega), which is measured in radians per second or revolutions per minute. The difference between average and instantaneous angular velocity is explained, with the former being the change in angular position over time and the latter being the derivative of angular position with respect to time. Angular acceleration, represented colloquially as 'fishy thing' (alpha), is also discussed, with its calculation methods and units (radians per second squared). The segment covers uniformly angularly accelerated motion, likened to uniformly accelerated motion, with five variables and four equations, emphasizing that knowing three variables allows solving for the remaining two. The concept of arc length is introduced with its equation and the importance of using radians for angular displacement. The video also touches on the relationship between arc length, linear velocity, and angular velocity, leading to the derivation of tangential velocity as the product of radius and angular velocity.

05:05
πŸ”„ Understanding Tangential and Centripetal Quantities

This paragraph delves into the concepts of tangential velocity and acceleration, which are linear measurements tangent to the circle's path and have units of meters per second and meters per second squared, respectively. The instructor clarifies that while the magnitude of tangential velocity remains constant in uniform circular motion, its direction changes continuously, necessitating a centripetal acceleration towards the circle's center. The equation for centripetal acceleration is presented, showing its relationship with tangential velocity, radius, and angular velocity. The video explains that centripetal force, derived from Newton's Second Law, is the net inward force causing circular motion and is not a new force but rather an inward-directed component of existing forces. The distinction between uniform and non-uniform circular motion is made, with the latter involving both centripetal and tangential accelerations, resulting in a total acceleration vector that is not aligned with the radius. The importance of radians in calculating these quantities is reiterated.

10:06
🌐 Advanced Concepts in Rotational Motion

The final paragraph reviews advanced concepts in rotational motion, starting with a recap of angular velocity and its relationship with the period of an object's revolution. The instructor uses the equation for angular velocity and explains how it relates to the period (T), with the formula for each being derived from the other. The video concludes with a brief mention of rotational dynamics and invites viewers to explore further through a dedicated AP Physics C review webpage. The instructor thanks the audience for their engagement, emphasizing the enjoyment of the learning process.

Mindmap
Keywords
πŸ’‘Angular Velocity
Angular velocity is a measure of the rate of change of an object's angular position, typically represented by the Greek letter omega (Ο‰). It is defined as the change in angular position divided by the change in time, and its units are radians per second. In the context of the video, angular velocity is fundamental to understanding rotational motion. For instance, the script mentions that 'average angular velocity equals the change in angular position over change in time' and 'instantaneous angular velocity equals the derivative of the angular position with respect to time'.
πŸ’‘Radian
A radian is a unit of angular measure used in many areas of mathematics, including physics, where it is particularly relevant for describing rotational motion. One radian is the angle subtended at the center of a circle by an arc equal in length to the radius of the circle. The video script emphasizes the importance of using radians when calculating arc length and other rotational quantities, as it states 'you must use radians for the angular displacement when using the equation for arc length'.
πŸ’‘Uniform Circular Motion
Uniform circular motion refers to the motion of an object moving in a circular path at a constant speed. In the video, it is mentioned that when the angular acceleration of an object equals zero, the motion is called uniform circular motion. This concept is crucial for understanding that even though the speed (magnitude of the tangential velocity) is constant, the direction of the velocity vector changes continuously, necessitating a centripetal force.
πŸ’‘Centripetal Acceleration
Centripetal acceleration is the acceleration experienced by an object moving in a circular path, directed towards the center of the circle. It is a result of the continuous change in the direction of the velocity vector, even if the speed is constant. The script explains that 'centripetal acceleration is the acceleration which is in toward the center of the circle, which the path of the object is describing' and is calculated as 'tangential velocity squared divided by the radius' or 'radius times the angular velocity squared'.
πŸ’‘Tangential Velocity
Tangential velocity is the component of an object's velocity that is tangent to the circular path it is following. It is the linear velocity of an object moving in a circle and is given by the equation 'tangential velocity equals radius times angular velocity'. The script uses this concept to explain that the derivative of arc length with respect to time yields the tangential velocity.
πŸ’‘Angular Acceleration
Angular acceleration is the rate at which an object's angular velocity changes over time, symbolized by alpha (Ξ±) and often referred to as a 'fishy thing' in the script. It is defined as the change in angular velocity over change in time or the derivative of angular velocity with respect to time. The script discusses angular acceleration in the context of non-uniform circular motion, where it contributes to the total acceleration of the object.
πŸ’‘Arc Length
Arc length is the linear distance traveled by an object along a circular path or arc. In the video, it is defined by the equation 's equals r delta theta', where 's' is the arc length, 'r' is the radius, and 'delta theta' is the angular displacement. The script emphasizes that radians must be used for angular displacement when using this equation.
πŸ’‘Tangential Acceleration
Tangential acceleration is the rate of change of tangential velocity with respect to time. It occurs when there is a change in the object's speed as it moves along a circular path. The script explains that 'tangential acceleration equals radius times angular acceleration', assuming the radius is constant.
πŸ’‘Centripetal Force
Centripetal force is the net force acting towards the center of a circular path in which an object is moving. According to Newton's Second Law, as explained in the script, there must be a centripetal force causing the centripetal acceleration when an object is in circular motion. It is calculated as mass times the centripetal acceleration.
πŸ’‘Non-Uniform Circular Motion
Non-uniform circular motion occurs when an object moves in a circle with a non-zero angular acceleration. This means the object's speed is changing as it moves along the circular path. The script mentions that in non-uniform circular motion, the object has both centripetal and tangential accelerations, resulting in a total acceleration that is the vector sum of these two components.
Highlights

Review of rotational kinematics in AP Physics C mechanics curriculum.

Symbol for angular velocity is the lowercase Greek letter omega.

Average angular velocity is change in angular position over time.

Instantaneous angular velocity is the derivative of angular position with respect to time.

Units for angular velocity are radians per second or revolutions per minute.

Symbol for angular acceleration is alpha, often called 'fishy thing'.

Average angular acceleration is change in angular velocity over time.

Instantaneous angular acceleration is the derivative of angular velocity with respect to time.

Units for angular acceleration are radians per second squared.

Uniformly angularly accelerated motion equations are similar to uniformly accelerated motion equations.

There are five U fishy M variables and four U fishy M equations in rotational kinematics.

Equation for arc length is s equals r times delta theta.

Arc length is the linear distance traveled along a circular path.

Radians are used for angular displacement in arc length equation.

Derivative of arc length with respect to time gives tangential velocity.

Tangential velocity equals radius times angular velocity.

Derivative of tangential velocity with respect to time gives tangential acceleration.

Tangential acceleration equals radius times angular acceleration.

Uniform circular motion occurs when angular acceleration is zero.

Centripetal acceleration is directed toward the center of the circular path.

Centripetal force is the net force acting inward on an object in circular motion.

Non-uniform circular motion involves both centripetal and tangential accelerations.

Angular velocity equals two pi divided by the period of the object.

Transcripts

Browse More Related Video

Rotational Motion: An Explanation, Angular Displacement, Velocity and Acceleration

AP Physics 1: Rotational Kinematics Review

Uniform Circular Motion Class 11

Circular Motion: Acceleration (Angular, Tangential, Centripetal)

Relating angular and regular motion variables | Physics | Khan Academy

Rotational Motion Physics, Basic Introduction, Angular Velocity & Tangential Acceleration

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Rotational KinematicsAP PhysicsEducational ContentPhysics CurriculumAngular VelocityCentripetal ForceUniform MotionNon-Uniform MotionConical PendulumTangential VelocityPhysics Review