Derivation of Formulas on Kinematics - Physics by maestirito
TLDRIn this informative video, the presenter guides viewers through the process of deriving essential kinematics formulas in physics, emphasizing the importance of understanding basic algebra and elementary mathematics concepts. The video focuses on three main formulas related to constant speed, average velocity, and acceleration, and demonstrates how to derive additional formulas from these, ultimately aiding in solving kinematics problems with ease and confidence.
Takeaways
- 📚 The video discusses kinematics, a topic in physics involving the study of motion without considering the forces that cause it.
- 🏃 Kinematics covers concepts like uniformly accelerated motion, free-falling bodies, and projectile motion, which are essential in physics education.
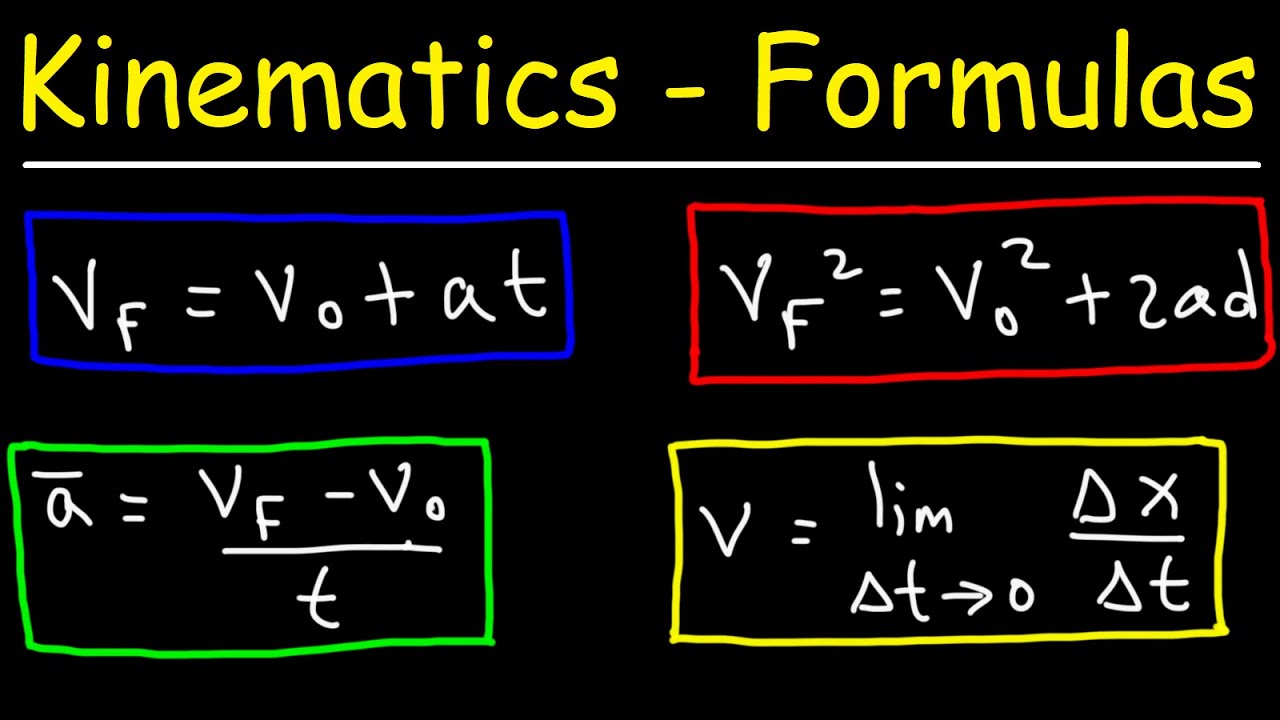
- 📝 Three main formulas are introduced: distance (D) = velocity (V) × time (T), average velocity (V_avg) = (VF + VI) / 2, and acceleration (a) = (VF - VI) / T.
- 🔄 The script emphasizes the importance of understanding how to derive formulas rather than just memorizing them, which can be beneficial for problem-solving.
- 🎯 The difference between scalar and vector quantities is highlighted, with speed being a scalar and velocity being a vector due to its directionality.
- 🤔 The video provides a method for deriving additional formulas from the three main ones, using basic algebra and elementary mathematical concepts.
- 📊 Cross-multiplication and the concept of the difference of squares are used to derive new formulas from the basic acceleration formula.
- 📈 The derived formulas allow for solving kinematics problems by substituting and manipulating the basic formulas to find unknown variables.
- 🌟 The video encourages viewers to have a strong foundation in elementary mathematics and algebra to better understand and apply kinematics formulas.
- 📌 The process of deriving formulas is demonstrated step by step, showing how to rearrange and simplify expressions to find solutions for various kinematics scenarios.
- 🚀 The video concludes by suggesting that a solid grasp of basic mathematical concepts can greatly assist in solving physics problems without the need to memorize numerous formulas.
Q & A
What are the three main kinematic formulas discussed in the video?
-The three main kinematic formulas discussed are: 1) Distance (D) = Velocity (V) * Time (T) for constant speed, 2) Average Velocity (V_avg) = (V_final + V_initial) / 2, and 3) Acceleration (a) = (V_final - V_initial) / T.
What is the significance of understanding the difference between speed and velocity?
-Understanding the difference between speed and velocity is crucial because speed is a scalar quantity with only magnitude while velocity is a vector quantity with both magnitude and direction. This distinction is important when dealing with kinematic problems as they often involve direction or displacement.
How can you derive the formula for time given only acceleration and final velocity?
-To derive the formula for time, rearrange the acceleration formula: a = (VF - VI) / T. By cross-multiplying and solving for T, you get T = (VF - VI) / a.
What is the method used in the video to derive additional kinematic formulas?
-The method used is cross-multiplication and applying basic algebraic concepts, such as factoring the difference of squares and simplifying expressions, to derive additional kinematic formulas from the three main formulas.
What is the difference of squares method mentioned in the video?
-The difference of squares method is an algebraic technique for factoring polynomials. For example, x^2 - 4 can be factored into (x + 2)(x - 2). This method is used in the video to simplify and derive kinematic formulas.
How can you find the final velocity (VF) using the acceleration formula?
-To find the final velocity (VF), rearrange the acceleration formula a = (VF - VI) / T by isolating VF, which gives VF = VI + aT.
What is the derived formula for distance when the velocity is not constant?
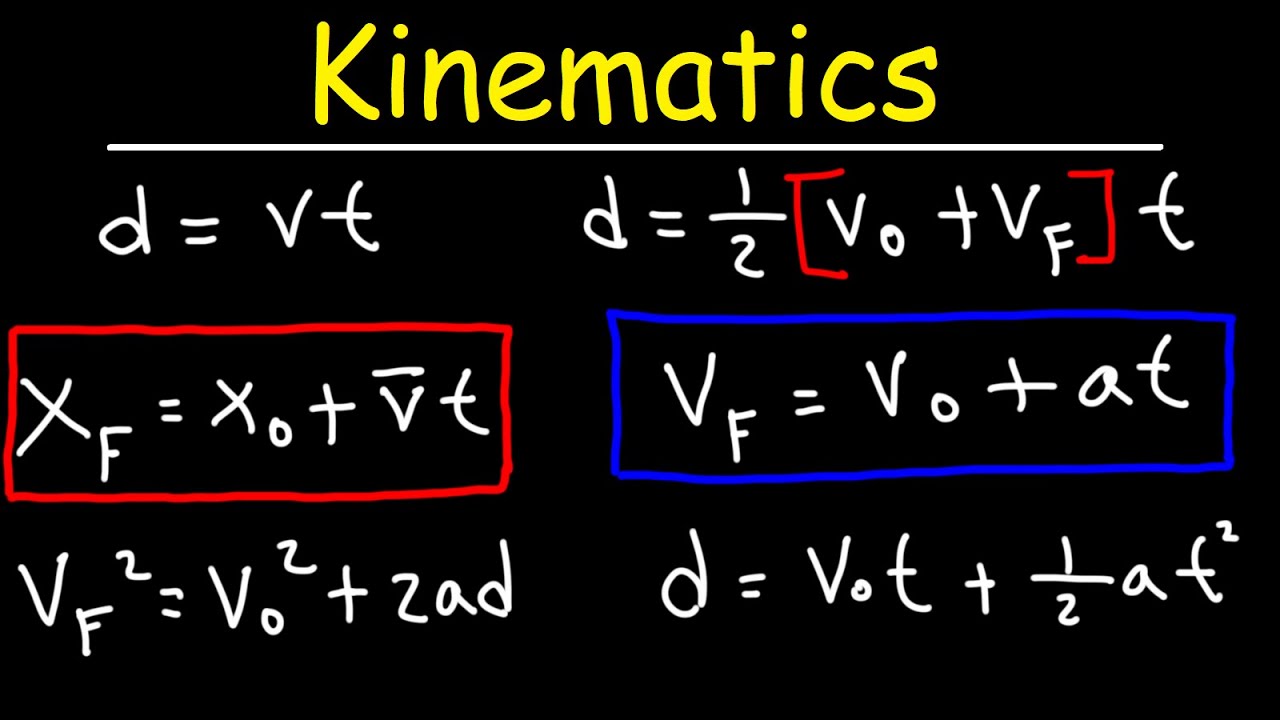
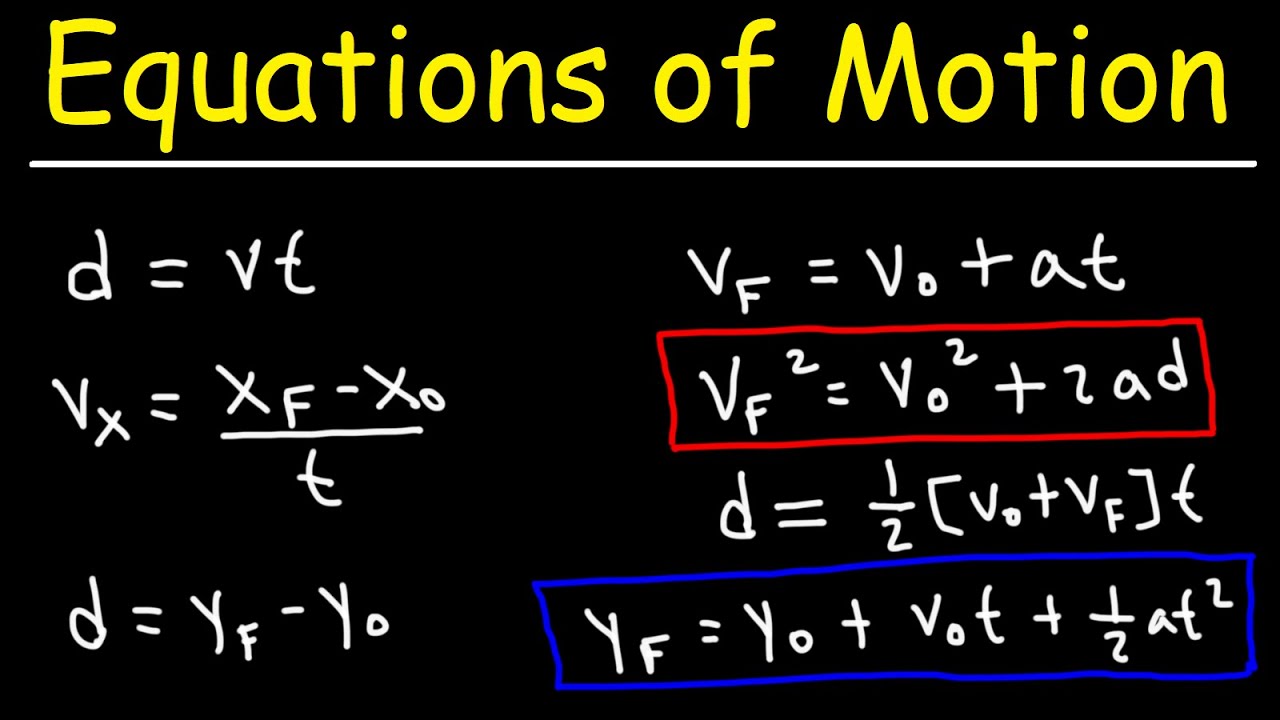
-The derived formula for distance when the velocity is not constant is D = VI*T + (a*T^2)/2.
What is the advice given in the video for solving physics problems?
-The advice given is to not always derive formulas but to use the basic concepts of algebra and elementary mathematics to manipulate and solve physics problems efficiently.
Why is it important to remember basic concepts from elementary mathematics and algebra?
-It is important because these fundamental concepts form the basis for solving more complex mathematical problems in kinematics and other areas of physics.
How does the video help in reducing the need to memorize numerous formulas?
-The video demonstrates how to derive various formulas from just three main kinematic formulas, thus reducing the need to memorize numerous formulas by understanding the underlying principles and relationships.
What is the role of cross-multiplication in deriving kinematic formulas?
-Cross-multiplication is used to rearrange and solve for different variables in the kinematic formulas, allowing for the derivation of additional formulas that can be used to solve a variety of kinematic problems.
Outlines
📘 Introduction to Kinematics and Basic Formulas
This paragraph introduces the topic of kinematics, a subfield of physics that deals with motion. The speaker explains that kinematics covers uniformly accelerated motion, free-falling bodies, and projectile motion. The main focus is on the formulas that will be used to solve problems in kinematics, specifically the distance (D), velocity (VF and VI), and acceleration (a). The speaker emphasizes that these formulas can be found in physics books and other educational resources, and the goal is to understand how they are derived rather than just memorizing them. The paragraph outlines the three main formulas: distance equals velocity times time (D = VT), average velocity (V_avg = (VF + VI) / 2), and acceleration (a = (VF - VI) / T). The speaker also differentiates between scalar and vector quantities, highlighting that velocity is a vector quantity with both magnitude and direction, while speed is a scalar quantity with only magnitude.
🔢 Deriving Additional Kinematic Formulas
In this paragraph, the speaker begins the process of deriving additional kinematic formulas from the basic ones introduced earlier. Starting with the formula for acceleration (a = (VF - VI) / T), the speaker uses cross-multiplication to derive formulas for time (T = (VF - VI) / a), final velocity (VF = VI + aT), and initial velocity (VI = VF - aT). The speaker then applies algebraic concepts, specifically the difference of squares, to further derive a formula for distance (D = VF^2 / 2a). The paragraph emphasizes the importance of understanding algebraic methods for solving kinematic problems and encourages the audience to review basic algebra and elementary mathematics concepts.
📚 Further Derivation and Simplification of Kinematic Formulas
The speaker continues the derivation process, focusing on simplifying the complex formulas obtained in the previous paragraph. By substituting VF and VI with expressions involving acceleration (a) and time (T), the speaker derives new formulas for distance (D = VI*T + 0.5*a*T^2). The paragraph emphasizes the application of basic algebra and elementary mathematics concepts, such as the distributive property and the concept of combining like terms, to simplify the formulas. The speaker also reiterates the importance of understanding these fundamental concepts to effectively work with kinematic problems.
🎓 Recap of Kinematic Formulas and Their Derivations
In this paragraph, the speaker recaps the main formulas discussed and the process of deriving additional formulas from them. The speaker reiterates the three main formulas: acceleration (a = (VF - VI) / T), average speed (V_avg = (VF + VI) / 2), and distance for constant speed (D = VT). The speaker then explains how these formulas can be used to derive other formulas, which can be helpful in solving various kinematic problems. The paragraph emphasizes the importance of understanding the derivation process rather than just memorizing formulas, as this understanding allows for better problem-solving and adaptability in physics.
🚀 Practical Application of Basic Kinematic Formulas
The speaker concludes the video by discussing the practical application of the basic kinematic formulas. The speaker suggests that in many cases, it is not necessary to derive formulas from scratch when solving physics problems; instead, one can directly use the basic formulas and apply fundamental algebraic and mathematical concepts. The speaker encourages the audience to be familiar with these basic concepts and to apply them in problem-solving. The video ends with a reminder that the speaker will demonstrate the application of these formulas in solving physics problems in future videos.
Mindmap
Keywords
💡Kinematics
💡Uniformly Accelerated Motion
💡Free-Falling Body
💡Projectile Motion
💡Acceleration
💡Velocity
💡Distance
💡Algebra
💡Scalar Quantity
💡Vector Quantity
💡Elementary Mathematics
Highlights
Introduction to the video's purpose of sharing how to derive formulas used in kinematics.
Discussion of uniformly accelerated motion, free-falling body, and projectile motion as part of kinematics in physics.
Explanation of the three main formulas used in kinematics: distance = velocity x time, average velocity = (VF + VI) / 2, and acceleration = (VF - VI) / time.
Clarification on the difference between speed and velocity, emphasizing that speed is a scalar quantity while velocity is a vector quantity.
Derivation of the formula for time using cross-multiplication from the acceleration formula.
Derivation of the formula for final velocity by isolating VF in the derived time formula.
Derivation of the formula for initial velocity by isolating VI in the derived time formula.
Application of the difference of squares in algebra to derive a new formula for distance given VF, VI, and acceleration.
Use of basic algebraic concepts to further simplify the derived distance formula.
Substitution of the derived velocity formulas into the distance formula to create additional derived formulas.
Emphasis on the importance of understanding fundamental concepts from elementary mathematics and algebra for problem-solving in physics.
The video's approach encourages understanding the derivation process rather than memorizing formulas for solving physics problems.
Preview of upcoming videos that will demonstrate solving physics problems using derived formulas.
Conclusion that memorizing formulas is not always necessary for solving physics problems due to the ability to manipulate and apply basic formulas effectively.
Advice for viewers to be familiar with basic mathematical concepts to better understand and solve physics problems.
Transcripts
Browse More Related Video
Kinematics in One Dimension Practice Problems: Constant Speed and Acceleration

Average velocity for constant acceleration | One-dimensional motion | Physics | Khan Academy
Kinematics Physics Formulas
Kinematics In One Dimension - Physics
Equations of Motion

Proving the Double and Half Angle Formulas for Trigonometry (Precalculus - Trigonometry 27)
5.0 / 5 (0 votes)
Thanks for rating: