Kinematics in One Dimension Practice Problems: Constant Speed and Acceleration

The Physics Teacher

15 Sept 201947:28

EducationalLearning

32 Likes 10 Comments

TLDRThe video script focuses on solving one-dimensional kinematics problems using key formulas for both constant speed and constant acceleration scenarios. It guides through calculating distances, average velocities, and accelerations, using examples of bicyclists on a trip, a skier going down a slope, a motorcycle with two different segments of motion, and trains meeting or chasing each other. The video emphasizes the importance of memorizing these formulas for successful problem-solving in quizzes and exams.

Takeaways

📐 The script focuses on solving one-dimensional kinematics problems using specific formulas for constant speed and constant acceleration.
🏃 For constant speed, the key formula is distance (d) equals velocity (v) times time (t), where v can be speed or velocity depending on the context.
🚴 The script provides four formulas for constant acceleration: the final velocity (v) is the sum of initial velocity (u), acceleration (a) times time (t), the square of final velocity equals the square of initial velocity plus 2 times acceleration times distance (s), and distance (s) equals half the sum of initial and final velocities times time (t).
📊 The script emphasizes the importance of memorizing these formulas for quizzes and exams as they are fundamental to solving kinematics problems.
🚴‍♀️ An example problem is given involving a bicyclist's trip in three parts with different speeds and times, demonstrating how to calculate total distance traveled and average velocity.
🏂 Another example problem involves a skier starting from rest and reaching a certain speed down a slope, highlighting the calculation of average acceleration and distance traveled.
🏍 A motorcycle's motion is dissected into two segments with different accelerations, showing how to find displacement in the second segment using the final velocity of the first segment as the initial velocity for the second.
🚴‍♂️ A competitive bicyclist accelerates to catch another bicyclist maintaining a constant speed, illustrating the concept of relative velocity and displacement.
🚂 The script also discusses two trains meeting when traveling in opposite directions and the same direction, emphasizing the use of relative velocity and constant speed formulas.
🚗 A car catches up to a bus traveling at a constant speed, using a quadratic equation to find the time it takes for the car to catch up, and highlighting the importance of considering the direction of motion.
📝 The script stresses the importance of unit consistency and proper unit conversion when plugging values into formulas to ensure accurate results.

Q & A

What is the formula for constant speed?
-The formula for constant speed is distance (d) equals velocity (v) times time (t), or d = v * t.
How many kinematic equations are there for constant acceleration?
-There are four kinematic equations for constant acceleration.
What is the first kinematic equation for constant acceleration?
-The first kinematic equation for constant acceleration is final velocity (v) equals initial velocity (u) plus acceleration (a) times time (t), or v = u + a*t.
What is the third kinematic equation for constant acceleration?
-The third kinematic equation for constant acceleration is distance (d) equals one-half of the initial velocity (u) plus the final velocity (v) times time (t), or d = (u + v)/2 * t.
How can you calculate the total distance traveled by the bicyclist in the given example?
-To calculate the total distance traveled by the bicyclist, you need to calculate the distance for each segment using the formula distance = velocity * time, and then sum up the individual distances.
What is the average velocity for the bicyclist's trip in the example?
-The average velocity for the bicyclist's trip is calculated by dividing the total distance traveled by the total time taken for the trip, which is approximately 6.73 meters per second.
How do you find the magnitude of average acceleration for the skier in the given example?
-To find the magnitude of average acceleration, use the formula final velocity (v) equals initial velocity (u) plus acceleration (a) times time (t). Solve for 'a' by rearranging the formula to a = (v - u) / t.
What is the displacement of the motorcycle during the second segment in the given example?
-The displacement of the motorcycle during the second segment is calculated using the formula final velocity (v) squared equals initial velocity (u) squared plus 2 times acceleration (a) times displacement (d). The initial velocity for the second segment is the final velocity of the first segment.
How can you determine the speed of the other bicyclist when one bicyclist catches up after one minute?
-To determine the speed of the other bicyclist, use the formula distance (d) equals velocity (v) times time (t). Since both bicyclists are at the same displacement after one minute, the distance covered by the second bicyclist is the same as the distance calculated for the first bicyclist.
What is the time it takes for two trains traveling in opposite directions to meet?
-The time it takes for two trains traveling in opposite directions to meet is found by setting up the equation for the total distance covered by both trains (distance1 + distance2) and equating it to the distance between them. Solve for time (T) using the formula distance = velocity * time.
How long will it take for a car to catch up to a bus traveling at a constant speed, given the car's initial speed and acceleration?
-To find the time it takes for the car to catch up to the bus, set up an equation where the car's final position (initial velocity times time plus one-half of acceleration times time squared) equals the bus's final position (initial lead plus constant speed times time). Solve for time using the quadratic formula if necessary.

Outlines

00:00

📚 Introduction to Kinematics

This paragraph introduces the topic of kinematics, focusing on one-dimensional motion. It emphasizes the importance of understanding and memorizing key formulas for constant speed and constant acceleration. The speaker provides four formulas for constant acceleration kinematics, which will be used to solve problems later in the video. The paragraph sets the stage for learning about the equations of motion and their application in physics.

05:02

🚴‍♀️ Solving a Multi-Segment Bicycle Trip

The speaker presents a problem involving a bicyclist's trip divided into three segments, each with different speeds and durations. The task is to calculate the total distance traveled and the average velocity of the bicyclist. The paragraph explains the process of using the distance formula (distance = velocity x time) for each segment and then summing the distances to find the total distance. It also discusses how to calculate the average velocity by dividing the total distance by the total time, providing a comprehensive walkthrough of the problem-solving process.

10:05

🏂 Calculation of Average Acceleration

This paragraph focuses on calculating the average acceleration of a skier starting from rest and reaching a certain speed after a specific time. The speaker provides the initial and final velocities, as well as the time taken, and explains how to use the kinematic formula to find acceleration. The paragraph also introduces another problem involving a gear's distance traveled under constant acceleration, demonstrating the application of the kinematic equations in different contexts.

15:06

🏍 Motorcycle Displacement with Changing Acceleration

The paragraph discusses a problem involving a motorcycle's displacement during two segments with different accelerations. The first segment starts from rest with a positive acceleration, and the second segment involves deceleration. The speaker explains how to find the final velocity at the end of the first segment and use it as the initial velocity for the second segment. The paragraph illustrates the application of the kinematic equation for constant acceleration to find the displacement during the second segment.

20:06

🚴‍♀️ Bicyclist Catch-Up Problem

This paragraph presents a scenario where one bicyclist is trying to catch up to another who is moving at a constant speed. The追赶者 accelerates at a given rate and catches up within a certain time. The paragraph explains how to calculate the distance each bicyclist travels and how to determine the speed of the constant-speed bicyclist. It highlights the importance of understanding the relationship between initial velocity, acceleration, and time in solving kinematic problems.

25:07

🚂 Trains Meeting in Opposite Directions

The paragraph explores a problem involving two trains traveling towards each other from a certain distance apart. The speaker explains how to calculate the time it takes for the trains to meet by using the distance formula and the relative speeds of the trains. The paragraph also considers a scenario where the trains are traveling in the same direction and introduces the concept of relative velocity to determine when they would meet.

30:10

🚗 Car Catching Up to a Bus

This paragraph presents a problem involving a car trying to catch up to a bus traveling at a constant speed. The speaker provides the initial conditions, including the car's initial speed, acceleration, and the distance between the two vehicles. The paragraph explains how to set up and solve a quadratic equation to find the time it takes for the car to catch up to the bus, demonstrating the application of kinematic equations in real-world scenarios.

Mindmap

Keywords

💡Kinematics

Kinematics is the branch of physics that deals with the motion of objects without considering the forces that cause the motion. In the video, it is the central theme as the speaker focuses on solving problems related to constant speed and constant acceleration using kinematic equations. The video demonstrates how to apply these principles to calculate various aspects of motion, such as distance traveled and average velocity.

💡Constant Speed

Constant speed refers to the motion of an object where its velocity remains unchanged over time. In the context of the video, problems involving constant speed utilize the formula distance equals velocity times time (d = v*t) to determine the distance traveled by an object moving at a uniform speed. This concept is fundamental in kinematics and is used to solve the first problem in the video where a bicyclist rides at a constant speed for different segments of a trip.

💡Constant Acceleration

Constant acceleration is a physical quantity that describes the rate of change of velocity per unit time, remaining constant throughout the motion. In the video, the speaker introduces four kinematic equations for scenarios involving constant acceleration, which are crucial for solving problems where the speed of an object changes at a steady rate. These equations allow the calculation of final velocity, distance traveled, and time taken based on initial conditions and acceleration.

💡Average Velocity

Average velocity is defined as the total displacement an object travels divided by the total time taken. It is a vector quantity that considers the overall change in position and the time interval, regardless of the speed variations during the motion. In the video, the speaker emphasizes the importance of calculating average velocity for problems involving changing speeds and uses it to find the bicyclist's average velocity over the entire trip.

💡Displacement

Displacement is the straight-line distance between the starting point and the final position of an object in motion. It is a vector quantity that has both magnitude and direction. In the context of the video, displacement is used to describe the change in position of objects like the bicyclist and the trains during their respective journeys. The speaker uses the concept of displacement to calculate the distance traveled by the objects in various segments of their motion.

💡Acceleration

Acceleration is the rate at which an object's velocity changes over time. It is a vector quantity, meaning it has both magnitude and direction. In the video, the speaker discusses constant acceleration, where the acceleration remains the same throughout the motion, and uses it to solve problems involving changes in velocity. The concept of acceleration is essential for understanding how quickly an object speeds up or slows down and is used to calculate the time it takes for a car to catch up to a bus.

💡Initial Velocity

Initial velocity is the speed of an object at the beginning of its motion or at a specific point in time. It is a crucial parameter in kinematic equations, especially when dealing with problems involving constant acceleration. In the video, the speaker uses initial velocity to set up equations for solving problems, such as the one where a bicyclist starts to accelerate to catch up to another cyclist maintaining a constant speed.

💡Final Velocity

Final velocity is the speed of an object at the end of a specified time interval or at a particular moment in its motion. It is an essential element in kinematic equations for calculating the motion of objects under constant speed or constant acceleration. In the video, the speaker uses final velocity to determine the motion outcomes, such as the speed of a skier at the end of a slope or the velocity of a car when it catches up to a bus.

💡Time

Time is a fundamental parameter in kinematics that represents the duration of an object's motion. It is used in conjunction with velocity and acceleration to calculate various aspects of motion, such as distance traveled and displacement. In the video, the speaker emphasizes the importance of time in solving kinematic problems, as it is a factor in determining the distance covered, the change in velocity, and the outcome of the motion.

💡Relative Velocity

Relative velocity is the velocity of an object with respect to another moving object. It takes into account the motion of both objects and is used to determine the change in position between them. In the video, the concept of relative velocity is applied when two trains are moving in the same direction, and the speaker uses it to calculate the time it takes for one train to catch up to the other.

Highlights

The video focuses on solving one-dimensional kinematics problems using specific formulas.

For constant speed, the formula used is distance equals velocity times time.

Four formulas are provided for constant acceleration kinematics problems.

The first formula for constant acceleration relates final velocity to initial velocity, acceleration, and time.

The second formula for constant acceleration involves the square of final velocity based on initial velocity, acceleration, and distance.

The third formula for constant acceleration calculates distance as the sum of half the initial and final velocities multiplied by time.

The last formula for constant acceleration computes distance as the product of initial velocity and time, plus half of acceleration times time squared.

The video presents a problem-solving approach by first memorizing the formulas and then applying them to specific scenarios.

A bicyclist trip example is given, involving three segments with varying speeds and times.

The total distance traveled by the bicyclist is calculated by summing the individual distances of each trip segment.

Average velocity for the bicyclist trip is determined by dividing the total distance by the total time.

Another problem involves a skier starting from rest and reaching a certain speed down a slope, highlighting the use of kinematic equations for constant acceleration.

The concept of average acceleration is introduced, calculated as the change in velocity over time.

A motorcycle example is used to demonstrate the application of kinematic equations in two distinct segments of motion with different accelerations.

The final velocity in the first segment of the motorcycle example is used as the initial velocity for the second segment, showcasing the connection between motion segments.

A problem involving two bicyclists is solved, one accelerating to catch up to the other maintaining a constant speed, illustrating the use of relative velocity and displacement concepts.

The video concludes with a problem about a car catching up to a bus, using quadratic equations to find the time it takes for the car to catch up.

The video emphasizes the importance of memorizing formulas for kinematics and applying them correctly to solve problems.

Transcripts

Browse More Related Video

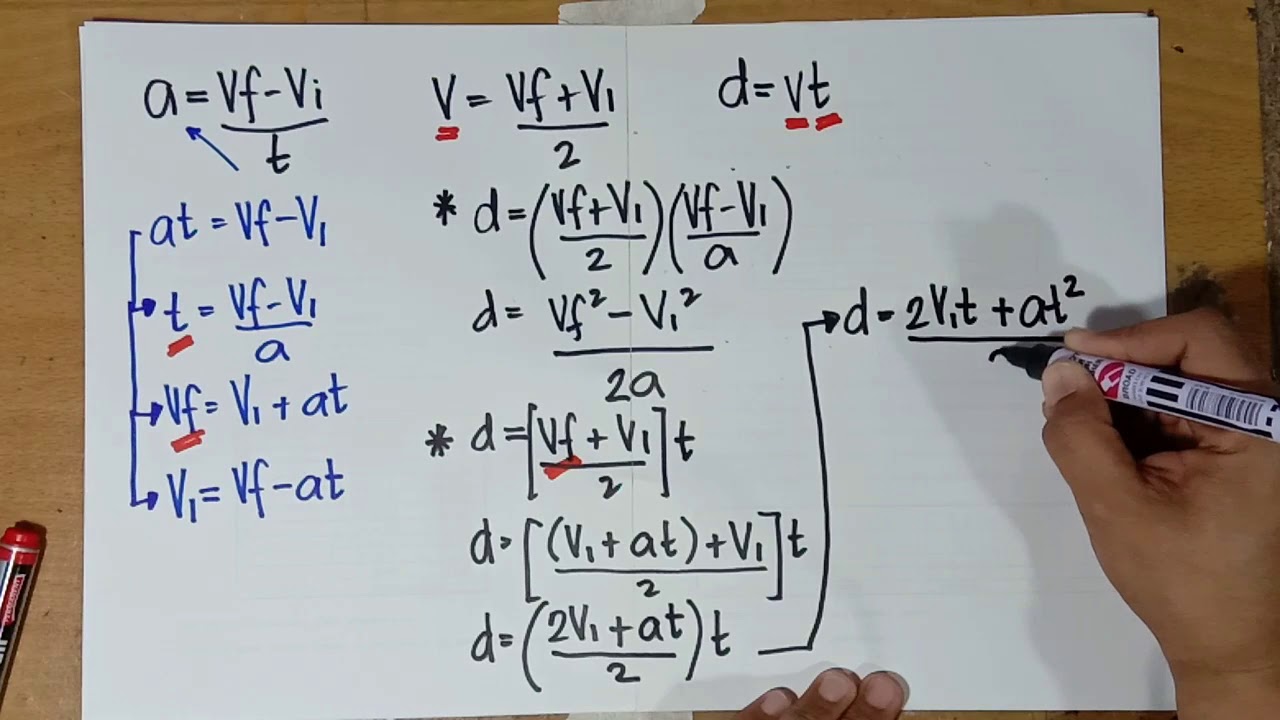
Derivation of Formulas on Kinematics - Physics by maestirito

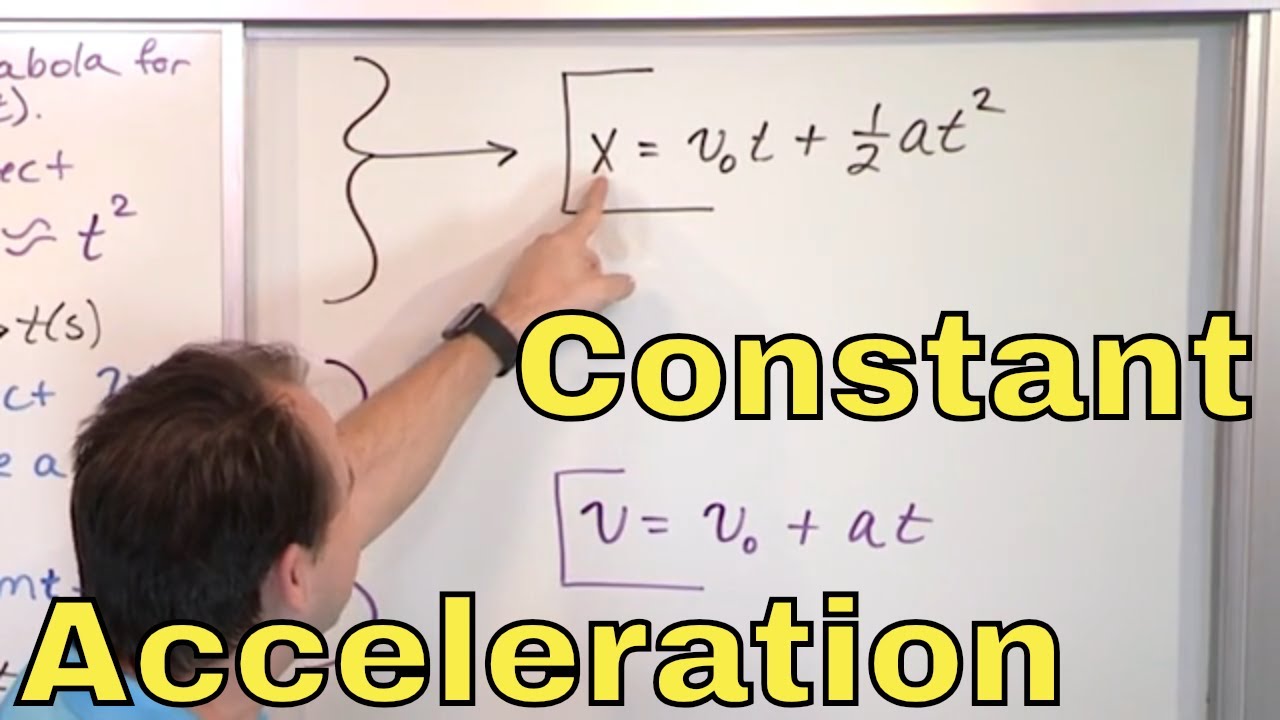
01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

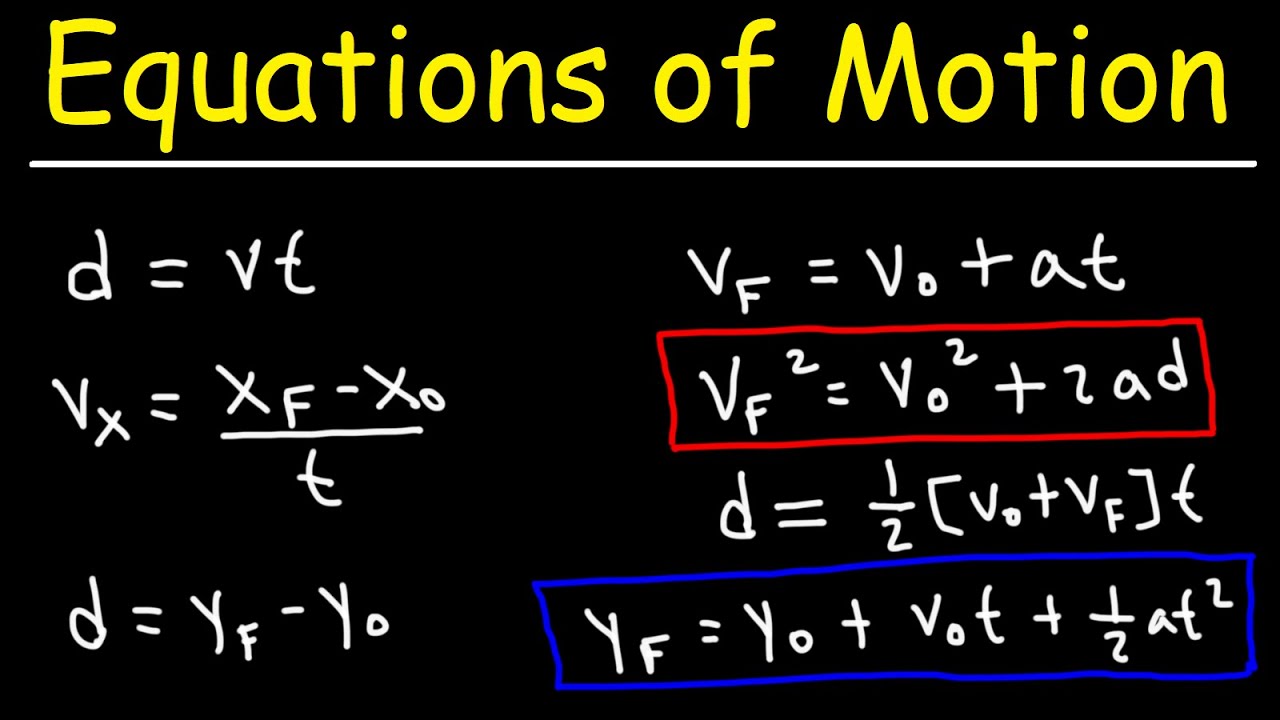
Equations of Motion

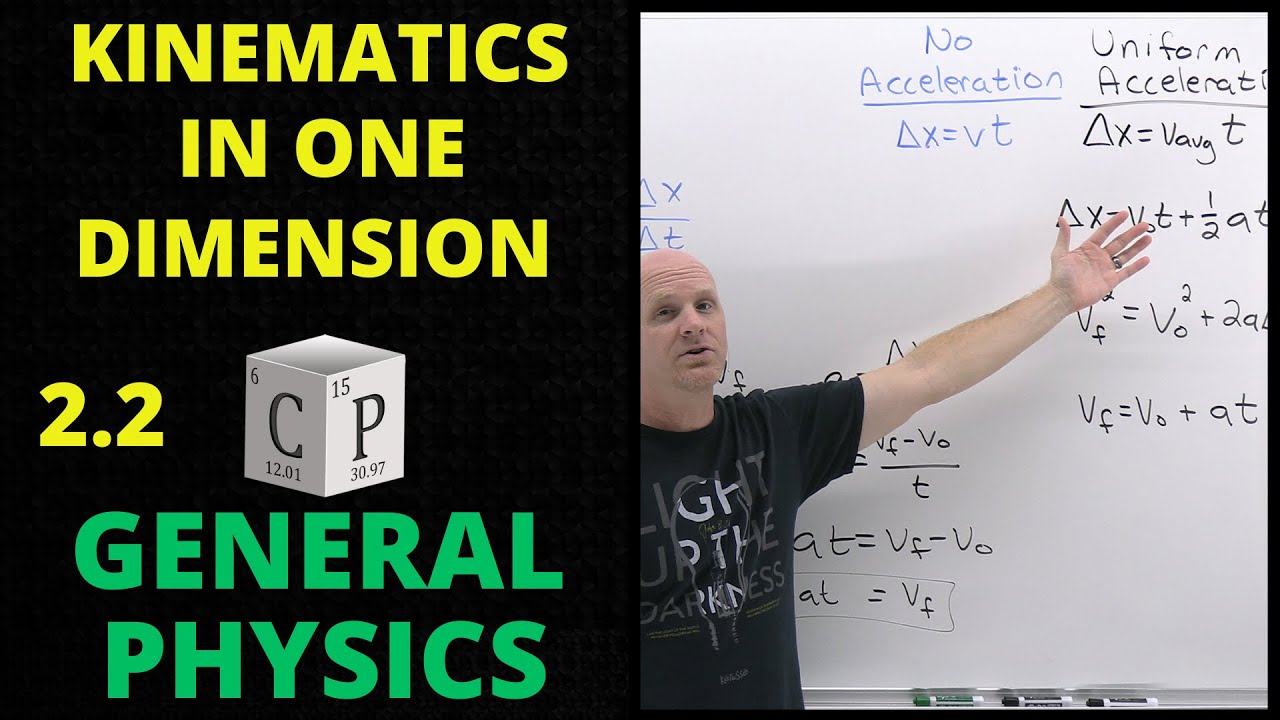
2.2 Kinematics in One Dimension | General Physics

College Physics 1: Lecture 10 - Solving 1-D Motion Problems

2 | FRQ (Question 4/5: Short Answer) | Practice Sessions | AP Physics 1

Related Tags

Physics Education Kinematics Formulas Problem Solving Constant Speed Acceleration Bicyclist Motion Train Meeting Car Catch-Up Quadratic Equations Real-World Applications