Average velocity for constant acceleration | One-dimensional motion | Physics | Khan Academy
TLDRThe video script delves into fundamental physics concepts, emphasizing the intuitive nature of formulas related to motion. It illustrates the relationship between velocity, acceleration, and distance traveled using a hypothetical scenario of constant acceleration. The video explains how velocity changes over time due to acceleration and how this affects the total distance covered. It also introduces the concept of average velocity as a means to calculate displacement under constant acceleration, demonstrating that physics formulas are grounded in logical reasoning and can be deduced from basic principles.
Takeaways
- 📐 The video aims to demystify physics concepts by showing they are rooted in common sense.
- 🚀 It uses a one-dimensional motion example with positive velocity indicating rightward movement and negative indicating leftward.
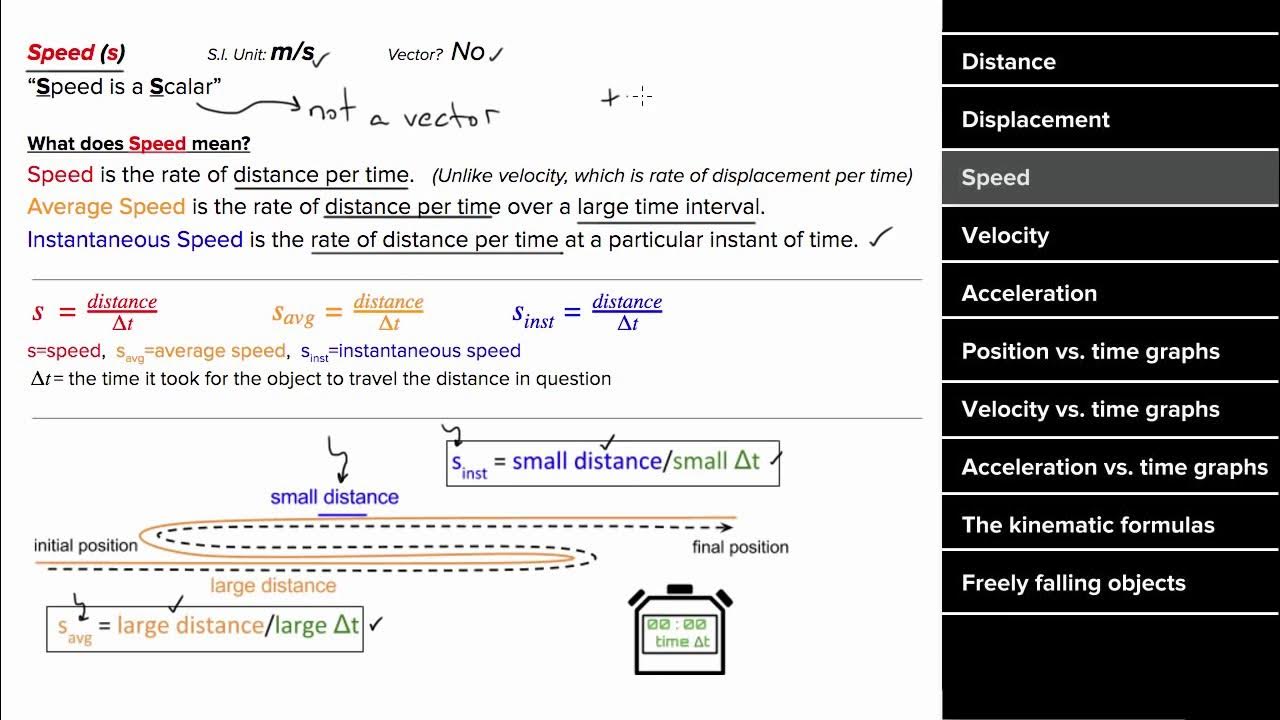
- 🛤️ The example starts with an initial velocity of 5 m/s to the right and a constant acceleration of 2 m/s² also to the right.
- 🕒 After 4 seconds, the final velocity is calculated by adding the initial velocity to the product of acceleration and time (5 m/s + 4s * 2 m/s² = 13 m/s).
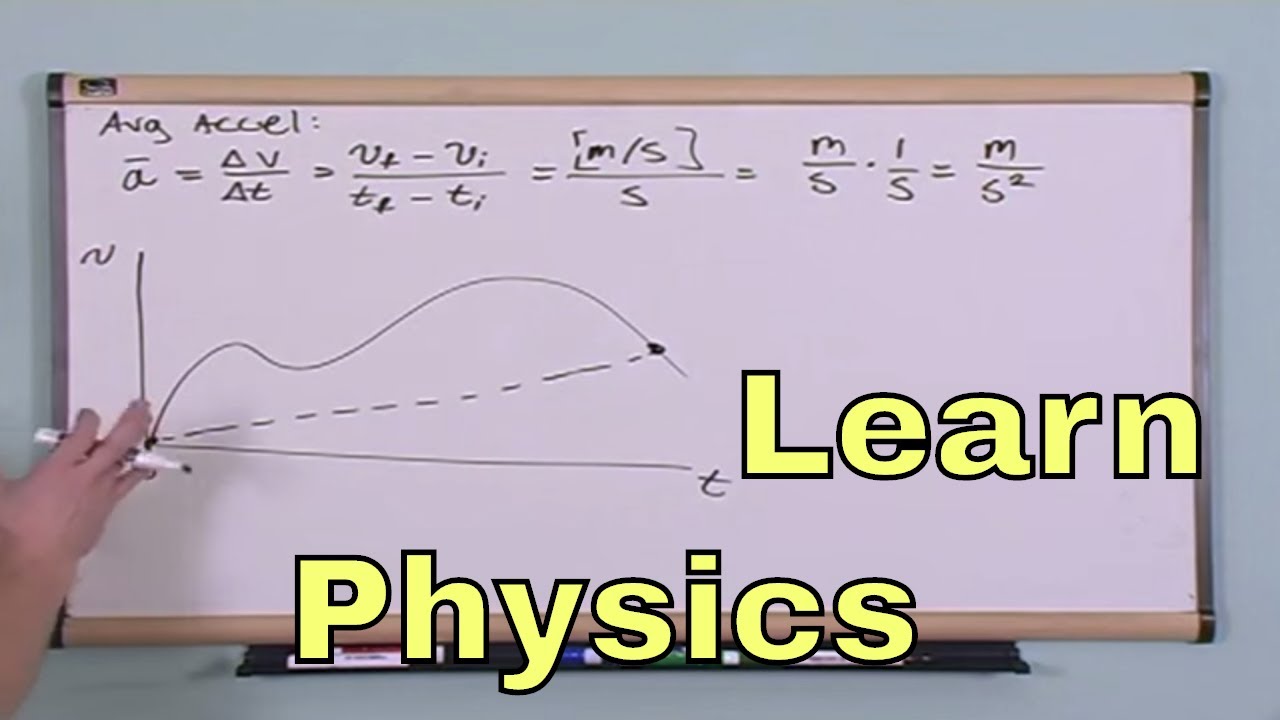
- 📈 The concept of acceleration is visualized as the slope of the velocity line, indicating the change in velocity over time.
- 🏁 The total distance traveled is derived from the area under the velocity-time curve, broken down into a rectangle and a triangle for simplicity.
- 📂 The area of the rectangle represents the distance with no acceleration, calculated as initial velocity times time (5 m/s * 4s = 20 m).
- 🔺 The area of the triangle represents the additional distance due to acceleration, calculated as 0.5 * base * height (0.5 * 4s * 8 m/s = 16 m).
- 📊 The total displacement is the sum of the areas of the rectangle and triangle, which equals 36 meters to the right.
- 🌟 The video emphasizes the importance of understanding the logic and reasoning behind formulas rather than just memorizing them.
- 🔄 The average velocity is introduced as a useful concept when acceleration is constant, defined as the arithmetic mean of initial and final velocities.
Q & A
What is the main goal of the video?
-The main goal of the video is to explore traditional physics concepts and formulas, emphasizing that they are based on common sense ideas.
How does the video simplify the concept of velocity?
-The video simplifies velocity by using positive and negative numbers to represent direction and magnitude, with positive indicating movement to the right and negative to the left.
What is the significance of constant acceleration in the video example?
-Constant acceleration, represented as two meters per second squared, indicates that the velocity increases by two meters per second for every second that passes, which affects the final velocity and distance traveled.
How does the video calculate the final velocity after a given time?
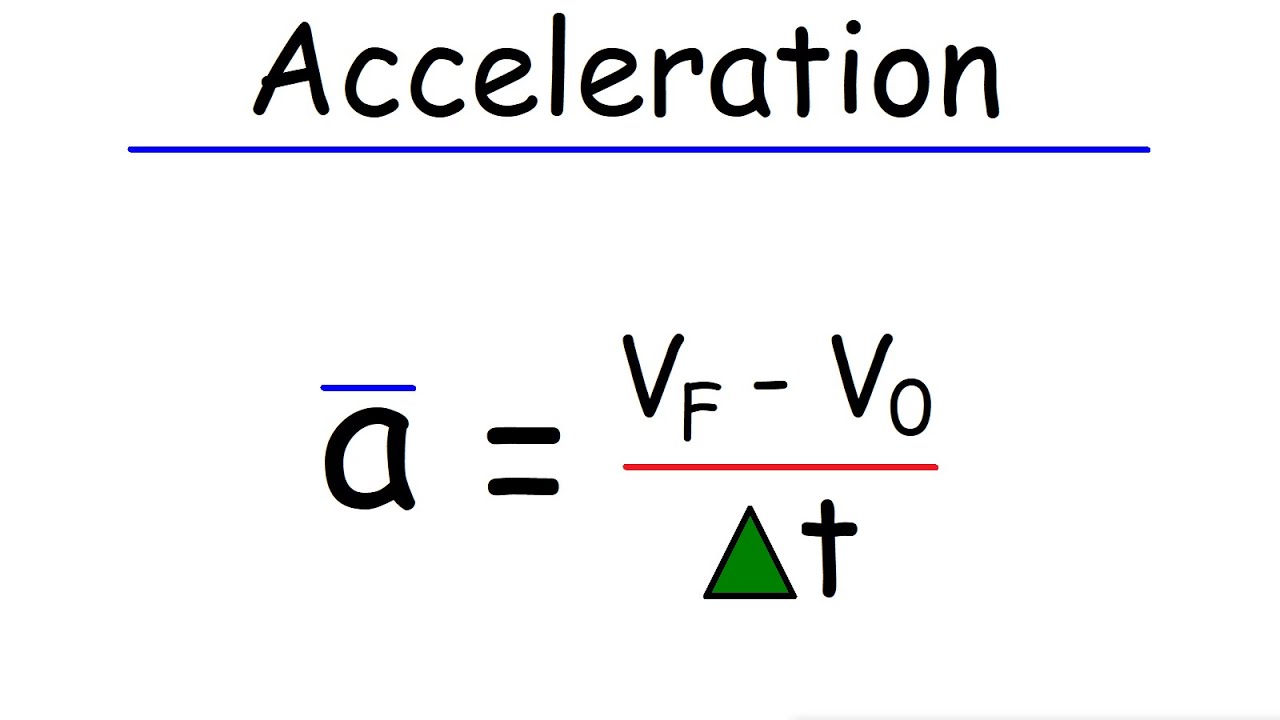
-The final velocity is calculated by adding the initial velocity to the product of the acceleration and the time duration (v_f = v_i + a * t).
What is the method used in the video to find the total distance traveled?
-The total distance traveled is found by calculating the area under the velocity-time graph, which is broken down into a rectangle and a triangle, and their combined area represents the displacement.
How does the concept of average velocity relate to the distance traveled in the video's example?
-The average velocity is used to find the total distance traveled when there is constant acceleration. It is calculated as half the sum of the initial and final velocities (v_avg = (v_i + v_f) / 2), and multiplying this by the time duration gives the distance.
What is the arithmetic mean of the initial and final velocities in the video example?
-The arithmetic mean of the initial velocity (5 m/s) and the final velocity (13 m/s) is 9 m/s, which is also the calculated average velocity used to determine the distance traveled.
How does the video emphasize the importance of understanding physics formulas?
-The video emphasizes that understanding physics formulas is crucial because they are not just memorized formulas but are derived from logical reasoning and common sense ideas that can be deduced through understanding the underlying concepts.
What is the relationship between the change in velocity and the time duration in the context of constant acceleration?
-The change in velocity due to constant acceleration is directly proportional to the time duration. The longer the duration, the greater the change in velocity, as acceleration remains constant.
What is the significance of the direction of velocity in the video's explanation?
-The direction of velocity is significant as it helps in understanding the positive and negative values representing movement to the right or left, respectively, and how these values affect the final velocity and displacement.
What is the final displacement calculated in the video example?
-The final displacement calculated in the video example is 36 meters, which is the total distance traveled to the right, considering both the initial velocity and the acceleration over time.
Outlines
🌟 Introduction to Velocity and Acceleration Concepts
The video begins by introducing the fundamental concepts of velocity and acceleration as they are typically taught in a physics class. It emphasizes the importance of understanding these ideas as common-sense notions rather than abstract formulas. The video sets a scenario where velocity is represented by a positive number indicating movement to the right and negative numbers to the left. An initial velocity of 5 meters per second is assumed, and a constant acceleration of 2 meters per second squared is introduced. The goal is to explore the final velocity after a duration of 4 seconds and the distance traveled during this time.
🚀 Calculating Velocity Over Time with Constant Acceleration
This paragraph delves into the calculation of velocity over time when a constant acceleration is applied. It explains how the velocity increases by 2 meters per second for every second that passes, starting from an initial velocity of 5 meters per second. The paragraph illustrates this with a step-by-step explanation, showing that after 1 second the velocity is 7 meters per second, after 2 seconds it is 9 meters per second, and so on, culminating in a final velocity of 13 meters per second after 4 seconds. The paragraph aims to demonstrate that the formula used to calculate this increase in velocity is intuitive and based on logical reasoning.
📏 Understanding Displacement through Geometry
The focus of this paragraph is on calculating the total distance traveled, or displacement, when there is constant acceleration. It introduces the concept of representing distance as the area under the velocity-time curve and simplifies this area into two easily calculable shapes: a rectangle and a triangle. The rectangle represents the distance that would be traveled without acceleration, calculated as the initial velocity (5 meters per second) multiplied by the time (4 seconds), resulting in 20 meters. The triangle represents the additional distance due to acceleration, calculated as half the product of the time (4 seconds) and the change in velocity (final velocity of 13 meters per second minus initial velocity of 5 meters per second, which equals 8 meters per second), resulting in an additional 16 meters. The total displacement is thus 36 meters to the right.
📈 Deriving the Formula for Distance Traveled
The final paragraph discusses the derivation of the formula for the distance traveled when there is constant acceleration. It explains how the total distance can be calculated by taking the initial velocity, adding the final velocity, and then dividing by two, which gives the average velocity in this specific case. This average velocity is used to determine the distance traveled by multiplying it by the time interval. The paragraph emphasizes that this method is only applicable when there is a constant acceleration and that the average velocity is the arithmetic mean of the initial and final velocities. It concludes by reinforcing the idea that physics formulas can be deduced logically rather than just memorized.
Mindmap
Keywords
💡Physics
💡Concepts
💡Formulas
💡Velocity
💡Acceleration
💡Displacement
💡Direction
💡Constant
💡Time
💡Area
💡Average Velocity
Highlights
Exploring concepts and formulas in traditional physics class through common sense ideas.
Using positive and negative numbers to represent direction in one-dimensional motion.
Defining velocity with magnitude and direction, such as positive velocity indicating movement to the right.
Introducing constant acceleration and its representation in a one-dimensional motion scenario.
Calculating final velocity after a given time period with constant acceleration using the formula v = u + at.
Visualizing the motion with a velocity-time diagram to understand the increase in velocity over time.
Deriving the total distance traveled by combining the areas under the velocity-time curve.
Using geometry to break down the curve into a rectangle and a triangle to calculate the distance traveled.
Calculating the area of the rectangle representing the distance with constant velocity.
Finding the area of the triangle representing the additional distance due to acceleration.
Summing the areas of the rectangle and triangle to get the total displacement in the motion scenario.
Expressing the total distance traveled with the formula d = (v_i + v_f)/2 * t, where v_i is initial velocity, v_f is final velocity, and t is time.
Highlighting the importance of understanding the logical flow behind physics formulas rather than just memorizing them.
Explaining how the average velocity can be used to calculate the distance traveled in constant acceleration scenarios.
Deducing the formula for distance traveled from first principles without relying on memorization.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: