Potential Energy and Conservative Forces - Gradient Vectors | Physics | Calculus
TLDRThis script delves into the relationship between force, potential energy, and momentum as described by Newton's Second Law. It explains how the net force on an object is related to changes in momentum and potential energy, highlighting the concept of conservative forces and their work. The script further illustrates how the direction of force relative to displacement affects potential energy, using examples such as gravity, springs, and electric fields. It also covers how to derive force functions from potential energy functions and emphasizes the negative gradient concept in determining the force vector. The explanation is complemented with practical examples and problem-solving techniques, reinforcing the principles of physics.
Takeaways
- 📚 Newton's Second Law states that the net force on an object is equal to the mass of the object times its acceleration, which can also be described as the rate of change of momentum.
- 🔄 The net force on an object is the derivative of the momentum function with respect to time, and the force is the negative derivative of the potential energy function with respect to position.
- ⚖️ Work done by a conservative force is equal to the negative change in potential energy, and work done by any force is equal to the magnitude of the force times the displacement.
- 💥 When force and displacement vectors are in the same direction, the object's kinetic energy increases and potential energy decreases, and vice versa when they are anti-parallel.
- 🌐 The force function can be derived from the potential energy function by taking the negative derivative with respect to the relevant coordinate (e.g., X, Y, or Z).
- 📈 The change in potential energy can be calculated graphically by finding the area under the force curve and multiplying by -1.
- 🔌 The electric force between two charges is derived from the potential energy function of the charges and is given by the equation F = k*q1*q2/r^2.
- 🔄 The relationship between force, displacement, and potential energy is such that potential energy increases when force and displacement are opposite and decreases when they are parallel.
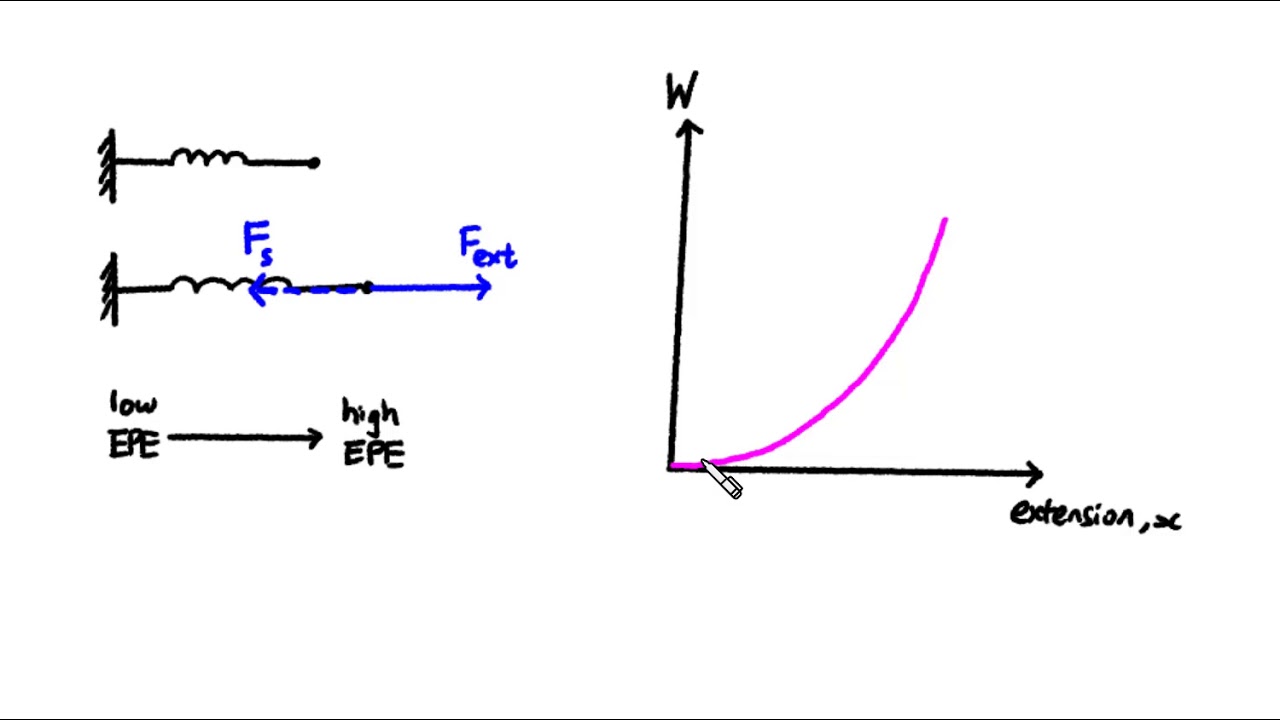
- 🎢 For a spring, the potential energy is given by 1/2 kx^2, and the force function derived from this is F = -kx, indicating the restoring force's magnitude and direction.
- 📊 The X component of force can be determined by calculating the partial derivative of the potential energy function with respect to X, and this applies similarly for Y and Z components.
- 🔧 The concept of gradient is introduced as the force being the negative gradient of the potential energy function, which can be used to find the direction and magnitude of the force vector.
Q & A
What is Newton's Second Law and how does it relate to force, mass, and acceleration?
-Newton's Second Law states that the net force acting on an object is equal to the mass of the object multiplied by its acceleration. This law establishes a fundamental relationship between these three quantities, showing that force is the product of mass and the rate of change of velocity (acceleration).
How is momentum defined and related to force and velocity?
-Momentum is defined as the product of an object's mass and its velocity. It is a vector quantity that represents the motion of an object. Since force is the rate of change of momentum with respect to time, the net force on an object is equal to the derivative of its momentum function.
What is the relationship between work done by a conservative force and potential energy?
-The work done by a conservative force is equal to the negative change in potential energy. This means that when a conservative force does work on an object, it results in a change in the object's potential energy without any loss of mechanical energy in the system.
How does the direction of force and displacement vectors affect the work done and potential energy?
-When the force and displacement vectors are in the same direction, the work done is positive, and the potential energy decreases. Conversely, when the vectors are in opposite directions (anti-parallel), the work done is negative, and the potential energy increases.
What happens to the potential energy of an object when it falls under the influence of gravity?
-As an object falls under the influence of gravity, its potential energy decreases. This is because the object is moving in the direction of the gravitational force, which is conservative, leading to a negative change in potential energy.
How is the force function derived from the potential energy function for a spring?
-The force function for a spring is derived by taking the negative derivative of the potential energy function with respect to the displacement from the equilibrium position. The potential energy of a spring is given by (1/2)kx^2, and its force function is kx, where k is the spring constant and x is the displacement.
What is the relationship between electric force and potential energy in the context of charged particles?
-The electric force between charged particles is the conservative force that leads to changes in potential energy. The potential energy between two charges is given by the equation U = (k*q1*q2)/r, where k is Coulomb's constant, q1 and q2 are the charges, and r is the distance between the charges. The electric force is the negative gradient of this potential energy function.
How can the force vector be represented in terms of its components and the unit vectors?
-The force vector can be represented as the negative sum of the partial derivatives of the potential energy function with respect to each coordinate direction, multiplied by the corresponding unit vector. For example, F = -(∂U/∂x)I - (∂U/∂y)J - (∂U/∂z)K, where I, J, and K are the unit vectors in the x, y, and z directions, respectively.
What is the significance of the negative sign in the relationship between force and potential energy?
-The negative sign indicates that the force and the change in potential energy are in opposite directions. When an object moves in the direction of the force (displacement and force vectors are parallel), potential energy decreases, and when it moves against the force (vectors are anti-parallel), potential energy increases.
How can the change in potential energy be calculated for an object moving along the x-axis under the influence of gravity?
-The change in potential energy can be calculated by integrating the force function (which is the negative derivative of the potential energy function) over the displacement along the x-axis. The potential energy change is equal to the negative of the integral of the force function from the initial to the final position.
What is the concept of gradient and how does it relate to force and potential energy?
-The gradient is a mathematical operation that finds the rate of change (or slope) of a function at a given point. In the context of force and potential energy, the force is the negative gradient of the potential energy function. This means that the direction of the force vector is opposite to the direction of the greatest increase in potential energy.
Outlines
📚 Introduction to Force, Momentum, and Potential Energy
This paragraph introduces fundamental concepts of physics, including Newton's Second Law, momentum, and potential energy. It explains the relationship between net force, mass, and acceleration, and how acceleration is the rate of change of velocity. The paragraph further discusses how momentum (mass times velocity) changes with the application of force over time. It also delves into the concept of potential energy in relation to work done by conservative forces and the concept of displacement. The key takeaway is understanding that the net force on an object is the derivative of the momentum function with respect to time, and the force in a conservative system is the negative derivative of potential energy with respect to position.
📈 Work, Kinetic Energy, and Potential Energy
This section discusses the relationship between work done by a force, kinetic energy, and potential energy. It explains how the work done by a conservative force is equal to the negative change in potential energy, and how force is related to the displacement of an object. The paragraph clarifies that when force and displacement vectors are in the same direction, the kinetic energy of the object increases while the potential energy decreases. Conversely, when the force and displacement vectors are anti-parallel, the potential energy increases. The examples of gravity and spring force are used to illustrate these concepts, emphasizing the importance of understanding the direction of force and displacement vectors in relation to potential energy changes.
🔄 The Dynamics of Charged Particles and Electric Fields
This paragraph explores the dynamics of charged particles in electric fields and the associated changes in potential energy. It explains how the electric force, which is a conservative force, affects the potential energy of a charge. The section discusses scenarios where a charge is moved against or along the direction of the electric force and how this affects the potential energy. The concept of electric field and its relationship with electric force is also clarified. The key point is understanding that the potential energy of a charge increases when it moves against the electric force and decreases when it moves in the direction of the electric force.
📊 Deriving Force from Potential Energy Functions
This section focuses on deriving force functions from potential energy functions for different scenarios. It explains how to calculate the force function by taking the negative derivative of the potential energy function. The examples of gravitational force, spring force, and electrostatic force are used to illustrate this process. The paragraph emphasizes the mathematical relationship between force and potential energy, and how to apply this knowledge to derive common force equations such as weight due to gravity, spring force, and electrostatic force between charges.
📐 Calculating Force Components and Gradients
This paragraph discusses the concept of force components and gradients in relation to potential energy. It explains how to calculate the X, Y, and Z components of force by taking the partial derivatives of the potential energy function with respect to each coordinate. The concept of negative gradient is introduced as a way to understand force as the direction opposite to the increase in potential energy. The paragraph also explains how to represent force vectors using unit vectors and their magnitudes. The key takeaway is understanding the mathematical representation of force components and their relationship with the gradients of potential energy.
📝 Practice Problem: Calculating Force and Potential Energy
This section presents a practice problem involving the calculation of force and potential energy. It provides a potential energy function and asks for the force function at a specific point, as well as the force vector at various points on a graph of the potential energy. The paragraph guides through the process of taking derivatives and evaluating them at given points to find the force components. It also explains how to calculate the change in potential energy using the area under the force curve on a graph. The key points are applying the concepts of derivatives and integrals to find forces and potential energy changes, and interpreting these results on a graphical representation.
📋 Summary of Concepts and Calculations
This paragraph summarizes the main concepts and calculations discussed in the video script. It reiterates the relationship between force, potential energy, and displacement, emphasizing the importance of the direction of these quantities. The paragraph highlights how potential energy changes in response to the direction of the conservative force and displacement vectors. It also recaps the method of calculating the change in potential energy from a force function, either through integration or by calculating the area under a graph. The key takeaway is the comprehensive understanding of the interplay between force, work, displacement, and potential energy in a conservative system.
Mindmap
Keywords
💡Newton's Second Law
💡Momentum
💡Potential Energy
💡Conservative Force
💡Derivative
💡Displacement
💡Kinetic Energy
💡Gradient Vector
💡Work
💡Restoring Force
Highlights
Newton's Second Law relates net force to mass and acceleration, providing a fundamental principle in physics.
Momentum, the product of mass and velocity, is central to understanding changes in an object's motion.
Derivative of momentum function with respect to time equals net force, offering an alternative expression of Newton's Second Law.
Work done by a conservative force is equal to the negative change in potential energy, a key concept in energy conservation.
Force is equal to the negative change in potential energy divided by displacement, highlighting the relationship between force and energy.
Force function can be derived from the potential function by taking the negative derivative, a crucial step in physics problem-solving.
When force and displacement vectors are parallel, potential energy decreases, as seen in objects moving in the direction of a conservative force.
An increase in potential energy occurs when force and displacement vectors are anti-parallel, as in moving against a conservative force.
Gravitational force is a classic example of a conservative force, with potential energy decreasing as an object falls due to parallel force and displacement vectors.
In contrast, lifting an object against gravity increases its potential energy, illustrating the anti-parallel force and displacement vector scenario.
The potential energy of a spring is dependent on its displacement from the equilibrium position, with force being the negative derivative of this potential energy.
The electric force between charges is another example of a conservative force, with potential energy changing based on the direction of movement relative to the force.
The concept of force and potential energy is critical in deriving equations such as the weight force equation from the potential energy equation.
The negative gradient of the potential energy function defines the force vector, a fundamental operation in vector calculus and physics.
Partial derivatives of the potential energy function with respect to each coordinate give the components of the force vector.
The force vector can be visualized as the negative of the gradient of the potential energy function, providing a geometric interpretation of force.
The relationship between force, displacement, and potential energy is encapsulated in the concept that potential energy increases when force and displacement are opposite, and decreases when they are parallel.
Calculating the change in potential energy can be done by evaluating the definite integral of the force function over a given displacement.
The area under the curve of a force-position graph represents the change in potential energy, providing a visual method for calculating energy changes.
Transcripts
Browse More Related Video
Mr Chua, can you explain the F=-dU/dx formula?

High School Physics - Work
(previous version) Introduction to Elastic Potential Energy with Examples
Why is Gravitational Potential Energy Negative? (Gravity, Physics)
Conservation of Energy (Learn to solve any problem)

AP Physics C: Work, Energy, and Power Review (Mechanics)
5.0 / 5 (0 votes)
Thanks for rating: