High School Physics - Electric Potential Difference
TLDRIn the video, Mr. Fullerton discusses the concepts of electric potential, energy, and electric potential difference. He explains how work done on a charge in an electric field results in electric potential energy, symbolized by W. The electric potential difference (V) is the work per unit charge, measured in volts. The video covers the formulas relating work (W), charge (Q), and potential difference (V), and provides examples of calculating charge, work, and potential difference. It also introduces electron volts as a non-SI unit for smaller charges, defining it as the energy needed to move an elementary charge through one volt of potential difference.
Takeaways
- 📌 Electric potential energy is given to a charge when work is done on it in an electric field.
- 🔋 The symbol capital W is commonly used to represent both electric potential energy and work.
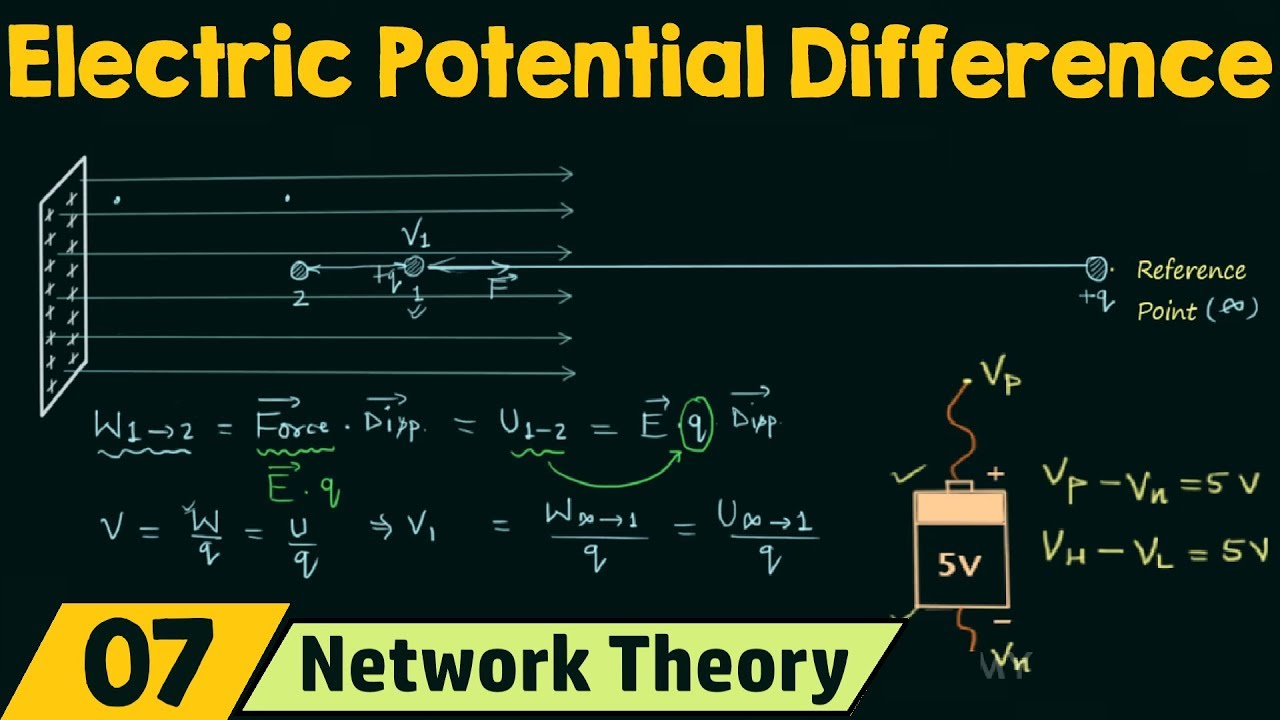
- 🔧 The work done per unit charge in an electric field is known as electric potential difference, symbolized by capital V.
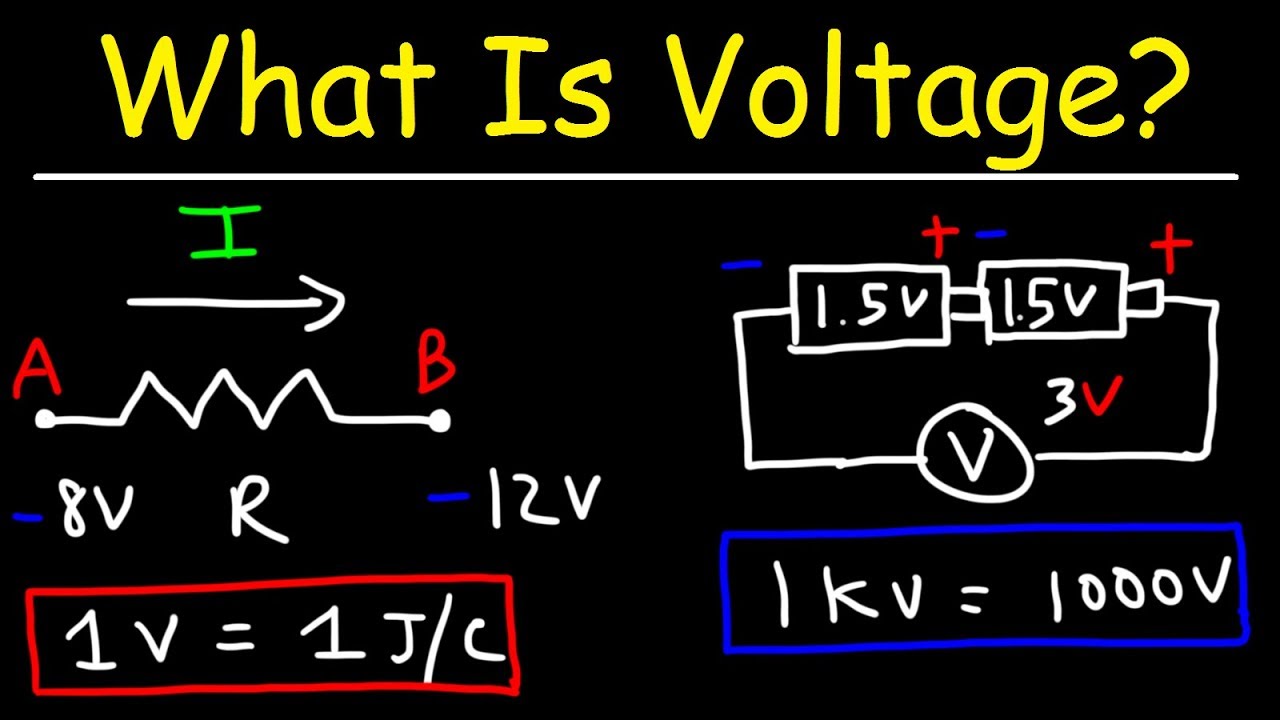
- ⚡ The unit of electric potential difference is伏特 (volts), where 1 volt = 1 Joule per Coulomb of charge.
- 📈 The formula to relate electric potential difference (V) to work (W) and charge (Q) is V = W/Q.
- 🔄 To find the charge that requires a certain amount of work, use the formula Q = W/V.
- 💡 To calculate electrical energy, use the formula W = Q * V.
- 🏋️♂️ When dealing with very small charges, the unit electron volt (eV) is often used instead of joules.
- 👉 One electron volt (eV) is the energy needed to move one elementary charge (the charge on a proton) through a potential difference of one volt.
- 🔎 The relationship between joules and electron volts is 1 eV = 1.6 * 10^-19 joules.
- 📝 When solving problems, it's important to convert between joules and electron volts as needed to maintain consistency with the units used.
Q & A
What is the main topic of the discussion led by Mr. Fullerton?
-The main topic of the discussion is electric potential and electric potential difference, including their definitions, calculations, and the use of electron volts as a unit of energy.
What is the relationship between work done and electric potential energy?
-The work done on an object is equal to the electric potential energy given to it. If an object with electric potential energy does work, the amount of work done is subtracted from its electric potential energy.
What is the symbol commonly used to represent both electric potential energy and work?
-The symbol capital W is commonly used to represent both electric potential energy and work.
How is electric potential difference defined in terms of work and charge?
-The electric potential difference is defined as the work done per unit charge in moving a charge between two points in an electric field.
What are the units of electric potential difference?
-The units of electric potential difference are volts (V), where one volt is equal to one joule per coulomb of charge.
How can you calculate the charge that requires a certain amount of work to move between two points in an electric field?
-You can calculate the charge by dividing the work done (in joules) by the potential difference (in volts). The formula is Q = W / V.
What is the formula to calculate the electrical energy required to move a charge through a potential difference?
-The formula to calculate the electrical energy (W) is W = Q * V, where Q is the charge in coulombs and V is the potential difference in volts.
What is an electron volt, and how is it related to joules?
-An electron volt (eV) is a non-SI unit of energy that represents the amount of energy gained or lost by a single electron moving through a potential difference of one volt. One electron volt is equal to 1.6 * 10^-19 joules.
How can you convert the work done in joules to electron volts?
-To convert work done in joules to electron volts, multiply the work in joules by the conversion factor of 1 eV / (1.6 * 10^-19 J). This simplifies to just multiplying by the number of electron volts since the joules cancel out.
What is the significance of using electron volts when dealing with very small charges?
-Electron volts are a more convenient unit for dealing with very small charges because they are much smaller than joules, allowing for easier calculation and representation of the energy involved in moving small amounts of charge through potential differences.
How can you calculate the electric potential difference if you know the work done and the charge moved?
-You can calculate the electric potential difference by dividing the work done (in joules) by the charge (in coulombs). The formula is V = W / Q.
Outlines
🔋 Introduction to Electric Potential and Energy
This paragraph introduces the concept of electric potential and energy. It defines electric potential as the work done to move a charge within an electric field and relates this to electric potential energy. The paragraph explains that work done on an object gives it energy, which in the context of an electric field, is referred to as electric potential energy. The symbol W is commonly used for both work and electric potential energy. The discussion then transitions into the concept of electric potential difference, denoted by the symbol V, which is the work done per unit charge. The unit of electric potential difference is the volt, and the relationship between work (W), charge (Q), and electric potential difference (V) is established using the formula V = W/Q. The paragraph concludes with examples that apply this formula to calculate charge and work in different scenarios involving potential difference and charge.
🤖 Electron Volts and Smaller Charges
This paragraph delves into the concept of electron volts (eV), a non-SI unit of energy that is particularly useful when dealing with smaller charges. An electron volt is defined as the amount of energy required to move a single elementary charge (the charge of a proton) through a potential difference of one volt. This equates to 1.6 x 10^-19 joules. The paragraph explains that when discussing small charges, it is often more convenient to use electron volts rather than joules, despite the latter being the SI unit of energy. The explanation is supported by examples that demonstrate how to calculate work in electron volts for different charges moved through an electric field. The paragraph emphasizes the process of converting joules to electron volts when necessary and concludes by encouraging further exploration of these concepts through additional resources.
Mindmap
Keywords
💡Electric Potential
💡Electric Potential Difference
💡Work
💡Charge
💡Energy
💡Electron Volts
💡Joule
💡Coulomb
💡Electric Field
💡Potential Energy
💡Displacement
Highlights
Define and calculate electric potential and energy.
Define and calculate electric potential difference.
Use electron volts as a unit of energy for small charges.
Work done on an object is the electric potential energy.
The work done per unit charge is known as the electric potential difference.
Electric potential difference is measured in volts.
The formula for electric potential difference is V = W/Q.
Calculate the charge that requires a certain amount of work to move between two points in an electric field.
Determine the electrical energy required to move a charge through a potential difference.
Solve for the electric potential difference given a certain amount of work and charge.
Understand the concept of an electron volt as a unit of electrical work or energy.
One electron volt is equal to 1.6 x 10^-19 joules.
Use electron volts for smaller charges when joules are not convenient.
Calculate work in electron volts for a given charge and potential difference.
Convert work from joules to electron volts for specific charge values.
Explore the relationship between electric potential difference, electrical potential energy, and electron volts.
Apply the concept of electric potential and potential difference to practical problem-solving scenarios.
Check out aplusphysics.com for more problems, help, or tutorials on electric potential and potential difference.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: