High School Physics - Work
TLDRIn this informative talk, Mr. Fullerton explains the concept of work in physics, emphasizing its relationship with energy transfer and force application. He clarifies that work is done when a force causes displacement, and introduces the formula for calculating work (W = F * D * cos(theta)). Through relatable examples, he illustrates how the direction of the force relative to displacement affects the work done, and demonstrates how graphical analysis can be used to determine work in varied scenarios. The discussion is capped with a practical problem-solving segment, reinforcing the principles of work and energy in physics.
Takeaways
- 📚 Work in physics is defined as the process of moving an object by applying a force.
- 💡 The rate of doing work is known as power output.
- 🔄 When work is done on an object, energy is transferred from one object to another, such as from gravitational potential energy to kinetic energy.
- 🚀 Examples of work include a stuntman accelerating with a jet pack and a child carrying a bag of candy across the yard.
- ⚙️ The formula for calculating work is Work = Force × Displacement, where work is measured in joules and force in Newtons.
- 📐 The force and displacement must be in the same direction for work to be done, or the force must have a component in the direction of displacement.
- 🌐 Work is calculated as W = F × D × cos(θ), where θ is the angle between the force and displacement vectors.
- 🔢 In instances where force is perpendicular to displacement, no work is done, as seen when pushing down on a car stuck on ice.
- 🏋️ Lifting an object involves overcoming the force of gravity, and the work done is equal to the weight of the object times the displacement.
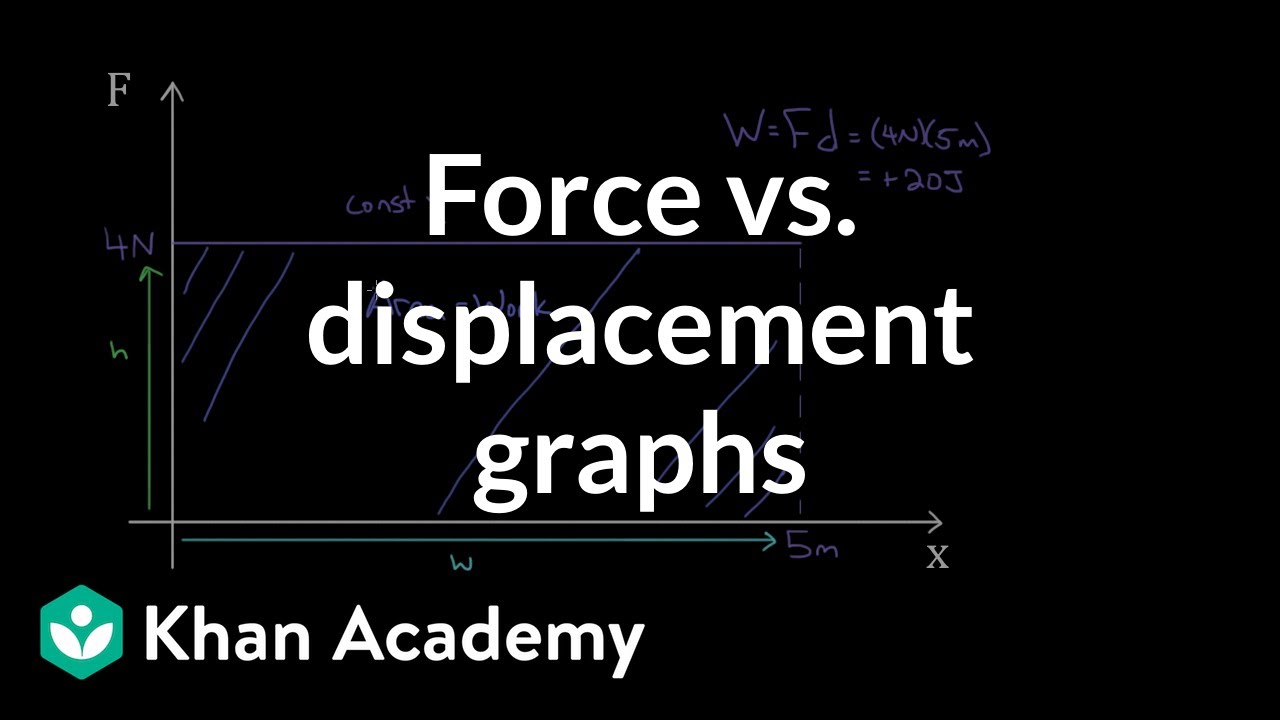
- 🛠️ Work can also be determined graphically by calculating the area under a force versus displacement graph.
- 🎯 Understanding the concept of work and its calculation is crucial for solving physics problems involving energy transfer and force applications.
Q & A
What is the definition of work in the context of physics?
-In physics, work is defined as the process of moving an object by applying a force. It involves the transfer of energy from one object to another and occurs when there is a displacement caused by the force applied.
How is power output related to work?
-Power output is the rate at which work is done. It measures how quickly or slowly work is performed. The more work done in a unit of time, the higher the power output.
What are the two main types of energy transferred when doing work?
-The two main types of energy transferred when doing work are kinetic energy and potential energy. Kinetic energy is given to an object when it is in motion, while potential energy, such as gravitational potential energy, is given when an object is lifted or positioned at a certain height.
What is the formula for calculating work?
-The formula for calculating work is W = F * D * cos(theta), where W is work done in joules, F is the force applied in Newtons, D is the displacement in meters, and theta is the angle between the force and the displacement vector.
Why is it important for the force and displacement to be in the same direction for work to be done?
-For work to be done, the force and displacement must be in the same direction because only the component of the force that is in the direction of the displacement contributes to the work. If they are not aligned, the work calculation must consider the component of the force that is in the direction of displacement.
What happens when a force is applied but there is no displacement?
-If a force is applied but there is no displacement, no work is done. This is because work requires movement of an object due to the force applied. Effort may be expended, but without displacement, there is no transfer of energy in the context of work.
How does the force versus displacement graph represent work?
-The force versus displacement graph represents work as the area under the curve. The shape of the graph, whether it is a rectangle or a triangle, determines the area, which corresponds to the amount of work done. The total work is the sum of the areas of all shapes in the graph.
What is the significance of the cosine term in the work formula?
-The cosine term in the work formula accounts for the angle between the force and the displacement. It determines the component of the force that is effectively contributing to the displacement. The cosine of the angle ranges from -1 to 1, where 1 means the force and displacement are in the same direction, 0 means they are perpendicular, and -1 means they are in opposite directions.
How much work is done in lifting an object against gravity?
-The work done in lifting an object against gravity is equal to the weight of the object (force due to gravity) times the vertical displacement. The weight is calculated as the mass of the object times the acceleration due to gravity (mg), and the displacement is the height the object is lifted.
What is the result of negative work in physics?
-Negative work indicates that the force applied is in the opposite direction of the displacement. This means that the object is moving against the direction of the applied force, and the force is not doing work in the direction of the displacement but rather resisting it.
How can graphical integration of a force-displacement graph help in calculating work?
-Graphical integration of a force-displacement graph helps in calculating work by visually representing the area under the curve, which corresponds to the total work done. This method allows for an easier calculation of work, especially when the force is varying and the relationship between force and displacement is complex.
Outlines
📚 Introduction to Work in Physics
This paragraph introduces the concept of work in physics, defining it as the process of moving an object by applying a force. It distinguishes between doing work and transferring energy, explaining that work is done when a force causes displacement. The speaker, Mr. Fullerton, uses everyday examples such as lifting a phone and throwing it sideways to illustrate how work transfers energy, giving the object potential and kinetic energy respectively. The paragraph also explains the importance of the direction of force relative to displacement in doing work, and introduces the formula for calculating work (W = F * D * cos(theta)), where W is work, F is force, D is displacement, and theta is the angle between the force and displacement.
🔧 Calculating Work with Examples
This paragraph delves into the calculation of work with various examples. It explains how to apply the work formula (W = F * D * cos(theta)) in different scenarios, emphasizing that only the component of force in the direction of displacement counts towards work done. The examples include a salesman pushing a refrigerator, a person pushing a car on ice, pushing a crate up a ramp, and lifting a box against gravity. The paragraph also discusses the concept of negative work, as seen in the crate sliding down the ramp example. Each example illustrates how to calculate the work done by considering the angle between the applied force and the displacement.
📊 Work Done Graphically
This paragraph discusses the graphical representation of work done, using force versus displacement graphs. It explains how to calculate work by finding the area under the graph for different scenarios, such as a block being pulled with a constant force and then the force decreasing. The examples provided include calculating work done when a force gradually tapers off and when a box is wheeled with a varying horizontal force. The paragraph highlights the use of geometric shapes like rectangles and triangles to find the area under the graph, which corresponds to the work done. This approach provides a visual and intuitive understanding of work in physics.
Mindmap
Keywords
💡Work (Physics)
💡Energy Transfer
💡Power Output
💡Force
💡Displacement
💡Joule
💡Cosine (Trigonometry)
💡Newton's Third Law
💡Gravitational Force
💡Kinetic Energy
💡Potential Energy
Highlights
Work in physics is defined as the process of moving an object by applying a force.
Power output is the rate at which work is done.
When work is done on an object, energy is transferred from one object to another.
No work is done if the object does not move, despite applying a force.
A stuntman in a jet pack does work as the jet pack applies a force causing movement.
A girl pushing a stalled car does not do work if the car does not move despite exerting effort.
A child carrying a bag of candy across the yard does not do work if the force applied is perpendicular to the displacement.
The formula for calculating work is Work = Force × Displacement.
The unit of work and energy is the Joule, which is a kilogram meter squared per second squared.
The force component in the direction of displacement is used when the force and displacement are not collinear.
The work done can be found using the formula W = F cos(theta) × D, where theta is the angle between the force and displacement.
An appliance salesman does 400 Joules of work by pushing a refrigerator 2 meters with a force of 200 Newtons.
Pushing down on a car stuck on ice does not do work because the force applied is perpendicular to the car's displacement.
Negative work (-40 Joules) is done when pushing up a ramp but the crate slides down, indicating displacement in the opposite direction.
Lifting an eight-kilogram box two meters does 157 Joules of work, overcoming the gravitational force.
Barry and Sidney do 8,660 Joules of work by pulling a 30-kilogram wagon with a 500-Newton force over 20 meters at a 30-degree angle.
A child applying a 20-Newton force at a 25-degree angle to move a wagon 4 meters does 72.5 Joules of work.
The area under a force versus displacement graph represents the work done by a force.
The total work done moving a box with varying force can be calculated by summing the areas of geometric shapes under the force-displacement graph.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: