AQA A-Level Chemistry - Kw and Bases
TLDRThis video script delves into the concept of pH and its calculation, particularly focusing on the ionic product of water (kW). It explains the dissociation of water and its equilibrium with hydroxide ions, emphasizing the significance of kW over the traditional equilibrium constant (kC). The video clarifies that at 25°C, the pH of water is neutral (pH 7), but this neutral point shifts with temperature. It further demonstrates how to calculate the pH of basic solutions using the relationship between H+, OH- ions, and the constant kW value at a given temperature.
Takeaways
- 🌊 The concept of water dissociation and its equilibrium is fundamental to understanding pH calculations and the ionic product of water (Kw).
- 📈 At room temperature (25°C or 298K), the value of Kw is 1 × 10^-14, which is a constant and does not include water in its expression.
- 🔢 The pH of water is neutral at 7, as determined by the square root of Kw (1 × 10^-7), indicating equal concentrations of H+ and OH- ions.
- 🌡️ The pH of water changes with temperature; at 100°C (373K), the pH is 6.14, which is considered neutral at that temperature.
- 🧪 The ionic product of water (Kw) allows us to calculate the H+ concentration in basic solutions using the formula: H+ = Kw / [OH-].
- 📊 Alkalis, such as sodium and potassium hydroxide, dissociate to produce hydroxide ions (OH-), which can be used to determine pH in basic solutions.
- 📈 The pH scale is not limited to 0-14; it can extend beyond these values, especially in strong bases or acids.
- 🔧 The dissociation of weak acids and bases involves equilibrium with reversible reactions, which is key to understanding their pH calculations.
- 📚 Understanding the relationship between H+, OH-, and Kw is crucial for solving pH-related problems in chemistry.
- 🎓 The concept of Kw and its application in pH calculations is an important topic that can influence exam results.
- 💡 Practice and understanding of the derivation of Kw and its application in finding the pH of solutions are essential for mastering this area of chemistry.
Q & A
What is the primary topic of the video?
-The primary topic of the video is the concept of pH and its calculation, particularly focusing on the use of the ionic product of water (kW) in determining the pH of bases.
How does water dissociation relate to the concept of pH?
-Water dissociation is crucial to the concept of pH because it involves the equilibrium between hydrogen ions (H+) and hydroxide ions (OH-) in water. The equilibrium constant associated with this dissociation is expressed as kW, which is used to calculate the pH of solutions.
What is the significance of the ionic product of water (kW)?
-The ionic product of water (kW) is significant because it represents the product of the concentrations of hydrogen ions and hydroxide ions in water at equilibrium. It is a constant value at a given temperature and is used to find the pH of both acidic and basic solutions.
How does the pH of water change with temperature?
-The pH of water changes with temperature because the dissociation of water is temperature-dependent. As the temperature increases, the dissociation of water increases, resulting in a higher concentration of H+ and OH- ions. For example, at 100 degrees Celsius, the pH of water is 6.14, which is lower than the pH of 7 at room temperature (25 degrees Celsius).
What is the relationship between the pH of a solution and the concentration of hydrogen ions (H+)?
-The pH of a solution is the negative logarithm (base 10) of the concentration of hydrogen ions (H+). A lower pH value indicates a higher concentration of H+ ions, making the solution more acidic, while a higher pH value indicates a lower concentration of H+ ions, making the solution more basic or alkaline.
How can you calculate the pH of a basic solution using the ionic product of water (kW)?
-To calculate the pH of a basic solution using kW, you first determine the concentration of hydroxide ions (OH-) in the solution. Then, you use the relationship kW = [H+][OH-] to find the concentration of hydrogen ions (H+). Finally, you calculate the pH using the formula pH = -log[H+].
What is the value of kW at 298 Kelvin (25 degrees Celsius)?
-At 298 Kelvin (25 degrees Celsius), the value of kW is 1 × 10^-14.
How does the dissociation of alkalis like sodium hydroxide and barium hydroxide affect the pH of the solution?
-Alkalis like sodium hydroxide and barium hydroxide dissociate in water to produce hydroxide ions (OH-). This increases the concentration of OH- ions in the solution, which in turn lowers the concentration of H+ ions, resulting in a higher pH value, indicating a more basic or alkaline solution.
What is the significance of the 1:1 dissociation ratio in calculating the pH of water?
-The 1:1 dissociation ratio of water means that for each molecule of water that dissociates, one hydrogen ion (H+) and one hydroxide ion (OH-) are produced. This ratio is significant because it allows us to equate the concentrations of H+ and OH- ions in water at equilibrium, which is essential for calculating the pH using the ionic product of water (kW).
How does the pH scale extend beyond the traditional range of 0 to 14?
-While the pH scale is traditionally taught to range from 0 (most acidic) to 14 (most basic), it can actually extend beyond these values. A pH less than 0 indicates a solution with a higher concentration of hydrogen ions than a neutral solution, making it superacidic. Conversely, a pH greater than 14 indicates a solution with a higher concentration of hydroxide ions than a neutral solution, making it superbasic.
What is the pH of a 0.5 M solution of sodium hydroxide (NaOH) at 298 K?
-For a 0.5 M solution of NaOH, which dissociates completely into Na+ and OH- ions, the concentration of OH- ions is 0.5 M. Using the ionic product of water (kW) at 298 K, which is 1 × 10^-14, we can calculate the concentration of H+ ions as kW / [OH-] = (1 × 10^-14) / 0.5 = 2 × 10^-15 M. The pH is then calculated as -log[H+], which gives a pH of 13.70.
Outlines
🌊 Introduction to the Concept of pH and the Role of Water
This paragraph introduces the video's focus on pH, particularly the basis of pH calculations using the unit kW. It explains the importance of understanding water's dissociation into H+ and OH- ions, and how this relates to the equilibrium concept. The paragraph emphasizes the use of the equilibrium expression for water dissociation and introduces the term 'Kw', which is the ionic product of water. It also hints at the application of this concept in real-world scenarios, such as calculating the pH of water at different temperatures.
🔢 pH Calculation and the Influence of Temperature
The second paragraph delves into the specifics of pH calculation, emphasizing the relationship between the dissociation constant 'Kw' and the concentrations of H+ and OH- ions. It explains how the pH of water at 298 Kelvin (25°C) is neutral with a pH of 7, but this neutral point changes with temperature. The example given shows that at 373 Kelvin (100°C), the pH of water is 6.14, indicating that the neutral point has shifted. This section provides a clear understanding of how temperature affects the pH scale and the importance of considering this in calculations.
🧪 Application of Kw in Determining pH of Alkaline Solutions
The final paragraph discusses the application of the 'Kw' concept in calculating the pH of alkaline solutions. It explains the dissociation process of bases like sodium hydroxide and how to use the 'Kw' value to find the concentration of H+ ions, ultimately leading to the pH calculation. The paragraph provides examples of finding the pH of different alkaline solutions, highlighting the method and the importance of understanding the one-to-one dissociation ratio. It concludes with a comprehensive understanding of how to apply the concept of 'Kw' in determining the pH of bases.
Mindmap
Keywords
💡kW
💡pH
💡Equilibrium
💡Dissociation
💡Concentration
💡Alkalinity
💡Temperature
💡Reversible reactions
💡Neutral
💡Strong bases
Highlights
The video primarily focuses on the concept of pH and its calculation using the term kW, which is essential for understanding the pH of bases.
Water is shown to dissociate very slightly, forming an equilibrium with its ions, which is a fundamental concept in understanding the pH scale.
The equilibrium expression for water dissociation is introduced, emphasizing the intrinsic relationship between acids, bases, and equilibrium.
kW, the ionic product of water, is a key term that replaces the equilibrium constant kC when water is not included in the expression.
The concentration of water is considered almost constant in the equilibrium expression, which simplifies the calculation of pH.
At room temperature (298 K), the value of kW is 1 x 10^-14, a constant that remains unchanged at a specific temperature.
The pH of water at 298 K is found to be 7, demonstrating that pure water is neutral at room temperature.
The pH of water changes with temperature; at 100 degrees Celsius (373 K), the pH is 6.14, indicating a shift in the neutral point.
The concept of the pH scale is extended to alkaline solutions, where the pH can exceed 7, contrary to the traditional scale.
The calculation of pH for strong alkalis, such as sodium hydroxide, is demonstrated using the kW value and the relationship between H+ and OH- ions.
The pH of a solution can be determined by the ratio of the concentrations of H+ and OH- ions, using the rearranged expression of kW.
The dissociation of bases like potassium hydroxide and barium hydroxide is discussed, emphasizing the one-to-one dissociation and its impact on pH calculation.
The video provides practical examples of calculating the pH of various solutions, reinforcing the application of the theoretical concepts.
The importance of understanding the derivation of kW and its application in finding the pH of water and alkaline solutions is highlighted.
The video concludes with an encouragement for viewers to practice and engage with the content to solidify their understanding of pH and its calculation.
Transcripts
Browse More Related Video

The Ionic Product of Water Kw
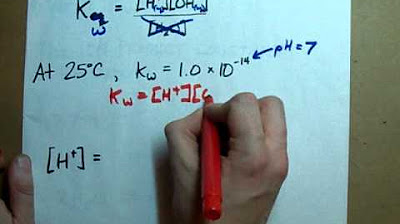
What is Kw (The Ion Product Constant of Water)
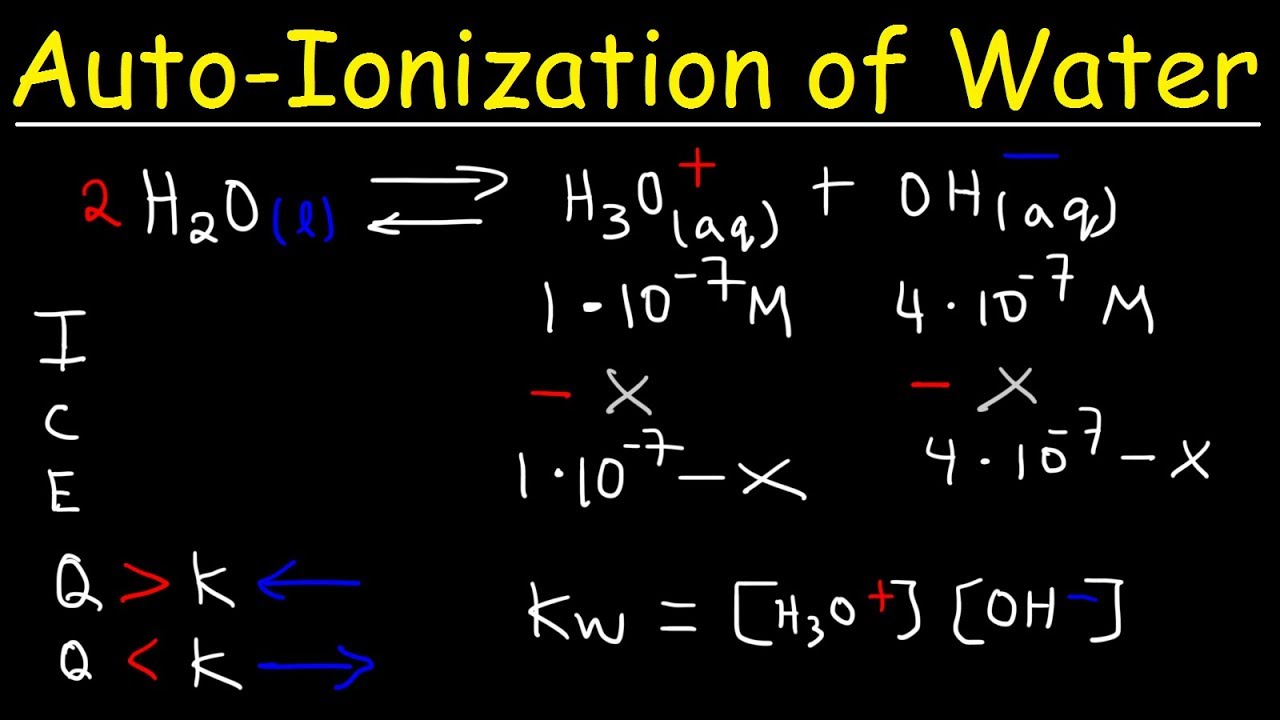
AutoIonization of Water, Ion Product Constant - Kw, Calculating H3O+, OH-, and pH Using Ice Tables
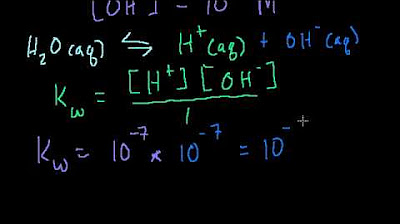
Introduction to pH, pOH, and pKw

pH, pOH of strong acids and bases | Chemistry | Khan Academy
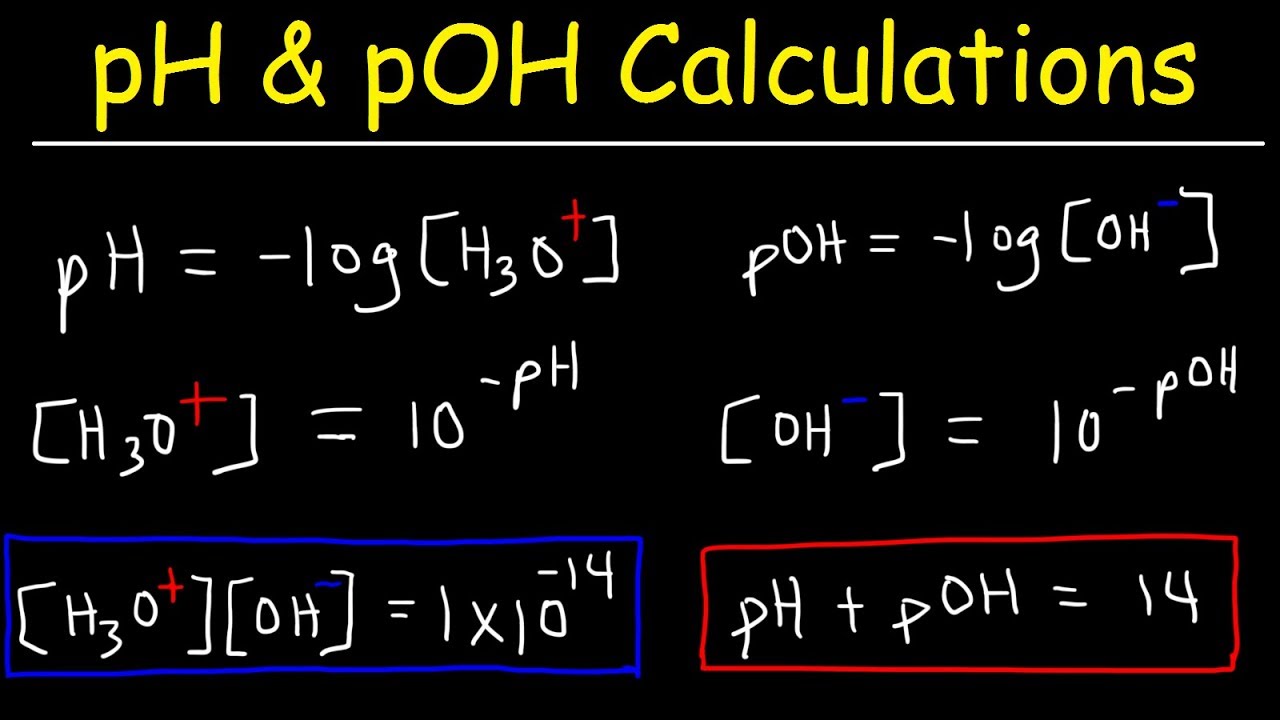
pH, pOH, H3O+, OH-, Kw, Ka, Kb, pKa, and pKb Basic Calculations -Acids and Bases Chemistry Problems
5.0 / 5 (0 votes)
Thanks for rating: