Intuition for second part of fundamental theorem of calculus | AP Calculus AB | Khan Academy
TLDRThis video script delves into the concept of the second fundamental theorem of calculus, illustrating the relationship between the rate of change of position with respect to time (velocity) and the change in position itself. It explains how the area under the velocity curve can be used to determine the exact change in position over a time interval. The script employs the use of Riemann sums and the concept of taking a limit to find the area under the curve, ultimately highlighting the power of calculus in solving real-world problems.
Takeaways
- 📈 The concept of a position function s(t) is introduced as a function of time, represented graphically with a parabola-like shape.
- 🕒 The change in position between two times, a and b, is given by the difference s(b) - s(a).
- 🚀 The derivative of the position function s(t) with respect to time gives the velocity function v(t), which is the rate of change of position.
- 📚 The velocity function v(t) can be graphed separately, showing how the rate of change of position (slope) varies over time.
- 📝 Riemann sums can be used to approximate the change in position over time intervals by dividing the time interval into rectangles and summing their areas.
- 🔍 The area under the velocity function v(t) curve represents the exact change in position between two times a and b.
- 🌟 The Second Fundamental Theorem of Calculus states that the definite integral of the velocity function v(t) dt from a to b equals the change in position s(b) - s(a).
- 📌 The antiderivative of the velocity function v(t), denoted as ∫v(t) dt, is used to find the exact area under the curve and the exact change in position.
- 🔧 The process of finding the exact area under the curve involves evaluating the antiderivative of the velocity function at the endpoints and taking the difference, denoted as F(b) - F(a).
- 🔄 The concept of taking a limit as the number of rectangles approaches infinity (n → ∞) is introduced as a method to find the exact area under the curve, which is the basis of the Riemann integral.
Q & A
What is the primary function of the graph with a horizontal time axis and a vertical y-axis?
-The primary function of the graph is to represent the position of an object as a function of time, denoted as s(t), where the horizontal axis represents time and the vertical axis represents the position of the object.
How is the change in position between two times, time a and time b, calculated?
-The change in position between time a and time b is calculated by finding the difference in the position values at those times, which is s(b) - s(a), where s(b) is the position at time b and s(a) is the position at time a.
What does the derivative of a position function s(t) represent?
-The derivative of a position function s(t), denoted as ds/dt or s'(t), represents the rate of change of position with respect to time, which is the velocity of the object.
How does the graph of the velocity function v(t) relate to the original position function s(t)?
-The graph of the velocity function v(t) is derived from the slope of the tangent line to the position function s(t) graph at any given point. The shape of v(t) reflects how the velocity changes as time progresses.
What is a Riemann sum and how is it used to approximate the change in position?
-A Riemann sum is a method used to approximate the definite integral of a function by dividing the interval between two points into smaller subintervals and summing the areas of rectangles constructed on these subintervals. It is used to approximate the change in position by considering the change in velocity over small time intervals.
How does the area under the velocity curve relate to the change in position?
-The area under the velocity curve represents the total change in position over the given time interval. By calculating the area under the curve, one can find the exact change in position between two times.
What is the Second Fundamental Theorem of Calculus?
-The Second Fundamental Theorem of Calculus states that the definite integral of a function f(t) from a to b can be found by evaluating the antiderivative F(t) of f(t) at b and subtracting the value of F(t) at a, which is F(b) - F(a).
How can one find the exact area under the curve of a velocity function?
-To find the exact area under the curve of a velocity function, one can take the limit of the Riemann sum as the number of rectangles approaches infinity, which is equivalent to calculating the definite integral of the velocity function from a to b.
What is the relationship between the definite integral and the antiderivative?
-The definite integral of a function f(t) over an interval [a, b] is equal to the difference in the values of the antiderivative F(t) evaluated at the endpoints of the interval, which is F(b) - F(a).
How does the concept of Riemann sums and the Second Fundamental Theorem of Calculus connect?
-The concept of Riemann sums provides an approximation method for the definite integral, which is essentially the area under the curve. The Second Fundamental Theorem of Calculus then provides a way to find the exact value of the definite integral by using antiderivatives, connecting the approximation method to a precise mathematical operation.
What is the significance of the Second Fundamental Theorem of Calculus in practical applications?
-The Second Fundamental Theorem of Calculus is significant in practical applications as it allows for the calculation of the exact area under a curve, which can be used to determine quantities such as work, distance, or change in quantity in various scientific and engineering fields.
Outlines
📈 Introduction to Position and Velocity
The paragraph introduces the concept of a position function, s(t), as a function of time, and illustrates it with a graph. It discusses the change in position between two times, a and b, and how this change is represented by the difference in the position function at those times, s(b) - s(a). The concept of the derivative is then introduced, relating it to the rate of change of position with respect to time, which is defined as velocity. The paragraph further explains that the derivative of the position function, s(t), gives the velocity function, v(t), and that the graph of v(t) represents how the rate of change of position varies over time.
📊 Riemann Sums and Approximating Change in Position
This paragraph delves into the use of Riemann sums to approximate the change in position over time intervals. It describes dividing the time interval into smaller rectangles, each representing a small change in position. The area of each rectangle is calculated as the product of the velocity at that time and the change in time, Δt. The sum of these areas approximates the total change in position between times a and b. The paragraph also touches on the concept of the area under the curve of the velocity function and how it relates to the change in position. It explains that calculating the area under the curve of v(t) is equivalent to finding the change in position between a and b.
🧮 The Second Fundamental Theorem of Calculus
The final paragraph introduces the Second Fundamental Theorem of Calculus, which provides a method for evaluating definite integrals and finding the area under a curve. It explains that the definite integral from a to b of the velocity function, v(t), is equal to the change in position from time a to b, which is s(b) - s(a), where s(t) is the antiderivative of v(t). The theorem is presented in a general notation, stating that the area under the curve of a function f(x) between two points a and b can be found by taking the antiderivative of f(x), evaluating it at the endpoints, and subtracting the values. The paragraph concludes by emphasizing the significance of this theorem and its close relation to the First Fundamental Theorem of Calculus.
Mindmap
Keywords
💡function of time
💡derivative
💡position change
💡velocity
💡Riemann sums
💡area under the curve
💡antiderivative
💡second fundamental theorem of calculus
💡limit
💡trapezoidal sum
💡definite integral
Highlights
The introduction of the function s(t) as a position function over time.
Graphing the position function s(t) with a parabola-like shape for simplicity.
Exploring the change in position between two times, a and b.
Deriving the position function to find the rate of change with respect to time, which is velocity.
Describing the derivative ds/dt as the velocity function v(t) and its representation as a function of time.
Graphing the velocity function v(t) to understand the rate of change in position.
Introducing the concept of Riemann sums to approximate the change in position and area under the curve.
Using left Riemann sums with large rectangles for ease of understanding.
Explaining how the area of each rectangle approximates the change in position over time intervals.
Relating the Riemann sum to both the change in position and the area under the curve.
Describing the process of taking the limit as the number of rectangles approaches infinity to find the exact area under the curve.
Expressing the definite integral as the limit of the Riemann sum, which equals the exact change in position.
Articulating the second fundamental theorem of calculus, which connects the definite integral to the antiderivative of the function.
Explaining that the definite integral from a to b of v(t) dt equals the change in position s(b) - s(a), where s(t) is the antiderivative of v(t).
Presenting the general notation for the second fundamental theorem of calculus and its application to finding the area under a curve.
Discussing the importance of the second fundamental theorem of calculus in evaluating definite integrals and its relation to the first fundamental theorem.
The practical application of the second fundamental theorem in calculating the exact area under the curve by evaluating the antiderivative at the endpoints.
The anticipation of applying the second fundamental theorem in future videos.
Transcripts
Browse More Related Video

Velocity Time Graphs, Acceleration & Position Time Graphs - Physics
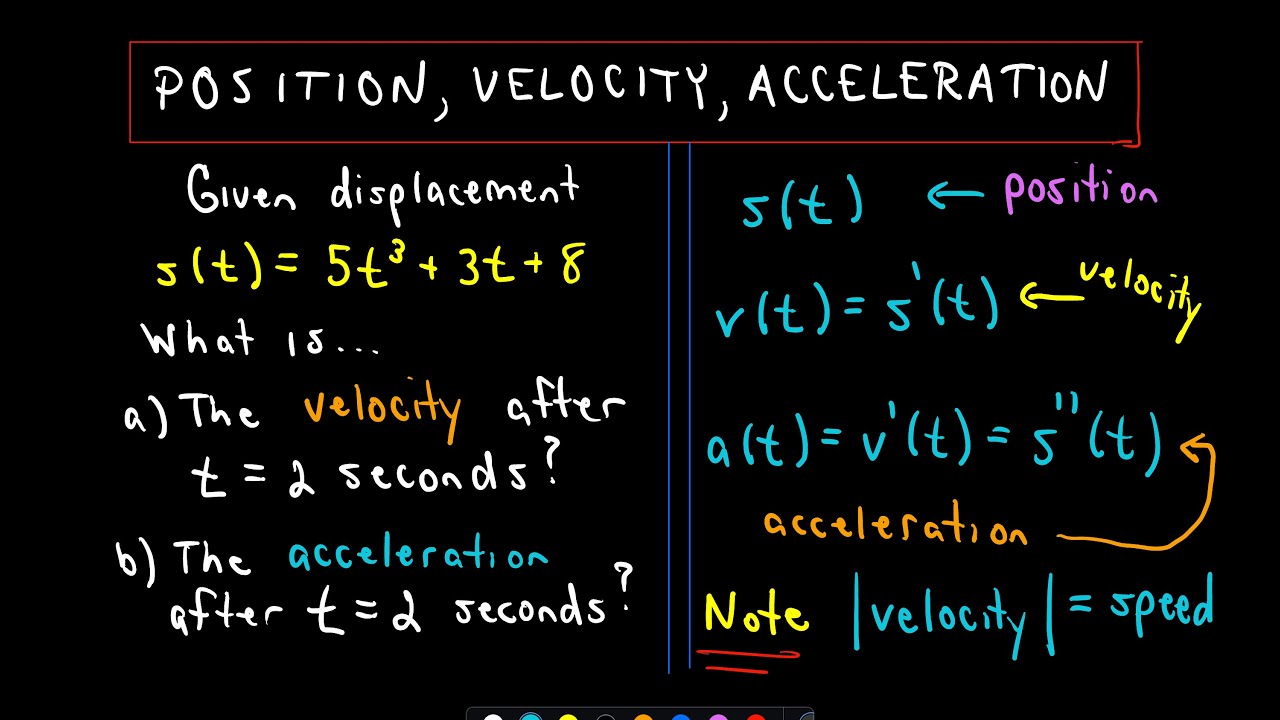
Position, Velocity, Acceleration using Derivatives
Motion Graphs - AP Physics 1: Kinematics Review Supplement
Fundamental theorem of calculus (Part 1) | AP Calculus AB | Khan Academy
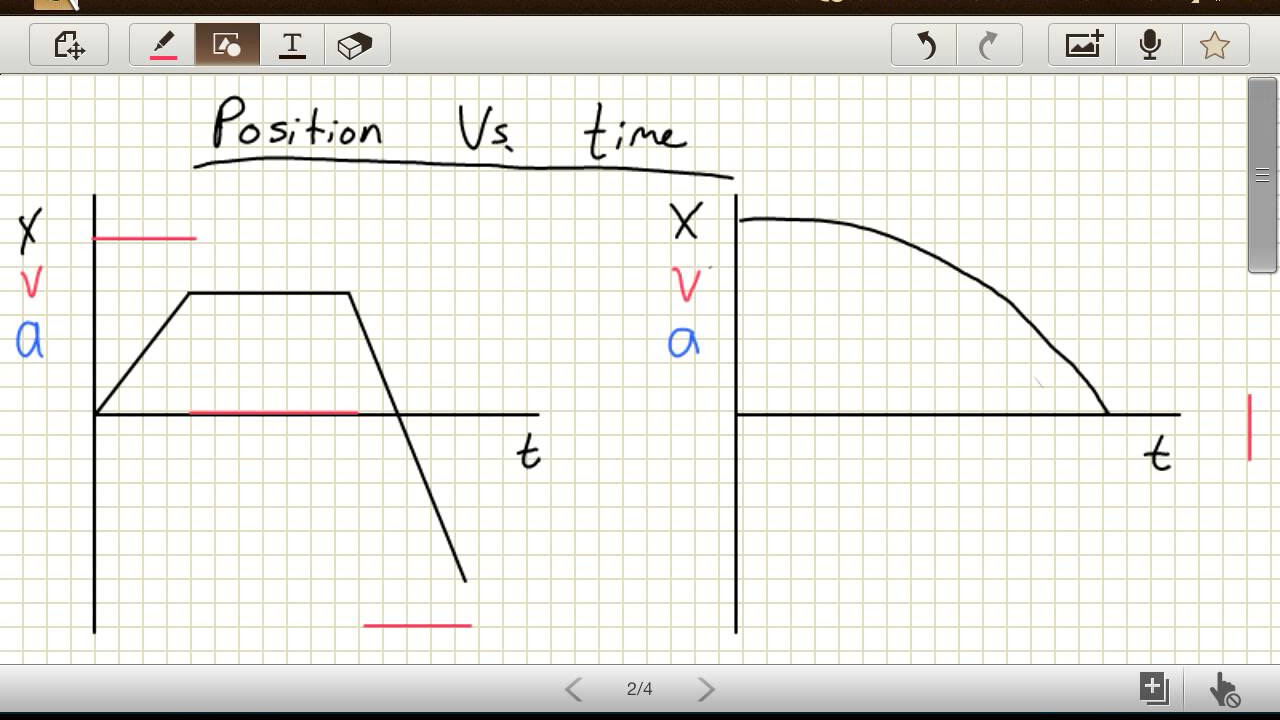
Graphing Motion

An Intuitive Understanding of the Fundamental Theorem of Calculus
5.0 / 5 (0 votes)
Thanks for rating: