Lesson 7 - Integration By Partial Fractions (Calculus 2 Tutor)
TLDRThis advanced calculus tutorial focuses on partial fraction expansion, a technique for integrating rational expressions that many find tedious due to its algebraic complexity and potential for errors. The instructor emphasizes the importance of taking one step at a time and checking each step to minimize mistakes. The video aims to demonstrate how partial fraction expansion can simplify the integration process, transforming complex integrals into more manageable forms that can be solved through natural logarithms and substitution methods. An example is given to illustrate the technique's effectiveness and to show how it can be used to combine fractions under a common denominator.
Takeaways
- 📚 The video is about teaching partial fraction expansion, a technique in calculus for integration.
- 😖 Many people find partial fraction expansion tedious and error-prone due to the algebra involved.
- 👨🏫 The instructor emphasizes the importance of taking one step at a time and checking each line for mistakes.
- 🔍 The goal of partial fraction expansion is to simplify integrals to make them easier to solve.
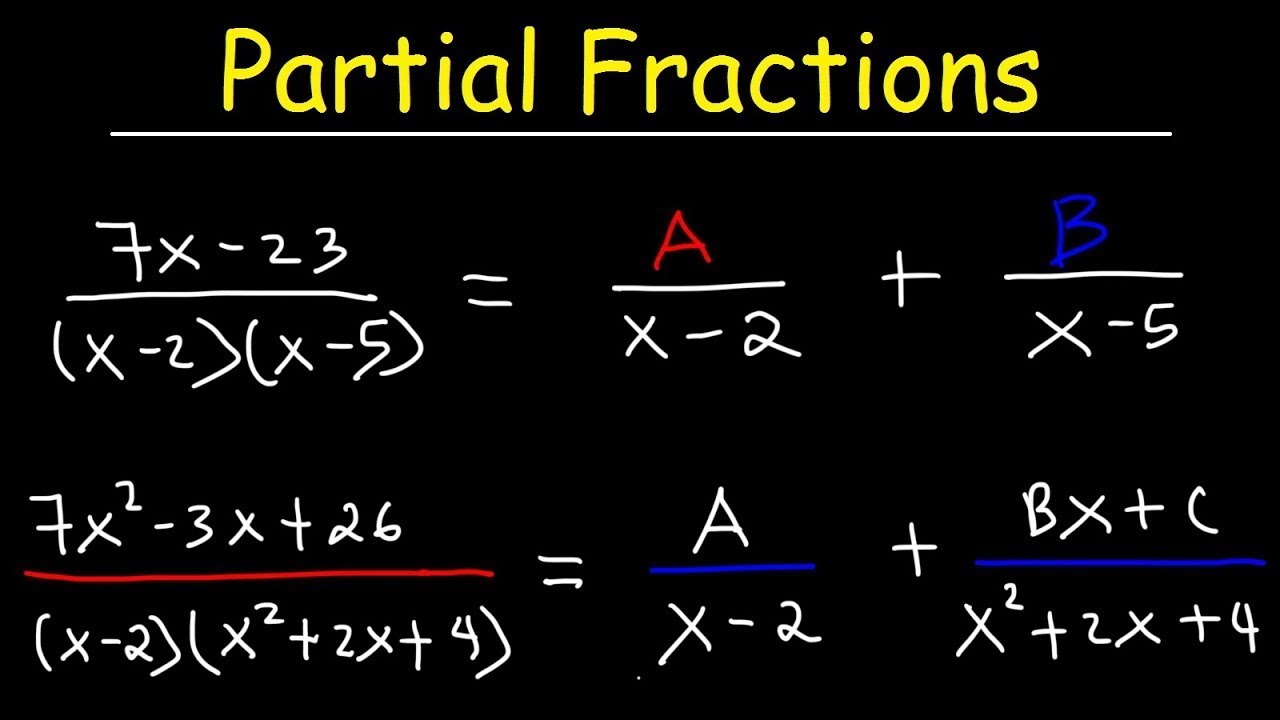
- 🌟 The example given is the integral of (3x + 1) over (x^2 + x), which cannot be easily solved with substitution.
- 🪄 The instructor suggests that integrals can be 'magically' transformed into simpler forms using partial fractions.
- 📈 The video aims to show how to break down complex rational expressions into partial fractions systematically.
- 📝 The process involves finding a way to express a rational function as a sum of simpler fractions.
- 🔢 An example is provided to demonstrate how to combine fractions with a common denominator by multiplying by appropriate factors.
- 📚 The technique is crucial for integrating functions that are rational expressions with polynomials in the numerator and denominator.
- 💡 The video is meant to convince viewers of the power and utility of partial fraction expansion in calculus.
Q & A
What is the main topic of this tutorial section?
-The main topic of this tutorial section is partial fraction expansion, a technique used in calculus for integrating rational expressions.
Why do many people find the section on partial fraction expansion tedious?
-Many people find the section on partial fraction expansion tedious because it involves a lot of algebra and multiple steps, which increases the chance for errors, especially sign errors.
What is the speaker's advice for dealing with the complexity of partial fraction expansion?
-The speaker advises to take math one step at a time, checking every line before moving on to the next to ensure everything is correct, and to practice regularly as everyone makes mistakes.
Can you provide an example of an integral that might be simplified using partial fraction expansion?
-An example given is the integral of (3x + 1) over (x^2 + x) dx, which does not lend itself to simple substitution and requires partial fraction expansion to simplify.
What is the purpose of using partial fraction expansion in integration?
-The purpose of using partial fraction expansion in integration is to break down a complex rational expression into simpler fractions that can be more easily integrated.
How does the speaker illustrate the potential simplification of an integral using partial fraction expansion?
-The speaker illustrates by hypothesizing that the integral of (3x + 1) over (x^2 + x) could be transformed into the sum of two simpler integrals, one involving a natural logarithm and the other a simple substitution.
What is the significance of finding a common denominator in the process of adding fractions, as shown in the script?
-Finding a common denominator is significant because it allows for the addition of fractions by ensuring that each term is over the same base, making it possible to combine them into a single fraction.
What is a 'conversion factor' mentioned in the script, and how is it used?
-A 'conversion factor' is a term used to create a common denominator when adding fractions. In the script, it is used to multiply the numerator and denominator of each fraction by appropriate terms to facilitate the addition.
How does the process of multiplying by 1 not change the value of a fraction, as explained in the script?
-Multiplying a fraction by 1 does not change its value because multiplying by 1 is the identity operation for multiplication; it leaves the original value unchanged.
What is the final goal of the process described in the script for adding fractions?
-The final goal of the process is to combine the fractions into a single fraction with a common denominator, which simplifies the expression and makes it easier to work with.
Outlines
📚 Introduction to Partial Fractions Expansion
The script begins with an introduction to the topic of partial fractions expansion, a technique used in calculus for integrating rational expressions. The speaker acknowledges the complexity and tediousness of this section, emphasizing the importance of algebra and the potential for errors. They advise taking one step at a time and checking each line to avoid mistakes. The concept of partial fractions is introduced as a method to simplify integrals, with the speaker promising to demonstrate its utility through an example involving the integral of (3x + 1) over (x^2 + x).
Mindmap
Keywords
💡Partial Fractions Expansion
💡Integration
💡Algebra
💡Error
💡Practice
💡Rational Expression
💡Substitution
💡Natural Logarithm
💡Trigonometric Substitution
💡Common Denominator
Highlights
Introduction to partial fractions expansion in advanced calculus.
Partial fractions expansion is often disliked due to its tedious nature and algebraic complexity.
The importance of simplifying integrals through partial fractions to solve them.
Advice on taking one step at a time and checking each line to avoid errors.
Example of an integral that cannot be easily solved with substitution.
Demonstration of transforming an integral into a simpler form using partial fractions.
Breaking down an integral into a sum of two integrable functions.
Explanation of how to integrate a simplified expression using natural logarithm and substitution.
The challenge of converting rational expressions into partial fraction expansions systematically.
The significance of finding a method to change rational functions into partial fractions.
Illustration of adding fractions by forming a common denominator.
Technique of multiplying by a conversion factor to facilitate common denominator formation.
The concept of multiplying by 1 to change the form without altering the value.
Final step of combining terms over a common denominator for addition.
Emphasis on the practical applications of partial fractions in calculus.
Encouragement for practice and acknowledging that everyone makes mistakes.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: