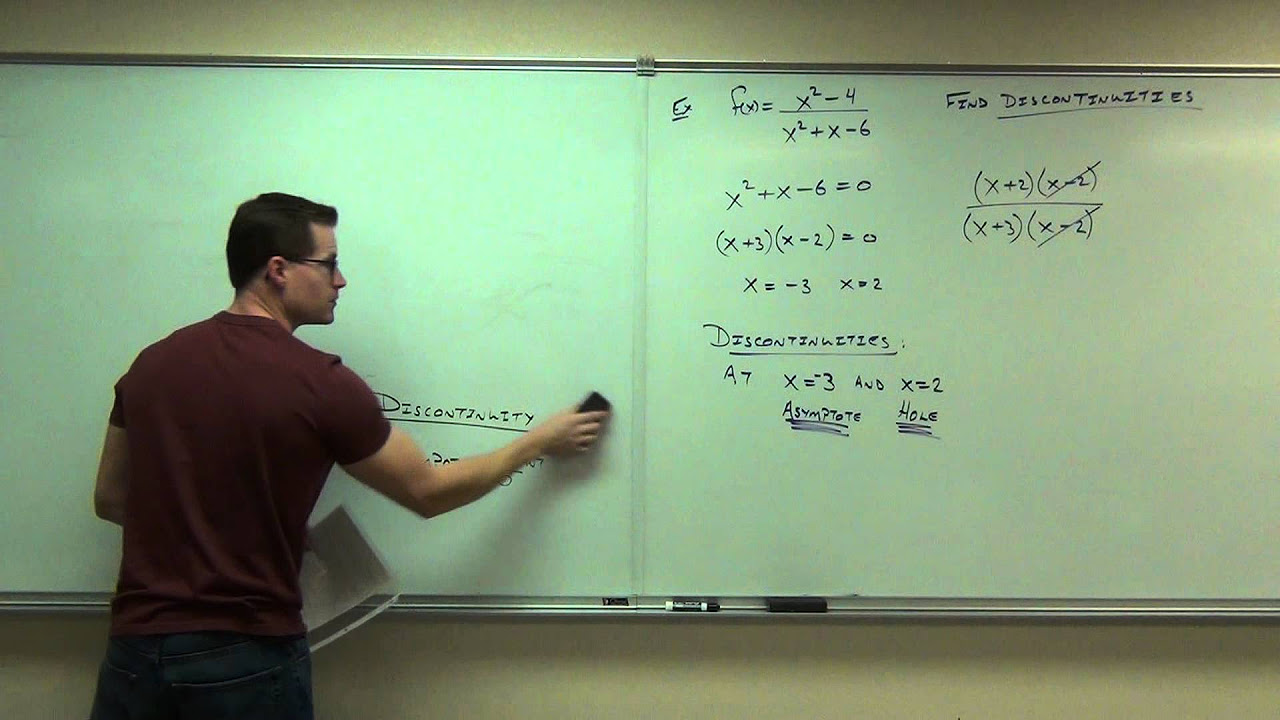Types of discontinuities | Limits and continuity | AP Calculus AB | Khan Academy
TLDRThis video tutorial delves into the various types of discontinuities in mathematical functions, linking these concepts to the understanding of two-sided and one-sided limits. It begins by revisiting point or removable discontinuities, showcasing how a function can be discontinuous at a specific point but potentially corrected. The tutorial further explores jump discontinuities, characterized by abrupt changes in function values, and asymptotic discontinuities, where the function approaches a vertical asymptote. Through graphical examples and definitions of continuity and limits, the instructor demystifies how these discontinuities relate to the foundational principle that a function is continuous if its limit as x approaches c equals the function's value at c.
Takeaways
- 📌 Discontinuities can be categorized and understood in relation to two-sided and one-sided limits.
- 📝 A removable discontinuity occurs at a point where the function is undefined but could be redefined to make it continuous.
- 🔍 For a function to be continuous at a point, the limit from both sides must equal the function's value at that point.
- 🚫 In the case of a removable discontinuity, the two-sided limit exists but does not match the function's value.
- ✏️ Jump discontinuities are characterized by a jump or gap in the graph that requires lifting the pencil while tracing.
- 🔄 Left and right limits exist in a jump discontinuity but are not equal, meaning the two-sided limit does not exist.
- 📈 Asymptotic discontinuities involve a vertical asymptote where the function approaches infinity on either side of the point of interest.
- 🌐 At an asymptotic discontinuity, both the left and right limits are unbounded, indicating they do not exist.
- 📊 The existence of a two-sided limit is a key requirement for a function to be considered continuous at a point.
- 🔧 Understanding discontinuities is crucial for analyzing the behavior of functions and their continuity properties.
Q & A
What is a point or removable discontinuity?
-A point or removable discontinuity occurs when there is a gap at a certain point on the graph of a function, but the function can be redefined at that point to make it continuous. This type of discontinuity is exemplified by a curve that resembles y = x^2 except at a specific point, such as x = 3, where there's a gap, and the function is defined differently at that point.
How does a point or removable discontinuity relate to the definition of continuity?
-For a function to be continuous at a point x = c, the limit of f(x) as x approaches c must equal the actual value of the function at x = c. A point or removable discontinuity fails this condition because, while the two-sided limit exists, it does not match the function's value at the discontinuity point.
What is a jump discontinuity?
-A jump discontinuity occurs when there is a sudden change or 'jump' in the function's value, which prevents the graph from being drawn continuously without lifting the pencil. This type of discontinuity is characterized by having left and right-handed limits that exist but are not equal, indicating a visual jump in the graph.
Why does a jump discontinuity fail the continuity test?
-A jump discontinuity fails the continuity test because, although one-sided limits exist, they approach different values. This means the two-sided limit does not exist, and thus, it cannot equal the value of the function at that point, violating the definition of continuity.
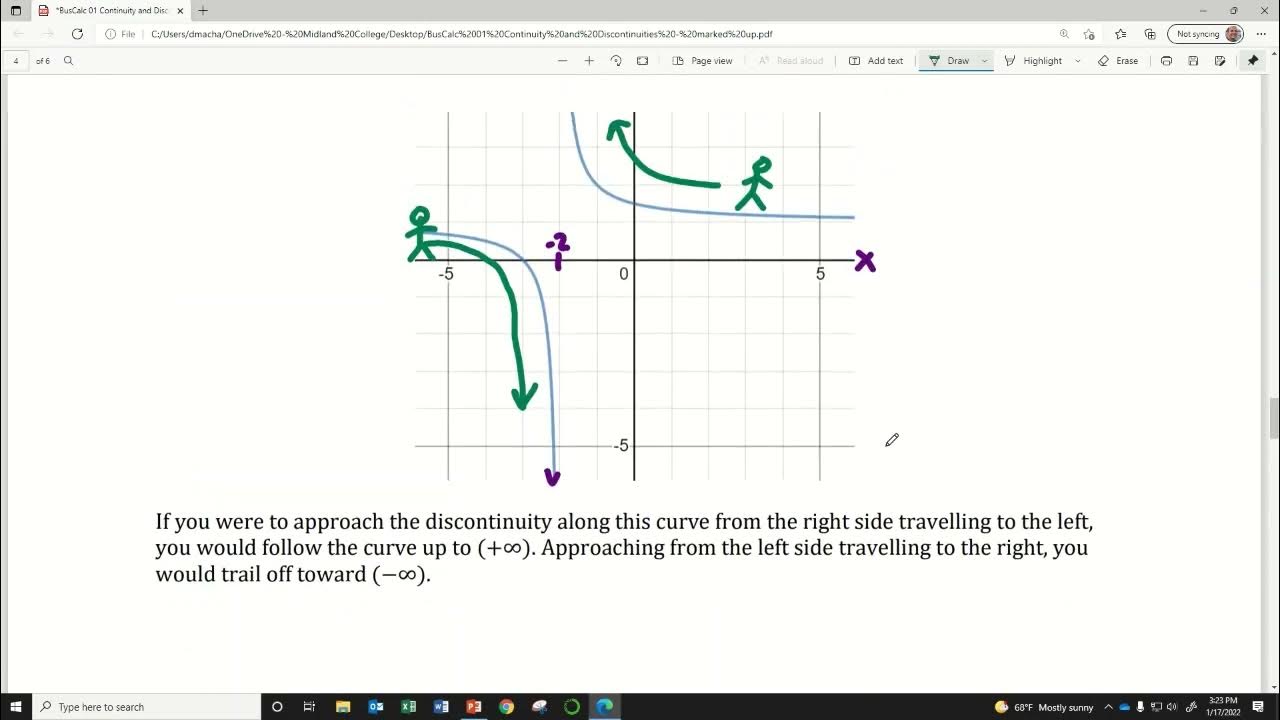
What is an asymptotic discontinuity?
-An asymptotic discontinuity occurs near a vertical asymptote, where the graph of the function becomes unbounded as it approaches a certain x-value from either side. This type of discontinuity is characterized by the limits being unbounded or going to infinity, meaning they don't exist in a conventional sense.
How does an asymptotic discontinuity relate to limits?
-In the case of an asymptotic discontinuity, both the left and right-handed limits as x approaches a certain point are unbounded, indicating they effectively don't exist. Since these limits don't exist, the condition for continuity, which requires the limit to equal the function's value at the point, cannot be met.
What conditions must be met for a function to be considered continuous at a point?
-A function is considered continuous at a point x = c if and only if the limit of f(x) as x approaches c equals the actual value of the function at x = c. This means the function must be defined at c, and there must be no jumps or gaps in the graph at that point.
How can you visually identify a point or removable discontinuity on a graph?
-Visually, a point or removable discontinuity can be identified by a gap at a certain point on the graph where the function is otherwise continuous. This gap indicates the function's value differs from what the continuous curve would suggest at that specific point.
What is the significance of one-sided limits in determining discontinuity?
-One-sided limits are significant in determining discontinuity because they help identify jump discontinuities. If the left and right-handed limits as x approaches a certain point differ, it indicates a jump discontinuity since the graph makes a 'jump' from one value to another.
Can a function with an asymptotic discontinuity be made continuous?
-No, a function with an asymptotic discontinuity cannot be made continuous at the point of discontinuity because the function becomes unbounded or goes to infinity as it approaches the vertical asymptote. This inherent characteristic of the graph prevents continuity at that point.
Outlines
📚 Understanding Discontinuities and Continuity
The paragraph begins with an introduction to the topic of discontinuities and how they relate to the concept of continuity in mathematics. The instructor explains different types of discontinuities using examples from algebra and precalculus, focusing on point or removable discontinuities and jump discontinuities. The explanation includes a review of the definition of continuity, which requires that the limit as x approaches a certain value equals the function's value at that point. The paragraph also discusses scenarios where the function is undefined at a point, leading to discontinuity despite the existence of a two-sided limit.
📉 Asymptotic Discontinuities and Their Characteristics
This paragraph delves into the concept of asymptotic discontinuities, specifically focusing on vertical asymptotes as an example. The instructor uses the vertical asymptote at x equals two to illustrate how the function becomes unbounded as it approaches the asymptote from either side. The discussion emphasizes that both the left and right limits are unbounded, meaning they do not exist, which leads to discontinuity. The explanation clarifies that despite the function's behavior being visually apparent, mathematically it is described as unbounded rather than approaching negative or positive infinity. The paragraph concludes by categorizing the asymptotic discontinuity as a type of discontinuity that arises from the non-existence of certain limits.
Mindmap
Keywords
💡discontinuities
💡two-sided limits
💡one-sided limits
💡removable discontinuity
💡jump discontinuity
💡asymptotic discontinuity
💡vertical asymptote
💡continuity
💡limit
💡graph
💡precalculus
Highlights
Introduction to the video on discontinuities in algebra and precalculus, emphasizing the importance of understanding two-sided and one-sided limits.
Review of discontinuity classification and the example of a curve resembling y = x squared with a point or removable discontinuity at x equals three.
Explanation of why a point or removable discontinuity occurs, based on the definition of continuity and the discrepancy between the two-sided limit and the actual value of the function.
Introduction to the jump discontinuity with an example where the function graph requires lifting the pencil at x equals two, indicating intuitiveness of discontinuity.
Discussion on how jump discontinuities relate to limits, illustrating the difference between left and right-handed limits and their impact on two-sided limits.
Explanation of asymptotic discontinuity using an example with a vertical asymptote at x equals two, demonstrating unbounded behavior.
Highlighting the difference between point or removable discontinuities and jump discontinuities in terms of limit existence and function value equality.
Clarification that both left and right-handed limits are unbounded in the case of asymptotic discontinuity, making these limits officially non-existent.
Illustration of how discontinuities are classified into point or removable, jump, and asymptotic discontinuities, each with unique characteristics.
Explanation of the criteria for a function to be considered continuous and how discontinuities fail to meet these criteria.
Discussion on the practical implication of understanding discontinuities in the context of algebra and precalculus, including their relation to limits.
Explanation of how discontinuities impact the graph of a function, specifically how they affect the drawing of the graph without lifting the pencil.
Detailed analysis of a graph example that combines different types of discontinuities to illustrate their respective characteristics.
Summary of how different discontinuities relate to the fundamental definition of limits and continuity in mathematical analysis.
Final remarks on the importance of being able to identify and understand the different types of discontinuities in the study of calculus.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: