Limits by factoring | Limits and continuity | AP Calculus AB | Khan Academy
TLDRThe video script discusses the process of finding the limit of a function as x approaches a certain value, using f(x) = (x^2 + x - 6) / (x - 2) as an example. It highlights the importance of not simply substituting the value when the function is undefined, such as at x=2 in this case. Instead, the script suggests factoring and simplifying the function where possible, leading to a new expression, f(x) = x + 3, for all x not equal to 2. Through both graphical and numerical methods, the script demonstrates that the limit of f(x) as x approaches 2 is 5, providing a clear and engaging explanation of the concept.
Takeaways
- 📚 The function f(x) is defined as (x^2 + x - 6) / (x - 2) and we're interested in its limit as x approaches 2.
- 🔍 The first approach to find the limit is to evaluate f(2), but this method doesn't always give the correct limit.
- 🚫 Directly evaluating f(2) results in an undefined function since the denominator becomes zero.
- 📈 Simplifying the function by factoring the numerator, we get (x + 3)(x - 2) / (x - 2), which simplifies to x + 3 for all x except x = 2.
- 📊 The graph of the function is not a straight line, and it is undefined at x = 2, creating a hole at that point.
- 🌐 Graphically, as x approaches 2 from either side, the function values seem to approach the same value, which can be found by evaluating x + 3 when x is 2, yielding 5.
- 🔢 Numerically, by substituting values closer to 2 into the simplified function, we also find that the limit tends to approach 5.
- 💡 The limit of f(x) as x approaches 2 can be found both graphically and numerically, and in this case, both methods indicate the limit is 5.
- 🎓 Understanding the concept of limits is crucial in calculus, and this example demonstrates the process of finding a limit when the function is not directly defined at the point of interest.
- 📝 This script serves as an educational example of how to handle limits at points of discontinuity in a function.
Q & A
What is the given function f(x) in the script?
-The given function f(x) is (x^2 + x - 6) / (x - 2).
Why can't we directly substitute x=2 into the function f(x)?
-We cannot directly substitute x=2 into the function because it results in a division by zero, which is undefined.
How does the script suggest to find the limit of f(x) as x approaches 2?
-The script suggests to first simplify the function and then evaluate the limit both graphically and numerically as x gets closer to 2 from both directions.
What is the simplified form of the function f(x)?
-The simplified form of the function f(x) is x + 3 for all x except x = 2.
What is the y-intercept of the graph of the simplified function?
-The y-intercept of the graph is 3, as when x is 0, f(x) becomes 3.
What is the slope of the line in the graph of the simplified function?
-The slope of the line in the graph is 1, as the simplified function is f(x) = x + 3, which represents a linear function with a slope of 1.
What is the numerical approach used in the script to find the limit as x approaches 2?
-The numerical approach used in the script is to evaluate the function with values of x very close to 2 from both directions (less than and greater than 2) and observe that the function values approach 5.
What does the graph of the function look like?
-The graph of the function is a straight line with a slope of 1 and a y-intercept at 3, except there is a hole or discontinuity at x = 2 where the function is undefined.
What is the limit of f(x) as x approaches 2, according to the script?
-The limit of f(x) as x approaches 2 is 5, as both numerical and graphical approaches indicate that the function values approach this number from both directions around 2.
How does the script demonstrate the concept of a limit?
-The script demonstrates the concept of a limit by showing how the function values approach a specific number (5 in this case) as the input (x) gets arbitrarily close to a certain value (2 in this case) from both the positive and negative directions.
What is the significance of the simplified form of the function in understanding the limit?
-The simplified form of the function allows us to visualize the function as a straight line on a graph, which makes it easier to understand and predict the behavior of the function, especially around the point where the original function was undefined. This simplification helps in identifying the limit as x approaches 2.
Outlines
📚 Understanding the Function and its Limit
This paragraph introduces a function, f(x) = (x^2 + x - 6) / (x - 2), and explores the limit of this function as x approaches 2. Initially, it suggests evaluating the function at x = 2 directly, but this results in an undefined value due to division by zero. To gain insight, the expression is factored and rewritten as x + 3 for all x except x = 2. This simplification helps in graphing the function, revealing it is not a straight line and has a y-intercept at 3 with a slope of 1, except at x = 2 where it is undefined. The discussion then focuses on determining the limit visually and numerically, suggesting that as x nears 2 from either side, the function approaches the value 5.
🔢 Numerical Approach to Finding the Limit
This paragraph delves into a numerical approach to confirm the limit of the function as x approaches 2. It starts by testing values very close to 2, such as 1.9999, and observes that the function's output gets closer to 5 as the input values get closer to 2. The paragraph concludes that, whether analyzed graphically or numerically, the limit of the function is equal to 5, providing a clear and comprehensive understanding of the function's behavior at x = 2.
Mindmap
Keywords
💡limit
💡function
💡undefined
💡factor
💡discontinuity
💡graph
💡slope
💡y-intercept
💡algebraic manipulation
💡numerical method
💡approach
Highlights
The function f(x) is defined as x squared plus x minus 6, over x minus 2.
The limit of f(x) as x approaches 2 is the main focus of the analysis.
Directly evaluating f(2) results in an undefined function due to a zero in the denominator.
The expression can be simplified and factored to cancel out the (x - 2) term.
The simplified function is f(x) = x + 3 for all x except x equals 2, where it is undefined.
The function can be graphed by considering the undefined point and the behavior of the function around it.
The graph of the function is not a straight line, and it has a break at x equals 2.
The y-intercept of the graph is at 3, and the slope is 1 for all x except x equals 2.
The limit of f(x) as x approaches 2 can be visually estimated by observing the graph.
The numerical approach to finding the limit involves calculating f(x) with values very close to 2.
Both graphical and numerical methods indicate that the limit approaches a value of 5 as x gets closer to 2.
The function's behavior when approaching 2 from both directions consistently approaches the value 5.
The limit of f(x) as x approaches 2 is equal to 5, which is the value of x plus 3 when x is set to 2.
The analysis demonstrates the importance of understanding the behavior of functions near undefined points.
This example showcases the application of algebraic simplification and graphical interpretation in evaluating limits.
Transcripts
Browse More Related Video

Defining a function at a point to make it continuous | Limits | Differential Calculus | Khan Academy
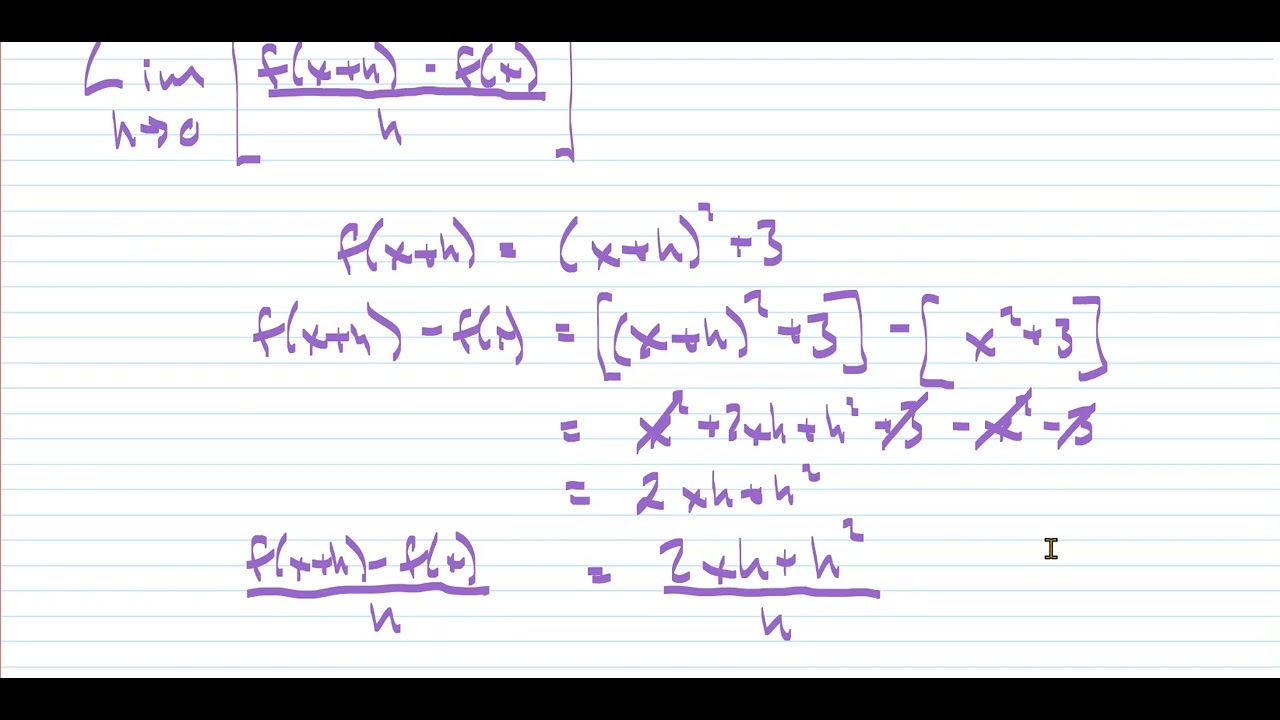
Finding Derivative Using Limit Definition
The Squeeze Theorem for Limits, Example 1

Fancy algebra to find a limit and make a function continuous | Differential Calculus | Khan Academy
2019 AP Calculus AB FRQ #6

Introduction to The Limit
5.0 / 5 (0 votes)
Thanks for rating: