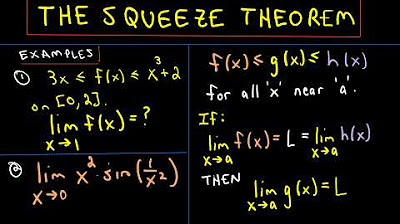2019 AP Calculus AB FRQ #6
TLDRThe video transcript discusses AP Calculus, focusing on response question number six from 2019. It introduces functions F, G, and H, which are twice differentiable, and establishes that G(2) and H(2) are equal. The line y = (4/3)x - 2 is tangent to both F and H at x = 2, leading to the calculation of H'(2) as the slope of the tangent line. The function a(x) = 3x^3 * H(x) is defined, and its derivative a'(x) is found using the product rule. The video then explores the use of L'Hôpital's rule to evaluate the limit as x approaches 2 of H(x), determining F(2) and F'(2). It concludes by discussing the continuity of a function K, which must satisfy certain inequalities and equal H(2) due to the squeeze theorem. The presenter corrects a minor mistake regarding the value of K(2), confirming it as 4.
Takeaways
- 📚 The video discusses AP Calculus, specifically focusing on response question number six from 2019.
- 🔍 Corrections and additional resources, such as PDF solutions, are provided in the video description for further clarification.
- 🎓 Functions F, G, and H are twice differentiable, with G(2) and H(2) being equal, and a tangent line to both their graphs at x=2.
- 📈 The slope of the tangent line at x=2 is 2/3, which is also the value of H'(2), the derivative of H at x=2.
- ✍️ The function a(x) is defined as 3x^3 * H(x), and its derivative a'(x) is found using the product rule.
- 🧮 To find a'(2), the values of H(2) and H'(2) are substituted into the expression for a'(x), resulting in a calculation.
- 🤔 The limit of H(x) as x approaches 2 is evaluated using L'Hôpital's rule, which requires an indeterminate form of 0/0.
- 🔑 It is determined that F(2) must equal 1 for L'Hôpital's rule to be applicable, leading to the calculation of F'(2).
- 🧵 The continuity of the function K is discussed, with K being bounded by G(x) and H(x), and K(2) is found to be 4.
- 📉 G(x) is stated to be less than or equal to H(x) for x between 1 and 3, which is used to justify the value of K(2).
- 📝 The Squeeze Theorem is mentioned in the context of proving the continuity of K at x=2, using the values of H(2) and G(2).
- 👋 The video concludes with an invitation to join the next video, indicating a series of educational content.
Q & A
What is the subject of the video being discussed?
-The subject of the video is AP Calculus, specifically focusing on response question number six from the 2019 exam.
What are the conditions given for functions F, G, and H?
-Functions F, G, and H are twice differentiable functions with G(2) equal to H(2) equal to 4.
What is the equation of the line that is tangent to both graphs of F and H at x equals 2?
-The equation of the tangent line is y = (4/3)x - 2.
What is the slope of the tangent line at x equals 2?
-The slope of the tangent line at x equals 2 is 4/3.
How is the function a(x) defined in the script?
-The function a(x) is defined as a(x) = 3x^3 * H(x).
What is the expression for a'(x) using the product rule?
-The expression for a'(x) is a'(x) = 9x^2 * H(x) + 3x^3 * H'(x).
What is the value of a'(2) in terms of H(2) and H'(2)?
-a'(2) is equal to 9 * (2^2) * H(2) + 3 * (2^3) * H'(2), which simplifies to 36 * H(2) + 24 * (2/3) * H'(2), resulting in 144 + 16 = 160.
What is required to use L'Hôpital's rule in the context of the limit as x approaches 2 of H(x)?
-To use L'Hôpital's rule, the limit as x approaches 2 must result in an indeterminate form of 0/0.
What is the value of F(2) based on the given conditions?
-The value of F(2) is 1, as it is the only value that satisfies the condition that 1 - F(2)^3 = 0.
How is F'(2) determined using L'Hôpital's rule?
-F'(2) is determined by taking the derivative of the numerator and the denominator and evaluating the limit as x approaches 2, which results in F'(2) = 4.
What is the relationship between the function K and the values of G(2) and H(2)?
-Since G(2) is less than or equal to H(2), and H(2) is 4, K(2) must be between G(2) and H(2), which means K(2) is also 4.
How does the continuity of K(x) affect the value of K(2)?
-The continuity of K(x) implies that the limit as x approaches 2 of K(x) must equal K(2), which is 4, as per the given conditions.
Outlines
📚 AP Calculus: Tangent Line and Derivatives
In this paragraph, Allan Bottle Stem Coach discusses AP Calculus, specifically focusing on a response question from 2019. The content involves functions F, G, and H, which are twice differentiable. The problem involves finding the slope of a tangent line at a specific point, and using this information to derive expressions for the derivative of a new function 'a'. The paragraph also covers the application of l'Hôpital's rule to evaluate a limit and the use of continuity to determine the value of a function at a point. The key points include the product rule for derivatives, the concept of a tangent line, and the use of l'Hôpital's rule for indeterminate forms.
🔍 Continuity and Squeeze Theorem Application
This paragraph continues the mathematical discussion, focusing on the continuity of a function K, which is bounded by functions G and H. The coach uses the squeeze theorem to establish that the value of K at a certain point must be equal to 4, given the inequalities provided. The paragraph emphasizes the importance of understanding continuity and its implications for the values that a function can take. The summary highlights the application of the squeeze theorem and the concept of continuity in mathematical proofs.
Mindmap
Keywords
💡AP Calculus
💡Twice Differentiable Functions
💡Tangent Line
💡Slope
💡Product Rule
💡L'Hôpital's Rule
💡Indeterminate Form
💡Chain Rule
💡Continuity
💡Squeeze Theorem
💡Inequality
Highlights
The AP Calculus AB response question number six from 2019 is being discussed.
Functions F, G, and H are twice differentiable with G(2) = H(2).
The line y = (4/3)x - 2 is tangent to both functions F and H at x = 2.
H'(2) is identified as the slope of the tangent line, which is 2/3.
A new function a(x) = 3x^3 * H(x) is introduced.
The derivative a'(x) is calculated using the product rule.
a'(2) is computed to be 160 by substituting the values of H(2) and H'(2).
L'Hôpital's rule is used to evaluate the limit as x approaches 2 of H(x).
It's determined that F(2) must equal 1 for the limit to be indeterminate.
F'(2) is found to be 0 by applying L'Hôpital's rule to the expression 1 - 3F(x)^2 * F'(x).
The inequality G(x) ≤ H(x) for x between 1 and 3 is mentioned.
A function K is defined to satisfy the inequality and is found to be continuous.
K(2) is determined to be 4 based on the given inequality and continuity.
The squeeze theorem is applied to confirm the value of K(2).
The video provides a step-by-step walkthrough of solving the calculus problem.
Corrections and PDF solutions are available in the video description for any mistakes.
The presenter ensures the viewers that the answers are correct after applying the methods.
The video concludes with an invitation to the next video in the series.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: