Limit properties | Limits and continuity | AP Calculus AB | Khan Academy

Khan Academy
22 Jan 201305:07
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses various properties of limits in functions, focusing on their intuitive understanding rather than rigorous proof. It explains the sum, difference, product, constant multiple, quotient, and exponent properties of limits. The key takeaway is that the limit of a sum or difference of functions equals the sum or difference of their individual limits, the limit of a product equals the product of their limits, and the limit of a function divided by another is the quotient of their limits. These properties are essential for simplifying and solving limit problems.

Takeaways
  • 📚 The video discusses various properties of limits in mathematical functions, providing intuitive understanding without rigorous proofs.
  • 🔢 If the limit of f(x) as x approaches c is L, and the limit of g(x) as x approaches c is M, then the limit of [f(x) + g(x)] as x approaches c is L + M.
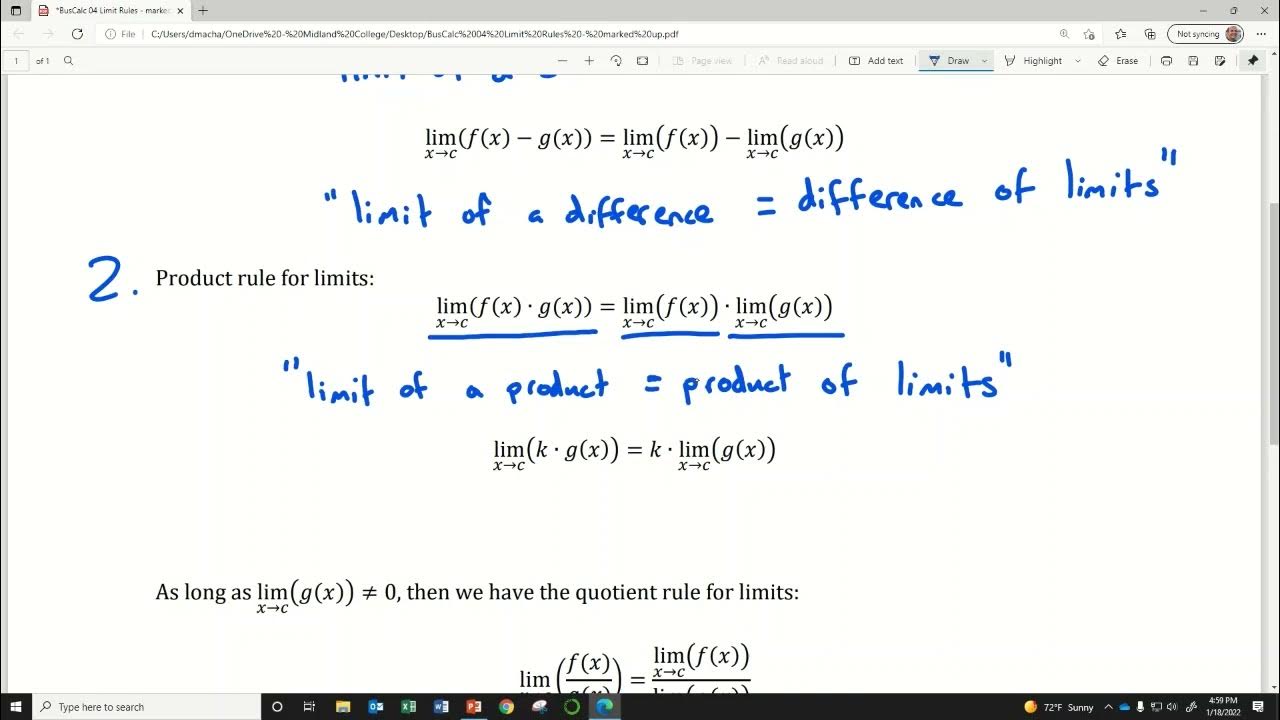
  • ➖ The limit of [f(x) - g(x)] as x approaches c is L - M, which is known as the difference property of limits.
  • 📈 For the product of functions, the limit of [f(x) * g(x)] as x approaches c equals the product of the individual limits (L * M).
  • 🅿️ When a function is multiplied by a constant k, the limit of [k * f(x)] as x approaches c is k times the limit of f(x), which simplifies to kL.
  • 🔄 The limit of [f(x) / g(x)] as x approaches c is the quotient of the individual limits (L / M), provided the denominator's limit is not zero.
  • 💪 The exponent property of limits states that the limit of [f(x)^(r/s)] as x approaches c is equal to (L^(r/s)), where r and s are non-zero integers.
  • 📊 Visual representation of functions can often help in understanding the properties of limits intuitively.
  • 🌟 The properties of limits are generally what one would naturally want to compute and are helpful in simplifying complex limit problems.
  • 📝 The epsilon-delta definition of limits will be covered in a separate tutorial for those seeking a more rigorous understanding.
Q & A

  • What is the main purpose of the video?

    -The main purpose of the video is to introduce and explain various properties of limits in mathematical functions, which can be helpful for simplifying limit problems.

  • Why isn't a rigorous proof provided for the properties of limits in this video?

    -A rigorous proof is not provided because it would require a detailed understanding of the epsilon-delta definition of limits, which will be covered in a separate tutorial.

  • If the limit of f(x) as x approaches c is L, and the limit of g(x) as x approaches c is M, what is the limit of [f(x) + g(x)] as x approaches c?

    -The limit of [f(x) + g(x)] as x approaches c is L + M, which is the sum of the individual limits.

  • What is the difference property of limits, and how is it applied?

    -The difference property of limits states that the limit of [f(x) - g(x)] as x approaches c is L - M, which is the difference of the individual limits.

  • How does the product property of limits work?

    -The product property of limits indicates that the limit of [f(x) * g(x)] as x approaches c is L * M, which is the product of the individual limits.

  • What happens when a constant is multiplied by a function that approaches a limit?

    -The limit of [k * f(x)] as x approaches c is equivalent to k times the limit of f(x) as x approaches c, which simplifies to k * L.

  • What is the quotient property of limits, and how is it used?

    -The quotient property of limits states that the limit of [f(x) / g(x)] as x approaches c is equal to the limit of f(x) as x approaches c divided by the limit of g(x) as x approaches c, which simplifies to L / M.

  • Explain the exponent property of limits.

    -The exponent property of limits indicates that the limit of [f(x)^(r/s)] as x approaches c is equal to [the limit of f(x) as x approaches c] raised to the r/s power, which simplifies to L^(r/s), provided r and s are integers and s is not zero.

  • How do the properties of limits help in simplifying limit problems?

    -The properties of limits provide a systematic way to break down and simplify complex limit problems by allowing us to perform operations such as addition, subtraction, multiplication, division, and exponentiation on the limits themselves, rather than the original functions.

  • Why are these limit properties considered intuitive?

    -These limit properties are considered intuitive because they often reflect the natural operations we would perform on the graphs of functions. For example, adding or subtracting the graphs of two functions corresponds to adding or subtracting their limits.

  • How can visual representation enhance understanding of limit properties?

    -Visual representation, such as graphs, can enhance understanding of limit properties by providing a geometric interpretation of the operations being performed on the functions. This can make the properties more tangible and easier to grasp intuitively.

Outlines
00:00
📚 Introduction to Limit Properties

The paragraph introduces the concept of limit properties in calculus, explaining that while a rigorous proof requires a formal definition of limits, the tutorial will focus on intuitive understanding. It sets the stage for discussing the sum rule, difference rule, constant multiple property, quotient property, and exponent property of limits, emphasizing their usefulness in simplifying limit problems.

05:01
📈 Visualization of Function Limits

This paragraph emphasizes the intuitive nature of limit properties by suggesting that visualizing the graphs of functions can help understand how limits behave under operations such as addition, subtraction, multiplication, and division. It reinforces the idea that the properties discussed are quite natural and align with what one might expect from a graphical perspective.

Mindmap
Keywords
💡limits
Limits in the context of the video refer to the value that a function approaches as the input (x) gets arbitrarily close to a certain point (c). It is a fundamental concept in calculus and is used to describe the behavior of functions. The video aims to explain various properties of limits to help simplify and understand limit problems better.
💡properties of limits
The properties of limits are rules or theorems that describe how limits behave under certain operations or transformations. In the video, these properties are used to illustrate how the limits of functions can be combined or manipulated to solve more complex problems. These properties are crucial for understanding and calculating limits in various mathematical scenarios.
💡epsilon-delta definition
The epsilon-delta definition is a rigorous mathematical way to define what a limit is. It involves the concept of arbitrary small positive numbers (epsilon) and the ability to find a number (delta) such that if the input to the function is within the delta neighborhood of a point, the function's output is within the epsilon neighborhood of the limit's value. The video mentions that a detailed proof of the properties of limits will be covered in a tutorial on this definition.
💡sum rule
The sum rule, or sum property, of limits states that the limit of the sum of two functions as x approaches a certain point is equal to the sum of the individual limits of those functions at that point. This property is intuitively illustrated in the video by adding the graphs of two functions and showing that the limit of their sum is the sum of their individual limits. For example, if the limit of f(x) as x approaches c is L and the limit of g(x) as x approaches c is M, then the limit of [f(x) + g(x)] as x approaches c is L + M.
💡difference rule
The difference rule, or difference property, of limits is similar to the sum rule but applies to the subtraction of functions. It states that the limit of the difference between two functions as x approaches a certain point is equal to the difference of the individual limits of those functions at that point. In the video, this is demonstrated by showing that if the limit of f(x) as x approaches c is L and the limit of g(x) as x approaches c is M, then the limit of [f(x) - g(x)] as x approaches c is L - M.
💡product rule
The product rule for limits asserts that the limit of the product of two functions as x approaches a certain point is equal to the product of the individual limits of those functions at that point. This property is straightforward and aligns with the multiplication of values. The video provides an example where if the limit of f(x) as x approaches c is L and the limit of g(x) as x approaches c is M, then the limit of [f(x) * g(x)] as x approaches c is L * M.
💡constant multiple property
The constant multiple property of limits indicates that the limit of a constant multiplied by a function as x approaches a certain point is equal to the constant multiplied by the limit of the function at that point. This property simplifies the process of finding limits when constants are involved. The video illustrates this by showing that if k is a constant and the limit of f(x) as x approaches c is L, then the limit of [k * f(x)] as x approaches c is k * L.
💡quotient property
The quotient property of limits explains how to find the limit of a function divided by another function as x approaches a certain point. It states that the limit of the quotient is equal to the quotient of the individual limits, provided the denominator's limit is not zero. The video clarifies this by demonstrating that if the limit of f(x) as x approaches c is L and the limit of g(x) as x approaches c is M, then the limit of [f(x) / g(x)] as x approaches c is L / M.
💡exponent property
The exponent property of limits deals with the behavior of limits when a function is raised to a power. It asserts that the limit of a function raised to an integer power as x approaches a certain point is equal to the limit of the function at that point raised to the same power. This property is applicable when both the numerator and the denominator of the power are integers and the denominator is not zero. The video provides an example where if the limit of f(x) as x approaches c is L, then the limit of [f(x)^(r/s)] as x approaches c is L^(r/s), where r and s are integers and s is not zero.
💡intuitive
The term 'intuitive' in the video refers to the ease with which one can understand or grasp the properties of limits without requiring rigorous mathematical proofs. The video emphasizes that while a formal proof will be provided in another tutorial, the properties discussed are fairly straightforward and make sense when considering the visual representation of functions on a graph.
💡rigorous proof
A 'rigorous proof' in mathematics is a detailed and exact demonstration that a statement is true, leaving no room for doubt or ambiguity. In the context of the video, it is mentioned that a rigorous proof of the properties of limits would require a formal understanding of the epsilon-delta definition, which is not covered in the current tutorial. The video aims to provide an intuitive understanding of the properties first, with the intention of covering a more formal approach in a subsequent tutorial.
Highlights

The video discusses various properties of limits in mathematics.

A rigorous proof of these properties requires a strict definition of limits, which will be covered in another tutorial.

The addition property of limits states that the limit of the sum of two functions equals the sum of their individual limits.

The subtraction property of limits indicates that the limit of the difference of two functions is the difference of their individual limits.

The product property of limits asserts that the limit of the product of two functions is the product of their individual limits.

The constant multiple property explains that the limit of a constant multiplied by a function is equal to the constant multiplied by the limit of the function.

The division property of limits, also known as the quotient property, means that the limit of the division of two functions is the quotient of their individual limits.

The exponent property of limits allows us to find the limit of a function raised to a power by raising the limit of the function to that power.

The video emphasizes the intuitive nature of these limit properties and their usefulness in simplifying limit problems.

The properties of limits discussed are essential for solving more complex mathematical problems involving functions.

The video provides a visual approach to understanding the properties of limits by relating them to the graphs of functions.

The sum rule, or addition property, of limits is a fundamental principle that simplifies the process of finding limits for the sum of functions.

The difference rule, or subtraction property, of limits is another basic principle that streamlines the computation of limits for the difference of functions.

The product property of limits is crucial for understanding how the multiplication of functions affects their limits.

The constant multiple property is key for determining the limit of a function when it is multiplied by a constant.

The quotient property of limits is important for calculating the limit of the division of two functions as they approach a certain value.

The exponent property of limits is a powerful tool for handling functions raised to fractional or integer powers in limit calculations.

Transcripts

Browse More Related Video

BusCalc 04 Limit Rules

Limits of combined functions: piecewise functions | AP Calculus AB | Khan Academy

Limit Laws | Breaking Up Complicated Limits Into Simpler Ones

Limits of combined functions | Limits and continuity | AP Calculus AB | Khan Academy

AP Calculus AB: Understanding how to evaluate limits algebraically and analyze limits graphically

AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus PropertiesLimits RulesSum PropertyDifference RuleProduct PropertyQuotient PropertyExponent PropertyIntuitive UnderstandingMath TutorialSimplification Techniques