AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits
TLDRIn this AP Calculus AB lecture, Mr. Bortnick delves into Unit 1, focusing on limits and continuity, particularly the algebraic properties of limits. He introduces basic limit theorems, such as constant, power, and polynomial function limits, and explores composite functions and trigonometric limits. The instructor also guides students through practice problems involving piecewise functions and composite limits, emphasizing the importance of understanding limit properties for solving calculus problems effectively.
Takeaways
- 📚 The lecture is part of an AP Calculus AB course focusing on Unit 1, Limits and Continuity, specifically Topic 1.5: Determining Limits using Algebraic Properties of Limits.
- 📝 The notes begin with an introduction to general properties of limits, which are foundational for understanding how to work with limits in calculus.
- 🔢 Theorem 1.1 establishes basic limits for constants and variables, including that the limit of a constant b as x approaches c is b, and the limit of x as x approaches c is c.
- 🆙 Theorem 1.2 introduces the properties of limits, allowing for operations such as scalar multiplication, addition, subtraction, product, quotient (with the caveat that the denominator cannot be zero), and power rules.
- 📈 Theorem 1.3 discusses the limits of polynomial and rational functions, stating that the limit of a polynomial p(x) as x approaches c is p(c), and similarly for rational functions provided the denominator is not zero at c.
- 🛑 Theorem 1.4 addresses the limit of a function involving a radical, with different conditions for odd and even roots, and for values of c greater than zero.
- 🔁 Theorem 1.5 explains how to handle composite functions, emphasizing that the limit of a composite function f(g(x)) as x approaches c is f(l), where l is the limit of g(x) as x approaches c.
- 📉 Theorem 1.6 confirms that all the limit properties discussed also apply to trigonometric functions, including sine, cosine, tangent, cotangent, secant, and cosecant.
- 📚 Practice problems are provided to apply the learned properties of limits, including working with piecewise functions and understanding when a limit exists or does not exist based on left and right limits.
- 📉 Emphasis is placed on the importance of correctly applying the properties of limits, especially when dealing with piecewise functions where the function's definition changes within different intervals.
- 💡 The script concludes with an invitation for students to ask questions, participate in class discussions, or seek help during office hours, highlighting the importance of engagement in the learning process.
Q & A
What is the main topic discussed in the video?
-The main topic discussed in the video is determining limits using algebraic properties of limits in the context of AP Calculus AB, specifically focusing on Unit 1 Limits and Continuity, Topic 1.5.
What are the basic limits theorems mentioned in the video?
-The basic limits theorems mentioned in the video are Theorem 1.1, which includes the limit of a constant, the limit of x as x approaches a constant c, and the limit of x raised to a power as x approaches a constant c.
What does Theorem 1.2 in the video allow us to do with limits?
-Theorem 1.2 allows us to perform basic operations with limits, such as scalar multiplication, addition or subtraction of limits, multiplication (product rule), division (quotient rule), and raising a limit to a power (power rule).
What is a polynomial function and how does the limit of a polynomial function behave as x approaches a constant c?
-A polynomial function is a function that involves only the basic arithmetic operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. According to the video, the limit of a polynomial function as x approaches a constant c is simply the polynomial evaluated at c, i.e., p(c).
What is a rational function and how is its limit determined as x approaches a constant c?
-A rational function is a function that can be expressed as the quotient of two polynomial functions. The limit of a rational function as x approaches a constant c is determined by substituting c into the function, provided the denominator does not evaluate to zero at c, resulting in p(c)/q(c).
What does Theorem 1.4 state about the limit of a function involving a radical?
-Theorem 1.4 states that the limit of a function involving a radical is valid for all c when the exponent n is odd, and valid for c greater than zero when n is even.
What is a composite function and how is its limit determined as x approaches a constant c?
-A composite function is a function composed of two functions, where one is applied within the other. The limit of a composite function as x approaches a constant c is determined by first finding the limit of the inner function as x approaches c, and then substituting that limit into the outer function.
What is the significance of the properties of limits discussed in the video for trigonometric functions?
-The properties of limits discussed in the video apply to trigonometric functions as well, meaning that the same rules for addition, subtraction, multiplication, division, and exponentiation of limits can be used for sine, cosine, tangent, cotangent, secant, and cosecant functions.
Can you provide an example of how to use the properties of limits to solve a problem, as demonstrated in the video?
-In the video, an example is given where the limit as x approaches 1 of f(-x) + g(x)/2 is calculated using the given limits of f(x) and g(x) as x approaches 1 and -1, respectively, and the properties of limits to separate and combine the limits accordingly, resulting in a final answer of 5.
How does the video approach the concept of limits for piecewise functions?
-The video explains that for piecewise functions, one must consider the appropriate rule based on the domain of the function where the limit is being taken. It demonstrates how to find the limit from the left and the right and how to determine if the overall limit exists by comparing these two values.
What is the importance of understanding the properties of limits in calculus?
-Understanding the properties of limits is crucial in calculus as they form the foundation for analyzing the behavior of functions, especially as input values approach certain points. They allow for the simplification and manipulation of limit expressions and are essential in solving problems involving complex functions and composite functions.
Outlines
📚 Introduction to Limits in AP Calculus AB
This paragraph introduces the topic of limits within the AP Calculus AB curriculum, focusing on the properties of limits and their algebraic manipulation. Mr. Bortnick begins by setting the stage for the lecture on 'Determining Limits using Algebraic Properties of Limits' and outlines the general properties that will be discussed. He emphasizes the foundational theorems that govern the behavior of limits, such as the limit of a constant being the constant itself, the limit of x as x approaches a value c being simply c, and the limit of x raised to a power n approaching c being c to the power of n. The paragraph lays the groundwork for understanding how limits can be applied to various mathematical functions.
🔍 Theorems and Properties of Limits
In this section, Mr. Bortnick delves into the specific theorems that govern the properties of limits. Theorems 1.1 and 1.2 are highlighted, which include the scalar multiple rule, sum/difference rule, product rule, quotient rule, and power rule for limits. These rules are essential for performing operations with limits, such as adding, subtracting, multiplying, dividing, and raising to a power. The explanation is clear and methodical, ensuring that students understand the principles behind each property and how they can be applied to solve limit problems.
📈 Limits of Polynomial, Rational, and Radical Functions
The third paragraph discusses the limits of polynomial and rational functions, as well as functions involving radicals. Mr. Bortnick explains that the limit of a polynomial function as x approaches a real number c is simply the function evaluated at c. For rational functions, the limit as x approaches c is the function evaluated at c, provided the denominator is not zero. Additionally, the limit of a function involving a radical is valid for all c when the radical is odd and for c greater than zero when the radical is even. This section provides a comprehensive understanding of how to handle different types of functions when determining limits.
🌐 Composite Functions and Trigonometric Limits
This paragraph introduces the concept of composite functions and their limits, as well as the limits involving trigonometric functions. The rule for composite functions is explained, stating that the limit of a composite function as x approaches c is the outer function evaluated at the limit of the inner function as x approaches c. Furthermore, it is mentioned that all the previously discussed limit operations apply to trigonometric functions, such as sine, cosine, tangent, cotangent, secant, and cosecant. This ensures that students are aware of the broad applicability of limit properties across various mathematical contexts.
📝 Practice Problems and Piecewise Functions
The focus of this paragraph is on applying the learned properties of limits to solve practice problems, particularly those involving piecewise functions. Mr. Bortnick provides a step-by-step approach to determining limits for piecewise functions, emphasizing the importance of considering the domain and the behavior of the function on either side of a point of discontinuity. The paragraph also covers how to handle limits for functions defined by different rules on different intervals, and the significance of the left and right limits in determining the overall limit of a function.
📉 Limits Involving Exponential Functions
In the final paragraph, Mr. Bortnick discusses the limits involving exponential functions, specifically highlighting the relationship between the natural logarithm and the base of the natural logarithm, e. He explains that when taking the limit of a function as x approaches e from the right side, and the function involves the natural logarithm of e to a power, the result simplifies to the power itself due to the inverse relationship between the natural logarithm and e. This insight is a valuable addition to the understanding of limits, especially in the context of exponential and logarithmic functions.
Mindmap
Keywords
💡AP Calculus AB
💡Limits
💡Continuity
💡Algebraic Properties of Limits
💡Theorems
💡Piecewise Functions
💡Composite Functions
💡Trigonometric Functions
💡Practice Problems
💡Mastery Check
Highlights
Introduction to the topic of determining limits using algebraic properties in AP Calculus AB.
Explanation of basic limit properties, such as the limit of a constant and the limit of x approaching a constant c.
Theorem 1.1 details the limits of basic functions like constants and x raised to a power.
Theorem 1.2 outlines properties of limits for operations such as scalar multiplication, sum/difference, product, quotient, and power rules.
The limit of polynomial and rational functions as per Theorem 1.3, emphasizing the substitution of the limit point in the function.
Theorem 1.4 discusses the limit of functions involving radicals and the conditions for odd and even integers.
Theorem 1.5 on the limits of composite functions and how to work from the inside out when evaluating them.
Theorem 1.6 confirms that all limit operations apply to trigonometric functions as well.
Practice problem approach by applying the properties of limits to piecewise functions and given values/functions.
Example 1 demonstrates using given limits to find the limit of a combination of functions without explicit function forms.
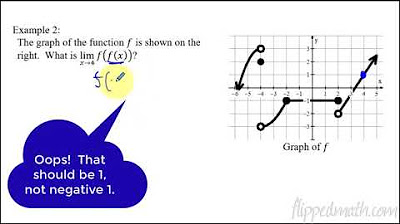
In Example 2, the concept of composite functions is applied using a graph to find the limit as x approaches a specific value.
Example 3 illustrates the use of limit properties for multiplication, addition, and subtraction with given function values and limits.
Explanation of how to handle piecewise functions in limit problems, as shown in Example 4, including the consideration of domain and function rules.
Finding limits from both the left and right for piecewise functions and determining if the overall limit exists.
The importance of understanding the domain of piecewise functions when evaluating limits from different directions.
Final notes encouraging students to practice problems and seek help if needed, emphasizing the importance of understanding limits in calculus.
Transcripts
Browse More Related Video

Calculus AB Homework 1.3 Evaluating Limits

AP Calculus AB - 1.3 Estimating Limit Values From Graphs

AP Calculus AB - 1.6b Determining Limits Using Algebraic Manipulation
Calculus AB/BC – 1.5 Determining Limits Using Algebraic Properties
AP Calculus AB - 1.7 Selecting Procedures for Determining Limits
AP Calculus AB - 1.9 Connecting Multiple Representations of Limits
5.0 / 5 (0 votes)
Thanks for rating: