Limits of combined functions: piecewise functions | AP Calculus AB | Khan Academy
TLDRThe video script discusses the concept of limits in calculus, emphasizing that the existence of a limit for a sum or product of functions does not necessarily depend on the individual limits of those functions. Through examples, it illustrates that even if the individual limits from the left and right do not exist or match, the overall limit of a sum or product may still exist and be equal if the left and right limits agree. This challenges the common misconception and highlights the nuances of limit calculations.
Takeaways
- 📌 The concept of limits is introduced with a focus on approaching values from the left and right.
- 🔍 The script presents a method to find limits by first attempting individual limits of functions and then combining them.
- ⚠️ It is highlighted that individual limits may not exist, but the combined limit of their sum or product might still exist.
- 💡 The example of approaching negative two demonstrates that even with conflicting individual limits, a combined limit can exist if both left and right approaches yield the same value.
- 📈 The sum of functions f(x) and g(x) at x = -2 is shown to have a limit of 4, despite individual functions having undefined limits.
- 🔄 The process of evaluating limits is reiterated with a new example approaching x = 1, emphasizing the importance of considering both left and right approaches.
- 🚫 The script points out that if left and right approaches yield different values, the limit does not exist, as seen with the sum of functions f(x) and g(x) at x = 1.
- ✖️ The product of functions f(x) and g(x) at x = 1 is found to have a limit of zero, despite the sum at the same point having no limit.
- 🤔 The examples serve to illustrate that the existence of a limit for a sum or product does not necessarily depend on the individual limits of the functions involved.
- 📚 The importance of understanding the behavior of functions as they approach certain values from different directions is emphasized for correctly evaluating limits.
Q & A
What is the main concept discussed in the video?
-The main concept discussed in the video is the evaluation of limits of functions, particularly focusing on scenarios where individual limits may not exist, but the combined limit of sums or products of functions does.
How does the instructor suggest approaching the first limit problem?
-The instructor suggests finding the limit as x approaches negative two for both f(x) and g(x), and then adding those two limits together.
What issue arises when trying to find the limit of f(x) and g(x) individually as x approaches negative two?
-The issue is that the limits do not exist because the values of f(x) and g(x) approach different numbers depending on whether x is approaching negative two from the left or the right.
Under what condition can the limit still exist despite the non-existence of individual limits?
-The limit can still exist if the limit from the left of the sum of f(x) and g(x) is equal to the limit from the right of the sum of f(x) and g(x).
What do the left and right limits of the sum of f(x) and g(x) approach when x is close to negative two?
-Both the left and right limits of the sum of f(x) and g(x) approach the same value, which is four.
What happens when trying to find the limit of f(x) and g(x) individually as x approaches one?
-The individual limits do not exist because the values of f(x) and g(x) depend on the direction from which x approaches one, leading to different values.
Does the limit of the sum of f(x) and g(x) as x approaches one exist?
-No, the limit of the sum of f(x) and g(x) as x approaches one does not exist because the left and right limits do not approach the same value.
What is the limit of the product of f(x) and g(x) as x approaches one?
-The limit of the product of f(x) and g(x) as x approaches one exists and is equal to zero, as both the left and right limits approach the same value.
Why are the examples given in the video significant?
-The examples are significant because they demonstrate cases where the component limits do not exist, yet the sum or product of the functions can have a well-defined limit, challenging the common assumption that the behavior of individual functions directly dictates the behavior of their sum or product.
What is the main takeaway from the video for students learning about limits?
-The main takeaway is that even if individual function limits do not exist, the sum or product of these functions can still have an existing limit, and it's important to evaluate each case separately.
Outlines
📚 Understanding Limit Calculations
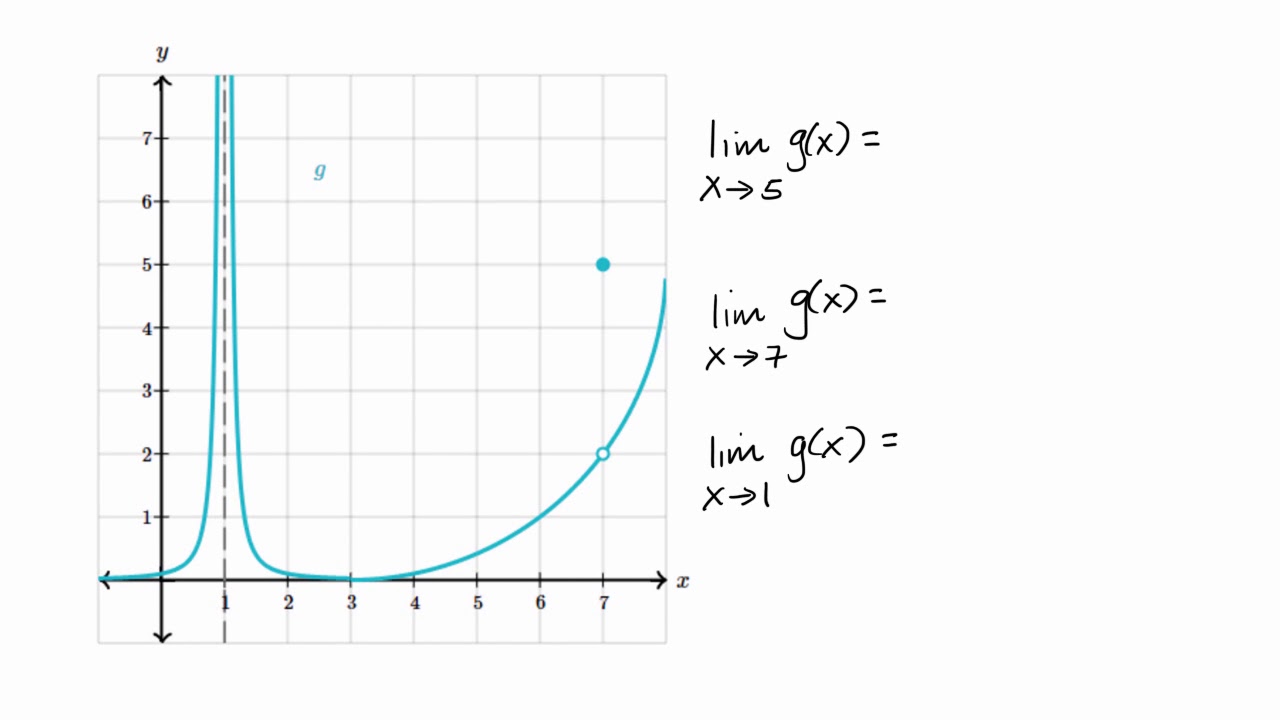
This paragraph discusses the process of finding limits for given functions, emphasizing the importance of trying to solve the problem independently before moving on to the solution. It introduces a specific problem involving two functions, f(x) and g(x), and their limits as x approaches negative two. The instructor explains that while individual limits of f(x) and g(x) do not exist from both sides, the limit of their sum can still exist if the left and right limits are equal. The paragraph provides a step-by-step analysis of the limits for the sum of the functions, the product of the functions, and the individual functions as x approaches specific values. It highlights the interesting cases where the component limits may not exist, but the sum or product of the functions does, offering a deeper understanding of limit calculations.
Mindmap
Keywords
💡limits
💡approaching
💡sum
💡product
💡discontinuities
💡functions
💡left and right
💡existence
💡additivity
💡multiplicity
💡behavior
Highlights
The concept of finding limits of functions as x approaches a certain value is introduced.
The strategy of finding limits of f(x) and g(x) separately and then adding them together is suggested for the first problem.
A problem is identified with the approach of adding separate limits when individual functions have different limits from the left and right.
The existence of a limit is possible if the left and right limits of the sum of functions exist and are equal.
For the first example, the left limit of f(x) is 1 and g(x) is 3, while the right limit for f(x) is 3 and g(x) is 1.
The sum of the limits from both directions for the first example equals 4, indicating the limit exists and is equal to 4.
The second example involves finding the limit as x approaches 1 for the sum of f(x) and g(x), which initially seems to not exist for individual functions.
Despite non-existence of individual limits, the limit of the sum from the left approach equals 2 for the second example.
The limit from the right approach for the sum in the second example equals negative 1, showing that the limit does not exist for the sum.
The third example explores the limit of the product of f(x) and g(x) as x approaches 1.
The left approach limit for the product results in a value of zero, as one function approaches 2 and the other zero.
The right approach limit for the product also results in zero, confirming the existence of the limit for the product.
The examples demonstrate that even if individual limits don't exist, the sum or product of functions might still have a limit.
The method of evaluating limits from different directions provides insight into the behavior of functions near certain points.
The importance of checking both left and right limits for the sum and product of functions is emphasized.
The transcript provides a clear explanation of the concept of limits and their application in evaluating functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: