AP Calc AB & BC Practice MC Review Problems #7
TLDRThe video script is a comprehensive review session for AP Calculus AB and BC exams, focusing on multiple-choice questions that require calculator proficiency. The presenter guides viewers through various calculus problems, emphasizing the importance of understanding the fundamental theorem of calculus, identifying intervals of concavity, and calculating volumes of solids with known cross-sections. The session also covers finding the rate of change of a particle's velocity, determining the slope of a graph at a specific point, and calculating the remaining water in a tank after a certain period. Throughout the script, the presenter shares tips on using a calculator effectively, maintaining accuracy, and the significance of rereading problems to avoid mistakes. The review aims to enhance students' problem-solving skills and build confidence in using calculators for complex calculus tasks.
Takeaways
- 📚 Start by understanding the basics of AP Calculus AB and BC, as the BC curriculum includes everything from AB.
- 🔗 Utilize additional resources such as playlists and problem sets for practice, which are often based on actual AP exam problems.
- 📝 The Fundamental Theorem of Calculus is essential for solving problems involving the position of a particle over time.
- 🧮 Master the use of your calculator for solving integrals and derivatives, as it can provide a significant time advantage during exams.
- ✅ Always double-check the problem's requirements to ensure you are solving for the correct quantity, such as the rate of change or the concavity of a graph.
- 📉 When analyzing the concavity of a graph, look for intervals where the second derivative is less than zero.
- 🚰 For problems involving rates of change, like water being pumped out of a tank, integrate the rate function over the given time interval to find the total change.
- 📈 To find when a particle's velocity is increasing most rapidly, look for the absolute maximum of the acceleration function.
- 🏗 For solids with known cross-sections, calculate the volume by integrating the cross-sectional area over the given interval.
- 🔍 When finding the slope of a graph at a specific point, use the derivative of the function at that point.
- 💧 When calculating the remaining water in a tank after a certain time, ensure you are using the correct sign for the rate of water being pumped out.
Q & A
What is the main topic of the video?
-The video is a review of multiple-choice calculator problems for the AP Calculus AB and BC exams.
Why are the problems in the video not from actual AP exams?
-The problems are not from actual AP exams because many of those exams cannot be released, but they are based on AP exam problems.
What is the significance of the Fundamental Theorem of Calculus in the first problem discussed in the video?
-The Fundamental Theorem of Calculus is used to relate the velocity function to the position function by integrating the velocity function over the time interval from T=3 to T=8.
How does the video demonstrate the use of a calculator for solving calculus problems?
-The video demonstrates the use of a calculator by defining functions, performing integration, and using various calculator shortcuts to solve calculus problems efficiently.
What does the term 'concave down' mean in the context of the second problem?
-In the context of the second problem, 'concave down' refers to the intervals where the graph of the second derivative of the function f is less than zero.
How does the video approach finding the intervals where the graph of a function is concave down?
-The video uses a calculator to find the second derivative of the function, then graphs it to visually identify the intervals where it is less than zero.
What is the role of the rate function in the third problem about the water tank?
-The rate function r(T) represents the rate at which the water depth in the tank increases per minute. It is integrated over time to find the total increase in water depth from T=0 to T=5.
How does the video determine the value of T when the particle's velocity is increasing most rapidly in the fourth problem?
-The video finds the derivative of the acceleration function and then uses the calculator to determine where the derivative has an absolute maximum, which corresponds to when the velocity is increasing most rapidly.
What is the geometric shape of the cross-section in the fifth problem about the solid with the region R as its base?
-The cross-section in the fifth problem is an isosceles right triangle, with its hypotenuse in the region R.
How does the video calculate the volume of the solid with known cross-sections in the fifth problem?
-The video calculates the volume by integrating the area of the cross-section (an isosceles right triangle) from one boundary to another of the region R.
What is the main takeaway from the video regarding the use of a calculator in AP Calculus exams?
-The main takeaway is the importance of being proficient with a calculator, as it can provide significant time-saving benefits during the exam, allowing students to focus more on understanding and solving the problems rather than how to use the calculator.
Outlines
📚 AP Calculus Exam Review
This video is a comprehensive review session focusing on multiple-choice questions for the AP Calculus AB and BC exams. The presenter emphasizes the importance of understanding both AB and BC material for those taking the BC exam. The video provides a detailed walkthrough of problem set number seven, using a calculator to solve complex calculus problems. The problems, while not from actual AP exams, are designed to mimic the style and difficulty of those found on the real exams. The presenter also advises viewers to consult with their teachers for access to actual AP exam problems and stresses the importance of calculator proficiency for the exam.
🔢 Calculator Techniques for AP Calculus
The presenter demonstrates advanced calculator techniques for solving calculus problems, specifically focusing on problems that require integration and differentiation. The video covers how to use a calculator to find the position of a particle given its velocity function, determine intervals where a graph is concave down by analyzing the second derivative, and calculate the increase in water depth in a tank over time. The presenter also discusses the importance of understanding the rate of change and how to integrate this rate over a given time period to find total change.
📈 Graphing and Derivatives in Calculus
The video script delves into the process of graphing functions and using derivatives to analyze the behavior of these functions. The presenter discusses how to adjust the window settings on a graphing calculator to focus on the relevant region of a function. They also explain how to find where a function is concave down by looking for where the second derivative is less than zero. Additionally, the presenter shows how to calculate the volume of a solid with a known cross-section and how to find the point at which a particle's velocity is increasing most rapidly by analyzing the absolute maximum of the acceleration function.
🧮 Calculating Volumes of Solids with Known Cross-Sections
The presenter provides a step-by-step guide on calculating the volume of a solid with a known cross-section, specifically an isosceles right triangle. They explain how to find the intersection points of two functions and how to set up the integral that represents the volume of the solid. The video demonstrates the use of a calculator to perform the integration and find the volume, emphasizing the importance of understanding the geometry of the cross-section and the limits of integration.
🔍 Finding the Slope of a Graph at a Specific Point
The video script outlines the process of finding the slope of a graph at the point where it crosses the x-axis. The presenter shows how to graph the function, find the point of intersection with the x-axis, and then calculate the derivative of the function at that point. They demonstrate two methods for finding the slope: using a graphing calculator's built-in dy/dx function and manually calculating the derivative using a stored x-value. The presenter also discusses the importance of verifying the results obtained from the graphing calculator.
🚰 Calculating Water Levels in a Tank
The presenter tackles a problem involving the calculation of water levels in a tank being drained. They provide a corrected approach after initially making a mistake in their calculation. The video demonstrates how to use a calculator to find the integral of the rate at which water is being pumped out, taking care to ensure the correct interpretation of the rate function. The presenter emphasizes the importance of re-reading the problem to understand the context and direction of the rate (e.g., inflow vs. outflow) and calculates the approximate amount of water remaining in the tank after a certain period.
📉 Identifying Local Maxima and Minima
The video script includes a problem-solving session for identifying local maxima and minima of a function. The presenter uses the given first derivative to graph the function and find where it transitions from positive to negative, indicating a local maximum. They demonstrate how to use the calculator to find the exact point of the local maximum by finding the zero of the first derivative. The presenter also advises on the importance of reading the problem carefully and understanding the context before proceeding with the calculation.
🏗 Calculating the Volume of a Solid with a Specific Cross-Section
The presenter explains how to calculate the volume of a solid with a base in the first quadrant, bounded by two functions. The cross-sections perpendicular to the x-axis are rectangles with heights that are five times their bases. The video demonstrates how to set up the integral that represents the volume of the solid, taking into account the dimensions of the cross-section. The presenter uses a calculator to find the integral and provides the final answer for the volume of the solid.
🚀 Applying the Fundamental Theorem of Calculus
The final part of the script involves applying the fundamental theorem of calculus to find the velocity of a particle at a future time point, given its velocity at a current time point. The presenter sets up the integral of the acceleration function over the time interval of interest and uses a calculator to compute the definite integral. They highlight the importance of being familiar with the calculator and the process of integration, as well as the need to read and understand the problem statement before attempting to solve it.
Mindmap
Keywords
💡AP Calculus
💡Fundamental Theorem of Calculus
💡Derivative
💡Integral
💡Concave Down
💡Velocity and Acceleration
💡Calculator
💡Rate of Change
💡Absolute Maximum
💡Solid of Revolution
💡Slope of a Graph
Highlights
Reviewing multiple-choice calculator problems for AP Calculus AB and BC exams
Problem set number seven is being reviewed, with a playlist available for other sets
The problems are not from actual AP exams but are based on them
Use of the fundamental theorem of calculus to solve for the position of a particle
Integration from 3 to 8 to find the position of a particle at time T equals eight
Using a calculator to define and manipulate functions for solving calculus problems
Identifying intervals where a graph is concave down by finding where the second derivative is less than zero
Using a graphing calculator to find intersection points and intervals for concavity
Calculating the increase in water depth in a tank over time using integration
Finding the value of T where a particle's velocity is increasing most rapidly by finding an absolute maximum
Using the derivative to analyze the behavior of velocity and acceleration functions
Calculating the volume of a solid with a known cross-section using integration
Determining the slope of a graph at a specific point where it crosses the x-axis
Solving for the amount of water remaining in a tank after a certain time with a pump rate given
Finding a local maximum of a function by analyzing the sign change of its derivative
Using the volume of known cross-sections to calculate the volume of a solid bounded by two graphs
Emphasizing the importance of reading problems carefully to avoid calculation errors
Demonstrating the process of using a calculator to solve calculus problems efficiently
Transcripts
Browse More Related Video

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92
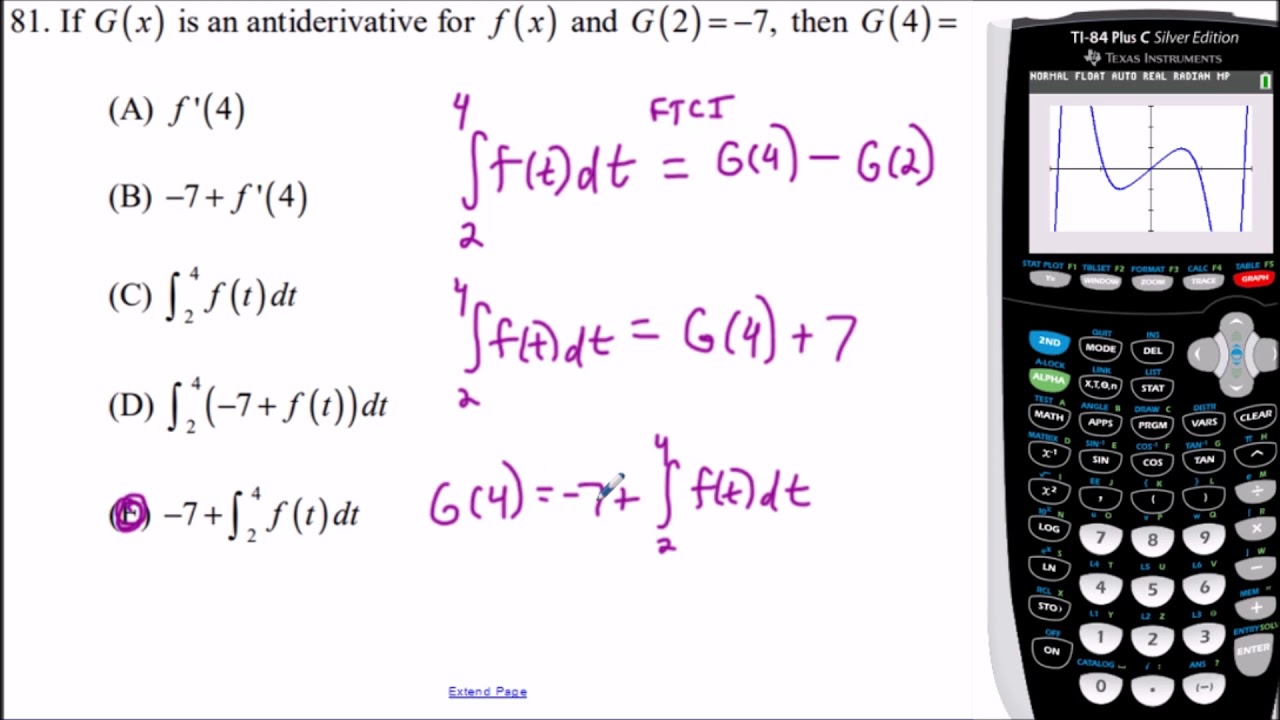
AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92

AP Calculus AB 2012 Multiple Choice (calculator) - Questions 76 - 92

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 2)
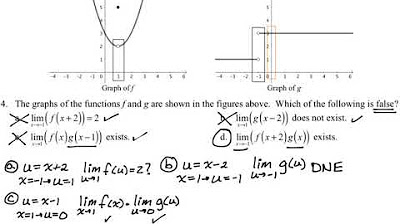
AP Calc AB & BC Practice MC Review Problems #3
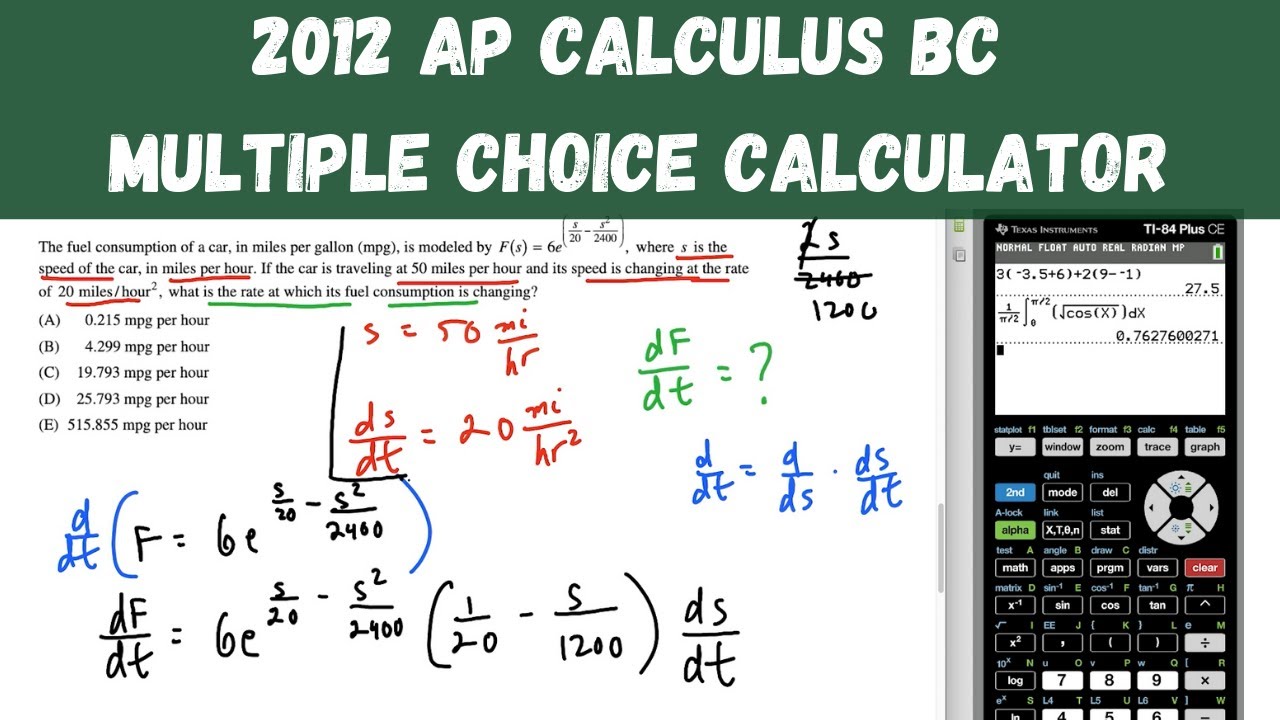
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92
5.0 / 5 (0 votes)
Thanks for rating: