Area between curves | Applications of definite integrals | AP Calculus AB | Khan Academy
TLDRThe video script discusses the concept of calculating the area between two curves using definite integrals. It explains that the area can be found by subtracting the integral of the lower curve from the integral of the upper curve over a given interval. The process is demonstrated for various scenarios, including when both curves are above the x-axis, when one is above and the other below, and when both are below the x-axis. The key takeaway is that the area between curves is represented by the integral of the difference of their functions over the interval where the upper function is greater than the lower function.
Takeaways
- 📊 The concept of using definite integrals to find the area between curves is discussed.
- 📈 To calculate the area between two curves, subtract the integral of the lower curve (g(x)) from the integral of the upper curve (f(x)) over the interval [a, b].
- 🔢 The formula for finding the area between two curves is ∫ from a to b of (f(x) - g(x)) dx.
- 🌐 When f(x) is above the x-axis and g(x) is below, the area between the curves is still calculated as the integral of (f(x) - g(x)).
- 🔄 The properties of integrals allow us to break down the area calculation into more manageable parts by using subtraction of integrals.
- 📉 If both functions f(x) and g(x) are below the x-axis, the area between them is found by taking the negative of the integral of g(x) and adding it to the negative of the integral of f(x).
- 🤔 The key to solving these problems is understanding the geometric interpretation of integrals and how they relate to the area under or above the x-axis.
- 🧩 The area between curves can be visualized as a series of smaller areas, each represented by the difference in integrals of the two functions over subintervals.
- 📚 The method is versatile and works for any interval where f(x) > g(x), regardless of the position of the curves relative to the x-axis.
- 🌟 The integral of a function below the x-axis represents a negative area, but the absolute value of this integral is used in the calculation of the area between curves.
Q & A
What is the main concept discussed in the video transcript?
-The main concept discussed in the video transcript is the calculation of the area between two curves using definite integrals.
How is the area between two curves defined in terms of definite integrals?
-The area between two curves is defined as the difference between the integrals of the two functions over the given interval, represented as the integral from 'a' to 'b' of (f(x) - g(x)) dx.
What happens when both functions, f(x) and g(x), are above the x-axis?
-When both functions are above the x-axis, the area between the curves is calculated by subtracting the integral of g(x) from the integral of f(x) over the interval.
How does the process differ when one function is above the x-axis and the other is below?
-When one function is above and the other is below the x-axis, the integral of the function below the x-axis yields a negative value. The area is then found by taking the positive area (from the function above the x-axis) and subtracting the absolute value of the negative area (from the function below the x-axis).
What is the significance of the parentheses in the integral (f(x) - g(x)dx)?
-The parentheses in the integral (f(x) - g(x)dx) indicate that the integral is being evaluated as a single expression, which is crucial for determining the area between the curves correctly.
How does the area between two curves change when both functions are below the x-axis?
-When both functions are below the x-axis, the area between the curves is still calculated using the integral of (f(x) - g(x)) over the interval. However, both integrals will yield negative values, and the area is found by adding the absolute values of these integrals.
What is the role of the definite integral in calculating the area between curves?
-The definite integral plays a crucial role in calculating the area between curves by summing up the infinitesimal areas under the curves over the given interval, allowing for the determination of the total area enclosed by the curves.
How does the sign (positive or negative) of the function affect the calculation of the area?
-The sign of the function affects the calculation by determining whether the area represented by the integral is positive (above the x-axis) or negative (below the x-axis). The final area between curves is obtained by combining these areas appropriately, considering their signs.
What is the application of the concept of area between curves in real-world scenarios?
-The concept of area between curves has numerous applications in real-world scenarios, such as calculating forces in physics, determining volumes in geometry, and modeling various phenomena in engineering and science.
How does the video transcript relate to the provided search results?
-The video transcript provides a conceptual understanding of the calculation of the area between curves using definite integrals, which is supported by the information found in the provided search results about definite integrals, their properties, and applications.
What are some limitations of using definite integrals to find the area between curves?
-Some limitations include the complexity of evaluating certain integrals, the need for appropriate functions to define the curves, and the potential for numerical approximations when exact solutions are not available.
Outlines
📚 Understanding Area Between Curves
This paragraph introduces the concept of calculating the area between two curves using definite integrals. The instructor explains that the area between curves f(x) and g(x) from x=a to x=b can be found by taking the integral of (f(x) - g(x)) dx. It is highlighted that this method works even when one curve is above the x-axis and the other is below, as the integral of the lower curve will yield a negative value, which can be subtracted from the area calculated by the upper curve's integral. The paragraph emphasizes the versatility of this approach, showing that it can be applied to various scenarios, including when both curves are below the x-axis.
📈 Applying Integration Properties
The second paragraph delves deeper into the application of integration properties to find the area between two curves. It explains that the integral from m to n of (f(x) - g(x)) dx can be broken down into the difference between the integrals of f(x) and g(x) individually. The paragraph clarifies that the integral of g(x) will yield a negative area, but when combined with the integral of f(x), it results in the total area of interest. The explanation includes a discussion of how negative values are handled and how they contribute to the final positive area between the curves. The paragraph reinforces the idea that the integral of the difference between the two functions is a consistent and effective method for determining the area between curves, regardless of their positions relative to the x-axis.
Mindmap
Keywords
💡Definite Integral
💡Area Between Curves
💡Interval
💡f(x) and g(x)
💡Negative Value
💡Integration Properties
💡x-axis
💡Signed Area
💡Subtraction of Integrals
💡Mathematical Notation
💡Contextual Understanding
Highlights
The concept of calculating the area between curves using definite integrals is introduced.
The method involves finding the area between two curves from x=a to x=b for functions f(x) and g(x).
The area can be determined by subtracting the integral of g(x) from the integral of f(x) over the given interval.
The integral of f(x) over the interval [a, b] gives the area below f(x) and above the x-axis.
The integral of g(x) over the same interval represents the area below the x-axis and above g(x).
The area between the curves is the net difference obtained by subtracting the two integrals.
The integral property allows the combination of the two integrals into a single integral of (f(x) - g(x)) dx from a to b.
The method is valid even when f(x) is above and g(x) is below the x-axis, as the negative value of g(x)'s integral is accounted for.
When both f(x) and g(x) are below the x-axis, the area of interest is the absolute value of the integral of (f(x) - g(x)).
The integral of (f(x) - g(x)) from c to d provides the area between the curves over any interval where f(x) > g(x).
The integral from m to n of f(x) - g(x) equals the difference between the integrals of f(x) and g(x) over the same interval.
The area below f(x) and above g(x) is found by taking the absolute value of the integral of (f(x) - g(x)) from m to n.
The integral of g(x) over the interval [m, n] gives a negative value, but its absolute value represents the area below g(x).
The area of interest is obtained by adding the absolute value of the integral of f(x) and subtracting the absolute value of the integral of g(x).
The method applies to all scenarios, providing a consistent way to find the area between curves over a given interval.
The process simplifies to the integral of (f(x) - g(x)) dx from the interval's endpoints, regardless of the curves' positions relative to the x-axis.
This approach to calculating the area between curves is a powerful tool in understanding the relationship between functions and their graphical representations.
Transcripts
Browse More Related Video
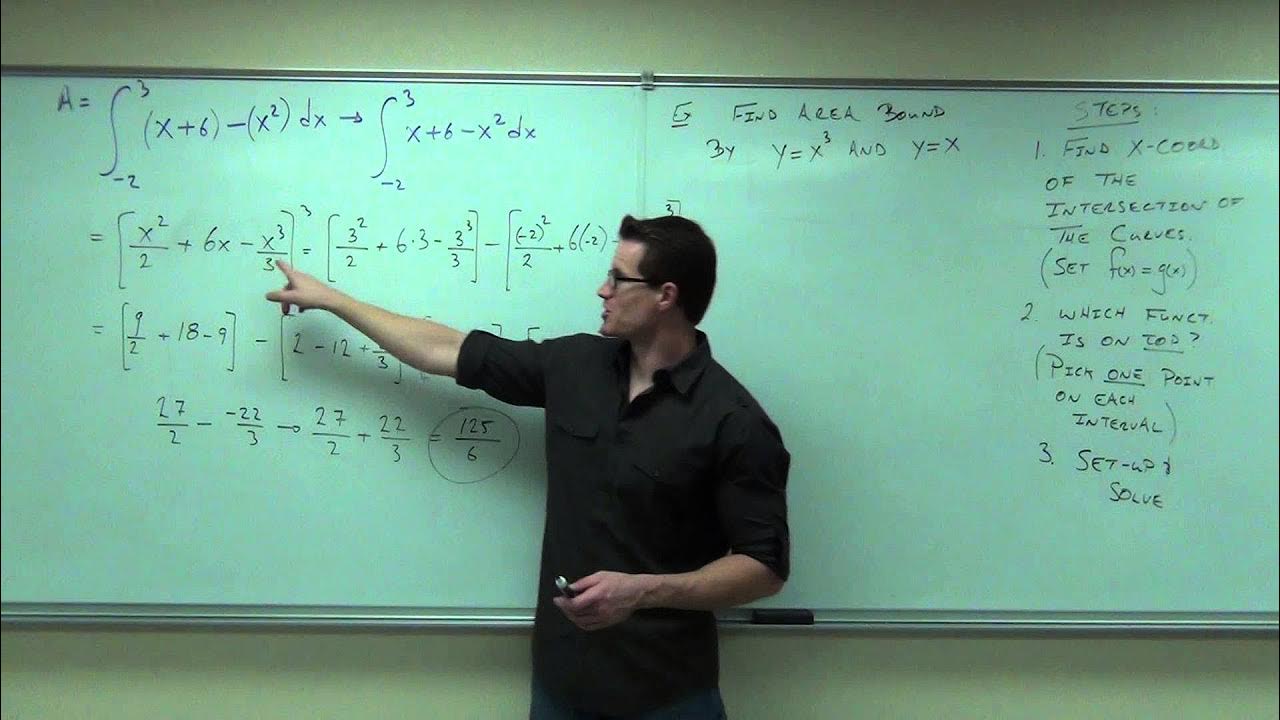
Calculus 1 Lecture 5.1: Finding Area Between Two Curves

Area between a curve and the x-axis: negative area | AP Calculus AB | Khan Academy

Definite Integrals!
Definite integrals (area under a curve) with GDC [IB Maths AI SL/HL]

Finding The Area Bounded By Three Curves Using Definite Integrals - Calculus

Area Between Two Curves-Curves that Cross
5.0 / 5 (0 votes)
Thanks for rating: