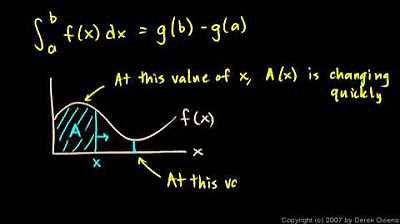Area between a curve and the x-axis: negative area | AP Calculus AB | Khan Academy
TLDRThis transcript delves into the concept of calculating the area under a curve, specifically using the cosine function as an example. It explains the process of finding the area between defined intervals on the x-axis, utilizing the definite integral and the second fundamental theorem of calculus. The explanation includes finding the antiderivative of the cosine function (the sine function) and evaluating it at given points to determine the area. The transcript also clarifies the concept of signed areas, where a negative area indicates the region lies below the x-axis. The process is demonstrated with various intervals, highlighting how the definite integral calculates the net area above the x-axis, which can be positive, negative, or zero depending on the function's position relative to the x-axis.
Takeaways
- 📊 The concept of the definite integral is introduced as a way to find the area under a curve and above the x-axis.
- 🌀 The area under the curve of y = cosine(x) between 0 and π/2 is calculated using the definite integral from 0 to π/2 of cosine(x) dx.
- 📈 The antiderivative of cosine(x) is sine(x), which is used to evaluate the definite integral for the area calculation.
- 🔄 The second fundamental theorem of calculus is key in evaluating the definite integral by finding the antiderivative and applying it to the given interval.
- 🏢 When the area under the curve is between π/2 and 3π/2, the result is negative, indicating that the area is below the x-axis.
- 🌍 The concept of a signed area is explained, where a negative area represents the region below the x-axis and a positive area above it.
- 🔢 The definite integral from 0 to 3π/2 of cosine(x) dx results in a net area of -1, showing that the area below the x-axis is greater than the area above it within the interval.
- 🔄 The integral from 0 to 2π of cosine(x) dx evaluates to 0, as the positive and negative areas cancel each other out over a complete period.
- 📊 The process of evaluating definite integrals involves finding the antiderivative, then applying the fundamental theorem of calculus to find the difference in the antiderivative's values at the interval's bounds.
- 🌐 The script uses the example of the cosine function to illustrate the principles of integration and the concept of signed areas in relation to the x-axis.
Q & A
What is the main topic discussed in the transcript?
-The main topic discussed in the transcript is the concept of calculating the area under the curve of a function, specifically y = cosine(x), over different intervals using definite integrals.
How is the area under the curve represented mathematically?
-The area under the curve is represented mathematically as the definite integral of the function over the given interval. For example, the area between x = 0 and x = π/2 is represented as the definite integral from 0 to π/2 of cosine(x) dx.
What is the fundamental theorem of calculus used to calculate the area under the curve?
-The second fundamental theorem of calculus is used to calculate the area under the curve. It states that the area can be found by evaluating the antiderivative of the function at the upper limit of integration and subtracting the value of the antiderivative at the lower limit of integration.
What is the antiderivative of cosine(x)?
-The antiderivative of cosine(x) is sine(x). This is because the derivative of sine(x) is cosine(x), according to the basic rules of differentiation.
How does the antiderivative include an arbitrary constant?
-The antiderivative includes an arbitrary constant because the derivative of any constant is zero. Therefore, the derivative of sine(x) plus any arbitrary constant will still yield cosine(x), making sine(x) plus an arbitrary constant a valid antiderivative of cosine(x).
What is the area under the curve of y = cosine(x) from x = 0 to x = π/2?
-The area under the curve from x = 0 to x = π/2 is equal to 1. This is found by evaluating the antiderivative sine(x) at π/2 and subtracting the value of sine(x) at 0, which gives sine(π/2) - sine(0) = 1 - 0 = 1.
What does a negative area indicate in the context of definite integrals?
-A negative area in the context of definite integrals indicates that the area under the curve is below the x-axis. The sign of the area reflects the relative position of the curve with respect to the x-axis.
What is the net area calculated when integrating cosine(x) from x = 0 to x = 3π/2?
-The net area calculated when integrating cosine(x) from x = 0 to x = 3π/2 is negative 1. This indicates that the total area above the x-axis is 1 (from 0 to π/2), and the total area below the x-axis is 2 (from π/2 to 3π/2), resulting in a net area of -1.
How does the definite integral find the net area above the x-axis?
-The definite integral finds the net area above the x-axis by evaluating the antiderivative of the function at the upper limit and subtracting the value of the antiderivative at the lower limit. If the result is positive, more area is above the x-axis; if negative, more area is below; and if zero, the areas above and below net out to zero.
What happens when the definite integral of cosine(x) is taken from x = 0 to x = 2π?
-When the definite integral of cosine(x) is taken from x = 0 to x = 2π, the result is 0. This is because the positive area under the curve from 0 to π/2 (which is 1) nets out with the negative area from π/2 to 3π/2 (which is -2), and the remaining area from 3π/2 to 2π also nets out to zero.
How does the concept of signed area relate to the position of the curve relative to the x-axis?
-The concept of signed area relates to the position of the curve relative to the x-axis by assigning a positive sign to the area above the x-axis and a negative sign to the area below the x-axis. This allows for a clear representation of the net area without regard to the curve's position, providing a single numerical value that can be positive, negative, or zero depending on the relative amounts of area above and below the x-axis.
Outlines
📊 Calculating Area Under the Cosine Curve (0 to π/2)
The paragraph discusses the process of calculating the area under the curve of the function y = cosine(x) over the interval from x = 0 to x = π/2. It explains the concept of definite integrals and how they relate to the sum of an infinite number of infinitely thin rectangles with height f(x) and width dx. The paragraph emphasizes the use of the second fundamental theorem of calculus to find the antiderivative of cosine(x), which is sine(x). By evaluating sine(x) at the upper and lower bounds of the interval, the area is determined to be 1, as sine(π/2) is 1 and sine(0) is 0, resulting in a net area of 1 - 0 = 1.
📉 Negative Area Under the Cosine Curve (π/2 to 3π/2)
This paragraph delves into the calculation of the area under the curve of y = cosine(x) from x = π/2 to x = 3π/2. It highlights the concept of negative area, which indicates that the area under consideration is below the x-axis. The antiderivative of cosine(x) is again used, and by evaluating it at the interval bounds, sine(3π/2) is found to be -1 and sine(π/2) is 1. The result is a negative 2, signifying an area of 2 below the x-axis. The paragraph also explains the interpretation of negative areas in the context of the real world, where areas are typically considered positive, and how the definite integral accounts for the net area above the x-axis by incorporating signed (positive or negative) values.
🔄 Net Area Calculation Over a Full Period (0 to 2π)
The final paragraph discusses the calculation of the definite integral from 0 to 2π of the function y = cosine(x). It demonstrates how the areas above and below the x-axis net out over a full period of the cosine function. The antiderivative, sine(x), is evaluated at the bounds of the integral, resulting in sine(2π) - sine(0), which equals 0 - 0 = 0. This outcome indicates that the positive area from 0 to π/2 and the negative area from π/2 to 2π cancel each other out, leaving no net area above the x-axis for the full period of the cosine function.
Mindmap
Keywords
💡Graph
💡Cosine
💡Definite Integral
💡Antiderivative
💡Second Fundamental Theorem of Calculus
💡Area
💡Interval
💡Sine
💡Net Area
💡Unit Circle
💡Signed Area
Highlights
The concept of calculating the area under a curve using definite integrals is introduced.
The area under the curve y = cosine of x from x = 0 to x = pi/2 is calculated.
The fundamental theorem of calculus is applied to find the area under the curve.
The antiderivative of cosine of x is identified as sine of x.
The area of the first region (0 to pi/2) is found to be 1.
The area under the curve from pi/2 to 3pi/2 is calculated, resulting in a negative area.
A negative area indicates that the region is below the x-axis.
The definite integral is explained as finding the net area above the x-axis.
The area under the curve from 0 to 3pi/2 is calculated and found to be -1.
The interpretation of a negative definite integral is discussed.
The integral from 0 to 2pi of cosine of x is calculated and found to be 0, indicating that areas above and below the x-axis net out.
The process of evaluating the antiderivative at the bounds of the interval is detailed.
The concept of an arbitrary constant in the antiderivative is explained.
The visualization of sine values on the unit circle is used to understand the results.
The practical application of definite integrals in calculating areas is emphasized.
The importance of understanding the concept of signed areas in calculus is highlighted.
The transcript provides a clear and detailed explanation of the relationship between the antiderivative and the definite integral.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: