Learn how to use the trapezoidal rule with 4 sub intervals

Brian McLogan
27 Mar 201809:23
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explores the trapezoidal method for calculating the area under a curve, highlighting its significance and efficiency. The instructor begins with a reminder of the trapezoid area formula, then demonstrates how this concept is adapted to approximate the area under a curve by dividing it into trapezoids. The script meticulously unfolds the process of calculating these areas, step by step, using the trapezoidal rule formula. It emphasizes understanding the transformation from traditional height to delta x and how function values serve as the trapezoid bases. The instructor guides through simplifying the calculation process, introducing a streamlined formula that factors out constants and efficiently sums up the function values at specified intervals. This educational dialogue not only reinforces the trapezoidal method's principles but also aims to enhance comprehension and application skills in practical scenarios.

Takeaways
  • 📚 The trapezoidal method is used for approximating the area under a curve by dividing it into trapezoids.
  • 🔸 The formula for the area of a trapezoid (1/2 * (base1 + base2) * height) is adapted to calculate the area under the curve.
  • 📈 The bases of the trapezoids correspond to function values at different points (f(a), f(b)), replacing traditional base lengths.
  • 📉 The height of each trapezoid is represented by Δx, the difference between consecutive x-values, instead of the traditional trapezoid height.
  • 🔧 The method involves summing up the areas of each trapezoid to approximate the total area under the curve.
  • 🔢 To simplify calculations, factors common to all trapezoids, such as 1/2 and Δx, can be factored out.
  • 📝 The simplified formula for the trapezoidal approximation is a sum involving the first and last y-values and twice the intermediate y-values, all multiplied by Δx/2.
  • 🖥 The example calculation transitions from a step-by-step sum of individual trapezoid areas to a streamlined formula application.
  • 📊 An error in the manual calculation is corrected through discussion, emphasizing the importance of careful calculation.
  • 📏 The final result of the approximation exercise is presented as 11/32 after correcting for a minor calculation mistake.
Q & A

  • What is the area formula for a trapezoid?

    -The area formula for a trapezoid is area equals one-half times the sum of base one and base two times the height.

  • How is the trapezoidal method applied in the context provided?

    -In the context provided, the trapezoidal method involves taking the sum of the areas of multiple trapezoids under a curve to approximate the integral of a function. The 'height' of each trapezoid is considered as Δx (delta x), and the 'bases' are the function values at the endpoints of each interval.

  • What does Δx represent in the trapezoidal method?

    -Δx represents the width of each interval or the 'height' of each trapezoid in the context of the trapezoidal method.

  • How are the function values represented in the trapezoidal method?

    -The function values are represented as f(a) and f(b), where a and b are the endpoints of the intervals. These values act as the 'bases' of the trapezoids in the trapezoidal method.

  • What is the approximation formula for the area under the curve using the trapezoidal method?

    -The approximation formula for the area under the curve is the sum of the areas of each trapezoid, calculated as one-half times the sum of the function values at the endpoints of each interval times the interval width (Δx).

  • How can the trapezoidal method be simplified for uniform Δx?

    -For uniform Δx, the trapezoidal method can be simplified by factoring out the one-half and Δx from each term, leading to a simplified formula involving the first function value, twice the sum of the middle function values, and the last function value, all multiplied by one-half times Δx.

  • What is the significance of the first and last terms in the simplified trapezoidal formula?

    -The first and last terms in the simplified trapezoidal formula are not multiplied by two because they represent the 'ends' of the approximated area under the curve, where the trapezoid only has one base adjacent to the area being calculated.

  • Can the delta x be factored out if the intervals are not uniform?

    -No, Δx cannot be factored out if the intervals are not uniform, as the width of each trapezoid would vary, requiring individual calculation for each trapezoid's area.

  • What was the final numerical result obtained using the trapezoidal method in the transcript?

    -The final numerical result obtained was 11 over 32, after simplifying the calculation for the area under the curve using the trapezoidal method.

  • Why is understanding the trapezoidal method important in calculus?

    -Understanding the trapezoidal method is important in calculus for approximating the area under a curve, which is essential for solving definite integrals when an antiderivative cannot be easily found or when dealing with empirical data.

Outlines
00:00
🔢 Introduction to the Trapezoidal Method

This segment introduces the trapezoidal method for calculating area, starting with the basic formula for the area of a trapezoid. The speaker elaborates on applying this concept to approximate the area under a curve, transforming the classic trapezoid's height into the delta x (Δx), and the bases into function values at points a and b (f(a) and f(b)). A detailed step-by-step calculation of the trapezoidal approximation for a function is presented, emphasizing the iterative process of calculating individual trapezoid areas along the curve and the significance of uniform delta x in the calculation. The explanation aims to make students comfortable with transforming the geometric understanding of a trapezoid into its application in numerical integration, highlighting differences from other methods like the left-hand, right-hand, and midpoint rules.

05:02
🧮 Simplifying the Trapezoidal Method Calculation

This part focuses on simplifying the trapezoidal method calculation process. The speaker shows how to reduce the complex formula into a more manageable form by combining terms, factoring out constants, and applying a formula that includes the first term, twice the middle terms, and the last term. This simplification process is demonstrated through a specific example, leading to a more concise and less time-consuming method of calculation. The goal is to help students memorize and understand a streamlined approach for applying the trapezoidal method, making it easier to compute areas under curves without extensive manual calculations. The speaker also discusses common pitfalls in calculation, emphasizes the importance of uniform Δx, and encourages students to verify their work, closing with a corrected calculation that highlights the importance of precision in mathematical operations.

Mindmap
Keywords
💡Trapezoidal method
The trapezoidal method is a numerical technique used for estimating the definite integral of a function. It works by approximating the region under the curve of a function as a series of trapezoids, calculating the area of each, and summing these areas to get an approximation of the total area under the curve. In the context of the video, the trapezoidal method is explained as a practical approach to approximate integrals, emphasizing its stepwise construction and the importance of understanding the geometric representation of trapezoids in calculating areas.
💡Area of a trapezoid
The area of a trapezoid is given by the formula 1/2 * (base1 + base2) * height, where base1 and base2 are the lengths of the parallel sides, and height is the distance between these sides. This formula is foundational for the trapezoidal method discussed in the video, as it underpins the calculation of the area under the curve being approximated.
💡Delta x
Delta x represents the interval between consecutive points along the x-axis, which corresponds to the width of each trapezoid in the trapezoidal method. In the video, delta x is highlighted as a crucial element, transforming the conceptual height of a standard trapezoid into the width of each interval used in the approximation process, illustrating the adaptability of mathematical concepts to different contexts.
💡Function values
Function values, denoted as f(a) and f(b) or f of any given point, represent the outputs of the function at specific points. These values serve as the heights of the trapezoids in the trapezoidal method. The video emphasizes the importance of these values in calculating the area of each trapezoid, where they act as the 'bases' in the trapezoidal area formula adapted to numerical integration.
💡Uniform delta x
A uniform delta x means that the intervals between consecutive x-values are constant across the entire domain over which the trapezoidal method is applied. The video stresses the significance of having a uniform delta x for simplifying the formula by factoring out the common delta x value, which makes the calculation process more efficient and straightforward.
💡Approximation formula
The approximation formula is the mathematical expression used to calculate the approximate integral of a function using the trapezoidal method. It involves summing the areas of trapezoids formed under the curve of the function. The video walks through the derivation of this formula, emphasizing its distinction from other numerical methods and highlighting the iterative process of improving approximation accuracy.
💡Simplification
Simplification in the context of the video refers to the process of reducing the complexity of the trapezoidal method's formula by factoring out constants and combining similar terms. This process is illustrated with the goal of making the method more accessible and less time-consuming to apply, demonstrating practical strategies for managing mathematical expressions.
💡Factoring out
Factoring out involves extracting common factors from terms in an expression, a technique used in the video to streamline the trapezoidal method's approximation formula. By identifying constants and uniform intervals (delta x) that can be factored out, the formula is significantly simplified, underscoring the value of algebraic manipulation in mathematical problem-solving.
💡Common denominator
The common denominator refers to a shared denominator used in adding or subtracting fractions, crucial in the process of combining terms in the trapezoidal method's formula. The video includes finding a common denominator as a step in simplifying the final approximation, showcasing practical arithmetic skills alongside more advanced mathematical concepts.
💡Numerical integration
Numerical integration is the process of calculating an approximation of the integral of a function when an exact analytical solution is difficult or impossible to obtain. The trapezoidal method, as described in the video, is a form of numerical integration that approximates the area under a curve by dividing it into trapezoids, reflecting the method's practical application in solving real-world problems.
Highlights

Introduction to the trapezoidal method for calculating area.

Explanation of the area formula for a trapezoid.

Demonstration of how trapezoids can approximate areas under curves.

Visual representation of trapezoids showing minimal error in approximation.

Conversion of trapezoid dimensions to function values for calculation.

Detailed step-by-step breakdown of the trapezoidal approximation method.

Introduction of the concept of delta x in the context of trapezoidal approximation.

Explanation of how function values are used as bases in the trapezoidal method.

The process of calculating area for multiple trapezoids under a curve.

Discussion on factoring out constants in the trapezoidal method formula.

Simplification of the trapezoidal method formula for easier computation.

Introduction to a more efficient formula for the trapezoidal method.

Example calculation using the trapezoidal method formula.

Clarification and correction of calculation mistakes.

Final approximation result of the trapezoidal method calculation.

Transcripts

Browse More Related Video

Estimating areas using trapezoidal rule [IB Maths AI SL/HL]

Trapezoidal sums | Accumulation and Riemann sums | AP Calculus AB | Khan Academy

Riemann and Trapezoidal Sums from Tables of Values

The Trapezoid Rule for Approximating Integrals

AP Calculus AB: Lesson 6.2 Riemann and Trapezoidal Sums

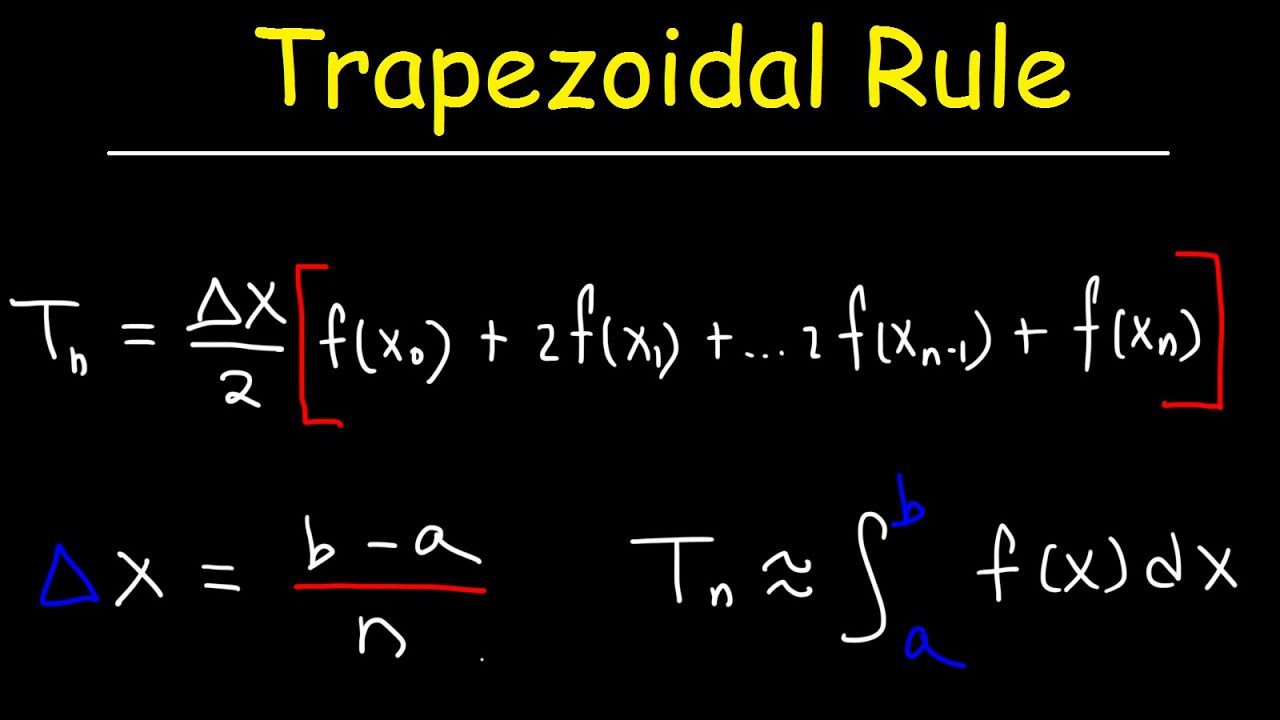
Trapezoidal Rule

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Trapezoidal MethodMathematicsEducationalArea ApproximationInteractive LearningFunction AnalysisCalculusClassroom DiscussionMath TheoryStudent Engagement