Simpson's Rule: Deriving the Basic Form (1 of 2)

Eddie Woo
16 Oct 201312:23
EducationalLearning
32 Likes 10 Comments

TLDRIn this enlightening video, the speaker dives into the topic of approximating areas under curves, crucial for understanding integration. Starting with a refresher on Riemann's method of rectangles and the trapezoidal rule, the presenter explores their limitations due to their linear nature. The focus then shifts to the challenge of approximating curved areas, highlighting the utility of parabolas over circles for their ease in calculation and relevance to the problem at hand. By detailing the process of using three points to define a unique parabola for approximation, the video simplifies complex mathematical concepts into understandable segments. This approach not only aids in grasping the concept of integration better but also demonstrates the practical application of parabolas in calculating areas under curves, making the content both engaging and educational.

Takeaways
  • 📚 The script discusses two methods of approximating areas under curves: Riemann's method using rectangles and the trapezoidal rule.
  • 📈 Riemann's method involves using rectangles to approximate the area under a curve and then applying limits to find the exact area.
  • ➡️ The trapezoidal rule approximates the area under a curve by using trapeziums, offering a ballpark figure for the area.
  • 🔫 Shapes like circles and parabolas are considered for approximating curved areas, but polygons are inadequate due to their straight sides.
  • ⚫️ The focus shifts to using parabolas for approximation, as they can closely match the curvature of many functions.
  • ✅ Three points are essential to uniquely define a parabola, similar to how three points can uniquely define a circle.
  • 📖 The midpoint (average of two points on the x-axis) is crucial for drawing a parabola that approximates the area under a curve.
  • 📉 A geometric approach is employed to simplify the approximation process, including translating the parabola to the origin for easier calculation.
  • 📝 The area under a curve can be approximated by calculating the area under a parabola, with adjustments made for simplicity.
  • ⤴️ The approximation will be exact if the original function is a parabola or a cubic function; otherwise, there will be some margin of error.
Q & A

  • What are the two methods of approximation discussed in the script?

    -The two methods of approximation discussed are Riemann's method, which involves using rectangles, and the trapezoidal rule, which uses trapeziums.

  • Why are rectangles and trapeziums not ideal for approximating areas under curves?

    -Rectangles and trapeziums are not ideal for approximating areas under curves because they have straight sides, whereas curves require a shape that can mimic their curvature.

  • What shape is suggested as a better alternative for approximating curved areas, and why?

    -A parabola is suggested as a better alternative for approximating curved areas because it has a curved shape that can more closely match the curvature of the function being approximated.

  • How many points are needed to uniquely define a parabola, and why?

    -Three points are needed to uniquely define a parabola because, similar to a circle, any three points can determine a unique parabolic shape, whereas two points could correspond to an infinite number of parabolas.

  • What is the significance of the midpoint in the method of approximation using a parabola?

    -The midpoint is significant because it provides the third necessary point (in addition to the two endpoints) to uniquely define the parabola for the approximation.

  • Why is it unnecessary to match the parabola exactly to the function outside the interval of interest?

    -It is unnecessary to match the parabola exactly to the function outside the interval of interest because the approximation is only concerned with the area under the curve between the two specific points (a and b), not the entire function.

  • What geometric transformation is applied to the parabola to simplify the approximation process?

    -The parabola is translated to the origin, which simplifies the approximation process by making the algebra involved easier to handle.

  • What is the advantage of moving the parabola to the origin for approximation?

    -Moving the parabola to the origin makes the calculation of the area under the curve algebraically simpler, facilitating an easier and more straightforward approximation process.

  • In which cases would the parabolic approximation yield an exact result?

    -The parabolic approximation would yield an exact result if the function being approximated is itself a parabola or a cubic function.

  • What is the primary goal of these methods of approximation?

    -The primary goal of these methods of approximation is to simplify the process of calculating the area under a curve, making it more accessible and manageable.

Outlines
00:00
📈 Introduction to Approximation Methods and Riemann's Rectangles

This paragraph introduces the concept of approximation methods, specifically focusing on integration and areas under curves. It begins by recalling the two methods of approximation previously discussed: Riemann's rectangles and the trapezoidal rule. Riemann's method involves creating rectangles under the curve and using limits to find the exact area. The trapezoidal rule is introduced as a way to approximate areas using trapezium shapes. The speaker also touches on the limitations of these methods when dealing with curved areas, hinting at the need for alternative approaches like using circles to approximate non-polygonal shapes. The introduction sets the stage for exploring a better way to approximate curved areas, specifically mentioning the use of parabolas as the next step.

05:02
📊 Utilizing Parabolas for Area Approximation

The speaker delves into the use of parabolas as a means to approximate areas under curves more accurately than rectangles or trapezoids. It is explained that while rectangles and trapezoids are limited due to their straight edges, parabolas, being curved, can better fit the shape of the area under a curve. The process of using a parabola involves finding three points through which the parabola can be uniquely defined, similar to how three points define a circle. The speaker then discusses the process of moving the parabola to a position where it can be more easily integrated, specifically translating it to the origin for simplicity. This paragraph sets up the groundwork for the mathematical manipulation and integration of the parabola to find the approximate area under the curve.

10:03
🔢 Algebraic Simplification and Area Calculation

In this paragraph, the speaker continues the discussion on approximating areas under curves using parabolas. The focus shifts to the algebraic aspect of the process, emphasizing the importance of simplifying the calculation. The speaker introduces a general form for the parabola, denoted as 'p', and explains that the exact area under the curve can be approximated by the area under this parabola. It is noted that the approximation will be exact in certain cases, such as when the function itself is a parabola or a cubic function. The speaker also acknowledges that there will be a margin of error when approximating other types of functions. The paragraph concludes with the speaker preparing to calculate the area under the parabola, indicating a transition to the next phase of the approximation process.

Mindmap
Keywords
💡Integration
Integration is a fundamental concept in calculus that deals with finding the area under curves. In the context of the video, integration is described as the underlying principle for approximating areas under curves. The video outlines different methods of approximation, like Riemann sums and the trapezoidal rule, before introducing a novel approach using parabolas. Integration is central to understanding these methods, as it provides a way to precisely calculate areas that would otherwise be difficult to measure.
💡Riemann Sums
Riemann sums are a method for approximating the total area under a curve. This concept is introduced early in the video as the 'first wave' of approximation methods. By breaking the area into small rectangles and summing their areas, Riemann sums provide a way to approximate the integral of a function. The video discusses Riemann's approach as foundational for understanding more complex methods of approximation, illustrating its significance in the study of integration.
💡Trapezoidal Rule
The trapezoidal rule is another method for approximating the area under a curve, discussed as the 'way we learned yesterday' in the video. Instead of rectangles, this method uses trapezoids, which can provide a closer approximation to curved areas compared to rectangles. The video highlights the trapezoidal rule as an effective but simple technique for approximation, especially in cases where the curve does not align well with rectangular shapes.
💡Parabola
A parabola is a symmetrical, curved shape that is the focus of the video's new method for approximating areas under curves. The presenter suggests using parabolas to approximate curved areas because their shape can more closely match that of many curves compared to rectangles or trapezoids. The discussion of parabolas is essential for introducing the method of approximation that requires three points to define a unique parabola, demonstrating a more sophisticated approach to approximation.
💡Approximation
Approximation refers to the process of finding a value or shape that is close to, but not exactly, the true value or shape. In the video, approximation is a recurring theme as it explores various methods to estimate the area under curves. Each method, from Riemann sums to the use of parabolas, represents a different strategy for approximation, with the goal of simplifying complex shapes into more manageable forms for calculation.
💡Curves
Curves are discussed extensively in the video as the main challenge in calculating areas under them through integration. The narrator points out the difficulty of using straight-edged shapes like rectangles and trapezoids to approximate areas under curves due to their inherent straight lines. The exploration of curves leads to the introduction of parabolas as a more fitting shape for approximation, highlighting the complexity of dealing with curved geometries in mathematics.
💡Function Values
Function values are mentioned in the context of using them to define the points necessary for drawing a parabola for approximation. The video explains that unlike the trapezoidal rule, which requires two function values, a parabola-based method needs three. This concept is crucial for understanding how specific points on a curve are used to determine a parabola that closely matches the area to be approximated.
💡Geometric Tricks
Geometric tricks refer to the strategies discussed in the video for simplifying the approximation process. These include using a parabola for a closer fit to the curve being approximated and translating the parabola to the origin to simplify calculations. These tricks highlight the presenter's aim to make the approximation of areas under curves as straightforward and accurate as possible, using geometric properties and transformations.
💡Algebraic Simplicity
Algebraic simplicity is a goal mentioned in the context of finding an equivalent area that is easier to calculate. The video discusses translating the parabola to the origin as a means to achieve algebraic simplicity, which would make the mathematical process of calculating the area more straightforward. This concept is key to understanding the motivation behind the approximation methods presented, emphasizing the desire to reduce complex calculations to simpler forms.
💡Definite Integral
The definite integral is ultimately what the video's discussion of approximation methods aims to calculate or estimate. It represents the exact area under a curve between two points. The video connects the various approximation methods, including the novel parabola approach, back to the goal of calculating a definite integral, emphasizing its importance in understanding the relationship between geometry and algebra in calculus.
Highlights

Introduction to two methods of approximation in integration and areas under curves.

Explanation of Riemann's method using rectangles to approximate areas under curves.

Introduction to the trapezoidal rule as a method for approximating area.

Discussion on the limitations of using polygons like rectangles and trapeziums for approximating curved areas.

The idea that circles and parabolas, due to their curves, are more suitable for approximating areas under curves than polygons.

Explanation of how three points uniquely define a circle, illustrating the concept with chord properties.

Parallel drawn between the uniqueness of a circle defined by three points and a parabola defined by three points.

Introduction of the concept of using a parabola to approximate the area under a curve, with a focus on the need for three specific points for accuracy.

Explanation of finding the midpoint in an interval for better approximation using a parabola.

The process of approximating the area under a curve with a parabola, focusing on the interval of interest.

Discussion on geometric tricks to simplify the approximation process, such as moving the parabola to the origin.

Introduction to algebraic simplification in approximation methods by translating the problem to a simpler form.

Definition of a general form of a parabola for approximation purposes, avoiding confusion with interval bounds.

Explanation that the area under a given function can be approximately equal to the area under a parabola over the same interval.

Mention of special cases where the approximation by a parabola is exact, including when approximating cubic functions.

Discussion on the inherent margin of error in approximations when the functions involved are not parabolic or cubic.

Transcripts

Browse More Related Video

What is Calculus - Lesson 4 | Integration | Don't Memorise

Explanation of Simpson's rule | MIT 18.01SC Single Variable Calculus, Fall 2010

AP Calculus AB - 6.2 Approximating Areas with Riemann Sums

4.4 - Properties of Definite Integrals

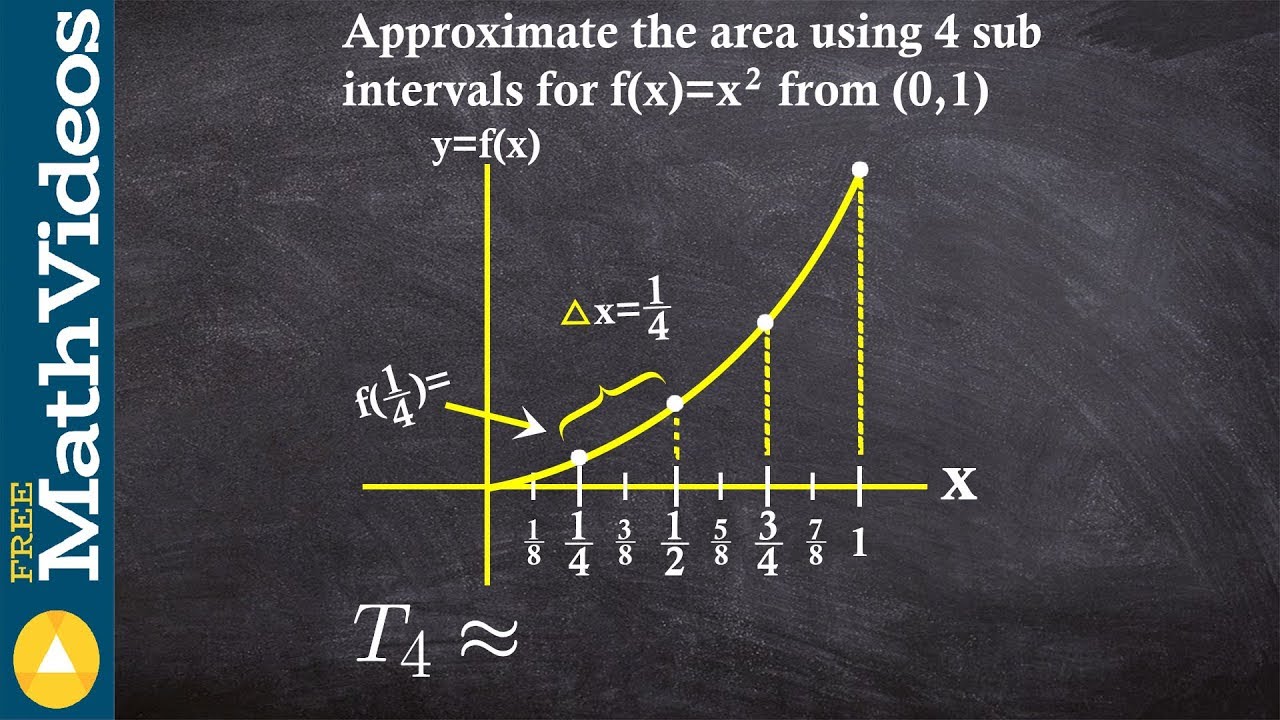
Learn how to use the trapezoidal rule with 4 sub intervals

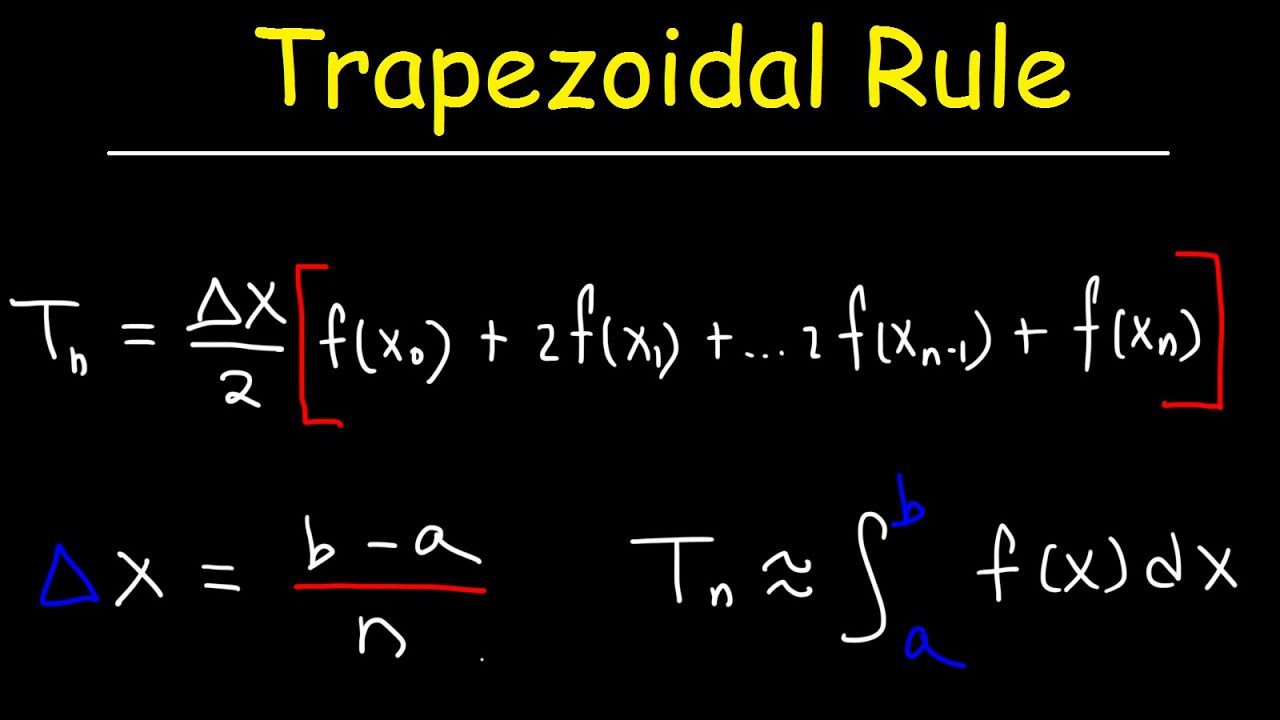
Trapezoidal Rule

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsIntegrationApproximationParabolasRiemannTrapezoidal RuleArea CalculationGeometryAlgebraLogarithmic Functions