2011 Calculus AB free response #2 (c & d) | AP Calculus AB | Khan Academy

Khan Academy
7 Sept 201107:27
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the application of the second fundamental theorem of calculus to evaluate definite integrals, using the context of temperature changes over time for both tea and biscuits. It explains how the definite integral of a function's derivative over an interval represents a change in temperature, calculating the specific temperature drop for both items over 10 minutes. The script concludes with a comparison, showing the biscuits are 8.82 degrees Celsius cooler than the tea after the specified time.

Takeaways
  • 📚 The definite integral from 0 to 10 of H'(t)dt represents the change in temperature over a 10-minute period.
  • 🔢 Evaluating the integral involves finding the anti-derivative of H(t) at 10 and subtracting it at 0, resulting in a temperature difference.
  • 🌡️ The temperature change is given by the final temperature minus the initial temperature, leading to a decrease of 23 degrees Celsius.
  • 🍪 The problem transitions from discussing tea to biscuits, with a focus on their temperature change over time.
  • 📈 The temperature of the biscuits is modeled by a differentiable function B(t) with a known derivative B'(t).
  • 🧮 To find the temperature of the biscuits at time t=10, we calculate the definite integral from 0 to 10 of B'(t)dt.
  • 🔍 The derivative B'(t) is given as -13.84e^(-0.173t), which we use to find the anti-derivative and thus the temperature change.
  • 📊 Evaluating the anti-derivative yields a change in temperature of -65.82 degrees Celsius for the biscuits over 10 minutes.
  • 🤔 By comparing the final temperatures of the biscuits (34.18 degrees Celsius) and the tea (43 degrees Celsius), we find a difference of 8.82 degrees Celsius.
  • 🎓 The biscuits are 8.82 degrees Celsius cooler than the tea after 10 minutes, as per the given models and calculations.
  • 👨‍🏫 The process demonstrates the application of the second fundamental theorem of calculus in real-world temperature change problems.
Q & A

  • What is the main concept discussed in the script?

    -The main concept discussed in the script is the application of the second fundamental theorem of calculus to evaluate definite integrals and understand their physical meaning in the context of temperature changes over time.

  • How is the definite integral from 0 to 10 of H prime of (t)dt interpreted in the context of temperature?

    -The definite integral from 0 to 10 of H prime of (t)dt represents the change in temperature over the 10-minute period, calculated as the temperature at 10 minutes minus the temperature at 0 minutes.

  • What is the temperature of the tea after 10 minutes according to the script?

    -The temperature of the tea after 10 minutes is 43 degrees Celsius.

  • How is the temperature of the biscuits modeled at time t?

    -The temperature of the biscuits at time t is modeled by a differentiable function B, for which the derivative B prime of t is given as a specific function.

  • What is the formula for the derivative of e to the ax?

    -The derivative of e to the ax is ae to the ax, which comes from the chain rule of differentiation.

  • How is the integral of e to the ax dx evaluated?

    -The integral of e to the ax dx is evaluated as 1 over a times e to the ax plus c, where c is the constant of integration.

  • What is the final calculated change in temperature for the biscuits over 10 minutes?

    -The final calculated change in temperature for the biscuits over 10 minutes is a decrease of 65.82 degrees Celsius.

  • What is the temperature of the biscuits after 10 minutes based on the calculation?

    -After 10 minutes, the temperature of the biscuits is 34.18 degrees Celsius.

  • How much cooler are the biscuits than the tea at time t equals 10?

    -At time t equals 10, the biscuits are 8.82 degrees Celsius cooler than the tea.

  • What is the significance of the negative sign in the calculated change in temperature?

    -The negative sign in the calculated change in temperature indicates a decrease in temperature over the given time period.

  • How does the script demonstrate the use of the second fundamental theorem of calculus in solving real-world problems?

    -The script demonstrates the use of the second fundamental theorem of calculus by applying it to evaluate definite integrals that represent changes in temperature over time for both tea and biscuits, showing how mathematical concepts can be used to understand and solve practical problems.

Outlines
00:00
📚 Calculus Fundamentals and Temperature Change

This paragraph discusses the application of the second fundamental theorem of calculus to evaluate a definite integral, which in this context, represents the change in temperature over a 10-minute period. The explanation includes the process of finding the anti-derivative of the given function, applying the bounds of integration (0 to 10 minutes), and interpreting the result as the temperature difference. It is noted that the temperature drops from 66 degrees Celsius at 0 minutes to 43 degrees Celsius at 10 minutes, indicating a decrease of 23 degrees Celsius.

05:00
🍪 Biscuit Cooling Dynamics and Comparison

The second paragraph delves into the cooling dynamics of biscuits, starting from an initial temperature of 100 degrees Celsius, and uses a differentiable function to model the temperature change over time. The calculation involves finding the definite integral from 0 to 10 minutes for the given function, which represents the change in temperature for the biscuits. The integral is evaluated using the anti-derivative of the function, which includes an exponential term with a coefficient. The final result indicates a temperature decrease of 65.82 degrees Celsius over the 10 minutes, leading to a final temperature of 34.18 degrees Celsius for the biscuits. This is then compared to the temperature of the tea, which is 43 degrees Celsius after the same time period, showing that the biscuits are 8.82 degrees Celsius cooler than the tea.

Mindmap
Keywords
💡Definite Integral
The definite integral is a fundamental concept in calculus that represents the accumulated quantity of a variable over a given interval. In the context of the video, it is used to calculate the change in temperature over a 10-minute period for both tea and biscuits. The definite integral of a function from 0 to 10 is the difference in the anti-derivative (or the area under the curve) evaluated at the upper limit (10) and the lower limit (0).
💡Anti-derivative
The anti-derivative, also known as the indefinite integral, is the reverse process of differentiation. It is used to find the original function from its derivative. In the video, the anti-derivative of the temperature function is used to determine the temperature at a specific time by integrating the rate of temperature change.
💡Second Fundamental Theorem of Calculus
The Second Fundamental Theorem of Calculus establishes a direct relationship between differentiation and integration. It states that the definite integral of a function's derivative over an interval can be computed as the difference between the values of the original function at the endpoints of the interval. This theorem is crucial for evaluating definite integrals in the video, as it allows the calculation of temperature changes over time.
💡Temperature Change
Temperature change refers to the difference in temperature between two points in time. In the video, it is the primary focus, as the problem involves calculating the change in temperature for both tea and biscuits over a 10-minute interval. The temperature change is found by subtracting the initial temperature from the temperature at a later time.
💡Biscuits
In the context of the video, biscuits refer to the subject of the temperature change problem. The problem models the temperature of the biscuits as a function of time, B(t), and uses calculus to determine how much cooler the biscuits become compared to the tea after 10 minutes.
💡Tea
Tea is used as a comparative example in the temperature change problem. The script discusses the temperature change of tea over a 10-minute interval and compares it to the temperature change of biscuits to determine which has cooled more.
💡Derivative
The derivative is a mathematical concept that represents the rate of change of a function with respect to its independent variable. In the video, the derivative is used to model the rate of temperature change for both the tea and the biscuits.
💡Chain Rule
The chain rule is a fundamental calculus technique used to find the derivative of a composite function. It states that the derivative of a function composed of multiple functions is the product of the derivative of the outer function and the derivative of the inner function. In the video, the chain rule is mentioned when discussing the derivative of an exponential function.
💡Integral of Exponential Function
The integral of an exponential function e to the ax dx is a fundamental result in calculus. It states that the integral is equal to 1 over a times e to the ax plus a constant c. This result is used in the video to find the anti-derivative of the rate of temperature change function for the biscuits.
💡Evaluation
Evaluation in mathematics refers to the process of finding the value of a function or expression at a specific point. In the video, evaluation is used to determine the temperature at specific times by plugging in values for the variables into the derived functions.
💡Temperature
Temperature is a physical quantity that measures the degree of hotness or coldness of an object. In the video, temperature is the variable of interest, and the goal is to calculate the change in temperature over time for both tea and biscuits.
💡Comparison
Comparison is the act of contrasting two or more items to determine their similarities or differences. In the video, the comparison is made between the temperature of the tea and the biscuits after 10 minutes to determine which has cooled more.
Highlights

The definite integral from 0 to 10 of H prime of (t)dt represents the change in temperature over the first 10 minutes.

The temperature change is calculated by evaluating the anti-derivative of H(t) at 10 and subtracting it from the anti-derivative at 0.

The temperature at 10 minutes for the tea is 43 degrees Celsius.

The initial temperature of the tea at 0 minutes is 66 degrees Celsius.

The change in temperature for the tea over 10 minutes is a decrease of 23 degrees Celsius.

The problem switches from discussing tea to biscuits, which have a different temperature model.

The temperature of the biscuits at time t is modeled by a differentiable function B(t).

The derivative B prime of t is given as a function involving an exponential term and a linear term.

The change in temperature for the biscuits over 10 minutes is calculated using the definite integral from 0 to 10 of B prime of (t)dt.

The anti-derivative of B(t) is found by applying the chain rule and properties of exponential functions.

The temperature of the biscuits at 10 minutes is found by subtracting the change in temperature from the initial temperature of 100 degrees Celsius.

After 10 minutes, the biscuits' temperature is 34.18 degrees Celsius.

Comparing the temperatures, the biscuits are 8.82 degrees Celsius cooler than the tea after 10 minutes.

The problem demonstrates the application of the second fundamental theorem of calculus in practical scenarios, such as temperature changes over time.

The use of calculus to model real-world phenomena, like cooling processes, is highlighted.

The process of evaluating definite integrals and applying them to find differences in temperature is clearly outlined.

The problem showcases the importance of understanding the context and units in mathematical modeling.

Transcripts

Browse More Related Video

2011 Calculus AB free response #2 (a & b) | AP Calculus AB | Khan Academy

2011 AP Calculus AB Free Response #2

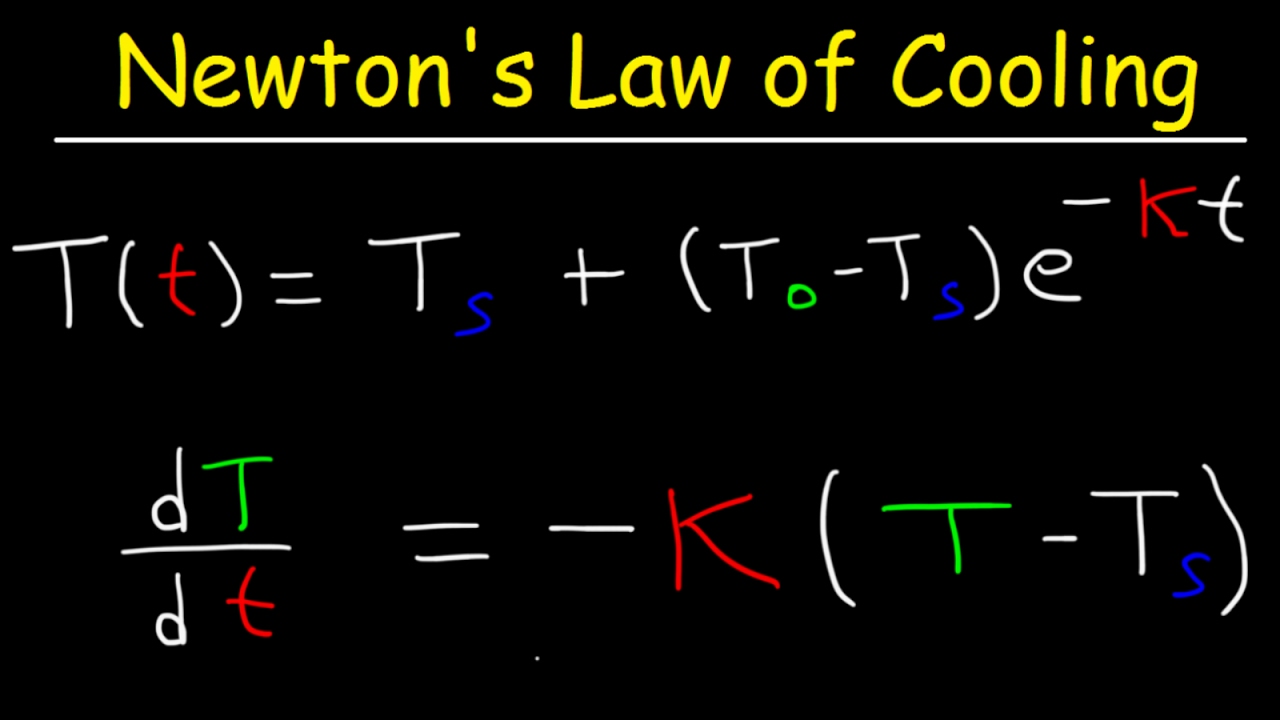
Newton's Law of Cooling Calculus, Example Problems, Differential Equations

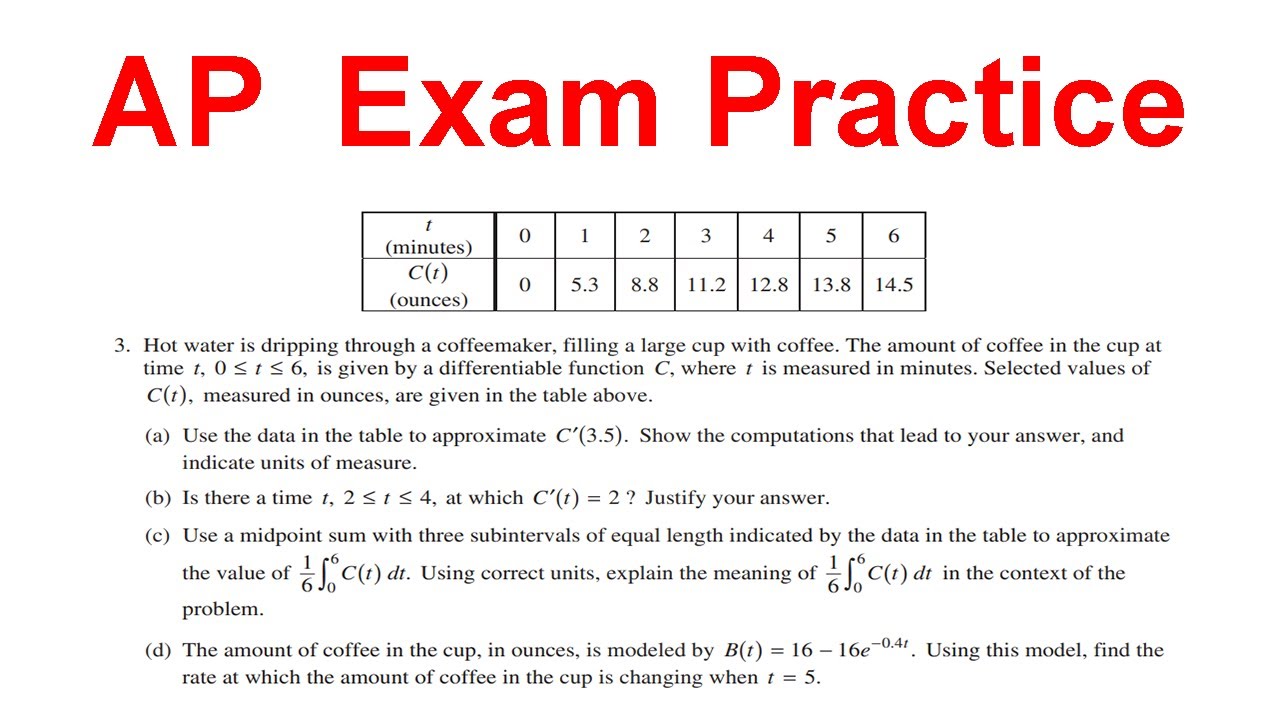
How to solve Table of Values Questions on the AP Calc AB Exam

Fundamental theorem of calculus (Part 2) | AP Calculus AB | Khan Academy

Definite Integrals!

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus ApplicationTemperature DynamicsDefinite IntegralsChange in TemperatureTea Cooling AnalysisBiscuit TemperatureMathematical ModelingHeat TransferThermal AnalysisCooking Science