2011 Calculus AB free response #2 (a & b) | AP Calculus AB | Khan Academy
TLDRThe video script discusses the mathematical modeling of the temperature change of a pot of tea as it cools, using a differentiable function h(t). It illustrates how to approximate the rate of temperature change at a specific time (t=3.5 minutes) by calculating the slope between two data points and arrives at a rate of negative 8 3rds degrees Celsius per minute. Furthermore, the script explains the concept of a definite integral in this context as the area under the curve representing the temperature change over time, and uses the trapezoidal sum method with four sub-intervals to estimate the average temperature over the 10-minute period, resulting in an approximate value of 52.95 degrees Celsius.
Takeaways
- 📉 The problem involves modeling the temperature of cooling tea as a function h(t), where t is time in minutes and h(t) is the temperature in degrees Celsius.
- 📈 Given data points of temperature at different times are used to approximate the rate of temperature change at t=3.5 minutes.
- 🤔 To approximate the rate of change, the slope between the temperatures at t=2 minutes and t=5 minutes is calculated.
- 📊 A graph is plotted to visualize the temperature change over time, showing the temperature decrease from 66°C at t=0 to 43°C at t=10 minutes.
- 🔽 The rate of change at t=3.5 minutes is found to be approximately -8 1/3 degrees Celsius per minute, indicating the temperature is decreasing.
- 🌐 Part B of the problem asks to explain the meaning of (1/10) times the definite integral from 0 to 10 of h(t) dt, using the provided data.
- 🏆 The definite integral from 0 to 10 of h(t) dt represents the area under the curve of the temperature function over the 10-minute period.
- 📝 (1/10) times this integral gives the average temperature over the 10-minute period, which is a measure of the overall cooling trend.
- 🔍 The trapezoidal sum method is used to approximate the integral by dividing the area under the curve into four trapezoids, each with a different base and height.
- 🧩 Each trapezoid's area is calculated by taking the average height and multiplying it by the base, then summing these areas to approximate the total area under the curve.
- 📌 The estimated average temperature over the 10 minutes is found to be 52.95 degrees Celsius after applying the trapezoidal sum and dividing by 10.
Q & A
What is the main topic of the transcript?
-The main topic of the transcript is the process of approximating the rate of change of temperature of cooling tea and calculating the average temperature over a given time period using mathematical techniques.
What is the time frame for the temperature data provided in the transcript?
-The time frame for the temperature data is from 0 to 10 minutes.
How is the temperature of the tea at time t equal to 3.5 minutes approximated in the transcript?
-The temperature change rate at time t equal to 3.5 minutes is approximated by calculating the slope between the temperature data points at 2 and 5 minutes, resulting in a negative 8 3rds degrees Celsius per minute.
What does the definite integral from 0 to 10 of h(t) dt represent in the context of the problem?
-The definite integral from 0 to 10 of h(t) dt represents the area under the curve of the temperature function h(t) over the time period, which corresponds to the total change in temperature of the tea.
How is the average temperature over the time period calculated?
-The average temperature is calculated by dividing the definite integral (area under the curve) by the total time elapsed, which is 10 minutes in this case.
What is the trapezoidal sum method mentioned in the transcript?
-The trapezoidal sum method is a numerical technique used to approximate the definite integral by dividing the area under the curve into trapezoidal sections and summing the areas of these trapezoids.
How many sub-intervals are used in the trapezoidal sum to estimate the integral?
-Four sub-intervals are used in the trapezoidal sum to estimate the integral.
What are the units for the average temperature calculated using the integral?
-The units for the average temperature calculated using the integral are degrees Celsius.
What is the estimated value of the definite integral using the trapezoidal sum method?
-The estimated value of the definite integral using the trapezoidal sum method is 529.5.
What is the final approximate value for the average temperature over the 10-minute period?
-The final approximate value for the average temperature over the 10-minute period is 52.95 degrees Celsius.
How does the visual representation of the temperature data help in understanding the problem?
-The visual representation of the temperature data as a graph helps in understanding the problem by providing a clear picture of how the temperature changes over time, making it easier to visualize the curve and the areas of interest for the calculations.
Outlines
📉 Temperature Change Rate Calculation
This paragraph discusses the process of approximating the rate at which the temperature of a cooling pot of tea is changing at a specific time, t equals 3.5 minutes. It explains the use of a differentiable function h(t) to model the temperature as a function of time, with given temperature values at selected times. The paragraph walks through the steps of graphing the data points, visualizing the temperature curve, and then calculating the slope of the curve at the specified time to determine the rate of temperature change. The computation involves finding the difference in temperature over a three-minute interval and dividing it by the time interval to approximate the rate of change in degrees Celsius per minute. The result is a rate of negative 8 1/3 degrees Celsius per minute at time t equals 3.5 minutes.
📊 Approximating the Definite Integral Using Trapezoidal Sum
The second paragraph focuses on the estimation of a definite integral from 0 to 10 minutes of the temperature function h(t), which represents the area under the curve of the cooling tea's temperature over time. Since the exact function is not known, the method of trapezoidal sum is used to divide the area into four trapezoids, each corresponding to the sub-intervals given in the table. The average height of each trapezoid is calculated by taking the average of the temperatures at the start and end of each sub-interval, and then the area of each trapezoid is found by multiplying the base (the time interval) by this average height. The areas of all four trapezoids are summed to approximate the definite integral. Finally, this value is divided by 10 to find the average temperature over the 10-minute period, which is found to be 52.95 degrees Celsius.
Mindmap
Keywords
💡temperature
💡differentiable function
💡rate of change
💡slope
💡definite integral
💡trapezoidal sum
💡average temperature
💡time interval
💡data table
💡graph
💡calculus
Highlights
The problem involves modeling the temperature of a pot of tea as it cools using a differentiable function h(t), where t is time in minutes and h(t) is the temperature in degrees Celsius.
The temperature data is given for specific time points: 0, 2, 5, 9, and 10 minutes.
At time zero, the temperature is 66 degrees Celsius, and it decreases as time progresses.
The task is to approximate the rate of temperature change at t=3.5 minutes using the provided data.
The rate of change is represented by the slope of the curve at time t=3.5 minutes.
The slope is approximated by finding the change in temperature over the three minutes centered at t=3.5.
The temperature at t=5 minutes is 52 degrees Celsius and at t=2 minutes is 60 degrees Celsius.
The approximate rate of temperature change at t=3.5 minutes is -8 1/3 degrees Celsius per minute.
The second part of the problem involves understanding the meaning of the definite integral of h(t) from 0 to 10 when divided by 10.
The integral represents the area under the curve of the temperature function over the 10-minute period.
Dividing the integral by 10 gives the average temperature over the 10 minutes.
The average temperature is calculated by integrating the temperature function and dividing by the total elapsed time.
The units for the average temperature remain in degrees Celsius, as the time component is canceled out.
A trapezoidal sum is used to estimate the definite integral, dividing the area under the curve into four trapezoids.
The first trapezoid's area is calculated using the heights of 66 and 60 degrees Celsius.
The second trapezoid's area is based on the heights of 60 and 52 degrees Celsius.
The third trapezoid uses the heights of 52 and 44 degrees Celsius.
The fourth and final trapezoid is based on the heights of 44 and 43 degrees Celsius.
The sum of the areas of the four trapezoids provides an approximation for the definite integral.
After dividing by 10, the estimated average temperature over the 10 minutes is 52.95 degrees Celsius.
Transcripts
Browse More Related Video

2011 Calculus AB free response #2 (c & d) | AP Calculus AB | Khan Academy
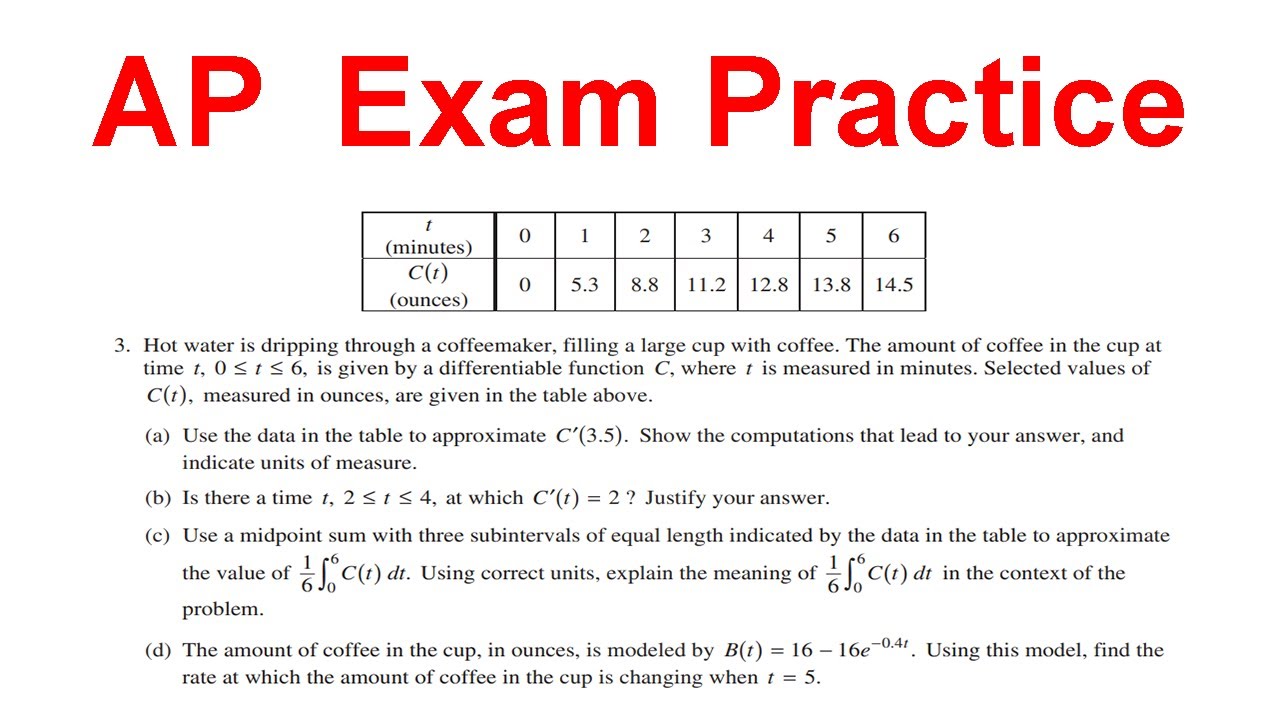
How to solve Table of Values Questions on the AP Calc AB Exam
2012 AP Calculus AB Free Response #1

2013 AP Calculus AB Free Response #3

2011 AP Calculus AB Free Response #2
Newton's Law of Cooling Calculus, Example Problems, Differential Equations
5.0 / 5 (0 votes)
Thanks for rating: