Cover-Up Method for Partial Fraction Decomposition

turksvids
4 Nov 201709:22
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial introduces the 'cover-up method' for partial fraction decomposition, a technique applicable to non-repeated linear factors. It demonstrates the process through examples, showing how to simplify complex fractions by equating coefficients and solving for unknowns. The method is efficient, skipping the need to clear the denominator entirely, and is particularly useful in calculus courses, making problem-solving quicker and more straightforward.

Takeaways
  • 🔍 The video discusses the cover-up method for partial fraction decomposition.
  • ℹ️ The cover-up method works on non-repeated linear factors, such as ax + b to the first power.
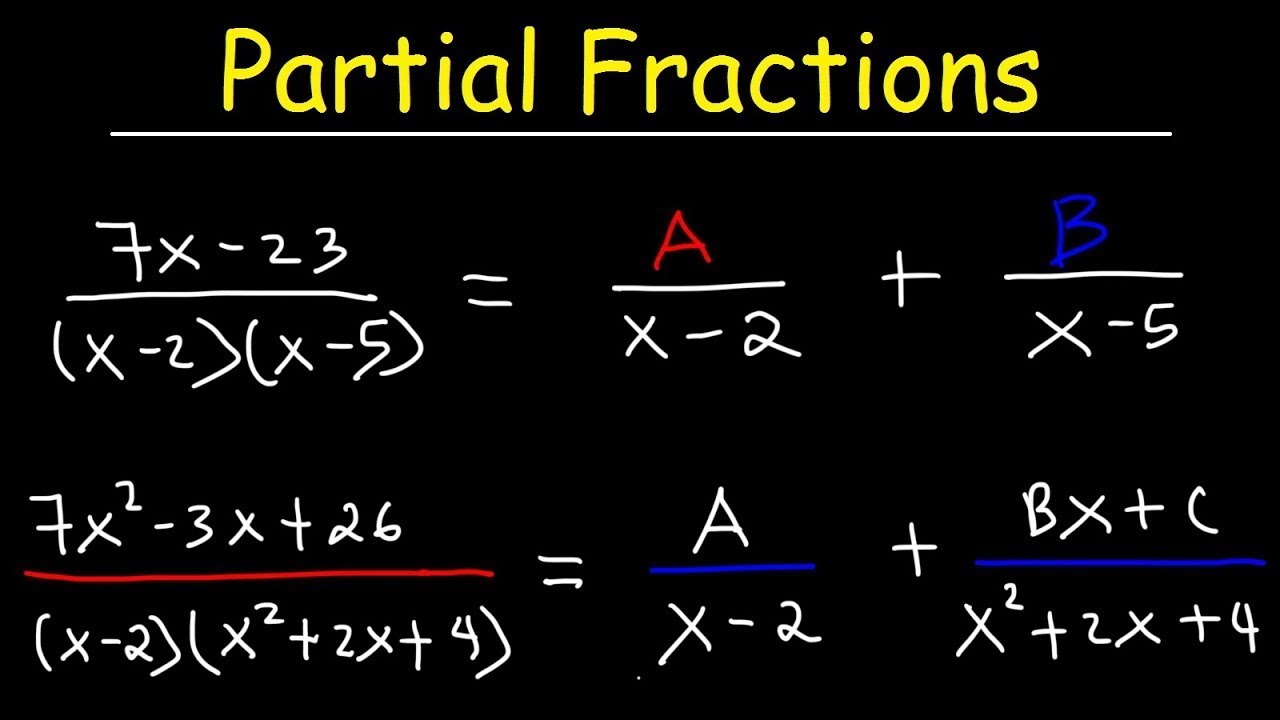
  • ➗ The example used starts with a partial fraction decomposition of 3x - 4 over (x - 3)(2x + 1).
  • 🧮 Traditional method involves clearing the denominator, expanding, and equating coefficients.
  • 📊 Using the system of equations, values for A and B are determined as 5/7 and 11/7 respectively.
  • 🔄 The cover-up method simplifies the process by focusing on individual factors and substituting their zeros.
  • ✅ By letting x equal the zero of a factor, one can solve for the corresponding variable directly.
  • 🚀 The method is faster and more efficient, especially with multiple linear factors.
  • 📉 The video provides multiple examples to illustrate the method, including one with three factors.
  • 💡 The cover-up method is useful in calculus, especially in Calc BC courses, for simplifying complex fraction decomposition problems.
Q & A

  • What is partial fraction decomposition?

    -Partial fraction decomposition is a method used in calculus to break down a complex rational function into simpler fractions, making it easier to integrate or differentiate.

  • What is the cover-up method?

    -The cover-up method is a specific technique within partial fraction decomposition that simplifies the process by strategically multiplying and substituting values to isolate and solve for unknown coefficients.

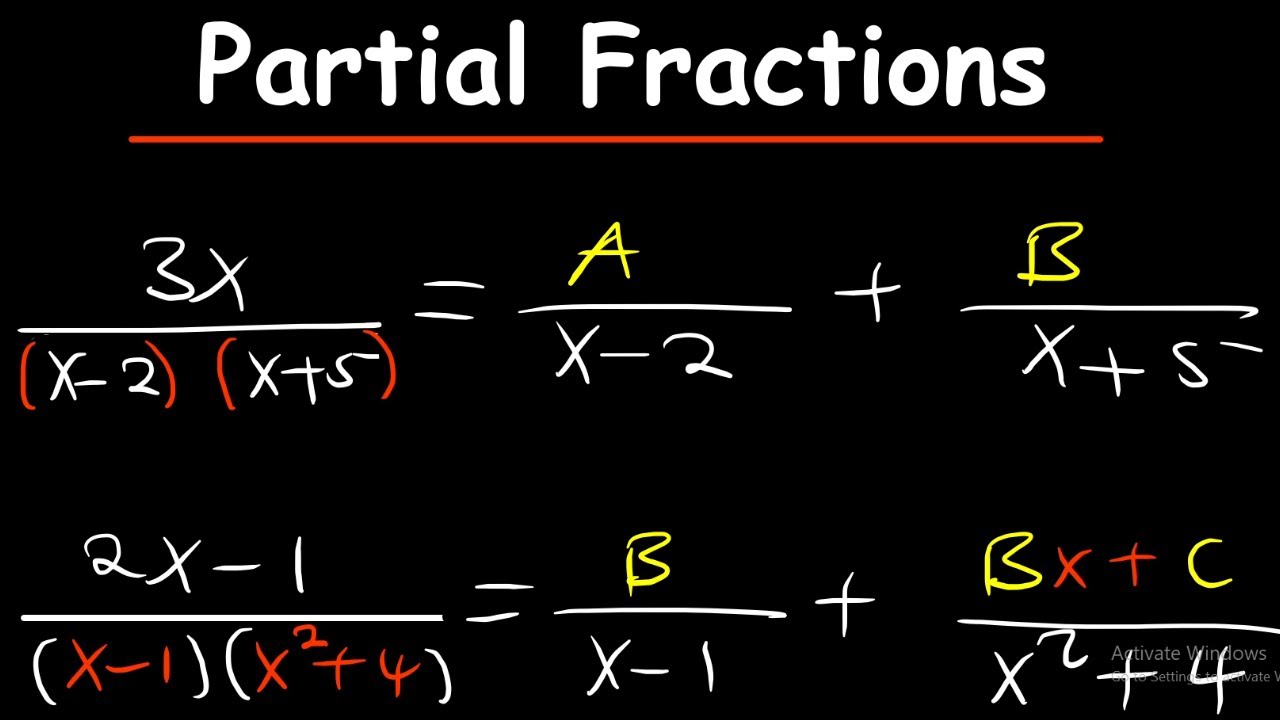
  • What are non-repeated linear factors?

    -Non-repeated linear factors are terms in the denominator of a rational function that are of the form ax + b, where a and b are constants, and the factor is not repeated.

  • Why is the denominator always in factored form in partial fraction decomposition?

    -The denominator must be in factored form to ensure that each term can be decomposed into a simple fraction, which is necessary for the application of the cover-up method.

  • How does the cover-up method simplify the process of solving for unknowns in partial fraction decomposition?

    -The cover-up method simplifies the process by allowing you to multiply by a specific factor and then substitute a value for x that zeroes out all but one term, making it easy to solve for the unknown coefficients without expanding and equating coefficients.

  • What is the first step in applying the cover-up method?

    -The first step is to ensure the denominator of the rational function is in factored form and then to multiply the entire equation by one of these factors.

  • How do you choose the value of x to substitute in the cover-up method?

    -You choose a value of x that zeroes out the factor you are currently focusing on, which simplifies the equation to a point where you can easily solve for the unknown coefficient.

  • What is the purpose of covering up terms in the cover-up method?

    -Covering up terms is a mental process to ignore certain parts of the equation that do not involve the unknown coefficient you are solving for, making it easier to focus on the relevant part of the equation.

  • Can the cover-up method be used with repeated linear factors?

    -The cover-up method is primarily used with non-repeated linear factors. It may not be as straightforward with repeated factors, which require a different approach.

  • What is the final step in the cover-up method after solving for all unknowns?

    -The final step is to substitute the solved values of the unknown coefficients back into the original equation to confirm that the decomposition is correct and to verify the result with a calculator if necessary.

Outlines
00:00
📚 Introduction to the Cover-Up Method in Partial Fractions

The video discusses the cover-up method for partial fraction decomposition, which is effective for non-repeated linear factors. The explanation begins with a standard example, showing how to decompose a rational expression into partial fractions by solving for unknowns A and B. The instructor details the process, including clearing the denominator, expanding terms, and equating coefficients to find the values of A and B.

05:00
📝 Implementing the Cover-Up Method

The video transitions into a practical demonstration of the cover-up method, using the same example. Instead of fully clearing the denominator, the method simplifies the process by selectively clearing factors and substituting convenient values for x to quickly find the coefficients. This method, as shown, can streamline solving for A and B, providing the same results as the longer traditional method.

Mindmap
Keywords
💡Partial Fraction Decomposition
Partial fraction decomposition is a technique used in calculus to break down a complex rational function into simpler fractions. It is integral to the video's theme as it is the main method being discussed and demonstrated. The script provides an example of decomposing a rational function into partial fractions, which simplifies the process of integration.
💡Cover-up Method
The cover-up method is a specific approach to partial fraction decomposition that simplifies the process by strategically choosing values for the variable to eliminate terms. It is central to the video's narrative, as the script walks through its application step by step, showing how it can streamline the decomposition process.
💡Non-repeated Linear Factors
Non-repeated linear factors refer to terms in the denominator of a fraction that are of the form ax + b, where a and b are constants, and the factor is not repeated. The script explains that the cover-up method works effectively with such factors, as they are the simplest form and do not require further factorization.
💡Coefficients
In the context of the script, coefficients are the numerical or constant terms that multiply the variables in an algebraic expression. The video discusses equating coefficients from both sides of an equation derived from the partial fraction decomposition process to solve for unknowns in the fractions.
💡System of Equations
A system of equations arises when multiple equations are used to solve for multiple unknowns simultaneously. The script describes how, after equating coefficients, one ends up with a system of equations that can be solved to find the values of the unknowns in the partial fractions.
💡Common Denominator
The common denominator is the least common multiple of the denominators of several fractions, which allows for the addition or combination of fractions. In the script, the common denominator is used initially to clear the fractions before applying the cover-up method.
💡Factored Form
Factored form refers to expressing a polynomial as a product of its factors. The script emphasizes the importance of having the denominator in factored form before applying the cover-up method, as it simplifies the process of identifying and solving for the unknowns.
💡Substitution
Substitution is a mathematical technique where a value is replaced with another in an equation or expression. The script uses substitution strategically to simplify the process of finding the unknowns in the partial fractions by setting the variable to values that zero out certain terms.
💡Equating Coefficients
Equating coefficients is the process of setting the coefficients of corresponding terms in two expressions equal to each other to find the values of unknowns. In the script, this technique is used after expanding both sides of the equation to derive a system of equations that can be solved.
💡Integration
Although not explicitly mentioned in the script, the ultimate goal of partial fraction decomposition is often to simplify the process of integration in calculus. The script implicitly relates to integration by demonstrating how to break down complex rational functions into simpler components that are easier to integrate.
💡Zero of a Factor
The zero of a factor is the value of the variable that makes the factor equal to zero. The script discusses how choosing the zero of a factor as the value for the variable simplifies the equation during the cover-up method, allowing for the direct calculation of the unknowns.
Highlights

Introduction to the cover-up method for partial fraction decomposition.

Explanation of non-repeated linear factors, such as ax + b.

Demonstration of the cover-up method on a simple rational expression.

Clearing the denominator to simplify the equation for unknowns.

Expanding and rearranging terms to compare coefficients.

Solving the system of equations for the coefficients a and b.

Substituting values of a and b back into the original equation.

Using a calculator to confirm the results of partial fraction decomposition.

Revisiting the problem with the cover-up method for a different approach.

Making a clever substitution by setting X to the zero of a factor.

Solving for a by covering up and substituting X with 1/3.

Repeating the process to solve for b by setting X to -1/2.

Illustrating the cover-up method with a more complex example.

Solving for a by focusing on the factor 3x - 1 and substituting X with 1/3.

Determining the value of a and then solving for b with a similar method.

Applying the cover-up method to find the value of c in a three-part fraction.

Finalizing the decomposition with values of a, b, and c.

Highlighting the efficiency of the cover-up method in calculus courses.

Encouraging the use of the cover-up method for quick problem-solving.

Transcripts

Browse More Related Video

Partial Fraction Decomposion

Integration By Partial Fractions

Partial Fractions Decomposition simplified

Ch. 10.7 Partial Fractions

AP Calculus BC Lesson 6.12

7.3.6 Partial Fraction Decomposition

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Partial FractionsCalculus MethodCover-Up TechniqueAlgebraic DecompositionMathematics TutorialFractional DecompositionEducational ContentSolving EquationsMathematical SimplificationCalculus Tips