AP Calculus BC Lesson 6.12

Elizabeth Fein

5 Nov 202230:37

EducationalLearning

32 Likes 10 Comments

TLDRThis video delves into the mathematical technique of partial fraction decomposition, essential for integrating complex rational functions where the numerator's degree is less than the denominator's. The process is demonstrated through step-by-step examples, showing how to break down integrals into simpler fractions, solve for coefficients, and ultimately evaluate the integrals using logarithmic properties. The video also touches on the application of partial fraction decomposition in solving definite integrals, emphasizing the importance of understanding logarithmic properties for simplification and problem-solving.

Takeaways

📚 Partial fraction decomposition is an integration technique used for integrals where the numerator's degree is less than the denominator's.
🔍 The technique involves rewriting the integral as the sum of simpler fractions, which can be integrated separately.
📐 When the degree of the numerator is less than the denominator, long division is not applicable and partial fraction decomposition must be used.
🤔 The process requires factoring the denominator and expressing the integral as a sum of fractions over the factored terms.
🔢 To solve for the coefficients (A, B, etc.), the equation is manipulated and specific values of x are chosen to eliminate certain terms.
🧩 Once coefficients are determined, the original integral can be split into separate integrals, simplifying the integration process.
📈 The video provides examples of how to use partial fraction decomposition for both indefinite and definite integrals.
🌟 Logarithm properties are crucial for simplifying the results of the integrals after applying partial fraction decomposition.
📊 The video includes a step-by-step breakdown of how to solve for coefficients and how to evaluate the integrals using logarithmic rules.
💡 The method can be applied to more complex integrals with multiple terms in the denominator by introducing additional coefficients and fractions.
🎓 Understanding and applying U-substitution and logarithmic properties are key to successfully using partial fraction decomposition in integration.

Q & A

What is the main topic of the video?
-The main topic of the video is the explanation of a new integration technique called partial fraction decomposition.
Why is partial fraction decomposition used in this case?
-Partial fraction decomposition is used when the degree of the numerator is less than the degree of the denominator, allowing for successful integration where long division would not be applicable.
How does the video demonstrate the process of partial fraction decomposition?
-The video demonstrates the process by first identifying a factorable denominator, then setting up an equation with variables A and B, and solving for these variables by choosing specific values for x that simplify the equation.
What is the significance of choosing specific values for x in the process of partial fraction decomposition?
-Choosing specific values for x helps to eliminate certain terms in the equation, making it easier to solve for the variables A and B, which are necessary to rewrite the integral in a form that can be evaluated.
How does the video handle the integral of a fraction with a factored denominator?
-The video shows that the integral can be split into separate fractions with simpler denominators, which can then be integrated individually using techniques such as u-substitution or direct evaluation of the natural logarithm.
What is the role of logarithm properties in solving integrals using partial fraction decomposition?
-Logarithm properties are crucial for simplifying the results of the integrals after they have been evaluated. They allow for the combination and simplification of terms involving natural logs, making the final answer more concise and in a form that matches standard mathematical expressions.
How does the video approach the solution for a, b, and d in the partial fraction decomposition?
-The video approaches the solution by setting up equations for a, b, and d based on the factored form of the denominator, then solving for each variable by assigning values to x that make certain terms of the equation equal to zero.
What is the purpose of the multiple-choice questions presented in the video?
-The multiple-choice questions serve as practical examples to demonstrate the application of partial fraction decomposition in solving integrals. They also help to reinforce the concepts and techniques discussed in the video.
How does the video address the evaluation of definite integrals using partial fraction decomposition?
-The video addresses the evaluation of definite integrals by first setting up the integral with the appropriate bounds, then applying partial fraction decomposition to simplify the integrand, and finally using logarithm properties to simplify and evaluate the resulting expressions.
What is the final result of the definite integral example given in the video?
-The final result of the definite integral example is the natural log of (2 rad 6 over 3) plus the natural log of (2/3), which matches answer choice A in the provided multiple-choice question.

Outlines

00:00

📚 Introduction to Partial Fraction Decomposition

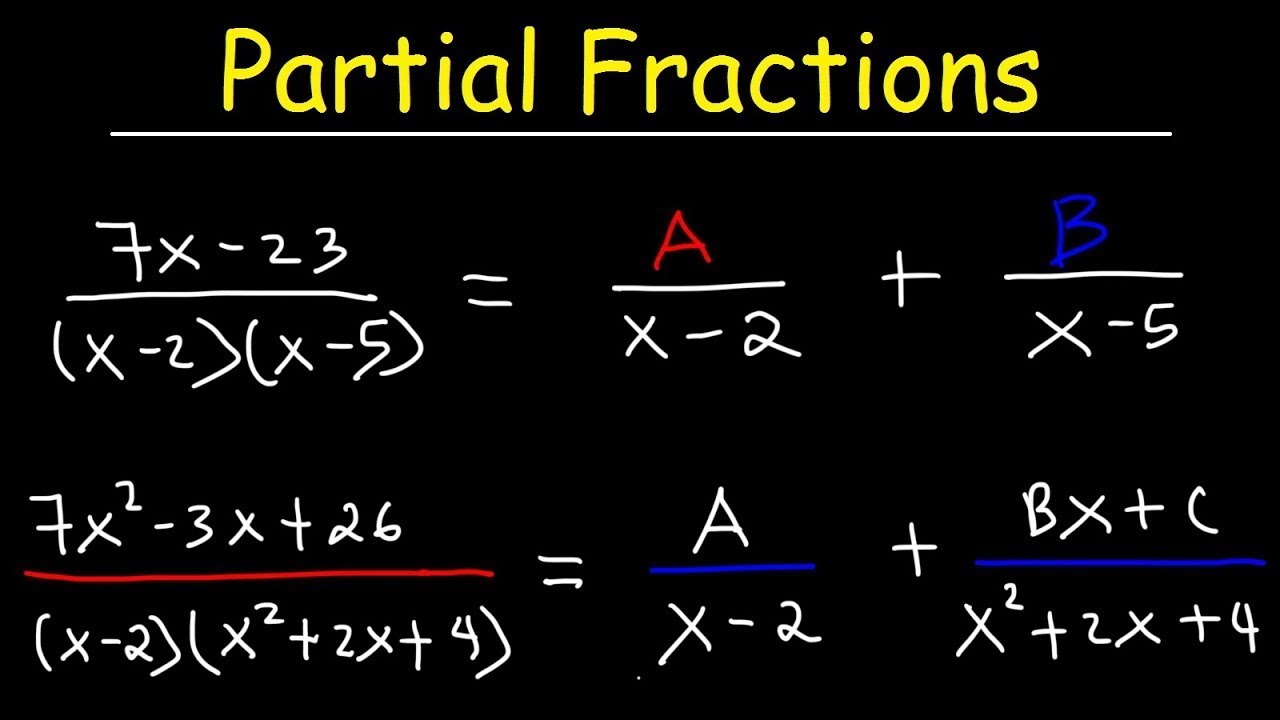
This paragraph introduces the concept of partial fraction decomposition, an integration technique used to rewrite complex integrals into simpler forms. The speaker explains that this method is applicable when the degree of the numerator is less than the degree of the denominator, contrasting it with situations where long division could be used. The paragraph details the process of factoring the denominator and splitting the integral into parts that can be integrated separately, emphasizing the importance of solving for constants A and B.

05:01

🧠 Solving for A and B with Partial Fractions

The speaker continues to delve into the process of using partial fraction decomposition by demonstrating how to solve for the constants A and B. This involves setting up equations based on the integral and using specific values for x to eliminate certain terms, ultimately leading to the values of A and B. The paragraph highlights the use of logarithmic properties and the importance of understanding these properties for simplifying the final answer, especially in the context of multiple-choice questions.

10:02

📈 Evaluating Integrals with Partial Fraction Decomposition

This paragraph focuses on the practical application of partial fraction decomposition to evaluate definite integrals. The speaker shows how to isolate integrands, factor denominators, and use the method to rewrite the integral into a form that can be easily evaluated. The explanation includes the use of U-substitution when dealing with non-integer coefficients and the manipulation of logarithmic expressions to match answer choices.

15:02

🔢 Solving for A, B, and D with a Tripartite Denominator

The speaker tackles a more complex scenario where the denominator has three terms, requiring the use of three constants (a, b, and d) for the partial fraction decomposition. The process of solving for these constants is explained, including the selection of x values that will eliminate certain terms and the multiplication of the entire equation by the denominator. The paragraph emphasizes the step-by-step approach to solving and the final integration of the individual terms.

20:04

📊 Complex Integral Evaluation with Logarithmic Simplification

In this paragraph, the speaker addresses a complex integral with a factored denominator that leads to a tripartite decomposition. The process of solving for the constants a, b, and d is detailed, followed by the evaluation of the definite integral. The speaker demonstrates the use of logarithmic properties to simplify the expression and rationalize the denominator to match the format of the provided answer choices, ultimately arriving at the correct answer.

Mindmap

Keywords

💡Partial Fraction Decomposition

Partial Fraction Decomposition is an integration technique used to break down a complex fraction into simpler fractions. This method is particularly useful when the degree of the numerator is less than the degree of the denominator, which allows for easier integration. In the video, this technique is applied to integrals that cannot be simplified using long division, such as when the denominator is factorable into different linear factors.

💡Integration

Integration is a fundamental process in calculus that involves finding the antiderivative of a function, which gives the area under the curve of that function. In the context of the video, integration is the goal, and the method of partial fraction decomposition is used to simplify the integral process for certain types of fractions.

💡Degree of Numerator and Denominator

The degree of a polynomial is the highest power of the variable present in the polynomial. In the context of the video, the degree of the numerator refers to the highest power of x in the numerator of the fraction, and the degree of the denominator refers to the highest power of x in the denominator. The technique of partial fraction decomposition is applicable when the degree of the numerator is less than the degree of the denominator.

💡Long Division

Long division in the context of algebra refers to the process of dividing one polynomial by another. In the video, it is mentioned that long division cannot be used when the degree of the numerator is less than the degree of the denominator, which is when partial fraction decomposition becomes the preferred method.

💡Factorable Denominator

A factorable denominator is one that can be expressed as the product of two or more factors. In the video, the ability to factor the denominator is crucial for applying the partial fraction decomposition technique. The process involves breaking down the original integral into simpler parts that can be integrated separately.

💡Equation Solving

Equation solving involves finding the values of variables that make a given equation true. In the video, solving for the coefficients A and B in the partial fraction decomposition equation is necessary to rewrite the original integral into a sum of simpler integrals. This process involves multiplying the equation by the denominator and then using specific values for x to isolate and solve for A and B.

💡Natural Logarithm

The natural logarithm, often denoted as ln, is a logarithm to the base e (where e is the mathematical constant approximately equal to 2.71828). It is a fundamental concept in calculus, particularly in integration, and is used to find the area under the curve of an exponential function. In the video, natural logarithms are used to evaluate the integrals after partial fraction decomposition.

💡Logarithm Properties

Logarithm properties are a set of rules that describe how logarithms can be manipulated and combined. These properties include the product rule (log(a*b) = log(a) + log(b)), the quotient rule (log(a/b) = log(a) - log(b)), and the power rule (log(a^n) = n*log(a)). These properties are essential for simplifying and evaluating logarithmic expressions, especially in integration problems.

💡U-Substitution

U-Substitution is a technique used in integration to simplify the process by changing the variable of integration. It involves setting u equal to a function of the original variable (x), and then changing the differential dx to du/d(x), which allows for the integral to be rewritten in terms of u. This method is particularly useful when the integral involves the derivative of the integrand, making the process easier.

💡Definite Integral

A definite integral represents the precise area under a curve of a function over a specified interval. It is denoted by the integral symbol with the function, the lower limit of integration, and the upper limit of integration. In the video, the concept of definite integrals is used to evaluate the area under the curve of a function between two specific x-values.

💡Antiderivatives

Antiderivatives, also known as indefinite integrals, are functions that have a derivative equal to the integrand. In the process of integration, the goal is often to find the antiderivative of a given function. The Fundamental Theorem of Calculus establishes the relationship between differentiation and integration, stating that the antiderivative of a function can be found by evaluating its integral.

Highlights

The video introduces a new integration technique called partial fraction decomposition.

Partial fraction decomposition allows rewriting an integral with a numerator of lower degree than the denominator for successful integration.

Long division is not applicable in this case due to the degree of the numerator being less than the denominator.

The denominator is factorable, which is a key step in applying the partial fraction decomposition technique.

The process involves rewriting the integral as the sum of two separate fractions to facilitate individual integration.

The values of A and B in the partial fraction decomposition are determined by plugging in specific values of x.

Once A and B are determined, the original integrand can be rewritten and integrated term by term.

The use of logarithm properties is crucial for simplifying the results of the integration process.

The video demonstrates how to solve for A and B using algebraic manipulation and substitution of values.

The integral can be simplified using U-substitution when the coefficient of x is one.

The video provides a step-by-step guide on how to apply partial fraction decomposition to solve complex integrals.

The technique can be used to evaluate definite integrals as well as indefinite integrals.

The video emphasizes the importance of understanding logarithm properties for solving multiple-choice questions.

The process of partial fraction decomposition is demonstrated with multiple examples to reinforce understanding.

The video concludes with a method for rationalizing the denominator in the final answer to match the answer choices.

Transcripts

Browse More Related Video

Unit VI: Lec 2 | MIT Calculus Revisited: Single Variable Calculus

Partial Fraction Decomposition - The Integration

7.3.6 Partial Fraction Decomposition

Integration By Partial Fractions

how to setup partial fractions (all cases)

Partial Fraction Decomposion

Related Tags

Mathematics Integration Partial Fractions Logarithmic Properties Algebra Calculus Educational Content Problem Solving Video Tutorial Math Techniques