Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (7 of 92) The Schrodinger Eqn. in 1-D (3/3)

Michel van Biezen
30 Jan 201704:20
EducationalLearning
32 Likes 10 Comments

TLDRIn this third part of a series, the video script explains the derivation and validation of the Schrödinger equation for a single particle in one dimension. Starting with the presumed form of the equation, the script takes the first and second derivatives with respect to time and position, leading to the canonical form of the Schrödinger equation. By substituting the expressions for momentum and energy of a photon, the script demonstrates that the derived equation simplifies to the basic energy-momentum relation, thus confirming its correctness as the proper differential equation describing a single particle's behavior in one dimension.

Takeaways
  • 🌟 The series aims to derive an equation describing a single particle in one dimension.
  • 📈 In the first part, three equations for a photon's energy and momentum in terms of h-bar, Omega, and K were established.
  • 🎥 The second video introduced a potential form of the Schrödinger equation, e^(i(Kx - Ωt)/h-bar).
  • 🔄 The third part focuses on confirming the correct form of the Schrödinger equation through mathematical derivations.
  • 📚 The process involves taking the first and second derivatives with respect to time and position.
  • 📶 The first derivative yields -iΩ times the original function, simplifying to -iΩψ.
  • 🔢 The second derivative results in -K² times the original function, simplifying to -K²ψ.
  • 🔄 By substituting these back into the presumed differential equation, the equation simplifies to h-bar squared times K squared over 2m times the function plus potential energy times the function equals h-bar Omega times i times the function.
  • 🌐 This simplification aligns with the basic energy equation for a particle, confirming the Schrödinger equation's correctness.
  • 📝 The final form of the Schrödinger equation for a single particle with mass m in one dimension is established.
  • 🔑 The equation can be represented in differential form or as an equivalent format, both confirming the total energy of a particle as the sum of kinetic and potential energy.
Q & A

  • What is the main goal of the three-part series discussed in the transcript?

    -The main goal is to derive an equation that describes a single particle in one dimension.

  • What were the three equations developed in the first part of the series?

    -The three equations describe the energy and momentum of a photon in terms of h-bar Omega K P and C H bar, which involves Planck's constant divided by 2 pi.

  • What form did the Schrödinger equation take in the second part of the series?

    -The Schrödinger equation was in the form of some constant times e to the power of another constant I times KX minus Omega T.

  • How does the first derivative of the Schrödinger equation with respect to time simplify?

    -The first derivative simplifies to minus I Omega times the original function.

  • What does the second derivative of the Schrödinger equation with respect to position yield?

    -The second derivative yields minus K squared times the original function.

  • How does the presumed Schrödinger equation relate to the total energy of a particle?

    -The total energy of a particle is equal to the kinetic energy plus the potential energy, which is proven by dividing the derived equation by the Schrödinger equation and simplifying it back to the basic energy equation.

  • What does the momentum of the photon represent when squared and compared to the energy of the particle?

    -When h-bar K is squared, it represents the momentum squared, and h-bar times Omega can be replaced by the energy of the particle, showing the relationship between momentum and energy.

  • What is the significance of the final form of the Schrödinger equation derived in the video?

    -The final form of the Schrödinger equation represents the proper differential equation format for a single particle with mass m in one dimension, confirming its correctness.

  • How does the process of taking derivatives and substituting values help in validating the Schrödinger equation?

    -By taking derivatives and substituting values, the process allows for the validation of the equation by showing that it simplifies back to the original energy equation, thus proving its correctness.

  • What is the role of Planck's constant in the equations discussed in the series?

    -Planck's constant, represented by h-bar, is a fundamental constant that appears in the equations for the energy and momentum of a photon, and is also used in the Schrödinger equation to relate the particle's energy and momentum.

  • How does the video script demonstrate the relationship between the differential equation and its alternative format?

    -The script shows that both the differential equation and its alternative format can be used to describe the single particle in one dimension, as they both lead to the same fundamental energy equation when simplified.

Outlines
00:00
📝 Introduction to the Schrödinger Equation

This paragraph introduces the third part of a series aimed at deriving an equation that describes a single particle in one dimension. It recaps the previous two parts, where three equations describing the energy and momentum of a photon were developed using Planck's constant and other constants. The focus now shifts to validating the presumed form of the Schrödinger equation by taking its first and second derivatives with respect to time and position, leading to a deeper understanding of its structure and relevance in quantum mechanics.

Mindmap
Keywords
💡Elektra Line
Elektra Line seems to be the name of the series or the show in which this video transcript is taken from. It is the context within which the speaker discusses the development of a surfer equation. The name 'Elektra Line' is not a scientific term but rather a title or a branding element for the series, which is focused on physics and mathematical equations.
💡Schrödinger Equation
The Schrödinger Equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. In this video, the speaker is working on deriving an equation similar to the Schrödinger Equation for a single particle in one dimension. The equation is central to the theme of the video, as it is the culmination of a three-part series aimed at developing a quantum mechanical description of a particle's behavior.
💡One Dimension
One dimension refers to the simplification of physical problems by considering motion or existence within a single, linear direction. In the context of this video, the speaker is focusing on a single-particle system that is confined to move or exist along a single line, which simplifies the complex, three-dimensional reality into a more manageable one-dimensional model.
💡Photon
A photon is a particle representing a quantum of light or other electromagnetic radiation. It has zero mass and is the carrier of electromagnetic force. In the video, the speaker discusses the energy and momentum of a photon, using it as an example to build up to the main topic of the Schrödinger Equation for a single particle.
💡h-bar
h-bar, or reduced Planck's constant, is a fundamental constant in quantum mechanics that is equal to Planck's constant divided by 2π (h/2π). It is used in various quantum mechanical equations, including those for the energy and momentum of particles. In the context of the video, h-bar is a key component in the equations that describe the photon's energy and momentum.
💡Energy
In the context of this video, energy refers to the property of a physical system that is associated with the capacity to do work. Specifically, the speaker discusses the energy of a photon and later relates it to the energy of a single particle in one dimension. Energy is a fundamental concept in physics and is central to understanding the behavior of particles at the quantum level.
💡Momentum
Momentum is the product of an object's mass and velocity and is a key concept in physics that describes the motion of an object. In quantum mechanics, the momentum of particles like photons is quantized, meaning it can only take on certain discrete values. The video uses the concept of momentum to derive equations and later connects it to the Schrödinger Equation.
💡Derivative
In calculus, a derivative is a measure of how a function changes with respect to its input variable. The first derivative gives the rate of change or the slope of the function, while higher-order derivatives provide more detailed information about the function's behavior. In the video, the speaker takes derivatives of the presumed Schrödinger Equation with respect to time and position to validate its form.
💡Exponential Function
An exponential function is a mathematical function where the base is a constant and the exponent is the variable. In the context of the video, the exponential function is part of the presumed form of the Schrödinger Equation. The properties of exponential functions are important in understanding how the equation behaves under differentiation.
💡Potential Energy
Potential energy is the stored energy of a system, which has the potential to be converted into kinetic energy. It is dependent on the position of objects relative to each other and other factors. In the video, potential energy is mentioned as a term that multiplies the function in the derived equation, indicating its role in the overall energy of the system.
💡Total Energy
Total energy in physics is the sum of all forms of energy in a system, including kinetic and potential energy. In the video, the speaker aims to show that the derived equation simplifies back to the basic equation representing the total energy of a particle, which is the sum of its kinetic and potential energy.
Highlights

The series aims to develop an equation that describes a single particle in one dimension.

In the first part, three equations describing the energy and momentum of a photon were derived using h-bar Omega K P and C H bar.

The second video presented a form of the Schrödinger equation involving e to the power of (I times KX minus Omega T).

This video demonstrates that the presumed Schrödinger equation is indeed the correct form.

Derivatives of the Schrödinger equation with respect to time and position were calculated to verify its correctness.

The first derivative with respect to time yields a function multiplied by minus I Omega.

The second derivative with respect to position results in a function multiplied by minus K squared.

The results of the derivatives, when plugged back into the presumed differential equation, lead to a relation involving h-bar squared case squared over 2m times the function plus potential energy times the function.

The equation simplifies to show that the energy of the particle is equal to the momentum of the particle divided by twice the mass.

The Schrödinger equation can be rewritten using the momentum and energy of the particle.

The equation is proven correct by simplifying it back to the basic equation of total energy of a particle.

The final form of the Schrödinger equation for a single particle in one dimension with mass m is established.

The proper differential equation format of the Schrödinger equation is presented.

The process confirms the validity of the Schrödinger equation through mathematical derivation and simplification.

The video series provides a step-by-step approach to understanding the Schrödinger equation.

The highlights showcase the development and verification of the Schrödinger equation in a clear and concise manner.

The series is a valuable resource for those interested in quantum mechanics and the mathematical foundations of particle physics.

Transcripts

Browse More Related Video

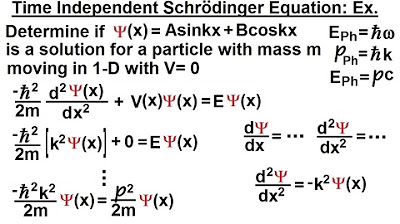
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (15 of 92) Time & Position Dependencies Ex.*

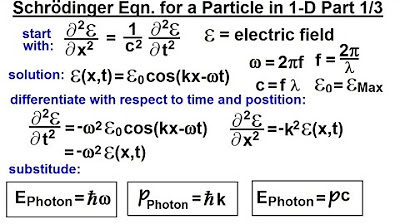
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (5 of 92) The Schrodinger Eqn. in 1-D (1/3)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (14 of 92) Time & Position Dependencies 3/3

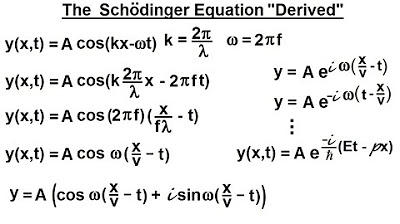
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (4 of 92) The Schrodinger Eqn. "Derived"

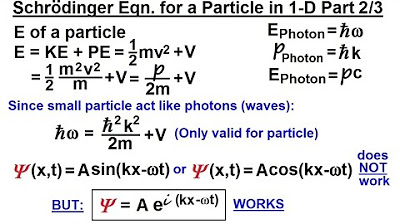
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (6 of 92) The Schrodinger Eqn. in 1-D (2/3)

Schrodinger Equation - A simple derivation

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsSchrodingerEquationParticlePhysicsOneDimensionEnergyMomentumPhysicsEducationDifferentialEquationsPhotonBehaviorMathematicalDerivationQuantumTheory