First Principles Calculus Grade 12
TLDRThis video script focuses on demystifying a challenging new formula introduced in grade 12, emphasizing practical application over theoretical background. The instructor guides students through breaking down the formula into manageable parts, illustrating the process of finding derivatives using the limit concept. By practicing the steps of replacing 'x' with 'x + h', multiplying, and simplifying, students are shown how to isolate terms with 'h' to eventually cancel them out, leading to the derivative. The script highlights the importance of correctly applying the limit process to avoid errors and concludes with the final derivative formula, emphasizing its significance as a measure of gradient.
Takeaways
- 📚 The script introduces a new formula in grade 12 that students often find challenging initially.
- 🤔 The mathematical background of the formula is complex and not the focus of the explanation due to students' time constraints.
- 👨🏫 The teacher aims to teach students how to use the formula for tests rather than its historical or theoretical aspects.
- 🔍 Students are advised to break the formula into smaller parts to simplify understanding and application.
- 📝 The process involves replacing 'x' with 'x + h' in the original formula and expanding the expression carefully.
- 🚫 Caution is given against incorrectly squaring terms when expanding, emphasizing the importance of proper algebraic expansion.
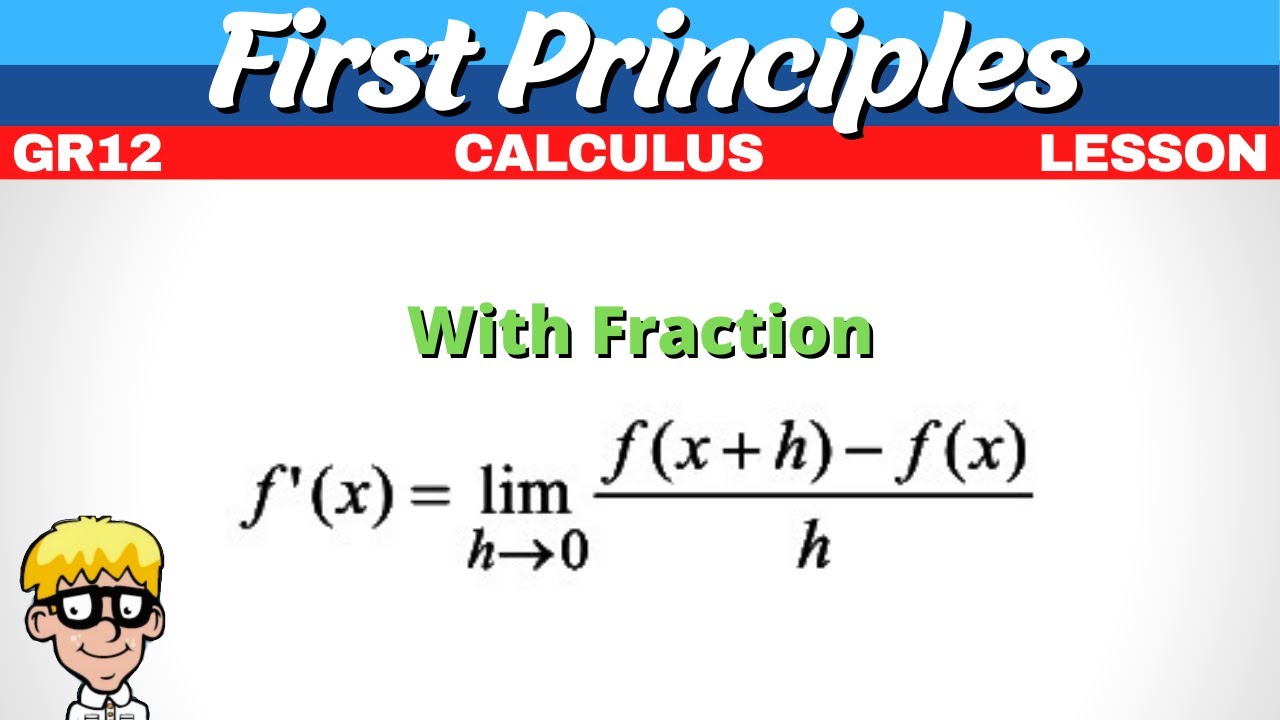
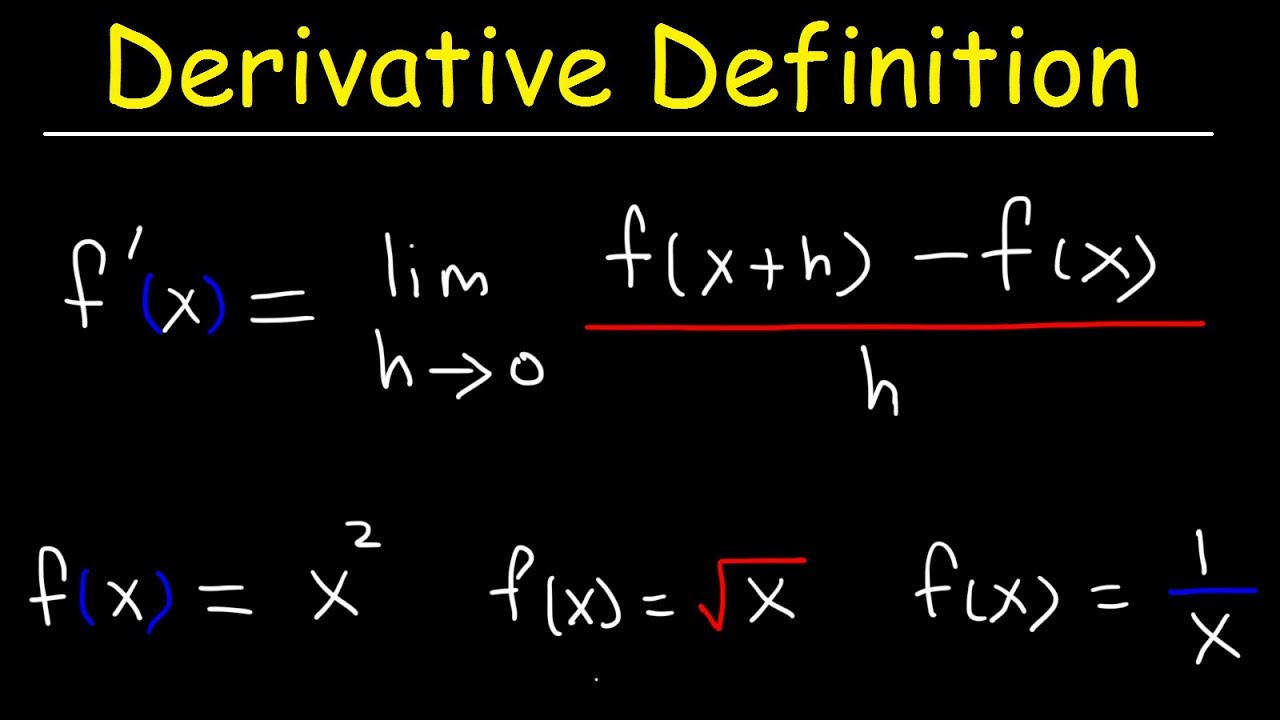
- 🔢 The formula is used to find the derivative of f(x), and the limit as h approaches zero is a crucial part of the process.
- ✅ The cancellation of terms without 'h' is an essential step to simplify the expression before taking the limit.
- 📉 The final simplification should leave terms with 'h' only, allowing for the extraction of 'h' as a common factor.
- 🎯 The limit process simplifies to a point where the value of 'h' can be substituted with zero without causing division by zero.
- 🏁 The final answer for the derivative is 2x - 3, and it's important not to misinterpret this as an equation to solve for 'x'.
- 🔄 The teacher plans to further explain the concept of the first derivative as the gradient in subsequent lessons.
Q & A
What is the main focus of the video script regarding the new formula introduced in grade 12?
-The main focus of the video script is to teach students how to use the new formula for finding derivatives, rather than delving into the mathematical background of how the formula was derived.
Why does the instructor choose not to explain the mathematical background of the formula?
-The instructor chooses not to explain the mathematical background because students are often not interested in it due to time constraints and the need to study multiple subjects.
What is the first step in using the new formula as per the script?
-The first step is to replace 'x' with 'x plus h' in the original formula wherever it appears.
Why is it important to multiply out the brackets correctly when dealing with 'f of x plus h'?
-It is important to multiply out the brackets correctly to avoid incorrectly squaring the terms, which would lead to an incorrect formulation of 'f of x plus h'.
What does the instructor mean by 'double bracket' in the context of the formula?
-The 'double bracket' refers to the process of multiplying each term inside the parentheses by the term outside, in this case, '(x + h)', to avoid incorrectly squaring the 'x' term.
What is the significance of the term 'f of x plus h' in the derivative formula?
-The term 'f of x plus h' represents the function evaluated at 'x plus h', which is crucial for finding the derivative as it helps in determining the rate of change of the function near the point 'x'.
Why is it necessary to cancel out terms that do not have an 'h' in the derivative formula?
-It is necessary to cancel out terms without an 'h' to simplify the expression and to prepare it for factoring out 'h', which is essential for evaluating the limit as 'h' approaches zero.
What is the purpose of the limit in the derivative formula?
-The purpose of the limit is to find the instantaneous rate of change of the function at a specific point 'x', which is done by letting 'h' approach zero.
Why is it incorrect to solve for 'x' after plugging in the limit value in the derivative formula?
-It is incorrect to solve for 'x' because the limit value is not meant to solve for 'x' but to evaluate the derivative at a specific point, which gives the gradient or slope of the function at that point.
What does the instructor mean by 'first derivative' being a 'fancy word for gradient'?
-The instructor is emphasizing that the first derivative of a function represents the gradient or slope of the function, which is a key concept in calculus and will be important as the course progresses.
What is the final result of the derivative formula as explained in the script?
-The final result of the derivative formula, after canceling terms and evaluating the limit, is '2x - 3'.
Outlines
📚 Understanding the Challenging New Formula
This paragraph introduces a complex formula that students often find difficult in grade 12. The speaker emphasizes the importance of practicing the formula to make it easier to use. The focus is on applying the formula during tests rather than understanding its mathematical derivation, as students are already overwhelmed with multiple subjects. The speaker also mentions a common student technique of breaking down the formula into smaller parts and provides a step-by-step guide on how to do so, including replacing 'x' with 'x + h' and simplifying the expression by multiplying terms and combining like terms. The explanation concludes with a reminder to include the limit notation when writing out the formula for the test.
🔍 Simplifying the Limit Process for Derivatives
In this paragraph, the speaker discusses the process of finding the derivative using limits, a concept that can be confusing at first. The explanation clarifies that initially, you cannot plug in the limit value because 'h' is in the denominator, which would cause an error. However, after simplifying the expression and canceling out terms without 'h', the 'h' in the denominator can be factored out, allowing the limit value to be substituted. The final step is to plug in the limit value (h=0) to obtain the derivative, which in this case results in 2x - 3. The speaker cautions against common mistakes, such as incorrectly solving for 'x' after plugging in the limit value, and emphasizes that the first derivative represents the gradient, a concept that will be further elaborated in upcoming videos.
Mindmap
Keywords
💡Formula
💡Derivative
💡Limit
💡Practice
💡Background
💡Test
💡Original Formula
💡Brackets
💡Like Terms
💡Common Factor
💡Gradient
Highlights
Introduction to a new formula that students often find challenging at first.
Emphasis on practice to make using the formula easier.
Mathematics behind the formula is briefly mentioned but not deeply explained due to time constraints.
Focus on understanding how to use the formula during tests, rather than its derivation.
Strategy suggested: breaking the formula into smaller parts for easier comprehension.
Explanation of f(x + h): replacing x with (x + h) in the original formula.
Important tip: Avoid the common mistake of simplifying (x + h)² as x² + h².
Demonstration of expanding the expression using double brackets.
Step-by-step process of multiplying terms and simplifying the expression.
Crucial check: ensuring that terms without h cancel out; if they don't, an error has occurred.
Introduction to the concept of the limit as h approaches zero.
Detail on how to apply the formula during tests, including the importance of writing equals to lim h -> 0.
Final simplification: canceling out h's to reach the derivative.
Emphasis on the final result of the derivative being 2x - 3.
Clarification that the first derivative represents the gradient, a concept that will be crucial in later discussions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: