First Principles Calculus Grade 12 | With Fraction
TLDRThe video script explains the concept of finding the first derivative using the first principle method. It clarifies that the derivative of a function is the limit of the difference quotient as h approaches zero. The example provided involves a function with a fraction, where the script demonstrates the process of finding a common denominator, simplifying the expression, and eventually canceling out the h's to find the derivative as negative two over x squared. The script emphasizes the importance of correctly handling fractions and the common mistakes students make, such as incorrectly simplifying the expression or failing to cancel out the h's.
Takeaways
- 🧑🏫 Teachers sometimes give tricky first principle questions involving fractions, which can be confusing at first.
- 🧠 Understanding how to determine the first derivative is important and becomes easy once you learn the method.
- 📉 The problem involves finding the derivative using the limit definition as h approaches zero.
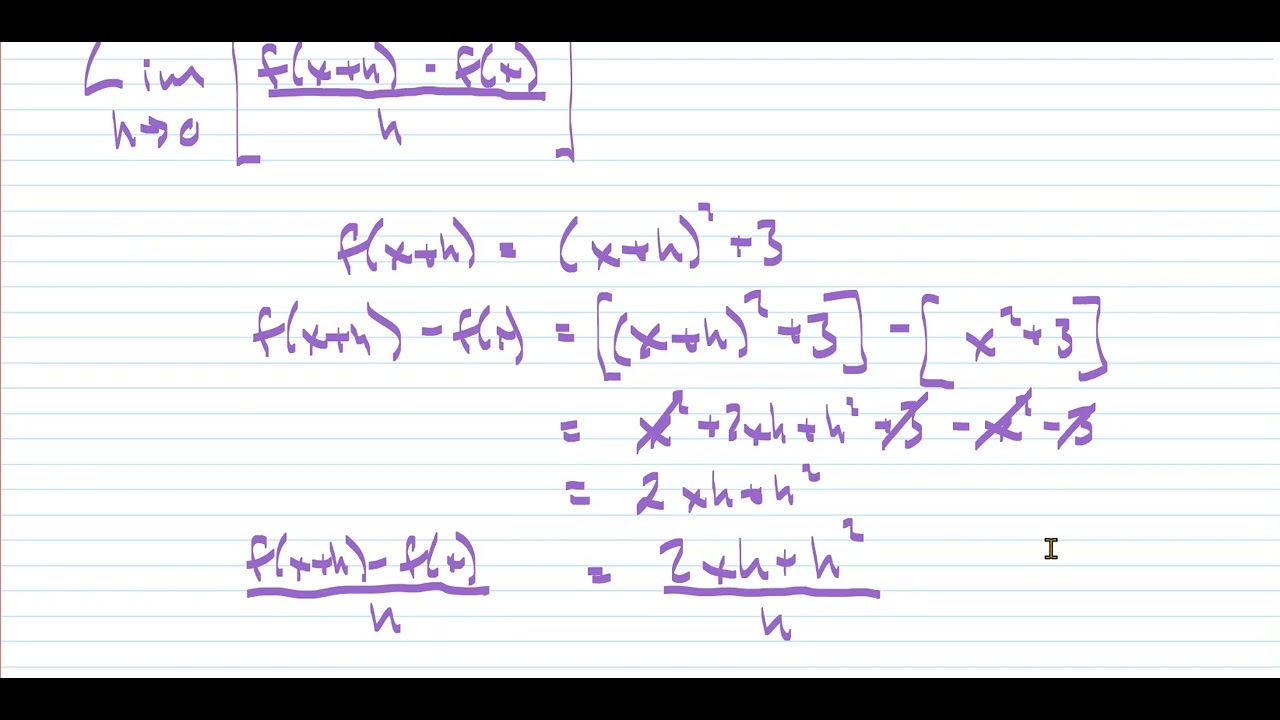
- 🔄 The process starts with substituting x with x + h in the function, followed by calculating the difference between f(x + h) and f(x).
- ✏️ Simplifying the expression involves getting a common denominator for the fractions before performing subtraction.
- ❌ It's important not to multiply out the common denominator; instead, simplify the numerator.
- ↕️ Handling a fraction over another fraction requires flipping the bottom fraction and multiplying.
- ✂️ Canceling out h in the numerator and denominator is a key step before letting h approach zero.
- ➗ The final simplified expression for the derivative is -2 over x squared.
- 📚 The script emphasizes the importance of understanding each step, particularly dealing with limits and fraction manipulation.
Q & A
What is a first principle question in the context of the transcript?
-A first principle question in this context refers to a fundamental question in calculus that involves determining the derivative of a function using the concept of limits, which is a foundational principle in calculus.
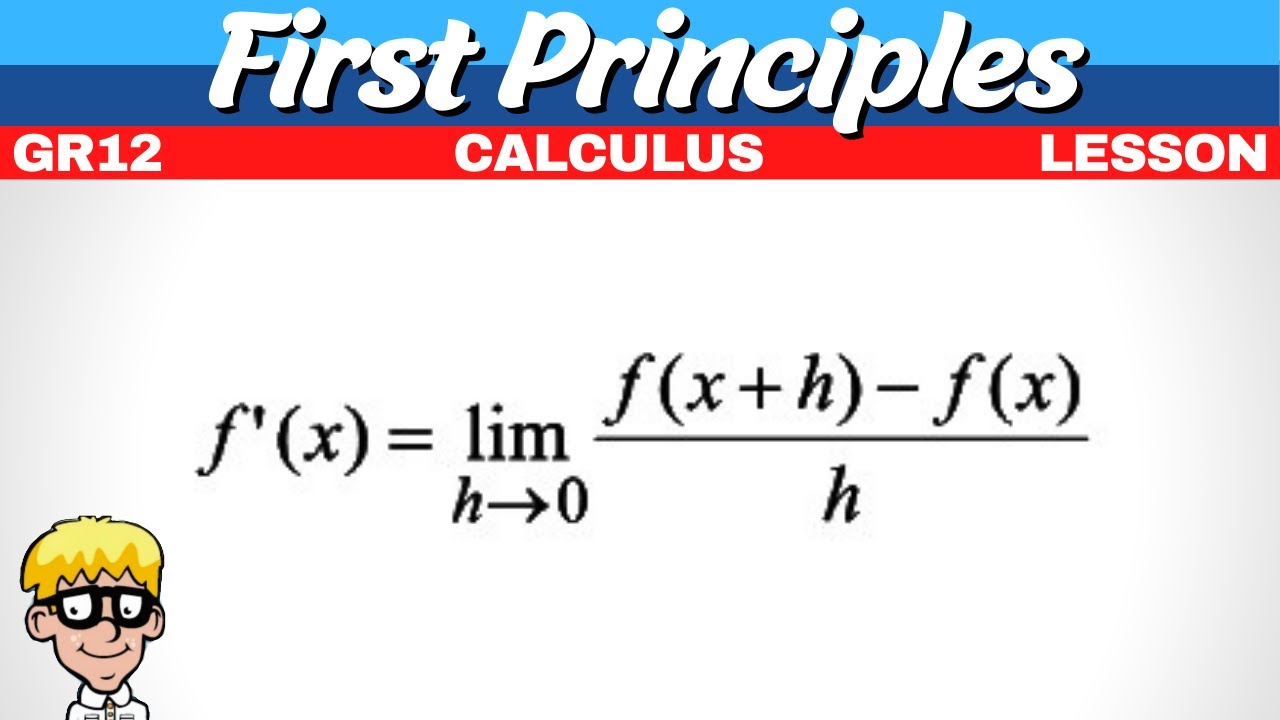
What is the definition of the derivative using the first principle?
-The derivative of a function f(x) is defined using the first principle as the limit of the difference quotient as h approaches zero: \( f'(x) = \lim_{h \to 0} \frac{f(x+h) - f(x)}{h} \).
Why is it important to replace x with x + h in the function when finding the derivative?
-Replacing x with x + h is important because it represents a small change in the input of the function, which is necessary to calculate the rate of change or the slope of the tangent line at a point on the function's graph.
What does the term 'lim' stand for and why is it used in the derivative formula?
-The term 'lim' stands for 'limit'. It is used in the derivative formula to denote the process of letting the change in the input (h) approach zero, which is necessary to find the instantaneous rate of change at a specific point.
Why is finding a common denominator necessary when combining fractions in the derivative formula?
-Finding a common denominator is necessary to combine fractions because it allows you to add or subtract the fractions by having the same base, which is essential for simplifying the expression and finding the limit.
What is the lowest common denominator in the given example of the derivative calculation?
-In the given example, the lowest common denominator is the product of the distinct denominators, which is \( x(x + h) \).
Why is it important to simplify the expression before taking the limit?
-Simplifying the expression before taking the limit is important to make the calculation more manageable and to avoid errors. It also allows for a clearer understanding of the behavior of the function as h approaches zero.
What is the purpose of multiplying the numerators by the missing factors to get a common denominator?
-Multiplying the numerators by the missing factors is done to create a common denominator, which simplifies the process of combining the fractions into a single expression that can be more easily simplified and evaluated as h approaches zero.
Why do the h's in the numerator and the denominator cancel out in the limit expression?
-The h's cancel out because they are common factors in both the numerator and the denominator of the fraction. This simplification is valid as long as h is not zero, which is the case when taking the limit as h approaches zero.
What is the final result of the derivative calculation in the transcript?
-The final result of the derivative calculation in the transcript is \( f'(x) = -\frac{2}{x^2} \).
Why is it recommended to plug in h as 0 first when dealing with limits?
-Plugging in h as 0 first is a quick check to see if the limit can be easily evaluated. However, in the case of the first principle of derivatives, it often results in an indeterminate form (like 0/0), which requires further algebraic manipulation before taking the limit.
Outlines
📚 Introduction to First Principle Derivative Calculation
This paragraph introduces the concept of calculating the first derivative using the first principle, which can be confusing for students who haven't encountered it before. The process involves taking the limit as h approaches zero of the difference quotient, which is the function evaluated at x plus h minus the function at x, all divided by h. The paragraph emphasizes the importance of finding a common denominator when dealing with fractions and the need to simplify the expression before taking the limit. It also touches on the common mistake of trying to plug in h as zero directly into the formula, which doesn't work due to the 0/0 indeterminate form that arises.
Mindmap
Keywords
💡First Principle
💡Derivative
💡Limit
💡Function
💡Fraction
💡Common Denominator
💡Lowest Common Denominator
💡Simplify
💡Multiplying Across
💡Cancel
💡Zero in the Denominator
Highlights
Introduction to first principle questions involving fractions in calculus
Determining the first derivative using the limit definition
Substituting x with x+h to find f(x+h)
Writing the limit as h approaches 0 for the derivative
Simplifying the expression by finding a common denominator
Multiplying numerators and writing over the common denominator
Cancelling out terms to simplify the expression further
Handling fractions on top of fractions by multiplying and flipping
Cancelling h terms to simplify before taking the limit as h approaches 0
Final result of the derivative is -2/x^2
Importance of always writing the limit as h approaches 0
Common struggles students face with first principle questions
Advice on trying to plug in h=0 first and why it often doesn't work
Steps to handle a 0 in the denominator when taking the limit
Using the common denominator approach to combine fractions
Applying the rule for fractions on top of fractions to simplify
Final steps to cancel h and take the limit as h approaches 0
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: